Skip to main content
Springer Nature Link
Log in

Error-Detection-Based Quantum Fault-Tolerance Threshold

  • Published:
Algorithmica Aims and scope Submit manuscript

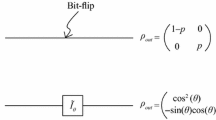
Abstract

A major hurdle in building a quantum computer is overcoming noise, since quantum superpositions are fragile. Developed over the last couple of years, schemes for achieving fault tolerance based on error detection, rather than error correction, appear to tolerate as much as 3–6% noise per gate—an order of magnitude higher than previous procedures. However, proof techniques could not show that these promising fault-tolerance schemes tolerated any noise at all; the distribution of errors in the quantum state has correlations that conceivably could grow out of control.

With an analysis based on decomposing complicated probability distributions into mixtures of simpler ones, we rigorously prove the existence of constant tolerable noise rates (“noise thresholds”) for error-detection-based schemes. Numerical calculations indicate that the actual noise threshold this method yields is lower-bounded by 0.1% noise per gate.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Aharonov, D., Ben-Or, M.: Fault-tolerant quantum computation with constant error rate. In: Proc. 29th ACM Symp. on Theory of Computing (STOC), pp. 176–188 (1997), quant-ph/9906129

  2. Aliferis, P., Cross, A.W.: Subsystem fault tolerance with the Bacon-Shor code. Phys. Rev. Lett. 98, 220502 (2007)

    Article  Google Scholar 

  3. Aaronson, S., Gottesman, D.: Improved simulation of stabilizer circuits. Phys. Rev. A 70, 052328 (2004)

    Article  Google Scholar 

  4. Aliferis, P., Gottesman, D., Preskill, J.: Quantum accuracy threshold for concatenated distance-3 codes. Quantum Inf. Comput. 6, 97–165 (2006)

    MATH  MathSciNet  Google Scholar 

  5. Aliferis, P., Gottesman, D., Preskill, J.: Accuracy threshold for postselected quantum computation. quant-ph/0703264 (2007)

  6. Aharonov, D., Kitaev, A., Preskill, J.: Fault-tolerant quantum computation with long-range correlated noise. Phys. Rev. Lett. 96, 050504 (2006)

    Article  Google Scholar 

  7. Aliferis, P., Terhal, B.M.: Fault-tolerant quantum computation for local leakage faults. Quantum Inf. Comput. 7(139–156) (2007)

    Google Scholar 

  8. Bacon, D.: Operator quantum error correcting subsystems for self-correcting quantum memories. quant-ph/0506023 (2005)

  9. Bravyi, S., Kitaev, A.: Universal quantum computation with ideal clifford gates and noisy ancillas. Phys. Rev. A 71, 022316 (2005)

    Article  MathSciNet  Google Scholar 

  10. Calderbank, A.R., Shor, P.W.: Good quantum error-correcting codes exist. Phys. Rev. A 54, 1098 (1996)

    Article  Google Scholar 

  11. Gottesman, D., Chuang, I.L.: Quantum teleportation is a universal computational primitive. quant-ph/9908010 (1999)

  12. Gottesman, D.: Fault-tolerant quantum computation with local gates. J. Mod. Opt. 47, 333–345 (2000)

    MathSciNet  Google Scholar 

  13. Kitaev, A.Y.: Quantum computations: algorithms and error correction. Russ. Math. Surv. 52, 1191–1249 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  14. Knill, E., Laflamme, R., Zurek, W.H.: Resilient quantum computation: error models and thresholds. Proc. R. Soc. Lond. A 454, 365–384 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  15. Knill, E.: Scalable quantum computation in the presence of large detected-error rates. quant-ph/0312190 (2003)

  16. Knill, E.: Fault-tolerant postselected quantum computation: schemes. quant-ph/0402171 (2004)

  17. Knill, E.: Fault-tolerant postselected quantum computation: threshold analysis. quant-ph/0404104 (2004)

  18. Knill, E.: Quantum computing with realistically noisy devices. Nature 434, 39–44 (2005)

    Article  Google Scholar 

  19. Metodiev, T., Cross, A.W., Thaker, D., Brown, K.R., Copsey, D., Chong, F.T., Chuang, I.L.: Preliminary results on simulating a scalable fault tolerant ion-trap system for quantum computation. In: 3rd Workshop on Non-Silicon Computing (2004)

  20. Poulin, D.: Stabilizer formalism for operator quantum error correction. Phys. Rev. Lett. 95, 230504 (2005)

    Article  Google Scholar 

  21. Reichardt, B.W.: Improved ancilla preparation scheme increases fault-tolerant threshold. quant-ph/0406025 (2004)

  22. Reichardt, B.W.: Improved magic states distillation for quantum universality. Quantum Inf. Process. 4, 251–264 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  23. Reichardt, B.W.: Error-detection-based quantum fault tolerance against discrete Pauli noise. PhD thesis, University of California, Berkeley (2006), quant-ph/0612004

  24. Reichardt, B.W.: Fault-tolerance threshold for a distance-three quantum code. In: Proc. ICALP. LNCS, vol. 4051, pp. 50–61 (2006), quant-ph/0509203

  25. Reichardt, B.W.: Quantum universality by distilling certain one- and two-qubit states with stabilizer operations. quant-ph/0608085 (2006)

  26. Svore, K.M., Cross, A.W., Chuang, I.L., Aho, A.V.: A flow-map model for analyzing pseudothresholds in fault-tolerant quantum computing. quant-ph/0508176 (2005)

  27. Shor, P.W.: Fault-tolerant quantum computation. In: Proc. Symp. on the Foundations of Computer Science (FOCS) (1996), quant-ph/9605011

  28. Svore, K.M., Terhal, B.M., DiVincenzo, D.P.: Local fault-tolerant quantum computation. Phys. Rev. A 72, 022317 (2005)

    Article  Google Scholar 

  29. Steane, A.M.: A fast fault-tolerant filter for quantum codewords. quant-ph/0202036 (2002)

  30. Steane, A.M.: Overhead and noise threshold of fault-tolerant quantum error correction. Phys. Rev. A 68, 042322 (2003)

    Article  Google Scholar 

  31. von Neumann, J.: Probabilistic logic and the synthesis of reliable organisms from unreliable components. In: Shannon, C.E., McCarthy, J. (eds.) Automata Studies, pp. 43–98. Princeton University Press, Princeton (1956)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute for Quantum Information, California Institute of Technology, Pasadena, CA, 91125, USA

    Ben W. Reichardt

Authors
  1. Ben W. Reichardt
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Ben W. Reichardt.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Reichardt, B.W. Error-Detection-Based Quantum Fault-Tolerance Threshold. Algorithmica 55, 517–556 (2009). https://doi.org/10.1007/s00453-007-9069-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00453-007-9069-7

Keywords