Abstract
We study two local feedback equivalence problems for a nonlinear control-affine system with two nested, controlled-invariant, embedded submanifolds in its state space. The first, less restrictive, result gives necessary and sufficient conditions for the dynamics of the system restricted to the larger submanifold and transversal to the smaller submanifold to be linear and controllable. This normal form facilitates designing controllers that locally stabilize the smaller set relative to the larger set. The second, more restrictive, result additionally imposes that the transversal dynamics to the larger set be linear and controllable. This result can simplify designing controllers to locally stabilize the larger submanifold. This is illustrated by sufficient conditions under which these normal forms can be used to locally solve a nested set stabilization problem.


Similar content being viewed by others
References
Banaszuk A, Hauser J (1995) Feedback linearization of transverse dynamics for periodic orbits in \({\mathbb{R}}^3\) with points of transverse controllability loss. Syst Control Lett 26(3):185–193
Brockett RW (1978) Feedback invariants for nonlinear systems. In: Proceedings of the IFAC world congress, Helsinki, pp 1115–1120
Cartan E (1914) Sur l’équivalence absolue de certains systèmes d’équations différentielles et sur certaines familles de courbes. Bull Soc Math France 42:12–48
Consolini L, Maggiore M, Nielsen C, Tosques M (2010) Path following for the PVTOL aircraft. Automatica 46(8):1284–1296
Doosthoseini A, Nielsen C (2013) Coordinated path following for a multi-agent system of unicycles. In: 52nd IEEE conference on decision and control, Florence, pp 2894–2899
Doosthoseini A, Nielsen C (2015) Coordinated path following for unicycles: a nested invariant sets approach. Automatica 60:17–29
Dörfler F, Bullo F (2014) Synchronization in complex networks of phase oscillators: a survey. Automatica 50(6):1539–1564
El-Hawwary M, Maggiore M (2010) Reduction principles and the stabilization of closed sets for passive systems. IEEE Trans Autom Control 55(4):982–987
El-Hawwary MI, Maggiore M (2007) Passivity-based stabilization of non-compact sets. In: 46th IEEE conference on decision and control, New Orleans, pp 1734–1739
Gardner R, Shadwick W (1990) Feedback equivalence for general control systems. Syst Control Lett 15(1):15–23
Gardner R, Shadwick W (1992) The GS algorithm for exact linearization to Brunovsky normal form. IEEE Trans Autom Control 37(2):224–230
Guay M (1999) An algorithm for orbital feedback linearization of single-input control affine systems. Syst Control Lett 38(4):271–281
Hermann R (1982) The theory of equivalence of Pfaffian systems and input systems under feedback. Math Syst Theory 15:343–356
Isidori A (1995) Nonlinear control systems, 3rd edn. Springer, New York
Isidori A (1999) Nonlinear control systems II. Springer, New York
Isidori A, Krener A (1982) On feedback equivalence of nonlinear systems. Syst Control Lett 2(2):118–121
Isidori A, Ruberti A (1984) On the synthesis of linear input-output responses for nonlinear systems. Syst Control Lett 4(1):17–22
Krener AJ (1973) On the equivalence of control systems and the linearization of nonlinear systems. SIAM J Control Optim 11(4):670–676
Krener AJ, Isidori A, Respondek W (1983) Partial and robust linearization by feedback. In: 22nd IEEE conference on decision and control, San Antonio, pp 126–130
Lee J (2002) Introduction to smooth manifolds. Springer, New York
Marino R (1986) On the largest feedback linearizable subsystem. Syst Control Lett 6(5):345–351
Marino R, Boothby W, Elliott DL (1985) Geometric properties of linearizable control systems. Math Syst Theory 18(1):97–123
MI El-Hawwary M, Maggiore M (2013) Reduction theorems for stability of closed sets with application to backstepping control design. Automatica 49(1):214–222
Nielsen C (2009) Set stabilization using transverse feedback linearization. PhD thesis, University of Toronto
Nielsen C, Maggiore M (2008) On local transverse feedback linearization. SIAM J Control Optim 47(5):2227–2250
Olfati-Saber R, Murray RM (2004) Consensus problems in networks of agents with switching topology and time-delays. IEEE Tran Autom Control 49(9):1520–1533
Poincaré H (1875) Note sur les propriétés des fonctions définies par les équations différentielles. J École Polytech 45:13–26
Respondek W, Tall I (2006) Feedback equivalence of nonlinear control systems: a survey on formal approach. Chaos Autom Control 137–262
Sommer R (1980) Control design for multivariable non-linear time-varying systems. Int J Control 31(5):883–891
Su R (1982) On the linear equivalents of nonlinear systems. Syst Control Lett 2(1):48–52
Wonham WM (2012) Linear multivariable control: a geometric approach. Stochastic modelling and applied probability. Springer, New York
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Compliance with ethical standards
This research was partially supported by the Natural Sciences and Engineering Research Council of Canada (N.S.E.R.C.) and by the University of Waterloo.
Additional information
This research was partially supported by the Natural Sciences and Engineering Research Council of Canada (N.S.E.R.C.).
Appendix: Supporting results and proofs
Appendix: Supporting results and proofs
Proof of Proposition 1
The proof that \(\dim {(P(q))} = \nu (q)\) is obvious from their definitions and is omitted. Next, we have
Similar computations yield \(\dim {(R(p))} = \sigma (p)\) on \(S_1\). \(\square \)
Proof of Proposition 2
Let \(U\subseteq {\mathbb {R}}^n\) be an open set containing \(\bar{x}\) and set \( V_1 = S_1\cap U \). If \(\dim {(T_{x}S_1\cap G_0(x))}\) is constant on \( V_1 \) then, since \( \dim {(T_xS_1)} \) and \( \dim {(G_0(x))} \) are constant on \( V_1 \), the function \( \sigma (x) \) in (11) is constant on \( V_1 \). If both \(\dim {(T_{x}S_2\cap G_0(x))}\) and \(\dim {(T_{x}S_1\cap G_0(x))}\) are constant on \( V_2 = S_2\cap U\) then the functions \( \nu \) and \( \rho \) in (11) are constant on \(V_2\).
Conversely, if the function \(\sigma \) is constant on an open set \( V_1 \subset S_1\) with \(\bar{x}\in V_1\), then since \( T_xS_1 \) and \( G_0(x) \) are constant dimensional and from the definition of \( \sigma \) it follows that \(\dim {(T_{x}S_1\cap G_0(x))}\) is constant on \( V_1 \). If \( \nu \), \(\rho \) are constant on an open set \( V_2 \subset S_2\) with \(\bar{x}\in V_2\) then from their definitions it follows that \(\dim {(T_{x}S_2\cap G_0(x))}\) is constant on \( V_2 \). \(\square \)
Proof of Proposition 3
Let \(\bar{x} \in S_2\) be a regular point of the distributions (10). Then, by Proposition 2 and Definition 4 P is non-singular in a neighbourhood \(V_2=V_1\cap S_2\), with \(V_1\subseteq S_1\) and containing \(\bar{x}\). Lemma 3 proves that P is also smooth in a neighbourhood of \(\bar{x}\), without loss of generality, \(V_2\). Proposition 1 shows that Q is non-singular on \( V_2 \) and R is non-singular on \( V_1 \). Furthermore, by Proposition 2, the assumed non-singularity of \(G_0\) and Lemma 3 we have, by possibly shrinking \(V_1\), and hence \( V_2 \), that \( G_0 \cap TS_1\) and \(\left[ G_0\cap TS_1\right] ^\perp \) are smooth on \( V_1 \) and \(\left[ G_0(x)\cap TS_2\right] ^\perp \) is smooth on \( V_2 \). Therefore, Q and R are the non-singular intersection of smooth non-singular distributions and by [14, Lemma 1.3.5] they are smooth themselves.
Conversely, suppose that the distribution R in (10) is smooth and non-singular in a neighbourhood \(V_1 \subseteq S_1\) containing \(\bar{x}\) and distributions P and Q in (10) are smooth and non-singular in \(V_2=V_1\cap S_2 \). By Proposition 1 and Definition 4 \(\bar{x}\) is a regular point of (11). \(\square \)
Proof of Lemma 1
Let \(x\in S_2\) be fixed but arbitrary and let \(\varXi \in {{\mathrm{\text {Diff}}}}{(U)}\) be a diffeomorphism onto its image with domain U containing x. Let \(\left( \alpha ,\beta \right) \) be a regular feedback transformation also defined on U and let \(\tilde{g}(x) :=g(x)\beta (x)\), \(\tilde{G}_0(x):={{\mathrm{\text {span}}}}\{\tilde{g}_1(x),\ldots ,\tilde{g}_m(x)\}\). Since each \(\tilde{g}_i(x)\) is a linear combination of \(g_1(x), \ldots , g_m(x)\), \(\tilde{G}_0(x) \subseteq G_0(x)\). Furthermore, since \(\beta :U \subseteq {\mathbb {R}}^n\rightarrow {{\mathrm{{\mathsf {GL}}}}}(m,{\mathbb {R}})\) is non-singular, \(\tilde{G}_0(x) = G_0(x)\) and, therefore,
Next, let \(\hat{g} :=\varXi _\star (g\beta ) = \varXi _\star (\tilde{g})\) and \(\hat{G}_0 :={{\mathrm{\text {span}}}}\{\hat{g}_1,\ldots ,\hat{g}_m\}\). Since \({\mathrm {d}}\varXi _x\) is an isomorphism at each \(x \in U\), we have
where the next to last equality comes from the fact that \({{\mathrm{\text {Ker}}}}{({\mathrm {d}}\varXi _x)} = \{0\}\). From this it follows that the value \(\nu (x)\) is unchanged under coordinate and feedback transformations. The same arguments hold for the other functions in (11). \(\square \)
Proof of Lemma 2
Let \(\bar{x}\in S_2\) be arbitrary. Since \(S_1\subseteq {\mathbb {R}}^n\) is an embedded submanifold there exist slice coordinates \(\left( V_1,\psi \right) \) for \({\mathbb {R}}^n\) with \( \bar{x}\in V _1\) such that
where, without loss of generality, we take the constants \(c_i\) to be zero. Let \(\pi _1:{\mathbb {R}}^n\rightarrow {\mathbb {R}}^{n-s_1}\) denote the projection onto the last \(n-s_1\) factors, i.e, \(\pi _1(x)=(x_{s_1+1},\ldots ,x_n)\). Define \(\varPhi _1:V_1\rightarrow {\mathbb {R}}^{n-s_1},x\mapsto \pi _1\circ \psi (x)\). Then, \(\varPhi _1\) is a submersion and
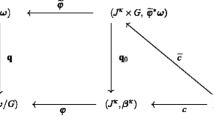
This construction is summarized in the following commutative diagram

We now apply a similar construction to \(S_2\). Let \(\left( V_2, \varphi \right) \) be slice coordinates for \({\mathbb {R}}^n\) with \(\bar{x}\in V_2\) and let \(\pi _2:{\mathbb {R}}^n\rightarrow {\mathbb {R}}^{n-s_2}\) be the projection onto the last \(n-s_2\) factors. Then, letting \(\varPhi _2:=\pi _2\circ \phi \) we have
and the commutative diagram

Let \(U:=V_1\cap V_2\) and note that \(\bar{x}\in U\). Since \(\varPhi _1\) and \(\varPhi _2\) are submersions we have that, for all \(x\in U\), \({{\mathrm{\text {rank}}}}({\text {d}}\varPhi _1)=n-s_1\) and \({{\mathrm{\text {rank}}}}({\text {d}}\varPhi _2)=n-s_2\). Furthermore, by [20, Lemma 8.15], for all \(x\in S_2\cap U\), \({{\mathrm{\text {Ker}}}}{{\text {d}}\varPhi _{1,x}}=T_xS_1\) and \({{\mathrm{\text {Ker}}}}{ {\text {d}}\varPhi _{2,x}}=T_xS_2\). Therefore, \({{\mathrm{\text {Ker}}}}{\text {d}}\varPhi _{2,x}\subset {{\mathrm{\text {Ker}}}}{\text {d}}\varPhi _{1,x}\) and
This allows us to construct a submersion \(\varPhi :U\rightarrow {\mathbb {R}}^{n-s_2}\). We take the last \(n-s_1\) components of \(\varPhi \) to be the function \(\varPhi _1\). From (30) we conclude that in the set \( \varPhi _2=\{\varphi _{s_2+1},\ldots ,\varphi _n\} \) it is possible to find \( s_1-s_2 \) functions, without of loss of generality \( \{\varphi _{s_2+1},\ldots ,\varphi _{s_1}\}=:\bar{\varPhi }_2 \), with the property that the \( n-s_2 \) differentials \( {\text {d}}\varphi _{s_2+1},\ldots ,{\text {d}}\varphi _{s_1},{\text {d}}\psi _{s_1+1},\ldots ,{\text {d}}\psi _n \) are linearly independent at \( \bar{x}\). Let \(\varPhi :=\left( \bar{\varPhi }_2,\varPhi _1\right) \).
Since, \({\text {d}}\varPhi (\bar{x})\) has rank \(n-s_2\) it has some \((n-s_2)\times (n-s_2)\) minor with non-zero determinant. By re-ordering the coordinates we assume that it is the minor corresponding to the first \(n-s_2\) rows and columns of \({\mathrm {d}}\varPhi (\bar{x})\). Relabel the coordinates as \((y,z)=(x_1,\ldots ,x_{n-s_2},x_{n-s_2+1},\ldots ,x_n)\) in \({\mathbb {R}}^n\). Define \(\varXi :U\rightarrow {\mathbb {R}}^n\) by \(\varXi (y,z):=(z,\varPhi (y,z))\). Its total derivative at \(\bar{x}\) is
which is non-singular because its columns are independent. Therefore, by the inverse function theorem [20, Theorem 7.6], by possibly shrinking U, \(\varXi \in {{\mathrm{\text {Diff}}}}{(U)}\). In the chart \(\left( U,\varXi \right) \) of \({\mathbb {R}}^n\) we have
and
\(\square \)
Proof of Proposition 4
Let \({\mathcal {N}}(M)\) be a tubular neighbourhood of M. By [20, Proposition 10.20] there exists a smooth retraction \(r : {\mathcal {N}}(M) \rightarrow M\). Let \(U\subseteq {\mathcal {N}}(M) \) be an open set containing x. Then, restriction \(\left. r \right| _U\) is a smooth retraction of U to \(M\cap U\). \(\square \)
Proof of Proposition 5
Apply Lemma 2 to obtain an open set \(U \subseteq {\mathbb {R}}^n\) containing \(\bar{x}\) and maps \( \varPhi _1 \) and \(\bar{\varPhi }_2 \) such that \(V_1=\varPhi _1^{-1}(0)\) and \(V_2=(\bar{\varPhi }_2,\varPhi _1)^{-1}(0)\) where \(V_1:=S_1\cap U\) and \(V_2:=S_2\cap U\).
Since \(S_1\) is a controlled-invariant submanifold there exists a smooth state feedback \(\alpha _1:V_1\rightarrow {\mathbb {R}}^m\) such that
Similarly, since \(S_2\) is a controlled-invariant submanifold there exists a smooth state feedback \(\alpha _2:V_2\rightarrow {\mathbb {R}}^m\) such that
We now modify \(\alpha _1\) so that the resulting state feedback simultaneously satisfies (31) and (32). We have that
Since \( \alpha _1 \) and \( \alpha _2 \) are both smooth, there exists a smooth \(\hat{v}(x)\in {{\mathrm{\text {Ker}}}}(\left. {\text {d}}\varPhi _1(x)g(x)\right| _{V_2})\) such that, for all \( x\in V_2\), \( \alpha _2(x)=\left. \alpha _1(x)\right| _{V_2}+\hat{v}(x) \). We have that
By hypothesis, \( \bar{x}\) is a regular point of (10) and by Proposition 2, by possibly shrinking \( V_1 \), \(\dim (T_xS_1\cap G_0(x))\) is constant and \( \dim G_0(x) \) is constant. Thus, \({{\mathrm{\text {rank}}}}( {\text {d}}\varPhi _1(x)g(x)) \) is constant on \( V_1 \). It implies that \( \dim \left( {{\mathrm{\text {Ker}}}}( {\text {d}}\varPhi _1(x)g(x))\right) \) is also constant on \( V_1 \). Assume that \( \dim \left( {{\mathrm{\text {Ker}}}}( {\text {d}}\varPhi _1(x)g(x))\right) =q \). By [14, Lemma 1.3.1], there exists a set \(\{v_1,\ldots ,v_q\} \) of smooth vector fields defined on \( V_1 \) such that at each \( x\in V_1 \), the vectors \( v_1(x),\ldots ,v_q(x) \) are linearly independent and
Thus, we can write
where \( \hat{c}_i:V_2\rightarrow {\mathbb {R}}\) are smooth real-valued functions. Apply Proposition 4 and, by possibly shrinking U , introduce a retraction \( r_1:V_1\rightarrow V_2 \) of \( V_1\) onto \( V_2\) and define
and
Let \(\alpha ^\prime :=\alpha _1+v\). It solves Eq. (31) since
Similarly it can be verified that it solves Eq. (32). Again, applying Proposition 4 we introduce a retraction \(r_2:U\rightarrow V_1 \) of U into \( V_1\) and define
The state feedback \( \alpha \) has the desired property. \(\square \)
Lemma 3
[24] Let \(N \subset M\) be an n-dimensional submanifold of the m-dimensional manifold M. Let \(p \in N\) be a regular point of a d-dimensional distribution D on M. Suppose there exists an open neighbourhood V of p in N such that \(k = \dim (T_qN \cap D(q))\) is constant for all \(q \in V\). Then, there exists a neighbourhood U of p in V such that \(TN\cap D\) is smooth on U.
Proof
Let \((W, \psi )\) be a coordinate chart of M adapted to N, that is, such that \(\psi (N \cap W) = \{x \in \psi (W): x_{n+1} = \cdots = x_m = 0\}\), and let \(\{f_1, \ldots , f_d\}\) be a set of local generators of D around p. Let \(\pi : (x_1, \ldots , x_m) \mapsto (x_1, \ldots , x_n)\) be the projection onto the first n factors. By making W smaller, we can assume that \(f_1, \dots , f_d\) are linearly independent on W.
Recall that \(\hat{\psi } := \pi \circ \psi : N \cap W \rightarrow {\mathbb {R}}^n\) is a diffeomorphism onto its image, and let
The vector fields \(\hat{f}_i\) are defined on an open set of \({\mathbb {R}}^n\). Letting \(\{e_1, \ldots , e_n\}\) denote the natural basis of \({\mathbb {R}}^n\), for each \(q \in N \cap W\) we have
Hence, \(d\hat{\psi }(TN\cap D)\) is a distribution on an open set of \({\mathbb {R}}^n\). By assumption, and since \(d\hat{\psi }_q\) is an isomorphism at each \(q \in N \cap W\), it is the intersection of two smooth non-singular distributions, and it has constant dimension near \(\psi (p)\). Therefore, by [14, Lemma 1.3.5], it is smooth. This implies that \(TN \cap D\) is also smooth on a neighbourhood V of p. \(\square \)
Rights and permissions
About this article
Cite this article
Doosthoseini, A., Nielsen, C. Local nested transverse feedback linearization. Math. Control Signals Syst. 27, 493–522 (2015). https://doi.org/10.1007/s00498-015-0149-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00498-015-0149-y