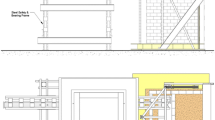
Abstract
The article presents a parametric study of optimal designs for geosynthetic-reinforced soil (GRS) bridge abutments. A mixed integer design optimization model GRS-BA was developed, which is comprised of an accurate objective function of the construction costs. The cost objective function was constrained by a set of geotechnical and design conditions that were in accordance with current practice rules and recommendations. The optimal design recommendation for GRS bridge abutments was developed. A typical example of such an abutment is presented in order to compare design solutions derived from conventional design methods with solutions obtained from the proposed optimal design procedure.




Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Abbreviations
- B :
-
Width of the abutment
- C :
-
Reinforcement effective unit parameter
- D :
-
Effective width of the applied load at depth z
- c exc :
-
Unit price of ground excavation
- c sta :
-
Unit price of the fill soil stabilization
- c geo,1 :
-
Coefficient for the cost calculation of various strengths of geotextiles
- c geo,2 :
-
Coefficient for the cost calculation of various strengths of geotextiles
- c fill,re :
-
Unit price of the reinforced fill soil
- c fill,ret :
-
Unit price of the retained fill soil
- c found :
-
Unit price of the concrete for the foundation at the base
- c sill :
-
Unit price of the reinforced concrete for the sill
- c batter :
-
Unit price of the front batter
- DL:
-
Vertical dead load
- d :
-
Clear distance
- FSsliding,sill,min :
-
Minimum safety factor for the sliding failure of the sill
- FSsliding,min :
-
Minimum safety factor for the sliding failure of the reinforced volume
- FSpullout,min :
-
Minimum safety factor against reinforcement pullout
- F 2 :
-
Horizontal load of the bridge
- F* :
-
Pullout resistance factor
- H 1 :
-
Height of the front wall
- H 2 :
-
Height of the back wall
- L :
-
Length of the geosynthetic reinforcement
- L′ :
-
Effective length of the geosynthetic reinforcement
- L e :
-
Length of embedment in the resistant zone behind the failure surface at depth z
- L found :
-
Width of the foundation at the base
- L i :
-
Length of embedment within the influence area inside the resistant zone
- L sill :
-
Width of the sill foundation
- L sill,ef :
-
Effective width of the sill foundation
- LL:
-
Vertical live load
- n prov,H2 :
-
Number of reinforcement layers in the back wall
- n exc,back :
-
Excavation slope of the retained soil
- n exc,face :
-
Inclination of the terrain slope
- q :
-
Traffic surcharge
- P r :
-
Pullout resistance
- R c :
-
Coverage ratio
- RFsill :
-
Reduction factor for the isolated sill
- T :
-
Strength of the geosynthetic reinforcement
- T max :
-
Maximum tensile force in the reinforcement at depth z
- T ε=1% :
-
Minimum required reinforcement stiffness
- t bridge :
-
Thickness of the bridge’s concrete slab
- t wall :
-
Thickness of the wall
- t found :
-
Thickness of the foundation at the base
- t sill :
-
Thickness of the sill
- α :
-
Scale effect correction factor
- γ bridge :
-
Unit weight of the reinforced concrete
- γ conc :
-
Unit weight of the concrete
- γ re :
-
Unit weight of the retained earth
- γ rf :
-
Unit weight of the fill soil
- φ fs :
-
Friction angle of the foundation soil
- φ re :
-
Friction angle of retained earth
- φ rf :
-
Friction angle of the fill soil
- Δh :
-
Spacing between the geosynthetic reinforcement layers
- Δσh :
-
Supplemental horizontal pressure at depth z
- Δσv :
-
Distributed vertical pressure from the sill
- Δσvs :
-
Vertical soil pressure at depth z
References
Abu-Hejleh N, Zornberg JG, Wang T, Watcharamonthein J (2002) Monitored displacements of unique geosynthetic-reinforced soil bridge abutments. Geosynth Int 9:71–95. https://doi.org/10.1680/gein.9.0211
Adams MT, Collin JG (1997) Large model spread-footing load tests on geosynthetics. J Geotech Geoenviromental Eng ASCE 123:66–72. https://doi.org/10.7550/rmb.37002
Adams M, Nicks J, Stabile T, Wu J, Schlatter W, Hartmann J (2010) Geosynthetic reinforced soil integrated bridge system—interim implementation guide, report no. FHWA-HRT-11-026, Federal Highway Administration, McLean, VA
Adams M, Nicks J, Stabile T, Wu J, Schlatter W, Hartmann J (2011) Geosynthetic reinforced soil integrated bridge system synthesis report, report no. FHWA-HRT-11-027, Federal Highway Administration, McLean, VA
Ardah A, Abu-Farsakh M, Voyiadjis G (2017) Numerical evaluation of the performance of a geosynthetic reinforced soil-integrated bridge system (GRS-IBS) under different loading conditions. Geotext Geomembr 45:558–569. https://doi.org/10.1016/j.geotexmem.2017.07.005
Attar IH, Fakharian K (2007) Static and seismic numerical modeling of geosynthetic-reinforced soil segmental bridge abutments. Geosynth Int 14:228–243. https://doi.org/10.1680/gein.2007.14.4.228
Baker R, Garber M (1977) Variational approach to slope stability. In: Proceedings of the ninth international conference on soil mechanics and foundation engineering
Bathurst RJ, Vlachopoulos N, Walters DL, Burgess PG, Allen TM (2006) The influence of facing stiffness on the performance of two geosynthetic reinforced soil retaining walls. Can Geotech J 43:1225–1237. https://doi.org/10.1139/t06-076
Briaud J-L, Gibbens R (1999) Behaviour of five large spread footings in sand. J Geotech Geoenvironmental Eng 125(9):787–796
Deb K (2000) An efficient constraint handling method for genetic algorithms. Comput Methods Appl Mech Eng 186:311–338. https://doi.org/10.1016/S0045-7825(99)00389-8
Deep K, Singh KP, Kansal ML, Mohan C (2009) A real coded genetic algorithm for solving integer and mixed integer optimization problems. Appl Math Comput 212:505–518. https://doi.org/10.1016/j.amc.2009.02.044
Jelusic P (2015) Soil compaction optimization with soft constrain. J Intell Fuzzy Syst 29:955–962. https://doi.org/10.3233/IFS-151624
Jelušič P, Žlender B (2013) Soil-nail wall stability analysis using ANFIS. Acta Geotech Slov 10:61–73. http://www.fg.uni-mb.si/journal-ags/pdfs/AGS_2013-1_article_5.pdf
Jelušič P, Žlender B (2018a) Optimal design of pad footing based on MINLP optimization. Soils Found 58:277–289. https://doi.org/10.1016/j.sandf.2018.02.002
Jelušič P, Žlender B (2018b) Optimal design of piled embankments with basal reinforcement. Geosynth Int 25:150–163. https://doi.org/10.1680/jgein.17.00039
Keller GR, Devin SC (2003) Geosynthetic-reinforced soil bridge abutments. Transp Res Rec 1819:362–368. https://doi.org/10.3141/1819b-46
Leshchinsky D, Kang B, Han J, Ling H (2014) Framework for limit state design of geosynthetic-reinforced walls and slopes. Transp Infrastruct Geotechnol 1:129–164. https://doi.org/10.1007/s40515-014-0006-3
Meier J, Schaedler W, Borgatti L et al (2008) Inverse parameter identification technique using PSO algorithm applied to geotechnical modeling. J Artif Evol Appl 2008:1–14. https://doi.org/10.1155/2008/574613
Mirmoradi SH, Ehrlich M (2014) Numerical evaluation of the behavior of GRS walls with segmental block facing under working stress conditions. J Geotech Geoenvironmental Eng 8:1–8. https://doi.org/10.1061/(ASCE)GT.1943-5606.0001235
Miyata K (1996) Walls reinforced with fiber reinforced plastic geogrids in Japan. Geosynth Int 3:1–11. https://doi.org/10.1680/gein.3.0050
Miyata Y, Bathurst RJ, Miyatake H (2015) Performance of three geogrid-reinforced soil walls before and after foundation failure. Geosynth Int 22:311–326. https://doi.org/10.1680/gein.15.00014
Nicks JE, Esmaili D, Adams MT (2016) Deformations of geosynthetic reinforced soil under bridge service loads. Geotext Geomembr 44:641–653. https://doi.org/10.1016/j.geotexmem.2016.03.005
Saghebfar M, Abu-Farsakh M, Ardah A, Chen Q, Fernandez B (2017) Performance monitoring of geosynthetic reinforced soil integrated bridge system (GRS-IBS) in Louisiana. Geotext Geomembr 45:34–47. https://doi.org/10.1016/j.geotexmem.2016.11.004
Tatsuoka F, Tateyama M, Uchimura T, Koseki J (1998) Geosynthetic-reinforced soil retaining walls as important permanent structures. Geosynth Int 4:81–136. https://doi.org/10.1680/gein.4.0090
Tatsuoka F, Tateyama M, Mohri Y, Matsushima K (2007) Remedial treatment of soil structures using geosynthetic-reinforcing technology. Geotext Geomembr 25:204–220. https://doi.org/10.1016/j.geotexmem.2007.02.002
The Mathworks Inc. (2016) MATLAB—MathWorks. http://www.mathworks.com/products/matlab/. Accessed 10 Apr 2016
Venkataraman P (2009) Applied optimization with Matlab programming. John Wiley & Sons, New York
Wang Y, Kulhawy FH (2008) Economic design optimization of foundations. J Geotech Geoenvironmental Eng 134:1097–1105. https://doi.org/10.1061/(ASCE)1090-0241(2008)134:8(1097)
Wu J, Lee K, Helwany S, Ketchart K (2006) Design and construction guidelines for geosynthetic-reinforced soil bridge abutments with a flexible facing, report no. 556, National Cooperative Highway Research Program
Zhang Y, Gallipoli D, Augarde CE (2009) Simulation-based calibration of geotechnical parameters using parallel hybrid moving boundary particle swarm optimization. Comput Geotech 36:604–615. https://doi.org/10.1016/j.compgeo.2008.09.005
Zheng Y, Fox PJ (2016) Numerical investigation of geosynthetic-reinforced soil bridge abutments under static loading. J Geotech Geoenvironmental Eng 142:04016004. https://doi.org/10.1061/(ASCE)GT.1943-5606.0001452
Acknowledgements
The authors acknowledge financial support from the Slovenian Research Agency; research core Funding No. P2-0268.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Communicated by V. Loia.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Jelušič, P., Žlender, B. Determining optimal designs for geosynthetic-reinforced soil bridge abutments. Soft Comput 24, 3601–3614 (2020). https://doi.org/10.1007/s00500-019-04127-8
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00500-019-04127-8