Abstract
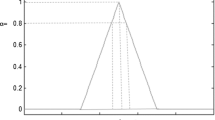
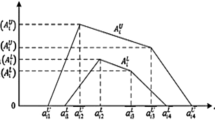
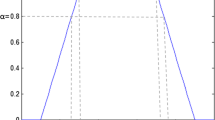
Statistical process control is an effective quality control technique to monitor a production process with balanced data under certain conditions. However, there are some situations where dealing with uncertainty and unbalanced data is considered. In such situations, the traditional statistical control charts are not effective to obtain control limits. The aim of this paper is fourfold. First of all, the collected unbalanced data are converted to triangular fuzzy numbers for each sample. Second, this paper develops a fuzzy \( \bar{X} - S \) control chart while dealing with unbalanced fuzzy data. Third, a proposed approach is presented on how to deal with unbalanced fuzzy data for calculations of control limits. Besides, fuzzy process capability analyses are conducted to measure process performance. Finally, an illustrative example is conducted to show the effectiveness of the proposed fuzzy \( \bar{X} - S \) control chart with unbalanced data for uncertainty.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Alipour H, Noorossana R (2010) Fuzzy multivariate exponentially weighted moving average control chart. Int J Adv Manuf Technol 48:1001–1007
Anderson TW, Darling DA (1954) A test of goodness of fit. J Am Stat Assoc 49(268):765–769
Erginel N (2014) Fuzzy rule-based p and n-p control charts. J Intell Fuzzy Syst 27(1):159–171
Erginel N, Şentürk S (2016) Fuzzy EWMA and fuzzy CUSUM control charts. In: Fuzzy statistical decision-making. Springer, Cham, pp 281–295
Erginel N, Şentürk S, Kahraman C, Kaya İ (2011) Evaluating the packing process in food industry using [stilde] control charts. Int J Comput Intell Syst 4(4):509–520
Gülbay M, Kahraman C (2007) An alternative approach to fuzzy control charts: direct fuzzy approach. Inf Sci 177(6):1463–1480
Hryniewicz O (2008) Statistics with fuzzy data in statistical quality control. Soft Comput 12(3):229–234
Kaplan Göztok K, Uçurum M, Özdemir A (2020) Analysis of 19 × 39 × 19 cm pumice brick material production with fuzzy statistical process control technique. El-Cezeri J Sci Eng 7(1):43–56. https://doi.org/10.31202/ecjse.591580
Kaya İ, Kahraman C (2011) Process capability analyses with fuzzy parameters. Expert Syst Appl 38(9):11918–11927
Kotz S, Johnson NL (2002) Process capability indices—a review, 1992–2000. J Qual Technol 34(1):2–19
Montgomery DC (2009) Introduction to statistical quality control, vol 7. Wiley, New York
Şentürk S, Erginel N (2009) Development of fuzzy X–R and X–S control charts using α-cuts. Inf Sci 179:1542–1551
Şentürk S, Erginel N, Kaya İ, Kahraman C (2011) Design of fuzzy u control chart. J Multiple Valued-Logic Soft Comput 17:459–473
Şentürk S, Erginel N, Kaya İ, Kahraman C (2014) Fuzzy exponentially weighted moving average control chart for univariate data with a real case application. Appl Soft Comput 22:1–10
Shu MH, Wu HC (2011) Fuzzy X and R control charts: fuzzy dominance approach. Comput Ind Eng 61(3):676–685
Yum BJ, Kim KW (2011) A bibliography of the literature on process capability indices: 2000–2009. Qual Reliab Eng Int 27(3):251–268
Funding
There is no funding reported for this article.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The author declares that there is no conflict of interest.
Ethical approval
This article does not contain any studies with human participants or animals performed by the author.
Additional information
Communicated by V. Loia.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Özdemir, A. Development of fuzzy \( \bar{X} - S \) control charts with unbalanced fuzzy data. Soft Comput 25, 4015–4025 (2021). https://doi.org/10.1007/s00500-020-05430-5
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00500-020-05430-5