Skip to main content

Advertisement

Springer Nature Link
Log in

Improved seven-dimensional (i7D) hyperchaotic map-based image encryption technique

  • Foundation, algebraic, and analytical methods in soft computing
  • Published:
Soft Computing Aims and scope Submit manuscript

Abstract

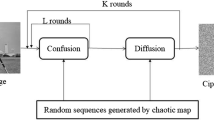
With the advancements in Internet technologies, a huge amount of images are transferred over the public networks. Therefore, during the transmission process, the images are prone to various security threats. To protect images against any unauthorized access, many researchers have designed various approaches such as encryption, stenography, and visual cryptography. Each approach has its own benefits over the others. The main objective of this paper is to design an efficient image encryption approach. Initially, various image encryption approaches have been studied. It is found that the majority of the existing image encryption approaches suffer from the hyperparameters tuning problem. Also, it is desirable to develop secret keys which are highly sensitive to plain images. Therefore, in this paper, an improved seven-dimensional hyperchaotic system (i7DHS) is adopted to generate secret keys. But, i7DHS is sensitive to its initial parameters. Therefore, a dual local search-based evolutionary algorithm (DEA) is utilized to tune the initial parameters of i7DHS. The computed optimal keys are utilized to encrypt the input images. Performance analysis shows that DEA-based i7DHS outperforms the competitive approaches. DEA-based i7DHS is highly sensitive to the input images and has large secret key space. It is found that DEA-based i7DHS can resist various security attacks.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data Availability Statement

Enquiries about data availability should be directed to the authors.

References

  • Ahmad J, Hwang SO (2016) A secure image encryption scheme based on chaotic maps and affine transformation. Multimedia Tools Appl 75(21):13951–13976

    Google Scholar 

  • Bechikh R, Hermassi H, El-Latif AAA, Rhouma R, Belghith S (2015) Breaking an image encryption scheme based on a spatiotemporal chaotic system. Signal Process Image Commun 39:151–158

    MATH  Google Scholar 

  • Benlashram A, Al-Ghamdi M, AlTalhi R, Laabidi PK (2020) A novel approach of image encryption using pixel shuffling and 3d chaotic map. J Phys Conf Ser 1447:012009

    Google Scholar 

  • Benrhouma O, Hermassi H, El-Latif AAA, Belghith S (2015) Cryptanalysis of a video encryption method based on mixing and permutation operations in the dct domain. SIViP 9(6):1281–1286

    Google Scholar 

  • Chen J, Zhu Z-L, Zhang L-B, Zhang Y, Yang B-Q (2018) Exploiting self-adaptive permutation-diffusion and dna random encoding for secure and efficient image encryption. Signal Process 142:340–353

    Google Scholar 

  • Cheng G, Wang C, Chen H (2019) A novel color image encryption algorithm based on hyperchaotic system and permutation-diffusion architecture. Int J Bifurc Chaos 29(09):1950115

    MathSciNet  MATH  Google Scholar 

  • Ding L, Ding Q (2020) A novel image encryption scheme based on 2d fractional chaotic map, dwt and 4d hyper-chaos. Electronics 9(8):1280

    Google Scholar 

  • El Assad S, Farajallah M (2016) A new chaos-based image encryption system. Signal Process Image Commun 41:144–157

    Google Scholar 

  • Fang D, Sun S (2020) A new secure image encryption algorithm based on a 5d hyperchaotic map. PLoS ONE 15(11):e0242110

    Google Scholar 

  • Hansen N, Arnold DV, Auger A (2015) Evolution strategies. Springer handbook of computational intelligence. Springer, New York, pp 871–898

    Google Scholar 

  • Haspolat E, Yildiz B (2021) Fractional order of a new 7d hyperchaotic lorenz-like system. Konuralp J Math (KJM) 9(1):76–89

    MathSciNet  Google Scholar 

  • Hong W, Tang K, Zhou A, Ishibuchi H, Yao X (2018) A scalable indicator-based evolutionary algorithm for large-scale multiobjective optimization. IEEE Trans Evol Comput 23(3):525–537

    Google Scholar 

  • Hua Z, Jin F, Xu B, Huang H (2018) 2d logistic-sine-coupling map for image encryption. Signal Process 149:148–161

    Google Scholar 

  • Hussain I, Shah T, Gondal MA (2012) Image encryption algorithm based on pgl (2, gf (2 8)) s-boxes and td-ercs chaotic sequence. Nonlinear Dyn 70(1):181–187

    MathSciNet  Google Scholar 

  • Kaur M, Kumar V (2018) Colour image encryption technique using differential evolution in non-subsampled contourlet transform domain. IET Image Proc 12(7):1273–1283

    Google Scholar 

  • Kaur M, Singh D (2021) Multiobjective evolutionary optimization techniques based hyperchaotic map and their applications in image encryption. Multidimen Syst Signal Process 32(1):281–301

    MATH  Google Scholar 

  • Kaur M, Singh D, Uppal RS (2020) Parallel strength pareto evolutionary algorithm-II based image encryption. IET Image Proc 14(6):1015–1026

    Google Scholar 

  • Kaur M, Singh D, Kumar V (2020) Color image encryption using minimax differential evolution-based 7d hyper-chaotic map. Appl Phys B 126(9):1–19

    Google Scholar 

  • Kaur M, Singh D, Kumar V, Gupta BB, Abd El-Latif AA (2021) Secure and energy efficient-based e-health care framework for green internet of things. IEEE Trans Green Commun Netw 5(3):1223–1231. https://doi.org/10.1109/TGCN.2021.3081616

    Article  Google Scholar 

  • Liu H, Wang X (2011) Color image encryption using spatial bit-level permutation and high-dimension chaotic system. Opt Commun 284(16–17):3895–3903

    Google Scholar 

  • Nunez-Perez J-C, Adeyemi V-A, Sandoval-Ibarra Y, Perez-Pinal F-J, Tlelo-Cuautle E (2021) Maximizing the chaotic behavior of fractional order Chen system by evolutionary algorithms. Mathematics 9(11):1194

    Google Scholar 

  • Pak C, Huang L (2017) A new color image encryption using combination of the 1d chaotic map. Signal Process 138:129–137

    Google Scholar 

  • Pak C, An K, Jang P, Kim J, Kim S (2019) A novel bit-level color image encryption using improved 1d chaotic map. Multimedia Tools Appl 78(9):12027–12042

    Google Scholar 

  • Rawat N, Kim B, Kumar R (2016) Fast digital image encryption based on compressive sensing using structurally random matrices and arnold transform technique. Opt Int J Light Elect Opt 127(4):2282–2286

    Google Scholar 

  • Rodriguez IF, Amaya EI, Suarez CA, Moreno JD (2017) Images encryption algorithm using the Lorenzs chaotic attractor. Ingeniería 22(3):396–412

    Google Scholar 

  • Sayed WS, Radwan AG, Abd-El-Hafiz SK (2018) Chaotic properties of various types of hidden attractors in integer and fractional order domains. Mathematical techniques of fractional order systems. Elsevier, Amsterdam, pp 503–528

    Google Scholar 

  • Sun S, Guo Y, Wu R (2019) A novel image encryption scheme based on 7d hyperchaotic system and row-column simultaneous swapping. IEEE Access 7:28539–28547

    Google Scholar 

  • Teng L, Wang X, Yang F, Xian Y (2021) Color image encryption based on cross 2d hyperchaotic map using combined cycle shift scrambling and selecting diffusion. Nonlinear Dyn 105:1859–1876

    Google Scholar 

  • Ul Haq T, Shah T (2021) 4d mixed chaotic system and its application to RGB image encryption using substitution-diffusion. J Inform Sec Appl 61:102931

    Google Scholar 

  • Wang X, Gao S (2020) Image encryption algorithm based on the matrix semi-tensor product with a compound secret key produced by a boolean network. Inf Sci 539:195–214

    MathSciNet  MATH  Google Scholar 

  • Wang X, He G (2011) Cryptanalysis on a novel image encryption method based on total shuffling scheme. Opt Commun 284(24):5804–5807

    Google Scholar 

  • Wang Y, Yang F (2021) A fractional-order cnn hyperchaotic system for image encryption algorithm. Phys Scr 96(3):035209

    MathSciNet  Google Scholar 

  • Wang X-Y, Zhang Y-Q, Zhao Y-Y (2015) A novel image encryption scheme based on 2-d logistic map and DNA sequence operations. Nonlinear Dyn 82(3):1269–1280

    MathSciNet  MATH  Google Scholar 

  • Wang X, Feng L, Zhao H (2019) Fast image encryption algorithm based on parallel computing system. Inf Sci 486:340–358

    MATH  Google Scholar 

  • While L, Hingston P, Barone L, Huband S (2006) A faster algorithm for calculating hypervolume. IEEE Trans Evol Comput 10(1):29–38

    Google Scholar 

  • Wu X, Wang D, Kurths J, Kan H (2016) A novel lossless color image encryption scheme using 2d dwt and 6d hyperchaotic system. Inf Sci 349:137–153

    Google Scholar 

  • Wu J, Liao X, Yang B (2018) Image encryption using 2d hénon-sine map and dna approach. Signal Process 153:11–23

  • Xiong Z, Wu Y, Ye C, Zhang X, Xu F (2019) Color image chaos encryption algorithm combining CRC and nine palace map. Multimedia Tools Appl 78(22):31035–31055

    Google Scholar 

  • Xuejing K, Zihui G (2020) A new color image encryption scheme based on DNA encoding and spatiotemporal chaotic system. Signal Process Image Commun 80:1156700

    Google Scholar 

  • Yang P, Tang K, Yao X (2017) Turning high-dimensional optimization into computationally expensive optimization. IEEE Trans Evol Comput 22(1):143–156

    Google Scholar 

  • Yang Q, Zhu D, Yang L (2018) A new 7d hyperchaotic system with five positive lyapunov exponents coined. Int J Bifurc Chaos 28(05):1850057

    MathSciNet  MATH  Google Scholar 

  • Yang F, Mou J, Luo C, Cao Y (2019) An improved color image encryption scheme and cryptanalysis based on a hyperchaotic sequence. Phys Scr 94(8):085206

    Google Scholar 

  • Yang F, Mou J, Liu J, Ma C, Yan H (2020) Characteristic analysis of the fractional-order hyperchaotic complex system and its image encryption application. Signal Process 169:107373

    Google Scholar 

  • Yang Y-G, Guan B-W, Zhou Y-H, Shi W-M (2021) Double image compression-encryption algorithm based on fractional order hyper chaotic system and dna approach. Multimedia Tools Appl 80(1):691–710

    Google Scholar 

  • Ye G (2010) Image scrambling encryption algorithm of pixel bit based on chaos map. Pattern Recogn Lett 31(5):347–354

    Google Scholar 

  • Yuan H-M, Liu Y, Lin T, Hu T, Gong L-H (2017) A new parallel image cryptosystem based on 5d hyper-chaotic system. Signal Process Image Commun 52:87–96

    Google Scholar 

  • Zhou S, He P, Kasabov N (2020) A dynamic dna color image encryption method based on sha-512. Entropy 22(10):1091

    MathSciNet  Google Scholar 

Download references

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. School of Electrical Engineering and Computer Science, Gwangju Institute of Science and Technology, Gwangju, South Korea

    Manjit Kaur

  2. School of Electrical Engineering and Computer Science, Gwangju Institute of Science and Technology, Gwangju, South Korea

    Dilbag Singh

  3. Computer Science and Engineering Department, NIT Hamirpur, Hamirpur, India

    Vijay Kumar

Authors
  1. Manjit Kaur
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Dilbag Singh
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Vijay Kumar
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Dilbag Singh.

Ethics declarations

Conflict of interest

The authors declare no competitive interest regarding the publication of this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kaur, M., Singh, D. & Kumar, V. Improved seven-dimensional (i7D) hyperchaotic map-based image encryption technique. Soft Comput 26, 3703–3712 (2022). https://doi.org/10.1007/s00500-022-06841-2

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-022-06841-2

Keywords