Skip to main content
Springer Nature Link
Log in

A generalized collision algorithm for geometric graphics

  • Foundations
  • Published:
Soft Computing Aims and scope Submit manuscript

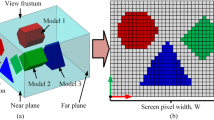
Abstract

The two-dimensional graphical nesting problem is widespread in industrial production and is an NP-complete problem. The core technology of various nesting methods is the graphical collision algorithm. In this paper, a general algorithm for geometric graphics is proposed. According to the geometrical characteristics of the packed parts, the idea of divide and conquer is adopted, and the corresponding collision strategies are designed, respectively. Two-point bidirectional collision calculation, aligned bidirectional collision and slipping calculation are proposed to determine the collision relationship between graphics. The interpolation strategy is used to reduce the computation of NFP (No-Fit-Polygon). The precise interpolation between graphics is achieved by first marking and positioning, and then sliding interpolation in both directions, which improves the interpolation efficiency. Finally, the results of the comparison test by several cases of different types show that the algorithm is effective, stable, reliable and adaptable.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

Enquiries about data availability should be directed to the authors.

References

  • Arbib C, Marinelli F, Pizzuti A (2021) Number of bins and maximum lateness minimization in two-dimensional bin packing. Eur J Oper Res 291:101–113

    Article  MathSciNet  Google Scholar 

  • Bódis A, Csirik J (2021) The variable-width strip packing problem. Cent Eur J Oper Res

  • Borgulya I (2021) A hybrid evolutionary algorithm for the offline bin packing problem. Cent Eur J Oper Res 29:425–445

    Article  MathSciNet  Google Scholar 

  • Braam F, Berg D (2022) Which rectangle sets have perfect packings? Oper Res Pers 9:100211

    MathSciNet  Google Scholar 

  • Chen K, Zhuang J, Zhong S, Zheng S (2020) Optimization method for guillotine packing of rectangular items within an irregular and defective slate. Mathematics 8:8111914

    Google Scholar 

  • Chen S, Xu J, Rong A et al (2021a) Optimal algorithm on rectangular workpiece layout combining blank rectangle filling and neighborhood search algorithms. Forg Stamp Tech 46(2):52–58

    Google Scholar 

  • Chen Y, Chen Z, Lin G, Wang L, Zhang A (2021b) A randomized approximation algorithm for metric triangle packing. J Comb Optim 41:12–27

    Article  MathSciNet  Google Scholar 

  • Chen M, Wu C, Tang X, Peng X, Zeng Z, Liu S (2019) An efficient deterministic heuristic algorithm for the rectangular packing problem. Comput Ind Eng 137:106097

    Article  Google Scholar 

  • Cid-Garcia N, Rios-Solis Y (2021) Exact solutions for the 2d-strip packing problem using the positions-and-covering methodology. PLoS ONE 16(1):0245267

    Article  Google Scholar 

  • Côté JF, Haouari M, Ioric M (2021) Combinatorial benders decomposition for the two-dimensional bin packing problem. Informs J Comput 33(3):963–978

    Article  MathSciNet  Google Scholar 

  • Erozan İ, Çalışkan E (2020) A multi-objective genetic algorithm for a special type of 2d orthogonal packing problems. Appl Math Model 77:66–81

    Article  MathSciNet  Google Scholar 

  • Fatma G, Samir E, BurakC Y (2020) The pallet loading problem: three-dimensional bin packing with practical constraints. Eur J Oper Res 287:1062–1074

    Article  MathSciNet  Google Scholar 

  • Frat H, Alpaslan N (2020) An effective approach to the two-dimensional rectangular packing problem in the manufacturing industry. Comput Ind Eng 148:106687

    Article  Google Scholar 

  • Gao B, Zhang H, Zhu M (2021) Optimization algorithm of irregular parts layout for intelligent manufacturing. Comput Integ Manuf Sys 27(6):1673–1680

    Google Scholar 

  • Guo W, Ji M, Deng W (2020) Rule algorithms study for rectangle packing problem. Mod Manuf Eng 2020(6):86–93

    Google Scholar 

  • Guo B, Sui Y (2021) Rectilinear parts cutting algorithm based on layout rectangle. Comput Sys Appl 30(1):162–167

    Google Scholar 

  • Hu X, Zhu W, Ma H, An B, Zhi Y, Wu Y (2021) Orientational variable-length strip covering problem: a branch-and-price-based algorithm. Eur J Oper Res 289:254–269

    Article  MathSciNet  Google Scholar 

  • Júnior A, Silva E, Francescatto M, Rosa C, Siluk J (2022) The rectangular two-dimensional strip packing problem real-life practical constraints: a bibliometric overview. Comput Oper Res 137:105521

    Article  MathSciNet  Google Scholar 

  • Li Y, Sang H, Xiong X et al (2021) An improved adaptive genetic algorithm for two-dimensional rectangular packing problem. Appl Sci-Basel 2021:11010413

    Google Scholar 

  • Martin M, Birgin E, Lobato R, Morabito R, Munari P (2020) Models for the two-dimensional rectangular single large placement problem with guillotine cuts and constrained pattern. Int T Oper Res 27:767–793

    Article  MathSciNet  Google Scholar 

  • Pan W, Fan Z, Huang M (2020) A four-block layout algorithm for unconstrained two dimensional plate guillotine cutting problem of rectangular items. Cont Dec 2020:1552

    Google Scholar 

  • Pantoja-Benavides G, Álvarez-Martínez D (2022) A hybrid approach of simulation and metaheuristic for the polyhedra packing problem. Int J Ind Eng Comput 13:81–100

    Google Scholar 

  • Polyakovskiy S, M’Hallah R (2021) Just-in-time two-dimensional bin packing. Omega 102:102311

    Article  Google Scholar 

  • Rakotonirainy R, Vuuren J (2020) Improved metaheuristics for the two-dimensional strip packing problem. Appl Soft Comput 92:106268

    Article  Google Scholar 

  • Rao Y, Wang P, Luo Q (2021) Hybridizing beam search with tabu search for the irregular packing problem. Math Probl Eng 2021:5054916

    Article  Google Scholar 

  • Schepler X, Rossi A, Gurevsky E, Dolgui A (2022) Solving robust bin-packing problems with a branch-and-price approach. Eur J Oper Res 297:831–843

    Article  MathSciNet  Google Scholar 

  • Tang D, Zhou Z (2016) No-fit-polygon-based heuristic nesting algorithm for irregular shapes. J Comput Appl 36(9):2540–2544

    Google Scholar 

  • Wang J, Qu S, Li K (2020) A two-dimensional irregular layout problem solving based on parallel cross genetic algorithm. Comput Appl Softw 37(7):188–193

    Google Scholar 

  • Wang X, Yin Z, Su D et al (2021) A novel approach of random packing generation of complex-shaped 3D particles with controllable sizes and shapes. Acta Geotech 2021

  • Zeng X, Wu Q, Yuan X (2020) Adaptive multi-island genetic algorithm optimization on layout problem for 2D rectangular parts. Forg Stamp Tech 45(12):53–58

    Google Scholar 

  • Zhang N, Zhao F, Gong Y et al (2021) Layout optimization based on dynamic lowest horizontal line method and ant colony algorithm. Comput Appl Softw 38(5):268–273

    Google Scholar 

  • ZhaoY CR, Carl H (2021) Optimizing 3D irregular object packing from 3D scans using metaheuristics. Adv Eng Inf 47:101234

    Article  Google Scholar 

  • Zhu K, Ji N, Li X (2020) Hybrid heuristic algorithm based on improved rules & reinforcement learning for 2D strip packing problem. IEEE Access 2020:3045905

    Google Scholar 

Download references

Funding

This study was supported by the Domestic Visiting Engineers Project of Zhejiang Education Department in 2020, China (Grant No. FG2020196, FG2020197), the second batch of teaching reform research projects in the 13th Five-Year Plan of Zhejiang Higher Education, China (Grant No. jg20190878), and the public welfare science and technology research project of Jinhua, Zhejiang Province, China. (Grant No. 2021-4-386).

Enquiries about data availability should be directed to the authors.

Author information

Authors and Affiliations

  1. School of Mechanical Information, Yiwu Industrial & Commercial College, Yiwu, 322000, China

    Pengfei Zheng & Jingjing Lou

  2. Zhejiang Provincial Key Laboratory of Third Generation Semiconductor Materials and Devices, Yiwu, 322000, China

    Pengfei Zheng & Chengjie Lian

  3. School of Mechanical and Power Engineering, East China University of Science and Technology, Shanghai, 200237, China

    Pengfei Zheng, Dajun Lin & Qi An

Authors
  1. Pengfei Zheng
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Jingjing Lou
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Chengjie Lian
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Dajun Lin
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. Qi An
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Pengfei Zheng.

Ethics declarations

Conflict of interest

The author(s) declared no potential conflicts of interest with respect to the research, authorship, and/or publication of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zheng, P., Lou, J., Lian, C. et al. A generalized collision algorithm for geometric graphics. Soft Comput 26, 4979–4989 (2022). https://doi.org/10.1007/s00500-022-06883-6

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-022-06883-6

Keywords