Skip to main content
Springer Nature Link
Log in

Algebraic rough sets via algebraic relations

  • Foundation, algebraic, and analytical methods in soft computing
  • Published:
Soft Computing Aims and scope Submit manuscript

Abstract

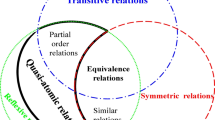
The aim of this paper is to discuss algebraic rough set and its relationships with convex space, rough set and generalized neighborhood space. Specifically, the notion of algebraic relations is introduced and a pair of lower approximation operator and upper approximation operator are presented. Then, several conditions of algebraic relations such as seriality, reflexivity, (resp., weak, primitive) symmetry and (resp., strong) transitivity are characterized by algebraic approximation operators. Based on this, relationships among algebraic rough sets, convex structures and generalized neighborhood systems are investigated. It is proved that the category of reflexive and transitive algebraic rough spaces is isomorphic to the category of convex spaces. In particular, the category of reflexive, weakly symmetric and transitive algebraic rough spaces is isomorphic to the category of convex matroids and the category of reflexive, weakly symmetric and transitive algebraic generalized neighborhood spaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data Availability

Enquiries about data availability should be directed to the authors.

References

  • Chang CL (1968) Fuzzy topological spaces. J Math Anal Appl 24:182–190

    MathSciNet  Google Scholar 

  • Dubois D, Prade H (1990) Rough fuzzy sets and fuzzy rough sets. Int J Gene Syst 17:191–209

    Google Scholar 

  • Han SE, Šostak A (2018) On the measure of \(m\)-rough approximation of \(L\)-fuzzy sets. Soft Comput 22:3843–3855

    Google Scholar 

  • Hong TP, Tseng LH, Wang SL (2002) Learning rules from incomplete training examples by rough sets. Expert Syst Appl 22:285–293

    Google Scholar 

  • Hong TP, Lin CE, Lin JH, Wang SL (2008) Learning cross-level certain and possible rules by rough sets. Expert Syst Appl 34:1698–1706

    Google Scholar 

  • Hońko P (2018) Horizontal decomposition of data table for finding one reduct. Int J Gene Syst 47:208–243

    MathSciNet  Google Scholar 

  • Kelly JL (1955) General topology. Van Nostrand, New York

    Google Scholar 

  • Li LQ, Jin Q, Yao BX, Wu JC (2020) A rough set model based on fuzzifying neighborhood systems. Soft Comput 24:6085–6099

    Google Scholar 

  • Li LQ, Yao BX, Zhan JM, Jin Q (2021) \(L\)-fuzzifying approximation operators derived from general \(L\)-fuzzifying neighborhood systems. Int J Mach Learn Cyber 12:1343–1367

    Google Scholar 

  • Lin TY (1992) Topological and fuzzy rough sets. In: Slowinski R (ed) Decision support by experience-application of the rough set theory. Kluwer Academic Publishers, Boston

    Google Scholar 

  • Lin TY, Liu Q (1994) Rough approximate operators: axiomatic rough set theory. In: Ziarko W (ed) Rough sets fuzzy sets and knowledge discovery. Springer, Berlin

    Google Scholar 

  • Lingras PJ, Yao YY (1998) Data mining using extensions of the rough set model. J Am Soc Inf Sci 49:415–422

    Google Scholar 

  • Liu GL (2008) Generalized rough sets over fuzzy lattices. Inf Sci 178:1651–1662

    MathSciNet  Google Scholar 

  • Liu GL (2013a) The relationship among different covering approximations. Inf Sci 250:178–183

    MathSciNet  Google Scholar 

  • Liu GL (2013b) Using one axiom to characterize rough set approximations. Inf Sci 223:285–296

    Google Scholar 

  • Liu XF, Li ZW, Zhang GQ, Xie NX (2019) Measures of uncertainty for a distributed fuzzy information system. Int J Gen Syst 48:625–655

    MathSciNet  Google Scholar 

  • Ma ZM, Hu BQ (2013) Topological and lattice structures of \(L\)-fuzzy rough sets determined by lower and upper sets. Inf Sci 218:194–204

    MathSciNet  Google Scholar 

  • Mi JS, Zhang WX (2004) An axiomatic characterization of a fuzzy generalization of rough sets. Int Sci 160:235–249

    MathSciNet  Google Scholar 

  • Mi JS, Leung Y, Zhao HY, Feng T (2018) Generalized fuzzy rough sets determined by a triangular norm. Int Sci 178:3203–3213

    MathSciNet  Google Scholar 

  • Morsi NN, Yakout MM (1998) Axioms for fuzzy rough sets. Fuzzy Sets Syst 100:327–342

    Google Scholar 

  • Pang B (2020) Convergence structures in \(M\)-fuzzifying convex spaces. Quaes Math 43:1541–1561

    MathSciNet  Google Scholar 

  • Pang B (2021) Hull operators and interval operators in \((L, M)\)-fuzzy convex spaces. Fuzzy Sets Syst 45:106–127

    MathSciNet  Google Scholar 

  • Pang B, Shi FG (2019) Fuzzy counterparts of hull operators and interval operators in the framework of \(L\)-convex spaces. Fuzzy Sets Syst 369:20–39

    MathSciNet  Google Scholar 

  • Pang B, Mi JS, Xiu ZY (2019) \(L\)-fuzzifying approximation operators in fuzzy rough sets. Inf Sci 480:14–33

    MathSciNet  Google Scholar 

  • Pattaraintakorn P, Cercone N (2008) Integrating rough set theory and medical applications. Appl Math Lett 21:400–403

    MathSciNet  Google Scholar 

  • Pawlak Z (1982) Rough sets. Int J Comput Inf Sci 11:341–356

    Google Scholar 

  • Qin KY, Pei Z (2005) On the topological properties of fuzzy rough sets. Fuzzy Sets Syst 151:601–613

    MathSciNet  Google Scholar 

  • Radzikowska AM, Kerre EE (2002) A comparative study of fuzzy rough sets. Fuzzy Sets Syst 126:137–155

    MathSciNet  Google Scholar 

  • Salamó M, López-Sánchez M (2011) Rough set based approaches to feature selection for case-based reasoning classifiers. Patten Recongnit Lett 32:280–292

    Google Scholar 

  • She Y, He X (2014) Rough approximation operators on \(r_0\)-algebras (nilpotent minimum algebras) with an application in formal logic \(\cal{L} ^*\). Inf Sci 277:71–89

    Google Scholar 

  • She Y, Wang G (2009) An axiomation approach of fuzzy rough set based on residuated lattices. Comput Math Appl 58:189–201

    MathSciNet  Google Scholar 

  • Shi FG, Xiu ZY (2014) A new approach to the fuzzification of convex structures. J Appl Math 2014:1–12

    MathSciNet  Google Scholar 

  • Son CS, Kim YN, Park HS, Kim MS (2012) Decision-making model for early diagnosis of congestive heart failure using rough set and decision tree approaches. J Bio Inf 45:999–1008

    Google Scholar 

  • Šostak A (2010) Towards the theory of \(M\)-approximation systems: fundamentals and examples. Fuzzy Set Syst 161:2440–2461

    MathSciNet  Google Scholar 

  • Syau YR, Lin EB (2014) Neighborhood systems and covering approximation spaces. Knowl Based Syst 66:61–67

    Google Scholar 

  • Thiele H (2000) On axiomatic characterizations of crisp approximation operators. Inf Sci 129:221–226

    Google Scholar 

  • van de Vel MLJ (1993) Theory of convex structures. Noth-Holland, Amsterdam

    Google Scholar 

  • Wang CL (2013) Single axioms for lower fuzzy rough approximation operators determined by fuzzy implications. Inf Sci 336:116–147

    MathSciNet  Google Scholar 

  • Wang CL, Hu BQ (2013) Fuzzy rough sets based on generalized residuated lattices. Inf Sci 248:31–49

    MathSciNet  Google Scholar 

  • Wang H, Wang S (2009) Discovering patterns of missing data in survey databases: an application of rough sets. Expert Syst Appl 36:6256–6260

    Google Scholar 

  • Wu XY, Li EQ (2023) Characterizations of \(L\)-concavities and \(L\)-convexities via derived relations. Hace J Math Stat 52:876–895

    MathSciNet  Google Scholar 

  • Wu WZ, Zhang WX (2004) Constructive and axiomatic approaches of fuzzy approximation operators. Inf Sci 159:233–254

    MathSciNet  Google Scholar 

  • Wu WZ, Leung Y, Shao MW (2013) Generalized fuzzy rough approximation operators determined by fuzzy implications. Int J Approx Reason 54:1388–1409

    Google Scholar 

  • Wu WZ, Leung Y, Guo SM (2015) Using one axiom to characterize fuzzy rough approximation operators determined by a fuzzy implication operator. Fundam Inf 142:87–104

    MathSciNet  Google Scholar 

  • Wu WZ, Xu YH, Shao MW, Wang GY (2016) Axiomatic characterizations of \((s, t)\)-fuzzy rough approximation operators. Inf Sci 20:17–43

    Google Scholar 

  • Yang T, Li QG (2010) Reduction about approximation spaces of covering generalized rough sets. Int J Approx Reason 51:335–345

    MathSciNet  Google Scholar 

  • Yao YY (1982) Constructive and algebraic methods of the theory of rough sets. Inf Sci 109:21–47

    MathSciNet  Google Scholar 

  • Yao YY (1998a) Relational interpretations of neighborhood operators and rough set approximation operators. Inf Sci 111:239–259

    MathSciNet  Google Scholar 

  • Yao YY (1998b) Generalized rough set model. In: Polkowski L, Skowron A (eds) Rough sets in knowledge discovery 1. Methodology and applications. Physica-Verlag, Heidelberg

  • Yao YY, Lin TY (1996) Generalization of rough set using modal logic. Intell Autom Soft Comput 22:103–120

    Google Scholar 

  • Yao YY, Yao BX (2012) Covering based rough set approximations. Inf Sci 200:91–107

    MathSciNet  Google Scholar 

  • Yao W, She YH, Lu LX (2019) Metric-based \(L\)-fuzzy rough sets: approximation operators a nd definable sets. Knowl Based Syst 163:91–102

    Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf Control 8:338–353

    Google Scholar 

  • Zhang YL, Li CQ, Lin ML, Lin YJ (2015) Relationships between generalized rough sets based on covering and reflexive neighborhood system. Inf Sci 319:56–67

    MathSciNet  Google Scholar 

  • Zhao ZG (2016) On some types of covering rough sets from topological points of view. Int J Approx Reason 68:1–14

    MathSciNet  Google Scholar 

  • Zhao FF, Shi FG (2021) \(L\)-fuzzy generalized neighborhood system operator-based \(L\)-fuzzy approximation operators. Int J Gen Syst 50:458–484

  • Zhu W (2007) Generalized rough sets based on relations. Inf Sci 177:4997–5011

    MathSciNet  Google Scholar 

  • Zhu W (2009) Relationship between generalized rough sets based on binary relation and covering. Inf Sci 179:210–225

    MathSciNet  Google Scholar 

  • Zhu W, Wang FY (2003) Reduction and axiomization of covering generalized rough sets. Inf Sci 152:217–230

    MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the anonymous reviewers and the area editor for their careful reading and valuable comments. This work is supported by Doctoral Scientific Research Foundation of Anhui Normal University (751966) and Provincial Postgraduate Academic Innovation Projects (2023xscx037 and 2022xscx041).

Funding

This work is supported by Doctoral Scientific Research Foundation of Anhui Normal University (751966) and Provincial Postgraduate Academic Innovation Projects (2023xscx037 and 2022xscx041).

Author information

Authors and Affiliations

  1. Anhui Normal University, Wuhu, China

    Xiu-Yun Wu & Hui-Min Zhang

  2. School of Mathematics, Hunan University of Science and Engineering, Yongzhou, 425100, China

    Chun-Yan Liao

Authors
  1. Xiu-Yun Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Chun-Yan Liao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Hui-Min Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

The first author provides the main thought and purpose of the paper. He introduces the notion of algebraic relation and characterizes seriality, reflexivity, (resp., weak, primitive) symmetry and (resp., strong) of algebraic relation by algebraic approximation operators. In addition, he also discusses relations between algebraic approximation spaces and generalized neighborhood spaces. The second the third authors discuss relationships among algebraic approximation spaces, approximation spaces and convex spaces. They also provides counterexamples in this paper.

Corresponding author

Correspondence to Xiu-Yun Wu.

Ethics declarations

Conflict of interest

The authors declare that they have no Conflict of interest.

Human and animal rights

This article does not contain any studies with human participants or animals performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, XY., Liao, CY. & Zhang, HM. Algebraic rough sets via algebraic relations. Soft Comput 28, 9157–9177 (2024). https://doi.org/10.1007/s00500-024-09820-x

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-024-09820-x

Keywords