Skip to main content

Advertisement

Springer Nature Link
Log in

Analysis on equilibrium points of cellular neural networks with thresholding activation function

  • Original Article
  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

Abstract

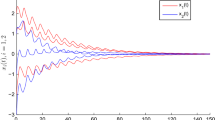
In previous paper, the number of equilibrium points of a cellular neural network (CNN) was studied. However, the region of different values of equilibrium points of every cell in the CNN is not investigated. Therefore, in this paper, the regions of the number of different values of equilibrium points in cellular neural networks (CNNs) with thresholding activation function are obtained by the relationship between parameters of CNNs. The influence of the values of connection weights of CNNs on the number of equilibrium points for a cell is found. Some sufficient conditions are obtained by using the relationship among connection weights. Depending on these sufficient conditions, inputs and outputs of a CNN, the regions of the values of parameters can be obtained. Finally, some numerical simulations are presented to support the effectiveness of the theoretical analysis.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Chua LO, Yang L (1988) Cellular neural networks: theory. IEEE Trans Circuits Syst 35:1257–1272

    Article  MathSciNet  MATH  Google Scholar 

  2. Chua LO, Yang L (1988) Cellular neural networks: applications. IEEE Trans Circuits Syst 35:1273–1290

    Article  MathSciNet  Google Scholar 

  3. Julian P, Dogaru R, Chua LO (2002) A piecewise-linear simplicial coupling cell for CNN gray-level image processing. IEEE Trans Circuits Syst I Fundam Theory Appl 49:904–913

    Article  Google Scholar 

  4. Grassi G, Grieco LA (2003) Object-oriented image analysis using the CNN universal machine: new analogic CNN algorithms for motion compensation, image synthesis, and consistency observation. IEEE Trans Circuits Syst I Fundam Theory Appl 50:488–499

    Article  Google Scholar 

  5. Itoh M, Chua LO (2007) Advanced image processing cellular neural networks. Int J Bifurcation Chaos 17:1109–1150

    Article  MathSciNet  MATH  Google Scholar 

  6. Werblin F, Roska T, Chua LO (1995) The analogic cellular neural network as bionic eye. Int J Circuit Theory Appl 23:541–569

    Article  Google Scholar 

  7. Chua LO, Roska T (2002) Cellular neural networks and visual computing. Cambridge University Press, Cambridge

    Book  Google Scholar 

  8. Zeng ZG, Wang J (2009) Associative memories based on continuous-time cellular neural networks designed using space-invariant cloning templates. Neural Netw 22:651–657

    Article  Google Scholar 

  9. Kaluzny P (1994) Number of stable equilibrium states of cellular neural networks. IEEE Trans Circuits Syst I Fundam Theory Appl 41:608–610

    Article  Google Scholar 

  10. Zheng CD, Lu LB, Wang ZS (2009) New LMT-based delay-dependent criterion for global asymptotic stability of cellular neural networks. Neurocomputing 72:3331–3336

    Article  Google Scholar 

  11. Xia YH, Cao JD, Cheng SS (2007) Global exponential stability of delayed cellular neural networks with impulses. Neurocomputing 70:2495–2501

    Article  Google Scholar 

  12. Zeng ZG, Huang DS, Wang ZF (2005) Memory pattern analysis of cellular neural networks. Phys Lett A 342:114–128

    Article  MATH  Google Scholar 

  13. Zeng ZG, Huang DS, Wang ZF (2008) Pattern memory analysis based on stability theory of cellular neural networks. Appl Math Model 32:112–121

    Article  MATH  Google Scholar 

  14. Zeng ZG, Wang J (2008) Design and analysis of high-capacity associative memories based on a class of discrete-time recurrent neural networks. IEEE Trans Syst Man Cybern Part B Cybern 38:1525–1536

    Article  Google Scholar 

  15. Gilli M, Biey M, Checco P (2004) Equilibrium analysis of cellular neural networks. IEEE Trans Circuits Syst I Regul Pap 51:903–912

    Article  MathSciNet  Google Scholar 

  16. Takahashi N (2000) A new sufficient condition for complete stability of cellular neural networks with delay. IEEE Trans Circuits Syst I Fundam Theory Appl 47:793–799

    Article  Google Scholar 

  17. Li XM, Huang LH, Wu JH (2003) Further results on the stability of delayed cellular neural networks. IEEE Trans Circuits Syst I Fundam Theory Appl 50:1239–1242

    Article  MathSciNet  Google Scholar 

  18. Liao XX, Wang J, Zeng ZG (2005) Global asymptotic stability and global exponential stability of delayed cellular neural networks. IEEE Trans Circuits Syst I Express Briefs 52:403–409

    Article  Google Scholar 

  19. Liao XX, Luo Q, Zeng ZG, Guo YX (2008) Global exponential stability in Lagrange sense for recurrent neural networks with time delays. Nonlinear Anal Real World Appl 9:1535–1557

    Article  MathSciNet  MATH  Google Scholar 

  20. Zheng CD, Zhang HG, Wang ZS (2009) New delay-dependent global exponential stability criterion for cellular-type neural networks with time-varying delays. IEEE Trans Circuits Syst II Express Briefs 56:250–254

    Article  Google Scholar 

  21. Xiao SP, Zhang XM (2009) New globally asymptotic stability criteria for delayed cellular neural networks. IEEE Trans Circuits Syst II Express Briefs 56:659–663

    Article  Google Scholar 

  22. Li CJ, Li CD, Liao XF, Huang TW (2011) Impulsive effects on stability of high-order BAM neural networks with time delays. Neurocomputing 74:1541–1550

    Article  Google Scholar 

  23. Zeng ZG, Wang J (2006) Complete stability of cellular neural networks with time-varying delays. IEEE Trans Circuits Syst I Regul Pap 53:944–955

    Article  MathSciNet  Google Scholar 

  24. Zeng ZG, Wang J (2006) Improved conditions for global exponential stability of recurrent neural networks with time-varying delays. IEEE Trans Neural Netw 17:623–635

    Article  Google Scholar 

  25. Zeng ZG, Wang J (2006) Multiperiodicity and exponential attractivity evoked by periodic external inputs in delayed cellular neural networks. Neural Comput 18:848–870

    Article  MathSciNet  MATH  Google Scholar 

  26. Zeng ZG, Wang J (2007) Analysis and design of associative memories based on recurrent neural networks with linear saturation activation functions and time-varying delays. Neural Comput 19:2149–2182

    Article  MathSciNet  MATH  Google Scholar 

  27. Han Q, Liao X, Li C (2012) Analysis of associative memories based on stability of cellular neural networks with time delay. Neural Comput Appl, published online: 20 Jan 2012

  28. Han Q, Liao X, Weng T, Li C, Huang H (2012) Analysis on equilibrium points of cells in cellular neural networks described using cloning templates. Neurocomputing 89:106–113

    Google Scholar 

  29. Zeng ZG, Wang J, Liao XX (2004) Stability analysis of delayed cellular neural networks described using cloning templates. IEEE Trans Circuits Syst I Regul Pap 51:2313–2324

    Article  MathSciNet  Google Scholar 

  30. Chua LO, Roska T (1993) The CNN paradigm. IEEE Trans Circuits Syst I Fundam Theory Appl 40:147–156

    Article  MathSciNet  MATH  Google Scholar 

  31. Liu D, Michel AN (1993) Cellular neural networks for associative memories. IEEE Trans Circuits Syst II Analog Digit Signal Process 40:119–121

    Article  MATH  Google Scholar 

Download references

Acknowledgments

This work was supported in part by the National Natural Science Foundation of China under Grant 60973114, Grant 61170249 and Grant 61003247, in part by the Natural Science Foundation project of CQCSTC under Grant 2009BA2024, and in part by the State Key Laboratory of Power Transmission Equipment and System Security and New Technology, Chongqing University, under Grant 2007DA10512711206, in part by Teaching and Research Program of Chongqing Education Committee (KJ110401), in part by the First Batch of Supporting Program for University Excellent Talents in Chongqing, and in part by Research Project of Chongqing University of Science and Technology under Grant CK2011Z17.

Author information

Authors and Affiliations

  1. School of Electrical and Information Engineering, Chongqing University of Science and Technology, Chongqing, 401331, China

    Qi Han, Tengfei Weng & Jun Peng

  2. State Key Laboratory of Power Transmission Equipment and System Security, College of Computer Science, Chongqing University, Chongqing, 400030, China

    Qi Han, Xiaofeng Liao, Chuandong Li & Liping Feng

Authors
  1. Qi Han
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Xiaofeng Liao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Tengfei Weng
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Jun Peng
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. Chuandong Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  6. Liping Feng
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Qi Han.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Han, Q., Liao, X., Weng, T. et al. Analysis on equilibrium points of cellular neural networks with thresholding activation function. Neural Comput & Applic 23, 23–29 (2013). https://doi.org/10.1007/s00521-012-1173-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-012-1173-1

Keywords