Abstract
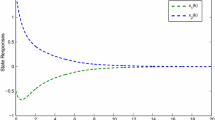
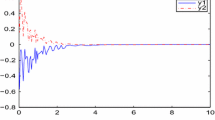
This paper is concerned with the stability problem for a class of impulsive neural networks model, which includes simultaneously parameter uncertainties, stochastic disturbances and two additive time-varying delays in the leakage term. By constructing a suitable Lyapunov–Krasovskii functional that uses the information on the lower and upper bound of the delay sufficiently, a delay-dependent stability criterion is derived by using the free-weighting matrices method for such Takagi–Sugeno fuzzy uncertain impulsive stochastic recurrent neural networks. The obtained conditions are expressed with linear matrix inequalities (LMIs) whose feasibility can be checked easily by MATLAB LMI Control toolbox. Finally, the theoretical result is validated by simulations.



Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Cao J (2003) Global asymptotic stability of a general class of recurrent neural networks with time-varying delays. IEEE Trans Circuits Syst I Fundam Theory Appl 6:34–44
Arik S (2000) Stability analysis of delayed neural networks. IEEE Trans Circuits Syst I Fundam Theory Appl 47(7):1089–1092
Li XD, Cao JD (2010) Delay-dependent stability of neural networks of neutral type with time delay in the leakage term. Nonlinearity 23:1709
Balasubramaniam P, Kalpana M, Rakkiyappan R (2011) Global asymptotic stability of BAM fuzzy cellular neural networks with time delay in the leakage term, discrete and unbounded distributed delays. Math Comput Model 3:839–853
Balasubramaniam P, Kalpana M, Rakkiyappan R (2011) Existence and global asymptotic stability of fuzzy cellular neural networks with time delay in the leakage term and unbounded distributed delays. Circuits Syst Signal Process 6:1595–1616
Long SJ, Song QK, Wang XH, Li DS (2012) Stability analysis of fuzzy cellular neural networks with time delay in the leakage term and impulsive perturbations. J Franklin Inst 9:2461–2479
Gao H, Lam J, Wang C (2006) Robust energy-to-speak filter design for stochastic time-delays systems. Syst Control Lett 55(2):101–111
Song Q, Cao J (2006) Global exponential robust stability of Cohen-Grossberg neural networks with time varying delays and reaction-diffusion terms. J Franklin Inst 343(7):705–719
Wang Z, Shu H, Fang J, Liu X (2006) Robust stability for stochastic Hopfield neural networks with time delays. Nonlinear Anal Real World Appl 7:1119–1128
Gopalsamy K (2007) Leakage delays in BAM. J Math Anal Appl 325:1117–1132
Li XD, Rakkiyappan R (2013) Stability results for Takagi–Sugeno fuzzy uncertain BAM neural networks with time delays in the leakage term. Neural Comput Appl 22:S203–S219
Balasubramaniam P, Vembarasan V, Rakkiyappan R (2012) Global robust asymptotic stability analysis of uncertain switched Hopfield neural networks with time delay in the leakage term. Neural Comput Appl 21:1593–1616
Li J, Hu MF, Cao JD, Yang YQ, Jin YH (2014) Stability of uncertain impulsive stochastic genetic regulatory networks with time-varying delay in the leakage term. Abstr Appl Anal 2014:1–15, Article ID 706720
Duan L, Huang LH (2013) Global exponential stability of fuzzy BAM neural networks with distributed delays and time-varying delays in the leakage terms. Neural Comput Appl 23:S171–S178
Li XD, Fu XL, Balasubramaniam P, Rakkiyappan R (2010) Existence, uniqueness and stability analysis of recurrent neural networks with time delay in the leakage term under impulsive perturbations. Nonlinear Anal Real World Appl 10:4092–4108
Li XD, Rakkiyappan R, Balasubramaniam P (2011) Existence and global stability analysis of equilibrium of fuzzy cellular neural networks with time delay in the leakage term under impulsive perturbations. J Franklin Inst 3:135–155
Balasubramaniam P, Vembarasan V (2011) Asymptotic stability of BAM neural networks of neutral-type with impulsive effects and time delay in the leakage term. Int J Comput Math 88(15): 3271–3291
Li XD, Bohner M (2012) An impulsive delay differential inequality and applications. Comput Math Appl 64:1875–1881
Shao H, Han Q (2011) New delay-dependent stability criteria for neural networks with two additive time-varying delay components. IEEE Trans Neural Netw 22:812–818
Tian JK, Zhong SM (2012) Improved delay-dependent stability criteria for neural networks with two additive time-varying delay components. Neurocomputing 77:114–119
Xiao N, Jia YM (2013) New approaches on stability criteria for neural networks with two additive time-varying delay components. Neurocomputing 118:150–156
Zhu QX, Cao JD (2010) Adaptive synchronization of chaotic Cohen–Crossberg neural networks with mixed time delays. Nonlinear Dyn 3:517–534
Wang Z, Liu Y, Li M, Liu X (2006) Stability analysis for stochastic Cohen–Grossberg neural networks with mixed time delays. IEEE Trans Neural Netw 17:814–820
Blythe S, Mao X, Liao X (2001) Stability of stochastic delay neural networks. J Franklin Inst 338:481–495
Fu XL, Li XD (2011) LMI conditions for stability of impulsive stochastic Cohen–Grossberg neural networks with mixed delays. Commun Nonlinear Sci Numer Simul 16:435–454
Li XD, Rakkiyappan R (2013) Impulsive controller design for exponential synchronization of chaotic neural networks with mixed delays. Commun Nonlinear Sci Numer Simul 18:1515–1523
Gopalsamy K (2004) Stability of artificial neural networks with impulses. Appl Math Comput 154:783–813
Song QK, Wang ZD, Long SJ, Xu DY (2008) Stability analysis of impulsive stochastic Cohen–Grossberg neural networks with mixed time delays. Phys A 387:3314–3326
Rakkiyappan R, Balasubramaniam P, Cao JD (2010) Global exponential stability results for neutral-type impulsive neural networks. Nonlinear Anal Real World Appl 11:122–130
Sakthivel R, Samidurai R, Anthoni SM (2010) New exponential stability criteria for stochastic BAM neural networks with impulses. Phys Scr 82:045802
Sakthivel R, Samidurai R, Anthoni SM (2010) Exponential stability for stochastic neural networks of neutral type with impulsive effects. Mod Phys Lett B 24:1099–1110
Sakthivel R, Raja R, Anthoni SM (2011) Exponential stability for delayed stochastic bidirectional associative memory neural networks with markovian jumping and impulses. J Optim Theory Appl 150:166–187
Li XD, Song SJ (2013) Impulsive control for existence, uniqueness, and global stability of periodic solutions of recurrent neural networks with discrete and continuously distributed delays. IEEE Trans Neural Netw Learn Syst 24:868–877
Song XL, Xin X, Huang WP (2012) Exponential stability of delayed and impulsive cellular neural networks with partially Lipschitz continuous activation functions. Neural Netw 29–30:80–90
Li XD, Akca H, Fu XL (2013) Uniform stability of impulsive infinite delay differential equations with applications to systems with integral impulsive conditions. Appl Math Comput 219:7329–7337
Mathiyalagan K, Sakthivel R, Marshal Anthoni S (2012) Exponential stability result for discrete-time stochastic fuzzy uncertain neural networks. Phys Lett A 376:901–912
Faydasicok O, Arik S (2013) An analysis of stability of uncertain neural networks with multiple time delays. J Franklin Inst 350:1808–1826
Takagi T, Sugeno M (1985) Fuzzy identification of systems and its applications to modeling and control. IEEE Trans Syst Man Cybern 15:116–132
Sakthivel R, Arunkumar A, Mathiyalagan K, Marshal Anthoni S (2011) Robust passivity analysis of fuzzy Cohen–Grossberg BAM neural networks with time-varying delays. Appl Math Comput 218:3799–3809
Muralisankar S, Gopalakrishnan N, Balasubramaniam P (2011) Robust exponential stability criteria for T–S fuzzy stochastic delayed neural networks of neutral type. Circuits Syst Signal Process 30:1617–1641
Rao RF, Pu ZL (2013) Stability analysis for impulsive stochastic fuzzy \(p\)-Laplace dynamic equations under Neumann or Dirichlet boundary condition. Bound Value Probl 2013:133
Park P, Ko JW, Jeong CK (2011) Reciprocally convex approach to stability of systems with time-varying delays. Automatica 47:235–238
Morel JM, Teissier B Almost periodic solution of impulsive differential equations, Lecture Note in Mathematics 2047
Acknowledgments
This work was supported by the Fundamental Research Funds for the Central Universities (JUSRP51317B, JUSRP211A21).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Li, J., Hu, M., Guo, L. et al. Stability of uncertain impulsive stochastic fuzzy neural networks with two additive time delays in the leakage term. Neural Comput & Applic 26, 417–427 (2015). https://doi.org/10.1007/s00521-014-1737-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00521-014-1737-3