Skip to main content
Springer Nature Link
Log in

An efficient computational approach for time-fractional Rosenau–Hyman equation

  • Original Article
  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

Abstract

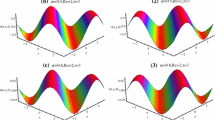
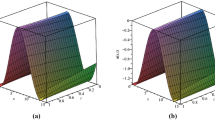
In this work, we concentrate on the analysis of the time-fractional Rosenau–Hyman equation occurring in the formation of patterns in liquid drops via q-homotopy analysis transform technique and reduced differential transform approach. The q-homotopy analysis transform algorithm can provide rapid convergent series by choosing the appropriate values of auxiliary parameters ħ and n at large domain. The reduced differential transform technique gives wider applicability due to reduction in computations and makes the calculation much simpler and easier. The proposed techniques are realistic and free from any assumption and perturbation for solving the time-fractional Rosenau–Hyman equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Atangana A, Alabaraoye E (2013) Solving a system of fractional partial differential equations arising in the model of HIV infection of CD4+ cells and attractor one-dimensional Keller–Segel equations. Adv Differ Equ. doi:10.1186/1687-1847-2013-94

    Article  MATH  Google Scholar 

  2. Rivero M, Trujillo J, Vazquez L, Velasco M (2011) Fractional dynamics of populations. Appl Math Comput 218:1089–1095

    MathSciNet  MATH  Google Scholar 

  3. Chen Y, An YH (2009) Numerical solutions of coupled Burgers equations with time and space fractional derivatives. Appl Math Comput 200:87–95

    MathSciNet  MATH  Google Scholar 

  4. Su X (2009) Boundary value problem for a coupled system of nonlinear fractional differential equations. Appl Math Lett 22:64–69

    Article  MathSciNet  Google Scholar 

  5. Jafari H, Tajadodi H (2015) Numerical solution of the fractional advection-dispersion equation. Progr Fract Differ Appl 1:37–45

    Google Scholar 

  6. Rao SB, Prajapati JC, Patel AD, Shukla AK (2014) Some properties of Wright-type generalized hypergeometric function via fractional calculus. Adv Differ Equ. doi:10.1186/1687-1847-2014-119

    Article  MathSciNet  Google Scholar 

  7. Zhang S (2009) Monotone iterative method for initial value problem involving Riemann–Liouville fractional derivatives. Nonlinear Anal 71:2087–2093

    Article  MathSciNet  Google Scholar 

  8. Kumar D, Singh J, Baleanu D (2016) Numerical computation of a fractional model of differential-difference equation. J Comput Nonlin Dyn 11(6):061004. doi:10.1115/1.4033899

    Article  Google Scholar 

  9. Oldham KB, Spanier J (1974) The fractional calculus: integrations and differentiations of arbitrary order. Academic Press, New York

    MATH  Google Scholar 

  10. Caputo M (1967) Linear models of dissipation whose Q is almost frequency independent. Part II. J R Astron Soc 13:529–539

    Article  Google Scholar 

  11. Samko SG, Kilbas AA, Marichev OI (1993) Fractional integrals and derivatives: theory and applications. Gordon and Breach, London

    MATH  Google Scholar 

  12. Carpinteri A, Mainardi F (1997) Fractional calculus in continuum mechanics. Springer, New York

    Book  Google Scholar 

  13. Podlubny I (1999) Fractional differential equations. Academic Press, San Diego

    MATH  Google Scholar 

  14. Miller KS, Ross B (1993) An introduction to the fractional calculus and fractional differential equations. Wiley, New York

    MATH  Google Scholar 

  15. Khan NA, Ara A, Mahmood A (2010) Approximate solution of time fractional chemical engineering equations: a comparative study. Int J Chem React Eng. doi:10.2202/1542-6580.2156

    Article  Google Scholar 

  16. Maraaba TA, Jarad F, Baleanu D (2008) On the existence and the uniqueness theorem for fractional differential equations with bounded delay within Caputo derivatives. Sci China Ser A Math 51:1775–1786

    Article  MathSciNet  Google Scholar 

  17. Rosenau P, Hyman JM (1993) Compactons: solitons with finite wavelength. Phys Rev Lett 70:564–567

    Article  Google Scholar 

  18. Mihaila B, Cardenas A, Cooper F, Saxena A (2010) Stability and dynamical properties of Rosenau–Hyman compactons using Padè approximants. Phys Rev E. doi:10.1103/PhysRevE.81.056708

    Article  Google Scholar 

  19. Bazeia D, Das A, Losano L, Santos MJ (2010) Traveling wave solutions of nonlinear partial differential equations. Appl Math Lett 23:681–686

    Article  MathSciNet  Google Scholar 

  20. Rus F, Villatoro FR (2007) Self-similar radiation from numerical Rosenau–Hyman compactons. J Comput Phys 227:440–454

    Article  MathSciNet  Google Scholar 

  21. Rus F, Villatoro FR (2007) Padè numerical method for the Rosenau–Hyman compacton equation. Math Comput Simul 76:188–192

    Article  Google Scholar 

  22. Rus F, Villatoro FR (2009) A repository of equations with cosine/sine compactons. Appl Math Comput 215:1838–1851

    MathSciNet  MATH  Google Scholar 

  23. Rus F, Villatoro FR (2008) Numerical methods based on modified equations for nonlinear evolution equations with compactons. Appl Math Comput 204:416–422

    MathSciNet  MATH  Google Scholar 

  24. Molliq RY, Noorani MSM (2012) Solving the fractional Rosenau–Hyman equation via variational iteration method and homotopy perturbation method. Int J Differ Equ 2012. Article ID 472030

  25. El-Tawil MA, Huseen SN (2012) The q-homotopy analysis method (q- HAM). Int J Appl Math Mech 8:51–75

    Google Scholar 

  26. El-Tawil MA, Huseen SN (2013) On convergence of the q-homotopy analysis method. Int J Contemp Math Sci 8:481–497

    Article  MathSciNet  Google Scholar 

  27. Liao SJ (1992) The proposed homotopy analysis technique for the solution of nonlinear problems. Ph.D. Thesis, Shanghai Jiao Tong University

  28. Liao SJ (2003) Beyond perturbation: introduction to the homotopy analysis method. Chaoman and Hall/CRC Press, Boca Raton

    Book  Google Scholar 

  29. He JH (1999) Homotopy perturbation technique. Comput Methods Appl Mech Eng 178:257–262

    Article  MathSciNet  Google Scholar 

  30. He JH (2003) Homotopy perturbation method: a new nonlinear analytical technique. Appl Math Comput 135:73–79

    MathSciNet  MATH  Google Scholar 

  31. He JH (2006) New interpretation of homotopy perturbation method. Int J Mod Phys B 20:2561–2568

    Article  Google Scholar 

  32. Khuri SA (2001) A Laplace decomposition algorithm applied to a class of nonlinear differential equations. J Appl Math 1:141–155

    Article  MathSciNet  Google Scholar 

  33. Khan Y, Wu Q (2011) Homotopy perturbation transform method for nonlinear equations using He’s polynomials. Comput Math Appl 61(8):1963–1967

    Article  MathSciNet  Google Scholar 

  34. Kumar D, Singh J, Kumar S (2015) A fractional model of Navier–Stokes equation arising in unsteady flow of a viscous fluid. J Assoc Arab Univ Basic Appl Sci 17:14–19

    Google Scholar 

  35. Kumar S, Kumar A, Kumar D, Singh J, Singh A (2015) Analytical solution of Abel integral equation arising in astrophysics via Laplace transform. J Egypt Math Soc 23(1):102–107

    Article  MathSciNet  Google Scholar 

  36. Khan M, Gondal MA, Hussain I, Karimi Vanani S (2012) A new comparative study between homotopy analysis transform method and homotopy perturbation transform method on semi-infinite domain. Math Comput Model 55:1143–1150

    Article  MathSciNet  Google Scholar 

  37. Kumar D, Singh J, Kumar S, Sushila (2014) Numerical computation of Klein-Gordon equations arising in quantum field theory by using homotopy analysis transform method. Alex Eng J 53(2):469–474

    Article  Google Scholar 

  38. Yin XB, Kumar S, Kumar D (2015) A modified homotopy analysis method for solution of fractional wave equations. Adv Mech Eng 7(12):1–8

    Article  Google Scholar 

  39. Keskin Y, Oturanc G (2010) Reduced differential transform method: a new approach to factional partial differential equations. Nonlinear Sci Lett A 1:61–72

    Google Scholar 

  40. Gupta PK (2011) Approximate analytical solutions of fractional Benney–Lin equation by reduced differential transform method and the homotopy perturbation method. Comput Math Appl 58:2829–2842

    Article  MathSciNet  Google Scholar 

  41. Srivastava VK, Awasthi MK, Tamsir M (2013) RDTM solution of Caputo time fractional-order hyperbolic telegraph equation. AIP Adv. doi:10.1063/1.4799548

    Article  Google Scholar 

  42. Caputo M (1969) Elasticita e dissipazione. Zani-Chelli, Bologna

    Google Scholar 

  43. Kilbas AA, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations. Elsevier, Amsterdam

    MATH  Google Scholar 

  44. Clarkson PA, Mansfield EL, Priestley TJ (1997) Symmetries of a class of nonlinear third-order partial differential equations. Math Comput Modell 25(8–9):195–212

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors are highly grateful to the referees for their invaluable suggestions and comments for the improvement of this article.

Author information

Authors and Affiliations

  1. Department of Mathematics, JECRC University, Jaipur, Rajasthan, 303905, India

    Jagdev Singh & Devendra Kumar

  2. Department of Mathematics, Arya Institute of Engineering and Technology, Riico Kukas, Jaipur, Rajasthan, 303101, India

    Ram Swroop

  3. Department of Mathematics, National Institute of Technology, Jamshedpur, Jharkhand, 831014, India

    Sunil Kumar

Authors
  1. Jagdev Singh
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Devendra Kumar
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Ram Swroop
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Sunil Kumar
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jagdev Singh.

Ethics declarations

Conflict of interest

The authors declare that there is no conflict of interests regarding the publication of this paper.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Singh, J., Kumar, D., Swroop, R. et al. An efficient computational approach for time-fractional Rosenau–Hyman equation. Neural Comput & Applic 30, 3063–3070 (2018). https://doi.org/10.1007/s00521-017-2909-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-017-2909-8

Keywords