Skip to main content
Springer Nature Link
Log in

A study of heat transfer analysis for squeezing flow of a Casson fluid via differential transform method

  • Original Article
  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

Abstract

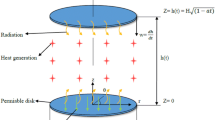
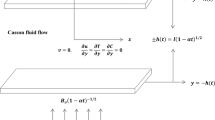
In this article, differential transform method is proposed and applied for semi-analytic solution of heat transfer analysis for the squeezing flow of a Casson fluid between parallel circular plates. Similarity transformation reduces this model into an equivalent system of two strongly nonlinear ordinary differential equations. Fourth-order Runge–Kutta method has also been applied to support our analytical solution, and the comparison shows an excellent agreement.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Abbreviations

\( z = \pm l\sqrt {1 - \alpha t} \) :

Distance between two plates

\( \mu_{B} \) :

Dynamic viscosity of the non-Newtonian fluid

\( p_{y} \) :

Stress of fluid

\( \pi \) :

Product of component of deformation rate

\( e_{ij} \) :

Deformation rate

\( \hat{u} \) and \( \hat{v} \) :

Velocity components in \( \hat{x} \) and \( \hat{y} \) directions

\( \hat{p} \) :

Pressure

T :

Temperature parameter

m :

Kinematic viscosity

\( \beta = \mu_{{\mathbf{B}}} \sqrt {2\pi_{c} } /p_{y} \) :

Casson fluid parameter

q :

Density

C p :

Specific heat

k :

Thermal conductivity

\( S = \frac{{\alpha l^{2} }}{2v} \) :

Non-dimensional squeeze number

\( Pr = \frac{{\mu C_{p} }}{k} \) :

Prandtl number

\( C_{f} = v\left( {1 + \frac{1}{\beta }} \right)\frac{{\left( {\frac{{\partial \hat{u}}}{{\partial \hat{y}}}} \right)_{{\hat{y} = h\left( t \right)}} }}{{v_{w}^{2} }} \) :

Skin friction

\( Nu = \frac{{ - lk\left( {\frac{\partial T}{{\partial \hat{y}}}} \right)_{{\hat{y} = h\left( t \right)}} }}{{kT_{H} }} \) :

Nusselt number

\( Ec = \frac{1}{{C_{p} }}\left( {\frac{{\alpha \hat{x}}}{{2\left( {1 - \alpha t} \right)}}} \right)^{2} \) :

Eckert number

S :

Squeeze number describes movement of the plates

References

  1. Stefan MJ (1874) Versuch, Uber die scheinbare adhesion, Sitzungsberichte der Akademie der Wissenschaften in Wien. Math Naturwissen 69:713–721

    Google Scholar 

  2. Reynolds O (1886) On the theory of lubrication and its application to Mr. Beauchamp Tower’s experiments, including an experimental determination of the viscosity of olive oil. Philos Trans R Soc Lond 177:157–234

    Article  Google Scholar 

  3. Archibald FR (1956) Load capacity and time relations for squeeze films. J Lubr Technol 78(A):231–245

    Google Scholar 

  4. Grimm RJ (1976) Squeezing flows of Newtonian liquid films: an analysis includes the fluid inertia. Appl Sci Res 32:149–166

    Article  Google Scholar 

  5. Wolfe WA (1965) Squeeze film pressures. Appl Sci Res 14:77–90

    Article  Google Scholar 

  6. Kuzma DC (1968) Fluid inertia effects in squeeze films. Appl Sci Res 18:15–20

    Article  Google Scholar 

  7. Tichy JA, Winer WO (1970) Inertial considerations in parallel circular squeeze film bearings. J Lubr Technol 92:588–592

    Article  Google Scholar 

  8. Rashidi MM, Shahmohamadi H, Dinarvand S (2008) Analytic approximate solutions for unsteady two-dimensional and axisymmetric squeezing flows between parallel plates. Math Probl Eng. doi:10.1155/2008/935095

    Article  MathSciNet  MATH  Google Scholar 

  9. Siddiqui AM, Irum S, Ansari AR (2008) Unsteady squeezing flow of a viscous MHD fluid between parallel plates, a solution using the homotopy perturbation method. Math Model Anal 13:565–576

    Article  MathSciNet  Google Scholar 

  10. Domairry G, Aziz A (2009) Approximate analysis of MHD squeeze flow between two parallel disks with suction or injection by homotopy perturbation method. Math Probl Eng. doi:10.1155/2009/603916

    Article  MATH  Google Scholar 

  11. Duwairi HM, Tashtoush B, Damseh RA (2004) On heat transfer effects in a viscous fluid squeezed and extruded between two parallel plates. Heat Mass Transf 41:112–117

    Article  Google Scholar 

  12. Mustafa M, Hayat T, Obaidat S (2012) On heat and mass transfer in the unsteady squeezing flow between parallel plates. Meccanica. doi:10.1007/s11012-012-9536-3

    Article  MathSciNet  MATH  Google Scholar 

  13. Tashtoush B, Tahat M, Probert SD (2001) Heat transfer and radial flows via a viscous fluid squeezed between two parallel disks. Appl Energy 68:275–288

    Article  Google Scholar 

  14. Bahadir AR, Abbasov T (2011) A numerical approach to hydromagnetic squeezed flow and heat transfer between two parallel disks. Ind Lubr Tribol 63:63–71

    Article  Google Scholar 

  15. Mrill EW, Benis AM, Gilliland ER, Sherwood TK, Salzman EW (1965) Pressure flow relations of human blood hollow fibers at low flow rates. J Appl Physiol 20:954–967

    Article  Google Scholar 

  16. McDonald DA (1974) Blood flows in arteries, 2nd edn. Arnold, London

    Google Scholar 

  17. Noor MA, Mohyud-Din ST (2007) Variational iteration technique for solving higher order boundary value problems. Appl Math Comput 189:1929–1942

    MathSciNet  MATH  Google Scholar 

  18. Noor MA, Mohyud-Din ST, Waheed A (2008) Variation of parameters method for solving fifth-order boundary value problems. Appl Math Inf Sci 2:135–141

    MathSciNet  MATH  Google Scholar 

  19. Khan U, Khan SI, Bano S, Mohyud-Din ST (2016) Heat transfer analysis for squeezing flow of a Casson fluid between parallel plates. Ain Shams Eng J 7(1):497–504

    Article  Google Scholar 

  20. Ellahi R, Hameed M (2012) Numerical analysis of steady flows with heat transfer, MHD and nonlinear slip effects. Int J Numer Methods Heat Fluid Flow 22(1):24–38

    Article  Google Scholar 

  21. Sheikholeslami M, Ellahi R, Hassan M, Soleimani S (2014) A study of natural convection 2 heat transfer in a nanofluid filled enclosure with elliptic inner cylinder. Int J Numer Methods Heat Fluid Flow 24(8):1906–1927

    Article  Google Scholar 

  22. Nawaz M, Zeeshan A, Ellahi R, Abbasbandy S, Rashidi S (2015) Joules heating effects on stagnation point flow over a stretching cylinder by means of genetic algorithm and Nelder-Mead method. Int J Numer Methods Heat Fluid Flow 25(3):665–684

    Article  Google Scholar 

  23. Sheikholeslami M, Rashidi MM (2016) “Non-uniform magnetic field effect on nanofluid hydrothermal treatment considering Brownian motion and thermophoresis effects. J Braz Soc Mech Sci Eng 38:1171–1184

    Article  Google Scholar 

  24. Borkakoti AK, Bharali A (1982) Hydromagnetic flow and heat transfer between two horizontal plates, the lower plate being a stretching sheet. Q Appl Math 40(4):461–467

    Article  MathSciNet  Google Scholar 

  25. Rashidi MM, Freidoonimehr N, Hosseini A, Anwar Bég O, Hung T-K (2014) Homotopy simulation of nanofluid dynamics from a nonlinearly stretching isothermal permeable sheet with transpiration. Meccanica 49(2):469–482

    Article  Google Scholar 

  26. Anwar Bég O, Rashidi MM, Bég TA, Asadi M (2012) Homotopy analysis of transient magnetobio-fluid dynamics of micropolar squeeze film in a porous medium: a model for magnetobio-rheological lubrication. J Mech Med Biol 12(03):1250051. doi:10.1142/S0219519411004642

    Article  Google Scholar 

  27. Rashidi MM, Erfani E (2012) Analytical method for solving steady MHD convective and slip flow due to a rotating disk with viscous dissipation and ohmic heating. Eng Comput 29(6):562–579

    Article  Google Scholar 

  28. Haq RU, Nadeem S, Khan ZH, Noor NFM (2015) MHD squeezed flow of water functionalized metallic nanoparticles over a sensor surface. Phys E 73:45–53

    Article  Google Scholar 

  29. Haq RU, Noor NFM, Khan ZH (2016) Numerical simulation of water based magnetite nanoparticles between two parallel disks. Adv Powder Technol 27(4):1568–1575. doi:10.1016/j.apt.2016.05.020

    Article  Google Scholar 

  30. Zhou JK (1986) Differential transformation and its applications for electrical circuits. Huazhong University Press, Wuhan (in Chinese)

    Google Scholar 

  31. Parsa AB, Rashidi MM, Anwar Bég O, Sadri SM (2013) Semi computational simulation of magneto-hemodynamic flow in a semi-porous channel using optimal homotopy and differential transform methods. Comput Biol Med 43(9):1142–1153

    Article  Google Scholar 

  32. Chen CK, Ho SH (1999) Solving partial differential equations by two dimensional differential transform method. Appl Math Comput 106:171–179

    MathSciNet  MATH  Google Scholar 

  33. Ayaz F (2004) Solutions of the systems of differential equations by differential transform method. Appl Math Comput 147:547–567

    MathSciNet  MATH  Google Scholar 

  34. Abdel-Halim Hassan IH (2008) Comparison differential transformation technique with Adomian’s decomposition method for linear and nonlinear initial value problems. Chaos Solitons Fractals 36:53–65

    Article  MathSciNet  Google Scholar 

  35. Casson N (1959) A flow equation for pigment-oil suspension of the printing ink-type. In: Rheology of disperse systems. Pergamon, London, pp 84–104

  36. Nakamura M, Sawada T (1987) Numerical study on the laminar pulsatile flow of slurries. J Non-Newton Fluid Mech 22:191–206

    Article  Google Scholar 

  37. Nakamura M, Sawada T (1988) Numerical study on the flow of a Non-Newtonian fluid through an axisymmetric stenosis. J Biomech Eng 110:137–143

    Article  Google Scholar 

  38. Huilgol RR (2015) Fluid mechanics of viscoplasticity. Springer, Berlin

    Book  Google Scholar 

Download references

Acknowledgements

Authors are highly grateful to the unknown referees’ for their valuable comments.

Author information

Authors and Affiliations

  1. Faculty of Sciences, HITEC University, Taxila Cantt, Pakistan

    Syed Tauseef Mohyud-Din & Muhammad Hamid

  2. School of Mathematical Science, Peking University, Beijing, 100871, China

    Muhammad Usman & Wei Wang

Authors
  1. Syed Tauseef Mohyud-Din
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Muhammad Usman
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Wei Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Muhammad Hamid
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Syed Tauseef Mohyud-Din.

Ethics declarations

Conflict of interest

All the authors declare that there is no conflict of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mohyud-Din, S.T., Usman, M., Wang, W. et al. A study of heat transfer analysis for squeezing flow of a Casson fluid via differential transform method. Neural Comput & Applic 30, 3253–3264 (2018). https://doi.org/10.1007/s00521-017-2915-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-017-2915-x

Keywords