Hysteretic upscaled constitutive relationships for vertically integrated porous media flow

Abstract

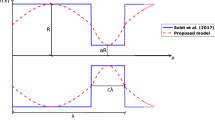
Subsurface two-phase flow in porous media often takes place in reservoirs with a high ratio between the associated lateral and vertical extent and the lateral and vertical flow time scales. This allows for a two-scale approach with effective quantities for two-dimensional horizontal flow equations obtained from reconstructed hydrostatic vertical pressure and saturation distributions. Here, we derive explicit expressions for the two dimensional constitutive relationships for a play-type hysteretic Brooks–Corey capillary pressure function with a pore-size distribution index of 2 and quadratic relative permeabilities. We obtain an explicit hysteretic parametrization for the upscaled capillary pressure function and the upscaled relative permeabilities. The size of the hysteresis loop depends on the ratio between buoyancy and the entry pressure, i.e. it scales with the reservoir height and the ratio between drainage and imbibition capillary pressure. We find that the scaling for the relative permeability is non-monotonic and hysteresis vanishes for both small and large reservoirs.

Appendix

1.1 Limits of capillary pressure relations for primary processes

For $B\rightarrow 0$ the branch $Z^{{\chi }}_1(S_w)$ is relevant. Hence, for the limit $\lim _{B\rightarrow 0}P_c^{{\chi }}$ we write

$$\begin{aligned} P_c^{{\chi }}(S_w)&= p_e^dB\left[ 1-\frac{1}{2}\left( 1-\sqrt{1+\frac{4{\varGamma }^2}{B^2\,S_w}}\right) \right] \nonumber \\&= \frac{p_e^d}{2}\left( B+\sqrt{B^2+\frac{4{\varGamma }^2}{S_w}}\right) \mathop {=}\limits ^{B= 0}p_c^{{\chi }}(S_w), \end{aligned}$$

(80)

where first the square root was approximated by its Taylor expansion and in the second approximation terms of order $B$ and higher have been neglected.

For $B\rightarrow \infty$ the branch $Z^{{\chi }}_2(S_w)$ is relevant. Therefore we write for the limit $\lim _{B\rightarrow \infty }P_c^{{\chi }}$

$$\begin{aligned} P_c^{{\chi }}(S_w)&= p_e^dB\left( 1\!-\! \frac{1\!+\!S_w}{2}\!+\!\frac{{\varGamma }}{B}\!+\!\sqrt{\frac{4{\varGamma }\!+\!B}{4B}\!-\!\frac{2{\varGamma }\!+\!B}{2B}S_w\!+\!\frac{S_w^2}{4}} \right) \nonumber \\&= p_e^d\left[ \frac{B}{2}(1\!-\!S_w)\!+\!{{\varGamma }}\!+\!\frac{B}{2}\left( 1\!-\!S_w\right) \sqrt{1\!+\!\frac{4{\varGamma }}{B}\frac{1}{1-S_w}} \right] \nonumber \\&\!\!\!\! \mathop {\approx }\limits ^{B\gg 1} p_e^d\left[ \frac{B}{2}(1\!-\!S_w)\!+\!{{\varGamma }}\!+\!\frac{B}{2}\left( 1\!-\!S_w\right) \left( 1\!+\!\frac{2{\varGamma }}{B}\frac{1}{1\!-\!S_w}\right) \right] \nonumber \\&= p_e^d\left[ B(1-S_w)\!+\!2{\varGamma }\right] , \end{aligned}$$

(81)

where the square root was approximated by its Taylor expansion. From Eq. (81) we immediately obtain the limit of the difference between drainage and imbibition as

$$\begin{aligned} \lim \limits _{B\rightarrow \infty }P_c^{d}(S_w)-P_c^{i}(S_w)= 2p_e^d(1-{\varPi }). \end{aligned}$$

(82)

1.2 Explicit expressions for secondary processes

Explicit analytic expressions for the relationship between coarse scale saturations and the position of vanishing fine-scale capillary pressure are given.

1.2.1 Drainage followed by an imbibition

The three functions for the position of the vanishing capillary pressure for a drainage followed by an imbibition are given by

$$\begin{aligned}&\!\!\! \! Z^{{di}}_1(S_w,{S^t})\nonumber \\&\quad = \frac{1}{ 2 B^2 S_w( Z^{d}_1-1) -2 } \bigg [2 {\varPi }-1 - (2 {\varPi }+B^2 S_w) Z^{d}_1 \nonumber \\&\qquad \left. + B^2 S_w{Z^{d}_1}^2 - \sqrt{ B^2 S_w( Z^{d}_1-1) Z^{d}_1 -1 } \right. \nonumber \\&\qquad \times \left. \sqrt{ 4 ({\varPi }^2- {\varPi }) ( Z^{d}_1-1) -1+ B^2 S_w( Z^{d}_1-1) Z^{d}_1}\right] \nonumber \\ \end{aligned}$$

(83)

and

$$\begin{aligned}&\!\!\! \! Z^{{di}}_2(S_w,{S^t})= \frac{1}{2 ( B^2 Z^{d}_1 -B^2)}\nonumber \\&\; \times \bigg [ 2 B{\varPi }-1 - B^2 S_w+ (B^2 S_w- B^2 - 2 B{\varPi })Z^{d}_1 + B^2 {Z^{d}_1}^2 \nonumber \\&\; \left. + \sqrt{ 2 B-1 \!-\! B^2 S_w\!+\! (B^2 S_w\!-\! 2 B\!+\! B^2) Z^{d}_1 \!-\! B^2 {Z^{d}_1}^2} \right. \nonumber \\&\;\times \left. \sqrt{ 2 B(2{\varPi }{-}1 ){-}1 {-} B^2 S_w{+} (2 B{+}B^2 {-} 4 B{\varPi }{+} B^2 S_w) Z^{d}_1 {-} B^2 {Z^{d}_1}^2}\,\right] \nonumber \\ \end{aligned}$$

(84)

and

$$\begin{aligned}&\!\!\!\! Z^{{di}}_3(S_w,{S^t})= \frac{1}{2 ( B^2 Z^{d}_2 -B^2)}\nonumber \\&\;\times \bigg [ 2 B{\varPi }-1 - B^2 S_w+ ( B^2 S_w- B^2 - 2 B{\varPi })Z^{d}_2 + B^2 {Z^{d}_2}^2 \nonumber \\&\; \left. + \sqrt{ 2 B-1- B^2 S_w+ (B^2 S_w- 2 B+ B^2 )Z^{d}_2 - B^2 {Z^{d}_2}^2} \right. \nonumber \\&\;\times \left. \sqrt{2 B(2{\varPi }\!-\!1 )\!-\!1 \!-\! B^2 S_w\!+\!( 2 B\!+\! B^2 \!-\! 4 B{\varPi }\!+\! B^2 S_w)Z^{d}_2 \!-\! B^2 {Z^{d}_2}^2}\right] .\nonumber \\ \end{aligned}$$

(85)

In Eqs. (83) and (84) the argument of $Z^{d}_1=Z^{d}_1({S^t})$ and in Eq. (85) the argument of $Z^{d}_2=Z^{d}_2({S^t})$ have been omitted to improve the readability.

1.2.2 Imbibition followed by a drainage

The three functions for the position of the vanishing capillary pressure for an imbibition followed by a drainage are given by

$$\begin{aligned}&\!\!\!\!\! Z^{{id}}_1(S_w,{S^t})=\frac{1}{2 [{\varPi }^2 - B^2 S_w( Z^{i}_1-1)]}\nonumber \\&\times \bigg [ {\varPi }^2 -2 {\varPi }+ (2 {\varPi }+ B^2 S_w)Z^{i}_1 - B^2 S_w{Z^{i}_1}^2 \nonumber \\&\left. - \sqrt{4 [ B^2 S_w(Z^{i}_1{-}1) {-}{\varPi }^2] ( Z^{i}_1{-}1) Z^{i}_1 {+} [{\varPi }^2 {+} 2 {\varPi }( Z^{i}_1{-}1) {-} B^2 S_w( Z^{i}_1{-}1) Z^{i}_1]^2}\!\right] \nonumber \\ \end{aligned}$$

(86)

and

$$\begin{aligned}&\!\!\!\!\! Z^{{id}}_2(S_w,{S^t})=\frac{1}{ 2 [{\varPi }^2 - B^2 S_w( Z^{i}_2-1)]}\nonumber \\&\times \bigg [ {\varPi }^2 -2 {\varPi }+ (2 {\varPi }+ B^2 S_w) Z^{i}_2 - B^2 S_w{Z^{i}_2}^2 \nonumber \\&\left. - \sqrt{4 [ B^2 S_w(Z^{i}_2{-}1) {-}{\varPi }^2] ( Z^{i}_2{-}1) Z^{i}_2 {+} [{\varPi }^2 {+} 2 {\varPi }( Z^{i}_2{-}1) {-} B^2 S_w( Z^{i}_2{-}1) Z^{i}_2]^2}\!\right] \nonumber \\ \end{aligned}$$

(87)

and

$$\begin{aligned}&\!\!\!\!\! Z^{{id}}_3(S_w,{S^t})= \frac{1}{2 ( B^2 Z^{i}_2-B^2)}\nonumber \\&\times \bigg [2 B- {\varPi }^2 - B^2 S_w+ ( B^2 S_w- 2 B- B^2 )Z^{i}_2 + B^2 {Z^{i}_2}^2 \nonumber \\&+ \left( \left[ {\varPi }^2 -2 B+ B^2 S_w+ (2 B+ B^2 - B^2 S_w)Z^{i}_2 - B^2 {Z^{i}_2}^2\right] ^2\right. \nonumber \\&- 4 \left[ B^2Z^{i}_2 -B^2\right] \nonumber \\&\left. \left. {\times }\left[ \! 2 {\varPi }{-}1 {-} {\varPi }^2 {+}(1 {-} 2 {\varPi }{+} 2 B{-} B^2 S_w)Z^{i}_2 {+} (B^2 S_w{-} 2 B){Z^{i}_2}^2\right] \right) ^{\frac{1}{2}}\right] . \end{aligned}$$

(88)

In Eq. (86) the argument of $Z^{i}_1=Z^{i}_1({S^t})$ and in Eqs. (87) and (88) the argument of $Z^{i}_2=Z^{i}_2({S^t})$ have been omitted to improve the readability.