Skip to main content
Springer Nature Link
Log in

Robust synchronization of linear multi-agent system with input/output constraints

  • Original Article
  • Published:
Artificial Life and Robotics Aims and scope Submit manuscript

Abstract

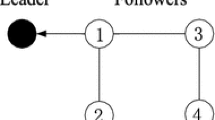
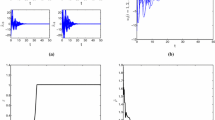
This paper is concerned with distributed cooperative control of linear uncertain agents with constraints on the amplitudes of inputs and outputs. We derive a condition for achieving both state synchronization and input/output constraints in the presence of norm-bounded uncertainties in the agent models. We develop a synthesis method of the robustly synchronizing state feedback gain in terms of linear matrix inequalities based on the notion of positively invariant sets. The desired feedback gain can be efficiently obtained by solving a certain convex programming whose size is independent of the number of agents.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Olfati-Saber R, Fax JA, Murray RM (2007) Consensus and cooperation in networked multi-agent systems. Proc IEEE 95(1):215–233

    Article  Google Scholar 

  2. Mesbahi M, Egerstedt M (2010) Graph theoretic methods in multi-agent networks. Princeton University Press, Princeton

    Book  Google Scholar 

  3. Blanchini F (1999) Set invariance in control—a survey. Automatica 35(11):1747–1767

    Article  MathSciNet  Google Scholar 

  4. Blanchini F, Miani S (2008) Set-theoretic methods in control. Birkhäuser, Boston

    MATH  Google Scholar 

  5. Godsil C, Royle G (2001) Algebraic graph theory. Springer, New York

    Book  Google Scholar 

  6. Zhang H, Lewis FL, Das A (2011) Optimal design for synchronization of cooperative systems: state feedback, observer, and output feedback. IEEE Trans Autom Control 56(8):1948–1952

    Article  MathSciNet  Google Scholar 

  7. Lewis F, Zhang H, Hengster-Movic K, Das A (2014) Cooperative control of multi-agent systems: optimal and adaptive design approaches. Springer, London

    Book  Google Scholar 

  8. Boyd S, El Ghaoui L, Feron E, Balakrishnan V (1994) Linear matrix inequalities in system and control theory. SIAM, Philadelphia

    Book  Google Scholar 

  9. Trentelman HL, Willems JC (1999) The dissipation inequality and the algebraic Riccati equation. In: Bittanti S, Laub AJ, Willems JC (eds) The Riccati equation. Springer, Berlin

    Google Scholar 

  10. Takaba K (2017) Synchronization of linear agents with input/output constaints. IFAC PapersOnline 50(1):1817–1821

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Electrical and Electronic Engineering, College of Science and Engineering, Ritsumeikan University, Kyoto, Japan

    Kiyotsugu Takaba

Authors
  1. Kiyotsugu Takaba
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Kiyotsugu Takaba.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Takaba, K. Robust synchronization of linear multi-agent system with input/output constraints. Artif Life Robotics 23, 577–584 (2018). https://doi.org/10.1007/s10015-018-0491-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10015-018-0491-5

Keywords