Abstract
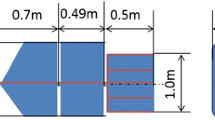
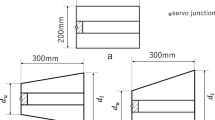
A fish-type balloon robot (FBR) is a biomimetic robot that introduces twisting and pectoral fin motions into an airship robot. The FBR can move back and forth, right and left, and up and down using the twisting and pectoral fin motions. The FBR can propel itself without using a propeller; therefore, it does not injure people during accidental collisions. From this perspective, the FBR can be considered very safe. Therefore, the FBR can be used as a monitoring satellite and an advertising platform. In this paper, we construct an automatic control system for such practical FBR applications. The FBR cannot skid sideways; therefore, it can be considered a robot with nonholonomic constrains, based on the Brockett’s study. In this research, we apply control using a time–state control form. The outline of this control method is as follows. First, we converted the FBR motion model into a time–state control form using a time state and a state control unit. Second, we constructed a feedback control system that stabilized the state control unit. Third, we measured the propulsion characteristics of the FBR using the pectoral fin motion, which is necessary for controlling the FBR’s motion using the time–state control form and for analyzing the measurement results. In addition, we varied the pectoral fin motion to simulate how the FBR’s propulsion and turning angular velocities become similar. Finally, based on the measurement results, we constructed and executed a simulated control system.









Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Haga M, Uchida M (2017) Propulsion modeling of caudal fin driving system on balloon fish robot. Artif Life Robot 22(1):10–16
Brockett R, Millman R, Sussuman H (1983) Differential geometric control theory. Springer Verlag, Berlin, pp 181–208
Coron JM (1990) A necessary condition for feedback stabilization. Syst Control Lett 14:227–232
Sampei M (1994) A control strategy for a class of nonholonomic systems—time-state control form and its application. In: Proceedings of 33rd IEEE conference on decision and control, pp 1120–1121
Sampei M, Kiyota H, Koga M et al (1995) Time-state control form and its application to a non-holonomic space robot. In: Proceedings of IFAC NOLCOS’95, pp 759–764
Astolfi A (1996) Discontinuous control of nonholonomic systems. Syst Control Lett 27:37–45
Imura J, Kobayashi K, Yoshikawa T (1996) Exponential stabilization problem of nonholonomic chained system with specified transient response. In: Proceedings of 35th IEEE conference on decision and control, pp 4733–4738
Sampei M (1997) Feedback control of nonholonomic system (in Japanese). Soc Instrum Control Eng 36(6):396–403
Matsumoto T, Nakaura S, Sampei M (2007) Classification and control for driftless system in view of parameter error in coordinate transformation. In: 7th IFAC symposium on nonlinear control system, pp 461–466
Acknowledgements
Part of this work ß was supported by JSPSKAK-ENHI Grant-in-Aid for Scientific Research (C) Number 17K00477. The authors would like to thank Enago (www.enago.jp) for the English language review.
Author information
Authors and Affiliations
Corresponding authors
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
About this article
Cite this article
Miyamae, H., Uchida, M. Constructing a control system using a time–state control form for a fish-type balloon robot. Artif Life Robotics 25, 316–321 (2020). https://doi.org/10.1007/s10015-019-00581-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10015-019-00581-7