Skip to main content

Advertisement

Springer Nature Link
Log in

Shipment in a multi-choice environment: a case study of shipping carriers in US

  • Published:
Central European Journal of Operations Research Aims and scope Submit manuscript

Abstract

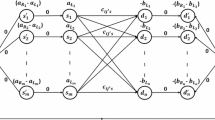
This research paper studies a bi-objective multi-choice scenario faced by a decision maker while shipping packets across a transportation network. A varying range of carriers with multiple time and cost options give rise to multi-choice parameters. In order to equip the decision maker for solving this multi-choice time–cost trade-off scenario, a multi-choice bi-objective transportation problem with fuzzy as well as crisp parameters is modeled. Firstly, a fuzzy transportation problem covering time critical services from a single shipping carrier is solved with the objective of minimizing total shipment cost along with the makespan. Further, the same problem has been analyzed considering multiple carriers for non-time critical services. The algorithms are validated on a use case covering ten major cities of the US with the time–cost data from UPS, FedEx and USPS.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  • Ahuja RK (1986) Algorithms for the minimax transportation problem. Nav Res Logist Q 33(4):725–739

    Article  Google Scholar 

  • Bellman RE, Zadeh LA (1970) Decision-making in a fuzzy environment. Manag Sci 17(4):B-141

    Article  Google Scholar 

  • Bhatia HL, Swarup K, Puri MC (1977) A procedure for time minimization transportation problem. Indian J Pure Appl Math 8(8):920–929

    Google Scholar 

  • Chakraborty D, Jana DK, Roy TK (2015) A new approach to solve multi-objective multi-choice multi-item Atanassov’s intuitionistic fuzzy transportation problem using chance operator. J Intell Fuzzy Syst 28(2):843–865

    Article  Google Scholar 

  • Chanas S, Kuchta D (1996) A concept of the optimal solution of the transportation problem with fuzzy cost coefficients. Fuzzy Sets Syst 82(3):299–305

    Article  Google Scholar 

  • Chang CT (2007) Multi-choice goal programming. Omega 35(4):389–396

    Article  Google Scholar 

  • Chang CT (2008) Revised multi-choice goal programming. Appl Math Model 32(12):2587–2595

    Article  Google Scholar 

  • Chang CT (2011) Multi-choice goal programming with utility functions. Eur J Oper Res 215(2):439–445

    Article  Google Scholar 

  • El-Wahed WFA, Lee SM (2006) Interactive fuzzy goal programming for multi-objective transportation problems. Omega 34(2):158–166

    Article  Google Scholar 

  • Garfinkel RS, Rao MR (1971) The bottleneck transportation problem. Nav Res Logist Q 18(4):465–472

    Article  Google Scholar 

  • Gupta S, Ali I, Ahmed A (2018) Multi-objective capacitated transportation problem with mixed constraint: a case study of certain and uncertain environment. Opsearch 55(2):447–477

    Article  Google Scholar 

  • Hammer PL (1969) Time-minimizing transportation problems. Nav Res Logist Q 16(3):345–357

    Article  Google Scholar 

  • Hammer PL (1971) Communication on "the bottleneck transportation problem" and "some remarks on the time transportation problem.". Nav Res Logist Q 18(4):487–490

    Article  Google Scholar 

  • Kaufmann A, Gupta MM (1988) Fuzzy mathematical models in engineering and management science. Elsevier Science Inc, Amsterdam

    Google Scholar 

  • Mahapatra DR, Roy SK, Biswal MP (2013) Multi-choice stochastic transportation problem involving extreme value distribution. Appl Math Model 37(4):2230–2240

    Article  Google Scholar 

  • Maity G, Roy SK (2014) Solving multi-choice multi-objective transportation problem: a utility function approach. J Uncertain Anal Appl 2(1):1

    Article  Google Scholar 

  • Nasseri S, Bavandi S (2020) Multi-choice linear programming in fuzzy random hybrid uncertainty environment and their application in multi-commodity transportation problem. Fuzzy Inf Eng 1–14

  • Opricovic S (1998) Multi-criteria optimization of civil engineering systems, Faculty of Civil Engineering, Belgrade

  • Pramanik S, Roy T (2006) A fuzzy goal programming technique for solving multi-objective transportation problem. Tamsui Oxf J Manag Sci 22(1):67–89

    Google Scholar 

  • Pramanik S, Roy TK (2008) Multiobjective transportation model with fuzzy parameters: priority based fuzzy goal programming approach. J Transp Syst Eng Inf Technol 8(3):40–48

    Google Scholar 

  • Quddoos A, ull Hasan MG, Khalid MM (2014) Multi-choice stochastic transportation problem involving general form of distributions. SpringerPlus 3(1):1

    Article  Google Scholar 

  • Ranarahu N, Dash J, Acharya S (2019) Computation of multi-choice multi-objective fuzzy probabilistic transportation problem. Operations research in development sector. Springer, Berlin, pp 81–95

    Chapter  Google Scholar 

  • Roy SK (2015) Lagrange’s interpolating polynomial approach to solve multi-choice transportation problem. Int J Appl Comput Math 1(4):639–649

    Article  Google Scholar 

  • Roy SK, Maity G (2017) Minimizing cost and time through single objective function in multi-choice interval valued transportation problem. J Intell Fuzzy Syst 32(3):1697–1709

    Article  Google Scholar 

  • Roy SK, Maity G, Weber GW, Gök SZA (2016) Conic scalarization approach to solve multi-choice multi-objective transportation problem with interval goal. Ann Oper Res 1–22

  • Sharma JK, Swarup K (1977) Time minimizing transportation problems. In: Proceedings of the Indian Academy of Sciences-Section A, vol 86. Springer, Berlin, pp 513-518

  • Singh S, Singh S (2018) Bi-criteria transportation problem with multiple parameters. Ann Oper Res 269:667–692

    Article  Google Scholar 

  • Singh S, Sonia (2017) Multi-choice programmin: an overview of theories and applications. Optimization 66(10):1713–1738

  • Tamiz M, Jones D, Romero C (1998) Goal programming for decision making: an overview of the current state-of-the-art. Eur J Oper Res 111(3):569–581

    Article  Google Scholar 

  • Ustun O (2012) Multi-choice goal programming formulation based on the conic scalarizing function. Appl Math Model 36(3):974–988

    Article  Google Scholar 

  • Van Leekwijck W, Kerre EE (1999) Defuzzification: criteria and classification. Fuzzy Sets Syst 108(2):159–178

    Article  Google Scholar 

  • Varian HR (1989) Price discrimination. Handb Ind Organ 1:597–654

    Google Scholar 

  • Yu PL (1973) A class of solutions for group decision problems. Manag Sci 19(8):936–946

    Article  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf Control 8(3):338–353

    Article  Google Scholar 

  • Zeleny M (1982) Multi criteria decision making. McGraw-Hills, New York

    Google Scholar 

  • Zhao R, Govind R (1991) Algebraic characteristics of extended fuzzy numbers. Inf Sci 54(1–2):103–130

    Article  Google Scholar 

Download references

Acknowledgements

We are thankful to Mr. Ankit Khandelwal, Director, Analytics and Optimization Solutions, FICO, Singapore for his painstaking efforts to provide valuable comments and suggestions.

Author information

Authors and Affiliations

  1. Operations and Quantitative Methods, Indian Institute of Management Raipur, Naya Raipur, Chhattisgarh, India

    Shalabh Singh

  2. Indian Institute of Management, Prabandh Nagar, Lucknow, Uttar Pradesh, 226013, India

    Sonia Singh

Authors
  1. Shalabh Singh
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Sonia Singh
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Shalabh Singh.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Information

Below is the link to the electronic supplementary material.

Supplementary material 1 (pdf 292 KB)

Supplementary material 2 (rar 1537 KB)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Singh, S., Singh, S. Shipment in a multi-choice environment: a case study of shipping carriers in US. Cent Eur J Oper Res 30, 1195–1219 (2022). https://doi.org/10.1007/s10100-021-00757-2

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10100-021-00757-2

Keywords