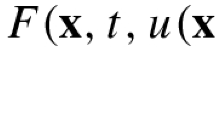Abstract
In this paper we propose a numerical reconstruction method for solving a backward heat conduction problem. Based on the idea of reproducing kernel approximation, we reconstruct the unknown initial heat distribution from a finite set of scattered measurement of transient temperature at a fixed final time. Standard Tikhonov regularization technique using the norm of reproducing kernel is adopt to provide a stable solution when the measurement data contain noises. Numerical results indicate that the proposed method is stable, efficient, and accurate.
Similar content being viewed by others
References
Aronszajn, N.: Theory of reproducing kernels. Trans. Am. Math. Soc. 68, 337–404 (1950)
Bukhgeĭm, A.L.: Introduction to the Theory of Inverse Problems. V.S.P. Intl Science (1999)
Carasso, A.: Error bounds in the final value problem for the heat equation. SIAM J. Math. Anal. 7, 195–199 (1976)
Chang, J.-R., Liu, C.-S., Chang, C.-W.: A new shooting method for quasi-boundary regularization of backward heat conduction problems. Int. J. Heat Mass Transfer 50, 2325–2332 (2007)
Chiwiacowsky, L., de Campos Velho, H.: Different approaches for the solution of a backward heat conduction problem. Inverse Probl. Eng. 11, 471–494 (2003)
Davies, E.B.: Heat kernels and spectral theory. In: Cambridge Tracts in Mathematics, vol. 92. Cambridge University Press, Cambridge (1990)
Fu, C.-L., Xiong, X.-T., Qian, Z.: Fourier regularization for a backward heat equation. J. Math. Anal. Appl. 331, 472–480 (2007)
Gao, X., Xiong, X.T., Nie, Y., Fu, C.L.: Fourier regularization method for solving a backward heat conduction problem. J. Lanzhou Univ. Nat. Sci. 42, 119–120 (2006)
Groetsch, C.W.: The Theory of Tikhonov Regularization for Fredholm Equations of the First Kind. Pitman, Boston, MA (1984)
Han, H., Ingham, D., Yuan, Y.: The boundary element method for the solution of the backward heat conduction equation. J. Comput. Phys. 116, 292–299 (1995)
Hansen, P.C., O’Leary, D.P.: The use of the L-curve in the regularization of discrete ill-posed problems. SIAM J. Sci. Comput. 14, 1487–1503 (1993)
Iijima, K., Onishi, K.: Lattice-free finite difference method for numerical solution of inverse heat conduction problem. Inverse Probl. Sci. Eng. 15, 93–106 (2007)
Itô, S.: Diffusion equations. In: Translations of Mathematical Monographs, vol. 114. American Mathematical Society, Providence, RI (1992)
Kirkup, S., Wadsworth, M.: Solution of inverse diffusion problems by operator-splitting methods. Appl. Math. Model. 26, 1003–1018 (2002)
Lattès, R., Lions, J.-L.: The Method of Quasi-reversibility. Applications to Partial Differential Equations. American Elsevier Publishing Co., Inc., New York (1969)
Lin, T., Ewing, R.: A direct numerical method for an inverse problem for the heat equation via hyperbolic perturbation, decision and control, 1988. In: Proceedings of the 27th IEEE Conference on, vol. 1, pp. 240–244 (1988)
Liu, C.-S.: Group preserving scheme for backward heat conduction problems. Int. J. Heat Mass Transfer 47, 2567–2576 (2004)
Liu, J.: Numerical solution of forward and backward problem for 2-D heat conduction equation. J. Comput. Appl. Math. 145, 459–482 (2002)
Matsuura, T., Saitoh, S., Trong, D.D.: Approximate and analytical inversion formulas in heat conduction on multidimensional spaces. J. Inverse Ill-Posed Probl. 13, 479–493 (2005)
Mera, N., Elliott, L., Ingham, D.D.B.: An inversion method with decreasing regularization for the backward heat conduction problem. Numer. Heat Transf. B 42, 215–230 (2002)
Mera, N., Elliott, L., Ingham, D., Lesnic, D.: An iterative boundary element method for solving the one dimensional backward heat conduction problem. Int. J. Heat Mass Transfer 44, 1937–1946 (2001)
Mera, N.S.: The method of fundamental solutions for the backward heat conduction problem. Inverse Probl. Sci. Eng. 13, 65–78 (2005)
Muniz, W., Ramos, F., de Campos Velho, H.: Entropy- and Tikhonov-based regularization techniques applied to the backwards heat equation. Comput. Math. Appl. 40, 1071–1084 (2000)
Muniz, W.B., de Campos Velho, H.F., Ramos, F.M.: A comparison of some inverse methods for estimating the initial condition of the heat equation. J. Comput. Appl. Math. 103, 145–163 (1999)
Payne, L.E.: Improperly Posed Problems in Partial Differential Equations. Society for Industrial and Applied Mathematics, Philadelphia, PA (1975)
Qian, Z., Fu, C.-L., Shi, R.: A modified method for a backward heat conduction problem. Appl. Math. Comput. 185, 564–573 (2007)
Saitoh, S.: Theory of reproducing kernels and its applications. In: Pitman Research Notes in Mathematics Series, vol. 189. Longman Scientific & Technical, UK (1988)
Schaback, R.: Solving the Laplace equation by meshless collocation using harmonic kernels. Adv. Comput. Math. 28, 283–299 (2008)
Shen, S.-Y.: A numerical study of inverse heat conduction problems. Comput. Math. Appl. 38, 173–188 (1999)
Shidfar, A., Zakeri, A.: A numerical technique for backward inverse heat conduction problems in one-dimensional space. Appl. Math. Comput. 171, 1016–1024 (2005)
Takeuchi, T., Yamamoto, M.: Tikhonov regularization by a reproducing kernel Hilbert space for the Cauchy problem for an elliptic equation. SIAM J. Sci. Comput. 31, 112–142 (2008)
Tikhonov, A.N., Arsenin, V.Y.: Solutions of Ill-posed Problems. Wiley, New York (1977)
Tikhonov, A.N., Goncharsky, A.V., Stepanov, V.V., Yagola, A.G.: Numerical Methods for the Solution of Ill-posed Problems. Kluwer Academic Publishers Group, Dordrecht (1995)
Wendland, H.: Scattered Data Approximation. Cambridge Monographs on Applied and Computational Mathematics. Cambridge University Press, Cambridge (2005)
Xiong, X.-T., Fu, C.-L., Qian, Z.: Two numerical methods for solving a backward heat conduction problem. Appl. Math. Comput. 179, 370–377 (2006)
Yamamoto, M., Zou, J.: Simultaneous reconstruction of the initial temperature and heat radiative coefficient. Inverse Probl. 17, 1181–1202 (2001)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Charles A. Micchelli.
The work described in this paper was fully supported by a grant from the Research Grants Council of the Hong Kong Special Administrative Region, China (Project No. CityU 101209).
Rights and permissions
About this article
Cite this article
Hon, Y.C., Takeuchi, T. Discretized Tikhonov regularization by reproducing kernel Hilbert space for backward heat conduction problem. Adv Comput Math 34, 167–183 (2011). https://doi.org/10.1007/s10444-010-9148-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10444-010-9148-1