Abstract
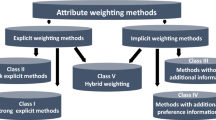
Attribute weighting is a task of paramount relevance in multi-attribute decision-making (MADM). Over the years, different approaches have been developed to face this problem. Despite the effort of the community, there is a lack of consensus on which method is the most suitable one for a given problem instance. This paper is the second part of a two-part survey on attribute weighting methods in MADM scenarios. The first part introduced a categorization in five classes while focusing on explicit weighting methods. The current paper addresses implicit and hybrid approaches. A total of 20 methods are analyzed in order to identify their strengths and limitations. Toward the end, we discuss possible alternatives to address the detected drawbacks, thus paving the road for further research directions. The implicit weighting with additional information category resulted in the most coherent approach to give effective solutions. Consequently, we encourage the development of future methods with additional preference information.

Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Allahi S, Mobin M, Vafadarnikjoo A, Salmon C (2015) An integrated AHP-GIS-MCLP method to locate bank branches. In: Proceedings of the 2015 Industrial and Systems Engineering Research Conference
Bao T, Xie X, Long P, Wei Z (2017) MADM method based on prospect theory and evidential reasoning approach with unknown attribute weights under intuitionistic fuzzy environment. Expert Syst Appl 88:305–317
Biswas P, Pramanik S, Giri BC (2016) Topsis method for multi-attribute group decision-making under single-valued neutrosophic environment. Neural Comput Appl 27(3):727–737
Biswas P, Pramanik S, Giri BC (2019) NH-MADM Strategy in Neutrosophic Hesitant Fuzzy Set Environment Based on Extended GRA. Informatica 30(2):213–242
Bottomley PA, Doyle JR (2001) A comparison of three weight elicitation methods: good, better, and best. Omega 29(6):553–560
Chen L, Guo G (2015) Nearest neighbor classification of categorical data by attributes weighting. Expert Syst Appl 42(6):3142–3149
Chen P (2019) On the diversity-based weighting method for risk assessment and decision-making about natural hazards. Entropy 21:269
Costa CABE, Vansnick JC (1999) In: (ed) The MACBETH approach: basic ideas, software, and an application. Springer, Dordrecht, pp 131–157
Chin Ks FuC, Wang Y (2015) A method of determining attribute weights in evidential reasoning approach based on incompatibility among attributes. Computer Ind Eng 87:150–162
Chin KS, Wang YM, Poon GK, Yang JB (2009) Failure mode and effects analysis using a group-based evidential reasoning approach. Computer Op Res 36(6):1768–1779
Dalapati S, Pramanik S (2018) A Revisit to NC-VIKOR Based MAGDM Strategy in Neutrosophic Cubic Set Environment. Neutrosophic S Syst 21(1):131–141
Deng H, Yeh CH, Willis RJ (2000) Inter-company comparison using modified TOPSIS with objective weights. Computer Op Res 27:963–973
Deng M, Yang Jb XuW (2004) Estimating the attribute weights through evidential reasoning and mathematical programming. Int J Inf Technol and Decis Making 3(3):419–428
Diakoulaki D, Mavrotas G, Papayannakis L (1995) Determining objective weights in multiple criteria problems: the CRITIC method. Computer Op Res 22:763–770
Doyle JR, Green RH, Bottomley PA (1997) Judging relative importance: direct rating and point allocation are not equivalent. Organ Behav Human Decis Process 70(1):65–72
Edwards W (1977) How to use multiattribute utility measurement for social decision making. IEEE Trans Syst, Man Cybern 7(5):326–340
Edwards W, Barron FH (1994) SMARTS and SMARTER: improved simple methods for multiattribute utility measurament. Organ Behav Human Decis Process 60:306–325
Fan ZP, Ma J, Zhang Q (2002) An approach to multiple attribute decision making based on fuzzy preference information on alternatives. Fuzzy Sets Syst 131:101–106
Farhadinia B, Xu Z (2018) Ordered weighted hesitant fuzzy information fusion-based approach to multiple attribute decision making with probabilistic linguistic term sets. Fundam Inf 159:361–383
Festinger L (1957) A theory of Cognitive Dissonance. Harper and Row Publishers, NY
Figueira J, Roy B (2002) Determining the weights of criteria in the ELECTRE type methods with a revised simos’ procedure. Eur J Op Res 139:317–326
Fu C, Huhns M, Yang SL (2014) A consensus framework for multiple attribute group decision analysis in an evidential reasoning context. Inf Fusion 17:22–35
Fu C, Wang Y (2015) An interval difference based evidential reasoning approach with unknown attribute weights and utilities of assessment grades. Computer Ind Eng 81:109–117
Fu C, Yang SL (2010) The group consensus based evidential reasoning approach for multiple attributive group decision analysis. Eur J Op Res 206(3):601–608
Fu C, Yang SL (2011) An attribute weight based feedback model for multiple attributive group decision analysis problems with group consensus requirements in evidential reasoning context. Eur J Op Res 202(1):179–189
Fu C, Yang SL (2012) An evidential reasoning based consensus model for multiple attribute group decision analysis problems with interval-valued group consensus requirements. Eur J Op Res 223:167–176
Gan X, Fernandez IC, Guo J, Wilson M, Zhao Y, Zhou B, Wu J (2017) When to use what: methods for weighting and aggregating sustainability indicators. Ecol Indic 81:491–502
Hatefi MA (2019) Indifference threshold-based attribute ratio analysis: a method for assigning the weights to the attributes in multiple attribute decision making. Appl Soft Comput 74:643–651
Horowitz I, Zappe C (1995) The linear programming alternative to policy capturing for eliciting criteria weights in the performance appraisal process. Omega 23(6):667–676
Horsky D, Rao MR (1984) Estimation of attribute weights from preference comparisons. Manag Sci 30(7):801–822
Jahan A, Mustapha F, Sapuan M (2012) Yusof Ismail Md Bahraminasab M: A framework for weighting of criteria in ranking stage of material selection process. Int J Adv Manuf Technol 58:411–420
Jin F, Pei L, Chen H, Zhou L (2014) Interval-valued intuitionistic fuzzy continuous weighted entropy and its application to multi-criteria fuzzy group decision making. Knowl-Based Syst 59:132–141
Keeney R, Raiffa H (1976) Decisions with multiple objectives: preferences and value-tradeoffs. Cambridge University Press, Cambridge
Khan MSA, Ali A, Abdullah S, Amin F, Hussain F (2018) New extension of TOPSIS method based on Pythagorean hesitant fuzzy sets with incomplete weight information. J Intell Fuzzy Syst 35:5435–5448
Lee CH (2018) An information-theoretic filter approach for value weighted classification learning in Naive Bayes. Data Knowl Eng 113:116–128
Lee J, Han B, Lim H, Kim Y, Saxena N, Chung T (2009) Optimizing access point allocation using genetic algorithmic approach for smart home environments. Computer J 52(8):938–949
Li D (1999) Fuzzy multiattribute decision-making models and methods with incomplete preference information. Fuzzy Set Syst 106:113–119
Liang X, Sun X, Shu G, Sun K, Wang X, Wang X (2013) Using the analytic network process (ANP) to determine method of waste energy recovery from engine. Energy Convers Manag 66:304–311
Liu P, Jin F (2012) A multi-attribute group decision-making method based on weighted geometric aggregation operators of interval-valued trapezoidal fuzzy numbers. Appl Math Modell 36(6):2498–2509
Liu P, Yu X (2014) 2-dimension uncertain linguistic power generalized weighted aggregation operator and its application in multiple attribute group decision making. Knowl-Based Syst 57:69–80
Liu S, Chan FT, Ran W (2016) Decision making for the selection of cloud vendor: An improved approach under group decision-making with integrated weights and objective/subjective attributes. Expert Syst Appl 55:37–47
Liu W (2006) Analyzing the degree of conflict among belief functions. Artif Int 170:909–924
Ma J, Fan Z, Huang L (1999) A subjective and objective integrated approach to determine attribute weights. Eur J Op Res 112:397–404
Ma J, Zhang Q, Zhou D, Fan ZP (1999) A subjective and objective integrated approach to multiple attribute decision making with preference information on alternatives. Citeseer, NJ
Mathematica Wolfram Research, I.: Version 12.0 (2019)
Matta C (2019) Qualitative research methods and evidential reasoning. Philos Soc Sci 49(5):385–412
Németh B, Molnár A, Bozóki S, Wijaya K, Inotai A, Campbell JD, Kaló Z (2019) Comparison of weighting methods used in multicriteria decision analysis frameworks in healthcare with focus on low-and middle-income countries. J Comp Eff Res 8(4):195–204
Paelinck JH (1976) Qualitative multiple criteria analysis, environmental protection and multiregional development. In: Papers of the Regional Science Association, vol. 36, pp. 59–74. Springer
Pang N, Zhang J, Zhang C, Qin X, Cai J (2019) PUMA: Parallel subspace clustering of categorical data using multi-attribute weights. Expert Syst Appl 126:233–245
Park DG, Kwun YC, Park JH, Park IY (2009) Correlation coefficient of interval-valued intuitionistic fuzzy sets and its application to multiple attribute group decision making problems. Math Computer Modell 50(9–10):1279–1293
Park JH, Park IY, Kgun YC, Tan X (2011) Extension of the TOPSIS method for decision making problems under interval-valued intuitionistic fuzzy environment. Appl Math Modell 35(5):2544–2556
Pecchia L, Crispino F, Morgan SP (2014) A software tool to support the health technology assessment HTA and the user need elicitation of medical devices via the Analytic Hierarchy Process AHP. In: Zhang YT (ed) The International Conference on Health Informatics. Springer, Cham, pp 292–295
Pei Z (2013) Rational decision making models with incomplete weight information for production line assessment. Inf Sci 222:696–716
Pena J, Nápoles G, Salgueiro Y (2020) Explicit methods for attribute weighting in multi-attribute decision-making: a review study. Artif Intell Rev 53:3127–3152
Pramanik S, Biswas P, Giri BC (2017) Hybrid vector similarity measures and their applications to multi-attribute decision making under neutrosophic environment. Neural Comput Appl 28(5):1163–1176
Pramanik S, Dalapati S, Alam S, Smarandache F, Roy TK (2018) NS-Cross Entropy-Based MAGDM under Single-Valued Neutrosophic Set Environment. Information 9(2):37
Pramanik S, Mallick R (2019) TODIM strategy for multi-attribute group decision making in trapezoidal neutrosophic number environment. Complex Intell Syst 5(4):379–389
Qi X, Liang C, Zhang J (2015) Generalized cross-entropy based group decision making with unknown expert and attribute weights under interval-valued intuitionistic fuzzy environment. Computer Ind Eng 79:52–64
Ram M (2018) Advanced fuzzy logic approaches in engineering science. IGI Global, Pennsylvania
Ran F, Huchang L, Jian-Bo Y, Dong-Ling X (2019) Generalised probabilistic linguistic evidential reasoning approach for multi-criteria decision-making under uncertainty. Journal of the Operational Research Society 1–15
Rao RV, Patel BK, Parnichkun M (2011) Industrial robot selection using a novel decision making method considering objective and subjective preferences. Robot Auton Syst 59(6):367–375
Roy B (1968) Classement et choix en présence de points de vue multiples. RAIRO - Op Res - Recherche Opérationnelle 2(1):57–75
Roy B, Bertier P (1971) Le méthode ELECTRE II Note de Travail. In: SEMA-METRA Metra International
S, G (2017) Application of entropy weight method in the evaluation of the road capacity of open area. In: AIP Conference Proceedings The American Institute of Physics
Saaty TL (1977) A scaling method for priorities in hierarchical structures. J Math Psychol 15:234–281
Saaty TL (1994) Fundamentals of decision making and priority theory with the Analytic Hierarchy Process. RWS Publications, PA
Shirland LE, Jesse RR, Thompson RL, Iacovou CL (2003) Determining attribute weights using mathematical programming. Omega 31(6):423–437
Solymosi T, Dombi J (1986) A method for determining the weights of criteria: the centralized weights. Eur J Op Res 26:35–41
Song Y, Fu Q, Yf Wang, Wang X (2019) Divergence-based cross entropy and uncertainty measures of Atanassov’s intuitionistic fuzzy sets with their application in decision making. Appl Soft Comput 84:105703
Ty Chen (2014) Interval-valued intuitionistic fuzzy QUALIFLEX method with a likelihood-based comparison approach for multiple criteria decision analysis. Inf Sci 261:149–169
Ty Chen, Li Ch (2010) Determining objective weights with intuitionistic fuzzy entropy measures: a comparative analysis. Inf Sci 180(21):4207–4222
Valkenhoef G, Tervonen T (2016) Entropy-optimal weight constraint elicitation with additive multi-attribute utility models. Omega 64:1–12
Wan Sp, Dong Jy (2015) Interval-valued intuitionistic fuzzy mathematical programming method for hybrid multi-criteria group decision making with interval-valued intuitionistic fuzzy truth degrees. Inf Fusion 26:49–65
Wan Sp, Li Df (2014) Atanassov’s intuitionistic fuzzy programming method for heterogeneous multiattribute group decision making with Atanassov’s intuitionistic. IEEE Trans Fuzzy Syst 22(2):300–312
Wan SP, Li DF (2015) A new method for Atanassov’s interval-valued intuitionistic fuzzy MAGDM with incomplete attribute weight information. Inf Sci 316:329–347
Wan Sp Xu, Gl Wang F, Jy Dong (2015) A new method for Atanassov’s interval-valued intuitionistic fuzzy MAGDM with incomplete attribute weight information. Inf Sci 316(168):329–347
Wang W, Liu X (2013) The multi-attribute decision making method based oninterval-valued intuitionistic fuzzy Einstein hybrid weighted geometric operator. Computer Math Appl 66:1845–1856
Wang Y, Fu G (1993) Using multiobjective decision making method to make decision for multiattributes. Control Decis 8(1):25–29
Wang Ym (2005) On fuzzy multiattribute decision-making models and methods with incomplete preference information. Fuzzy Set Syst 151:285–301
Wang Ym , Luo Y (2010) Integration of correlations with standard deviations for determining attribute weights in multiple attribute decision making. Math Computer Modell 51:1–12
Wang Ym, Parkan C (2006) A general multiple attribute decision-making approach for integrating subjective preferences and objective information. Fuzzy Set Syst 157:1333–1345
Wang YM, Yang JB, Xu DL (2006) Environmental impact assessment using the evidential reasoning approach. Eur J Op Res 174(3):1885–1913
Wang YM, Yang JB, Xu DL (2016) Multicriteria Pythagorean fuzzy decision analysis: a hierarchical QUALIFLEX approach with the closeness index-based ranking methods. Inf Sci 330:104–124
Yang JB (2001) Rule and utility based evidential reasoning approach for multiattribute decision analysis under uncertainties. Eur J Op Res 131:31–61
Zhang H, Jiang L, Yu L (2020) Class-specific attribute value weighting for Naive Bayes. Inf Sci 508:260–274
Zhang L, Zhan J, Yao Y (2020) Intuitionistic fuzzy TOPSIS method based on CVPIFRS models: an application to biomedical problems. Inf Sci 517:315–339
Zhang Q, Chen JCH, Chong PP (2004) Decision consolidation: criteria weight determination using multiple preference formats. Decis Support Syst 38:247–258
Zhang Y (2015) TOPSIS method based on entropy weight for supplier evaluation of power grid enterprise. In: 2nd International Conference on Education Reform and Modern Management, pp. 334–337
Zhou L, Tao Z, Chen H, Liu J (2014) Continuous interval-valued intuitionistic fuzzy aggregation operators and their applications to group decision making. Appl Math Modell 38:2190–2205
Acknowledgements
The authors would like to thank the anonymous reviewers for their constructive feedback. This paper was partially supported by the Special Research Fund (BOF) of Hasselt University through the project BOF20KV01.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Pena, J., Nápoles, G. & Salgueiro, Y. Implicit and hybrid methods for attribute weighting in multi-attribute decision-making: a review study. Artif Intell Rev 54, 3817–3847 (2021). https://doi.org/10.1007/s10462-020-09941-3
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10462-020-09941-3