Abstract
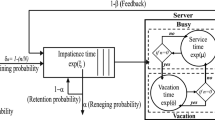
This paper deals with a generalized M/G/1 feedback queue in which customers are either “positive" or “negative". We assume that the service time distribution of a positive customer who initiates a busy period is G e (x) and all subsequent positive customers in the same busy period have service time drawn independently from the distribution G b (x). The server is idle until a random number N of positive customers accumulate in the queue. Following the arrival of the N-th positive customer, the server serves exhaustively the positive customers in the queue and then a new idle period commences. This queueing system is a generalization of the conventional N-policy queue with N a constant number. Explicit expressions for the probability generating function and mean of the system size of positive customers are obtained under steady-state condition. Various vacation models are discussed as special cases. The effects of various parameters on the mean system size and the probability that the system is empty are also analysed numerically.
Similar content being viewed by others
References
Artalejo, J.R. (2000). G-networks: “A versatile approach for work removal in queueing networks.” European Juornal of Operational Research 126, 233–249.
Bocharov, P.P. and V.M. Vishnevskii. (2003). G-networks: “Development of the theory of multiplicative networks.” Automation and Remote Control 64, 714–739.
Chang, W. (1968). “Queues with feedback for time-sharing computer system analysis.” Operations Research 16, 613–627.
Chao, X., M. Miyazawa and M. Pinedo. (1999). Queueing Networks: Customers, Signals and Product Form Solutions (John Wiley & Sons, Chichester).
Disney, R.L. D.C. McNickle and B.Simon. (1980). “The M/G/1 queue with instantaneous Bernoulli feedback.” Naval Research Logistics 27, 635–644.
Disney, R.L., D. Konig and V. Schmidt. (1984). “Stationary queue-length and waiting-time distributions in single-server feedback queues.” Advanced Applied Probability 16, 437–446.
Disney, R.L. and D. Konig. (1985). “Queueing Networks: A survey of their random process.” SIAM Rev. 27 335–403.
Doshi, B.T. (1990). “Single-server queues with vacations.” In: Stochastic Analysis of Computer and Communications Systems ed. H.Takagi (Elsevier, Amsterdam).
Fontana, B. and C.D. Berzosa. (1985). “M/G/1 queue with N-priorities and feedback: Joint queue-length distributions and response time distribution for any particular sequence.” In: Teletraffic Issues in Advanced Information Society , ITC-11 ed. W. Akiyama, (Elsevier, North-Holland, Amsterdam) 452–458.
Fuhrmann, S.W. and R.B. Cooper. (1985). “Stochastic decomposition in the M/G/1 queue with generalized vacations, Operations Research 33, 1117–1129.
Gelenbe, E. and G. Pujolle. (1998). Introduction to Queueing Networks, (John Wiley & Sons, Chichester).
Harrison, P.G. and N.M. Pitle. (1993). Performance Modelling of Communication Networks and Computer Architectures, (Addidon Wesely, Newyork).
Harrison, P.G. and E. Pitle. (1996). “The M/G/1 queue with negative customers.” Advanced Applied Probability 28, 540–566.
Hur, S. and S.J. Paik. (1999). “The effect of different arrival rates on the N-policy of M/G/1 with server setup.” Applied Mathematical Modelling 23, 289–299.
Kella, O. (1989). “The threshold policy in the M/G/1 queue with server vacations.” Naval Research Logistics 36, 111–123.
Lam S.S. (1977). “Delay analysis of a time division multiple access (TDMA) channel.” IEEE Transaction on Communications, COM-25 1489–1494.
Lee, H.S. and M.M. Srinivasan. (1989). “Control policies for the MX/G/1 queueing system.” Management Science, 35, 708–721.
Lee, H.W., S.S. Lee and K.C. Chae. (1994a). “On a batch arrival queue with N-policy and single vacation.” Computers & Operations Research 22, 173–189.
Lee, H.W., S.S. Lee, J.O. Park and K.C. Chae. (1994b). “Analysis of MX/G/1 queue with N-policy and multiple vacations.” Journal of Applied Probability 31, 467–496.
Lee, H.W. and J.O. Park. (1997). “Optimal strategy in N-policy production system with early set-up time.” Journal of Operations Research Society 48, 306–313.
Levy, Y. and U. Yechiali. (1975). “Utilization of idle time in an M/G/1 queueing system.” Management Science 22, 202–211.
Levy, H. and L. Kleinrock. (1986). “A queue with starter and a queue with vacations: delay analysis by decomposition.” Operations Research 34, 426–436.
Medhi, J. and G.C. Templeton. (1992). “A Poisson input queue under N-policy and with a general startup time.” Computers & Operations Research 19, 35–41.
Scholl, M.O. and L. Kleinrock. (1983). “On the M/G/1 queue with rest periods and certain service-independent queueing diciplines.” Operations Research 31, 705–719.
Takacs, L. (1963). “A single server queue with feedback.” Bell System Technical Journal 42, 505–519.
Takagi, H. (1991). Queueing Analysis: A foundation of performance evaluation. Vol. I, Vacation and Priority Systems, (North-Holland, Amsterdam).
Teghem, J. (1986). “Control of the service process in a queueing system.” European Journal of Operational Research 23, 141–158.
Tijms, H.C. (1986). Stochastic Modelling and Analysis: A Computational Approach, (John Wiley & Sons, Chichester).
Welch, P.D. (1964). “On a generalized M/G/1 queueing process in which the first customer of each busy period receives exceptional service.” Operations Research 12, 736–752.
Yadin, M. and P. Naor. (1963). “Queueing systmes with a removable service station.” Operations Research Quarterly 14, 393–405.
Author information
Authors and Affiliations
Corresponding author
Additional information
AMS Subject Classification: Primary: 60 K 25 · Secondary: 60 K 20, 90 B 22
Rights and permissions
About this article
Cite this article
Kumar, B.K., Arivudainambi, D. & Krishnamoorthy, A. Some results on a generalized M/G/1 feedback queue with negative customers. Ann Oper Res 143, 277–296 (2006). https://doi.org/10.1007/s10479-006-7388-8
Issue Date:
DOI: https://doi.org/10.1007/s10479-006-7388-8