Abstract
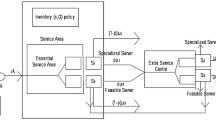
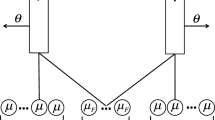
Motivated by an industry example, we study a two-station serial system in which we allocate flexible servers in order to maximize throughput. We investigate two cases which are different in the way that servers work together when at the same station; namely collaboratively or non-collaboratively. For the collaborative case we prove the optimal policy to be such that the servers work together at a single station at any point in time. In addition to the policy being state-dependent, it also follows a switching-curve structure. In the non-collaborative case, on the other hand, it may be optimal to allocate servers to different stations. Some numerical examples and results regarding policy assignments, switching curves, and system throughput are presented.
Similar content being viewed by others
References
Ahn, H. S., Duenyas, I., & Zhang, R. (1999). Optimal stochastic scheduling of a two-stage tandem queue with parallel servers. Advances in Applied Probability, 31, 1095–1117.
Ahn, H. S., Duenyas, I., & Lewis, M. E. (2002). Optimal control of a two-stage tandem queuing with flexible servers. Probability in the Engineering and Informational Sciences, 16, 453–469.
Andradottir, S., & Ayhan, H. (2005). Throughput maximization for tandem lines with two stations and flexible servers. Operations Research, 53, 516–531.
Andradottir, S., Ayhan, H., & Down, D. G. (2001). Server assignment policies for maximizing the steady-state throughput of finite queuing systems. Management Science, 47, 1421–1439.
Andradottir, S., Ayhan, H., & Down, D. G. (2007). Dynamic assignment of dedicated and flexible servers in tandem lines. Probability in the Engineering and Informational Sciences, 21(4).
Bell, S. L., & Williams, R. J. (2001). Dynamic scheduling of a system with two parallel servers in heavy traffic with complete resource pooling: Asymptotic optimality of a continuous review threshold policy. Annals of Applied Probability, 11, 608–649.
Bischak, D. P. (1996). Performance of a manufacturing module with moving workers. IIE Transactions, 28, 723–733.
Buzacott, J. A. (1996). Commonalities in reengineered business processes: Models and issues. Management Science, 42, 768–782.
Duenyas, I., Gupta, D., & Olsen, T. L. (1998). Control of a single server tandem queuing system with setups. Operations Research, 46, 218–230.
Farrar, T. M. (1993). Optimal use of an extra server in a two station tandem queuing network. IEEE Transactions on Automatic Control, 38, 1296–1299.
Hajek, B. (1984). Optimal control of interacting service stations. IEEE Transactions on Automatic Control, 29(6), 491–499.
Harrison, J. M., & Lopez, M. J. (1999). Heavy traffic resource pooling in parallel server systems. Queuing Systems, 33, 339–368.
Hillier, F. S., & So, K. C. (1996). On the simultaneous optimization of server and work allocations in production line systems with variable processing times. Operations Research, 44, 435–443.
Irvani, S. M. R., Posner, M. J. M., & Buzacott, J. A. (1997). A two-stage tandem queue attended by a moving server with holding and switching costs. Queuing Systems, 26, 203–228.
Lippman, S. A. (1975). Applying a new device in the optimization of exponential queuing systems. Operations Research, 23(4), 687–710.
Mandelbaum, A., & Stoylar, A. L. (2004). Scheduling flexible servers with convex delay costs. Operations Research, 52, 836–855.
McClain, J. O., Thomas, L. J., & Sox, C. (1992). “On the fly” line balancing with very little wip. International Journal of Production Economics, 27, 283–289.
Ostolaza, J., Thomas, L. J., & McClain, J. O. (1990). The use of dynamic (state-dependent) assembly-line balancing to improve throughput. Journal of Manufacturing Operations Management, 3, 105–133.
Pandelis, D. G., & Teneketzes, D. (1994). Optimal multiserver stochastic scheduling of two interconnected priority queues. Advances in Applied Probability, 26, 258–279.
Porteus, E. L. (1982). Conditions for characterizing the structure of optimal strategies in infinite horizon dynamic programs. Journal of Optimization Theory and Applications, 36, 419–432.
Reiman, M. I., & Wein, L. M. (1998). Dynamic scheduling of a two-class queue with setups. Operations Research, 46, 532–547.
Rosberg, Z., Varaiya, P. P., & Walrand, J. C. (1982). Optimal control of service in tandem queues. IEEE Transactions on Automatic Control, 27(3), 600–609.
Ross, S. M. (1970). Applied probability models with optimization applications. San Francisco: Holden-Day.
Ross, S. M. (2003). Introduction to probability models (8 ed.) New York: Academic Press.
Squillante, M. S., Xia, C. H., Yao, D. D., & Zhang, L. (2001). Threshold based priority policies for parallel-server systems with affinity scheduling. In Proceedings of 2001 American control conference (pp. 2992–2999).
Van Oyen, M. P., & Teneketzis, D. (1994). Optimal stochastic scheduling of forest networks with switching penalties. Advances in Applied Probability, 26, 474–497.
Van Oyen, M. P., Gel, E. G. S., & Hopp, W. J. (2001). Performance opportunity for workforce agility in collaborative and noncollaborative work systems. IIE Transactions, 33, 761–777.
Williams, R. J. (2000). On dynamic scheduling of a parallel server system with complete resource pooling. Proceedings of the Fields Institute Workshop on Analysis and Simulation of Communication Networks, 28, 49–71.
Yamazaki, G., Sakasegawa, H., & Shanthikumar, J. G. (1992). On optimal arrangement of stations in a tandem queuing system with blocking. Management Science, 38, 137–153.
Zavadlav, E., McClain, J. O., & Thomas, L. J. (1996). Self-buffering, self-balancing, self-flushing production lines. Management Science, 42, 1151–1164.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Arumugam, R., Mayorga, M.E. & Taaffe, K.M. Inventory based allocation policies for flexible servers in serial systems. Ann Oper Res 172, 1–23 (2009). https://doi.org/10.1007/s10479-008-0465-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-008-0465-4