Stability of vertically differentiated Cournot and Bertrand-type models when firms are boundedly rational

Abstract

This paper compares the dynamic of Cournot and Bertrand duopolies with vertical product differentiation and under bounded rationality. We find that an increase in the product differentiation degree destabilizes the Nash equilibrium under quantity competition, while the Bertrand–Nash equilibrium becomes more stable. From a global dynamic analysis, we show that an increase in the firms’ adjustment speed constitutes a source of complexity in both models. There is a cascade of flip bifurcations leading to increasingly complex attractors, and there are global bifurcations generating complex basins of attraction.

Appendix

From (8) and (9) the trace and the determinant of the matrix $JT_C (E_C^{*} )$ can be given by:

$$\begin{aligned} \left\{ {\begin{array}{l} Tr=2-2\alpha (sq_1^{*} +q_2^{*} )=2-4\frac{\alpha s^{2}\overline{{v}}}{4s-1} \\ Det=Tr-1+M,\hbox { with }M=\alpha ^{2}(4s-1)q_1^{*} q_2^{*} =\alpha ^{2}\frac{(2s-1)s\overline{{v}}^{2}}{4s-1}>0 \\ \end{array}} \right. \end{aligned}$$

(32)

Thus, the eigenvalues of the matrix $JT_C (E_C^{*} )$ in terms of Tr and M are:

$$\begin{aligned} \lambda _1 =\frac{Tr}{2}-\sqrt{\left( {\frac{Tr-2}{2}} \right) ^{2}-M}\le \lambda _2 =\frac{Tr}{2}+\sqrt{\left( {\frac{Tr-2}{2}} \right) ^{2}-M} \end{aligned}$$

Analyzing $\lambda _1$ and $\lambda _2$, we can characterize the equilibrium $E_C^{*}$ and subsequently the evolution of the solution trajectories of the dynamic system in a neighborhood of this equilibrium. The following proposition is established.

Proposition 4

Under Cournot competition:

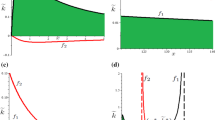
(i) If $\alpha \overline{{v}}<(\alpha \overline{{v}})_3^C =\frac{2s}{2s-1}-\frac{\sqrt{s(4s^{3}-8s^{2}+6s-1}}{s(2s-1)}$, then $0<\lambda _1 <\lambda _2 <1$ (region A in Fig. 1).

(ii) If $(\alpha \overline{{v}})_3^C <\alpha \overline{{v}}<(\alpha \overline{{v}})_1^C$, then $-1<\lambda _1 <0<\lambda _2 <1$ (region B in Fig. 1).

(iii) If $\alpha \overline{{v}}=(\alpha \overline{{v}})_1^C$, then $-1=\lambda _1 <\lambda _2 <1$, and there is a Flip bifurcation (curve $(\alpha \overline{{v}})_F^C =(\alpha \overline{{v}})_1^C$ in Fig. 1).

(iv) If $(\alpha \overline{{v}})_1^C <\alpha \overline{{v}}<(\alpha \overline{{v}})_2^C$, then $\lambda _1 <-1<\lambda _2 <1$ (region C in Fig. 1).

(v) If $(\alpha \overline{{v}})_2^C <\alpha \overline{{v}}$, then $\lambda _1 <\lambda _2 <-1$ (region D in Fig. 1).

(vi) There is neither a transcritical bifurcation nor a Neimark–Sacker bifurcation.

Proof

We deduce the following:

• (a) $\left( {\frac{Tr-2}{2}} \right) ^{2}-M\ge 0$ for all $s > 1$, $\overline{{v}}>0$ and $\alpha >0$ given that:

  $$\begin{aligned} (Tr-2)^{2}-4M\ge & {} 0\Leftrightarrow s^{2}q_1^{*2}+q_2^{*2}-(2s-1)q_1^{*} q_2^{*} \\= & {} \left( {s^{2}-\frac{(2s-1)^{2}}{4}} \right) q_1^{*2}+\left( {q_2^{*} -\frac{2s-1}{s}q_1^{*} } \right) ^{2}\ge 0 \end{aligned}$$

  Therefore, $\lambda _1 \hbox { and }\lambda _2$ cannot be complex values. Consequently, there is no Neimark–Sacker bifurcation.

• (b) As $M > 0$, $\forall s>1,\alpha >0,\overline{{v}}>0$, then $\lambda _2 <1$, and there is no transcritical bifurcation.

• (c) If $Tr<\frac{-M}{2}$, then $\lambda _1 <-1<\lambda _2 <1$, and the Nash equilibrium is a saddle point.

• Substituting (32) into the inequality $Tr<\frac{-M}{2}$ we deduce $(\alpha \overline{{v}})_1^C <\alpha \overline{{v}}<(\alpha \overline{{v}})_2^C$, defining region C in Fig. 1, being:

  $$\begin{aligned} (\alpha \overline{{v}})_1^C= & {} \frac{4s}{2s-1}-\frac{2\sqrt{s(4s^{3}-8s^{2}+6s-1)}}{s(2s-1)}\hbox { and }\\ (\alpha \overline{{v}})_2^C= & {} \frac{4s}{2s-1}+\frac{2\sqrt{s(4s^{3}-8s^{2}+6s-1)}}{s(2s-1)} \end{aligned}$$

• (d) If $\frac{-M}{2}<Tr<2-2\sqrt{M}$ and $M>4$, then $\lambda _1 <\lambda _2 <-1$, and for any initial condition near to equilibrium, the trajectory moves away in a fluctuating manner.

• Substituting (32) into the above inequalities, we deduce $(\alpha \overline{{v}})_2^C <\alpha \overline{{v}}$, leading to region D in Fig. 1.

• (e) If $Tr=\frac{-M}{2}$ and $M<4$, then $\lambda _1 =-1<\lambda _2 <1$. In this case, there is a flip bifurcation.

• Substituting (32) into the above expressions, we deduce $\alpha \overline{{v}}=(\alpha \overline{{v}})_1^C$, obtaining the curve $(\alpha \overline{{v}})_F^C =(\alpha \overline{{v}})_1^C$ in Fig. 1.

• (f) In the stability area ($\alpha \overline{{v}}<(\alpha \overline{{v}})_1^C$), according to the sign of the eigenvalues, we can distinguish the following three subregions:

  • If $1-M<Tr<2-2\sqrt{M}$ and $0<M<1$, then $0<\lambda _1 <\lambda _2 <1$ and the Nash equilibrium is a stable node. Substituting (32) into these expressions, it follows that $\alpha \overline{{v}}<(\alpha \overline{{v}})_3^C =\frac{2s}{2s-1}-\frac{\sqrt{s(4s^{3}-8s^{2}+6s-1)}}{s(2s-1)}$, giving rise to region A in Fig. 1.

  • If $-\frac{M}{2}<Tr<1-M$, then $-1<\lambda _1 <0<\lambda _2 <1$ and the Nash equilibrium is asymptotically stable; however, there is no stable node. As a negative eigenvalue exists (with modulus lower than one), there may appear to be a fluctuating convergence. Introducing (32) into the above expressions holds that $(\alpha \overline{{v}})_3^C <\alpha \overline{{v}}<(\alpha \overline{{v}})_1^C$, leading to region B in Fig. 1.

  • If $1-M<Tr<2-2\sqrt{M}$ and $1<M<2$ or $\frac{-M}{2}<Tr<2-2\sqrt{M}$ and $2<M<4$, then $-1<\lambda _1 <\lambda _2 <0$ and for any initial condition near to equilibrium, the trajectory converges to it in a fluctuating manner.

    Substituting (32) into these expressions, we conclude that the set of parameters $(s, \alpha , \overline{{v}})$, such that the above inequalities hold, is empty. $\square$