Dynamic procurement from multiple suppliers with random capacities

Abstract

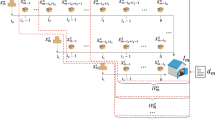
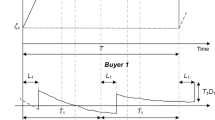
We study a periodic-review inventory model. In each period, the buyer can source from N suppliers that have random capacities. The optimal ordering policy for this problem has been shown to be a reorder point policy in a setting that includes price-dependent demand (Feng and Shi in Prod Oper Manag 21(3):547–563, 2012). We show that if one of the suppliers is reliable, then the optimal order quantity from each unreliable and cheaper supplier stays constant when inventory level is less than the reorder point of the reliable supplier. This implies that having one reliable supplier would result in a relatively stable ordering policy, despite the unreliability of the rest of the suppliers. For the special case of sourcing from two suppliers, we first prove that the optimal policy is monotone in the inventory level. Then, we provide insights into order allocation between an expensive but more reliable supplier and a cheaper but less reliable one. Specifically, the optimal policy tends to allocate more units to the more reliable one when inventory level is low. For the special case of all-or-nothing supply, there is a threshold such that if the inventory is below the threshold, then the expensive supplier’s order quantity is larger than that of the cheaper. Finally, we show that the marginal cost of holding inventory increases, while the optimal order quantities decrease over the planning horizon.

Appendix: Proofs

Lemma 1

For every convex function f, a random variable K with distribution $F_{K}$ and ${\tilde{x}}=\arg \min _{x\ge 0} f(x)$, it should be true that

$$\begin{aligned} \mathbf{E}_{K}[f(\lambda (x_1\wedge K) + {\bar{\lambda }}(x_2\wedge K))]\ge \mathbf{E}_{K}[f({\tilde{x}}\wedge K)], \, \, \forall x_1\ge 0, x_2\ge 0 \end{aligned}$$

(2)

Proof of Lemma 1

Considering every realization of $\check{k}$, we have two cases.

If $\check{k}<{\tilde{x}}$, then we have

$$\begin{aligned} \lambda (x_1\wedge \check{k}) + {\bar{\lambda }}(x_2\wedge \check{k}) \, \le \, \lambda \check{k} + (1-\lambda )\check{k}\, =\, \check{k} = {\tilde{x}}\wedge \check{k} \end{aligned}$$

The inequality comes from $\check{k}<{\tilde{x}}$.

Notice that f is a convex function and $\lambda (x_1\wedge \check{k}) + {\bar{\lambda }}(x_2\wedge \check{k})\le \check{k}<{\tilde{x}}$ where ${\tilde{x}}=\arg \min _{x\ge 0} f(x)$ . Hence, $\check{k}$ is closer to the minimum point than $\lambda (x_1\wedge \check{k}) + {\bar{\lambda }}(x_2\wedge \check{k})$ and that both are on the left side of a convex function. Thus,

$$\begin{aligned} f(\lambda (x_1\wedge \check{k}) + {\bar{\lambda }}(x_2\wedge \check{k})) \, \ge \, f(\check{k}) \, = \, f({\tilde{x}}\wedge \check{k}). \end{aligned}$$

If $\check{k}\ge {\tilde{x}}$, then we notice that ${\tilde{x}}=\arg \min _{x\ge 0} f(x)$. Hence,

$$\begin{aligned} f(\lambda (x_1\wedge \check{k}) + (1-\lambda )(x_2\wedge \check{k})) \, \ge \, \min _{x\ge 0} f(x)\, = \, f({\tilde{x}}\wedge \check{k}) \end{aligned}$$

From the discussion above two scenarios, we have

$$\begin{aligned} \mathbf{E}_{K}[f(\lambda (x_1\wedge K) + {\bar{\lambda }}(x_2\wedge K))]\ge \mathbf{E}_{K}[f({\tilde{x}}\wedge K)] \end{aligned}$$

(3)

for every $x_1, x_2\ge 0$, every random variable K with distribution $F_{K}$ and every convex function f. $\square$

Lemma 2

The following properties hold:

• (i) $V_t(I)$ is convex in I and $V_t(I)\ge 0$ for every I.²

• (ii) $\pi _t(I, \mathbf {q})$ is jointly convex in $(I, \mathbf {q})$.

• (iii) $L_t(I)$ is convex in I.

• (iv) $J_t(I, \mathbf {q})$ is convex in $I, q_1,\ldots ,$ or $q_N$ in each dimension.³

Proof of Lemma 2

We use mathematical induction to proof. we have $V_{T+1}(I)=c_1 \max \{-I,0\} + c_e \max \{I,0\}\ge 0$, which is convex in I. We assume $V_{t+1}(I)$ is convex in I and $V_{t+1}(I)\ge 0$. We first show that $V_t(I)\ge 0$. Notice that

$$\begin{aligned} V_t(I)= & {} \min _{q_i\ge 0, 1\le i\le N}\sum _{i=1}^N c_i\mathbf{E}_{K_i}(q_i\wedge K_i) + \mathbf{E}_{D,K_i, 1\le i\le N} [H(I+\sum _{i=1}^N q_i\wedge K_i-D)]\nonumber \\&+ \;\alpha \mathbf{E}_{D,K_i, 1\le i\le N}[V_{t+1}(I+\sum _{i=1}^N q_i\wedge K_i- D)] \end{aligned}$$

where $\sum _{i=1}^N c_i\mathbf{E}_{K_i}(q_i\wedge K_i)\ge 0$, $H(x)\ge 0$ and $V_{t+1}(x)\ge 0$ for every x. Hence, $V_t(I)\ge 0$.

Notice that

$$\begin{aligned} L_t(x)=\mathbf{E}_{D}[H(x- D)]+ \alpha \mathbf{E}_{D}[V_{t+1}(x- D)] \end{aligned}$$

where H(.) and $V_{t+1}(.)$ are convex and D follows a continuous distribution. Hence $L_t(x)$ is convex in x. We also notice that

$$\begin{aligned} \pi _t(I, \mathbf {q})=\sum _{i=1}^N c_i q_i + L_t\left( I + \sum _{i=1}^N q_i\right) \end{aligned}$$

From the convexity of $L_t(.)$ and linear combination of $I + \sum _{i=1}^N q_i$, we have $L_t(I + \sum _{i=1}^N q_i)$ is jointly convex in $(I,\mathbf {q})$. Clearly, $\sum _{i=1}^N c_i q_i$ is jointly convex in $(I,\mathbf {q})$. Therefore, $\pi _t(I,\mathbf {q})$ is jointly convex in $(I,\mathbf {q})$.

Notice that $J_t(I,\mathbf {q})=\mathbf{E}_{K_i,1\le i\le N}[\pi _t(I,q_1\wedge K_1,\ldots ,q_N\wedge K_N)]$. we have $J_t(I, \mathbf {q})$ is convex in $I, q_1,\ldots ,$ or $q_N$ in each dimension.

For every $I^a$, $I^b$ and $\lambda \in [0,1]$, denote ${\bar{\lambda }}=1-\lambda$ and ${\hat{I}}=\lambda I^a+{\bar{\lambda }} I^b$. We have

$$\begin{aligned}&\lambda V_t(I^a) +{\bar{\lambda }} V_t(I^b)\nonumber \\&\quad =\lambda J_t(I^a, \mathbf {q}_t^*(I^a)) + {\bar{\lambda }} J_t(I^b, \mathbf {q}_t^*(I^b))\nonumber \\&\quad =\lambda \mathbf{E}_{K_i, 1\le i\le N}[\pi _t(I^a, q_{1,t}^*(I^a)\wedge K_1, \ldots , q_{N,t}^*(I_N)\wedge K_N)] \nonumber \\&\qquad +\;{\bar{\lambda }} \mathbf{E}_{K_i, 1\le i\le N}[\pi _t(I^b, q_{1,t}^*(I^b)\wedge K_1, \ldots , q_{N,t}^*(I^b)\wedge K_N)]\nonumber \\&\quad =\mathbf{E}_{K_i, 1\le i\le N}[\lambda \pi _t(I^a, q_{1,t}^*(I^a)\wedge K_1, \ldots ,q_{N,t}^*(I^a)\wedge K_N) \nonumber \\&\qquad +\;{\bar{\lambda }}\pi _t(I^b, q_{1,t}^*(I^b)\wedge K_1,\ldots , q_{N,t}^*(I^b)\wedge K_N)]\nonumber \\&\quad \ge \mathbf{E}_{K_i, 1\le i\le N}[\pi _t({\hat{I}},\, \, \lambda (q_{1,t}^*(I^a)\wedge K_1) + {\bar{\lambda }}(q_{1,t}^*(I^b)\wedge K_1), \dots ,\lambda (q_{N,t}^*(I^a)\wedge K_N) \nonumber \\&\qquad +\;{\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N))] \end{aligned}$$

(4)

From the definitions above and Lemma 1, we have

$$\begin{aligned}&\mathbf{E}_{K_j, \forall j}\big [\pi _t\big ({\hat{I}},\,\, \lambda (q_{1,t}^*(I^a)\wedge K_1) + {\bar{\lambda }}(q_{1,t}^*(I^b)\wedge K_1), \,\,\ldots , \,\,\lambda (q_{N,t}^*(I^a)\wedge K_N) \nonumber \\&\qquad +\;{\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ] \ge \min _{q_1\ge 0} \mathbf{E}_{K_j, \forall j} \big [\pi _t\big ({\hat{I}}, \,\, q_1\wedge K_1, \,\,\lambda (q_{2,t}^*(I^a)\wedge K_2) \nonumber \\&\qquad +\;{\bar{\lambda }}(q_{2,t}^*(I^b)\wedge K_2), \,\, \ldots , \,\, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ] \nonumber \\&\quad \ge \min _{q_1\ge 0} \bigg \{\min _{q_2\ge 0}\mathbf{E}_{K_j, \forall j} \big [\pi _t\big ({\hat{I}}, \,\, q_1\wedge K_1, \,\,q_2\wedge K_2, \,\,\lambda (q_{3,t}^*(I^a)\wedge K_3) \nonumber \\&\qquad + {\bar{\lambda }}(q_{3,t}^*(I^b)\wedge K_3), \,\, \ldots , \,\, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ] \bigg \}\nonumber \\&\quad \ge \min _{q_1\ge 0} \bigg \{\min _{q_2\ge 0}\bigg \{\min _{q_3\ge 0}\mathbf{E}_{K_j, \forall j} \big [\pi _t\big ({\hat{I}}, \,\, q_1\wedge K_1, \,\,q_2\wedge K_2, q_3\,\,\nonumber \\&\qquad \,\wedge K_3, \,\, \lambda (q_{4,t}^*(I^a)\wedge K_4) + {\bar{\lambda }}(q_{4,t}^*(I^b)\wedge K_4), \ldots , \,\, \lambda (q_{N,t}^*(I^a)\wedge K_N) \nonumber \\&\qquad + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ]\bigg \} \bigg \}\nonumber \\&\quad \ge \cdots \ge \min _{q_1\ge 0}\bigg \{\min _{q_2\ge 0}\bigg \{\cdots \bigg \{\min _{q_i\ge 0} \mathbf{E}_{K_j, \forall j} \big [\pi _t\big ({\hat{I}}, \,\, q_1\wedge K_1, \,\, q_2\wedge K_2, \,\, \nonumber \\&\qquad \ldots , \, \, q_{i}\wedge K_i, \,\lambda (q_{i+1,t}^*(I^a)\wedge K_{i+1}) + {\bar{\lambda }}(q_{i+1,t}^*(I^b)\wedge K_{i+1}),\nonumber \\&\qquad \ldots , \,\, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ] \bigg \}\bigg \}\cdots \bigg \}\nonumber \\&\quad \ge \min _{q_1\ge 0}\bigg \{\min _{q_2\ge 0}\bigg \{\cdots \bigg \{\min _{q_{i+1}\ge 0} \mathbf{E}_{K_j, \forall j} \big [\pi _t\big ({\hat{I}}, \,\, q_1\wedge K_1, \,\, q_2\wedge K_2, \,\, \cdots , \, \, \nonumber \\&\qquad q_{i}\wedge K_i, \, q_{i+1}\wedge K_{i+1}, \cdots , \,\, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N)\big )\big ] \bigg \}\bigg \}\cdots \bigg \}\nonumber \\&\quad \ge \cdots \ge \nonumber \\&\quad \ge \min _{q_1\ge 0}\bigg \{\min _{q_2\ge 0}\bigg \{\cdots \bigg \{\min _{q_N\ge 0} \mathbf{E}_{K_j, \forall j} [\pi _t({\hat{I}}, q_1\wedge K_1, \ldots , q_N\wedge K_N)] \bigg \}\bigg \}\cdots \bigg \}\nonumber \\&\quad =\min _{q_1\ge 0}\bigg \{\min _{q_2\ge 0}\bigg \{\cdots \bigg \{\min _{q_N\ge 0} J_t({\hat{I}}, q_1, \ldots , q_N) \bigg \}\bigg \}\cdots \bigg \}\nonumber \\&\quad \ge \min _{q_1\ge 0, q_2\ge 0, \ldots , q_N\ge 0} J_t({\hat{I}}, q_1, \ldots , q_N) =V_t({\hat{I}}) \end{aligned}$$

The explanation of the first inequality is as follows. For every given $({\hat{I}}, q_{2,t}^*(I^a),q_{2,t}^*(I^b),q_{3,t}^*(I^a),$ $q_{3,t}^*(I^b),\ldots ,q_{N,t}^*(I^a),q_{N,t}^*(I^b))$, the expression $\mathbf{E}_{K_j, \forall j\ne 1}[\pi _t({\hat{I}}, x, \,\,\lambda (q_{2,t}^*(I^a)\wedge K_2) + {\bar{\lambda }}(q_{2,t}^*(I^b)\wedge K_2), \, \ldots , \,\lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N))]$ can been looked as a one-dim convex function about x. Hence, from Lemma 1, we have the first inequality. That is to say, the first inequality holds for every given $({\hat{I}}, q_{2,t}^*(I^a),q_{2,t}^*(I^b), q_{3,t}^*(I^a),$ $q_{3,t}^*(I^b),\ldots ,q_{N,t}^*(I^a),q_{N,t}^*(I^b))$.

The explanation of the second inequality is as follows. For every given $({\hat{I}}, q_1,q_{3,t}^*(I^a),q_{3,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$, the expression $\mathbf{E}_{K_j, \forall j\ne 2} [\pi _t({\hat{I}}, q_1\wedge K_1, x, \,\lambda (q_{3,t}^*(I^a)\wedge K_3) + {\bar{\lambda }}(q_{3,t}^*(I^b)\wedge K_3), \,\ldots , \, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N))]$ can been looked as a one-dim convex function about x. Hence, from Lemma 1, we have the second inequality. That is to say, the second inequality holds for every given $({\hat{I}}, q_1,q_{3,t}^*(I^a),q_{3,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$.

The explanation of the third inequality is as follows. For every given $({\hat{I}}, q_1, q_2, q_{4,t}^*(I^a), q_{4,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$, the expression $\mathbf{E}_{K_j, \forall j\ne 3}[\pi _t({\hat{I}}, q_1\wedge K_1, q_2\wedge K_2, x, \, \lambda (q_{4,t}^*(I^a)\wedge K_4) + {\bar{\lambda }}(q_{4,t}^*(I^b)\wedge K_4), \, \ldots , \, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N))]$ can been looked as a one-dim convex function about x. Hence, from Lemma 1, we have the third inequality. That is to say, the third inequality holds for every given $({\hat{I}}, q_1, q_2, q_{4,t}^*(I^a),q_{4,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$.

The explanation of the sixth inequality is as follows. For every given $({\hat{I}}, q_1, q_2,\ldots , q_i, q_{i+2,t}^*(I^a),q_{i+2,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$, the expression $\mathbf{E}_{K_j, \forall j\ne i+1} [\pi _t({\hat{I}}, q_1\wedge K_1,q_2\wedge K_2, \ldots , q_i\wedge K_i, x, \,\lambda (q_{i+2,t}^*(I^a)\wedge K_{i+2}) + {\bar{\lambda }}(q_{i+1,t}^*(I^b)\wedge K_{i+1}), \ldots , \, \lambda (q_{N,t}^*(I^a)\wedge K_N) + {\bar{\lambda }}(q_{N,t}^*(I^b)\wedge K_N))]$ can been looked as a one-dim convex function about x. Hence, from Lemma 1, we have the sixth inequality. That is to say, the sixth inequality holds for every given $({\hat{I}}, q_1, q_2,\ldots ,q_i, q_{i+2,t}^*(I^a),q_{i+2,t}^*(I^b),\ldots ,$ $q_{N,t}^*(I^a),q_{N,t}^*(I^b))$.

The explanation of the ninth inequality is as follows. For every given $({\hat{I}}, q_1, q_2,\ldots ,q_{N-1})$, the expression $\mathbf{E}_{K_j, \forall j\ne i+1} [\pi _t({\hat{I}}, q_1\wedge K_1,q_2\wedge K_2, \ldots , q_{N-1}, x)]$ can been looked as a one-dim convex function about x. Hence, from Lemma 1, we have the ninth inequality. That is to say, the ninth inequality holds for every given $({\hat{I}}, q_1, q_2,\ldots ,q_{N-1})$.

The tenth inequality comes from sequential minimization is bigger than the global minimization.

Combining with (4), we have

$$\begin{aligned} \lambda V_t(I^a) +{\bar{\lambda }} V_t(I^b) \ge V_t({\hat{I}}) \end{aligned}$$

Therefore $V_t(I)$ is convex in I. By induction, $V_t$ is convex $\forall t$, concluding the proof. Parts (ii) and (iii) follow from the discussion above. $\square$

Theorem 7

The optimal ordering policy is a reorder point policy; i.e.,

$$\begin{aligned} q_{i,t}^*(I)= \left\{ \begin{array}{cl} >0 &{} \hbox { for } I<I_{i,t}\\ =0 &{} \hbox { for } I\ge I_{i,t} \end{array} \right. \end{aligned}$$

and $V_t^{\prime }(I_{i,t})= -c_i$; $1 \le i \le N$.

Proof of Theorem 7

From the first order conditions (FOC) with respect to $q_i$ for all i, we have

$$\begin{aligned}&\frac{\partial J_t}{q_i}={\bar{G}}_i(q_i)\phi _{i,t}(I,\mathbf {q})\nonumber \\&\phi _{i,t}(I,\mathbf {q})=c_i+ L_t^{\prime }(I+q_i+\sum _{j\ne i}q_j\wedge K_j) \end{aligned}$$

(5)

From convexity of $L_t$, we have $\phi _{i,t}(I,\mathbf {q})$ increasing in $q_l$, for all $1\le l\le N$.

In the following discussion, we will show that the optimal ordering policy for Supply i is a reorder-point policy.

Denote

$$\begin{aligned} \mathfrak {J}_{t}^i(I, q_1, \ldots , q_N)=c_iq_i+\sum _{j\ne i}^N c_j\mathbf{E}_{K_j}(q_j\wedge K_j) + \mathbf{E}_{K_j,j\ne i} [L_t(I+q_i+\sum _{j\ne i} q_j\wedge K_j)]\\ ({\tilde{q}}_{1,t}^i(I), \ldots , {\tilde{q}}_{N,t}^i(I))=\arg \min _{q_j\ge 0, \forall j} \, \, \mathfrak {J}_{t}^i(I, q_1, \ldots , q_N) \end{aligned}$$

We next will show that $q_{i,t}^*(I)=0$ if and only if ${\tilde{q}}_{i,t}^i(I)=0$.

If ${\tilde{q}}_{i,t}^i=0$, then

$$\begin{aligned}&J_{t}^i(I, {\tilde{q}}_{1,t}^i(I), \ldots , {\tilde{q}}_{N,t}^i(I))=\mathfrak {J}_{t}^i(I, {\tilde{q}}_{1,t}^i(I), \ldots , {\tilde{q}}_{N,t}^i(I)) \nonumber \\&\quad \le \mathfrak {J}_{t}^i(I, q_{1,t}^*(I), \ldots , q_{N,t}^*(I))\le J_{t}^i(q_{1,t}^*(I), \ldots , q_{N,t}^*(I)) \end{aligned}$$

The first inequality comes from the optimality of $({\tilde{q}}_{1,t}^i(I), \ldots , {\tilde{q}}_{N,t}^i(I))$. The second inequality comes from Jensen’s inequality. Recall that we always pick the smallest optimal solution. Therefore, $q_{i,t}^*(I)=0$.

If $q_{i,t}^*(I)=0$, then we have

$$\begin{aligned} \frac{\partial \mathfrak {J}_{t}^i}{q_j}=\frac{\partial J_t}{q_j}, \, \, \forall j \end{aligned}$$

That is to say $(q_{1,t}^*(I),\ldots , q_{N,t}^*(I))$ is also the minimizer of $\mathfrak {J}_{t}^i$. Hence, ${\tilde{q}}_{i,t}^i=0$.

From the discussion above, we have $q_{i,t}^*(I)=0$ if and only if ${\tilde{q}}_{i,t}^i(I)=0$. Hence, to show that the optimal ordering policy for Supply i is a reorder-point policy, we only need to show that the optimal ordering quantity, ${\tilde{q}}_{i,t}^i(I)$, of Supply i in minimizing $\mathfrak {J}_{t}^i$ is a reorder-point policy. Denote

$$\begin{aligned} f(x)=\min _{q_j, \forall j\ne i} \sum _{j\ne i}^N c_j\mathbf{E}_{K_j}(q_j\wedge K_j) + \mathbf{E}_{K_j,j\ne i} [L_t(x+\sum _{j\ne i} q_j\wedge K_j)] \end{aligned}$$

From convexity of $L_t$, we have convexity of f. We have

$$\begin{aligned}&\min _{q_j, \forall j} \mathfrak {J}_{t}^i(q_1, \ldots , q_N)= \min _{q_i} \big [c q_i+f(I+q_i)\big ]. \end{aligned}$$

Notice that the right hand side is supermodular in $(I,q_i)$. That is to say, ${\tilde{q}}_{i,t}^i(I)$ is decreasing in I. Combining with the result that $q_{i,t}^*(I)=0$ if and only if ${\tilde{q}}_{i,t}^i(I)=0$, we have the optimal ordering policy for Supply i is a reorder-point policy; i.e., $q_{i,t}^*(I)>0$ for $I<I_{i,t}$ and $q_{i,t}^*(I)=0$ for $I\ge I_{i,t}$.

Next we will show that $V_t^{\prime }(I_{i,t})= -c_i$. From $q_{i,t}^*(I)>0$ for $I<I_{i,t}$ and $q_{i,t}^*(I)=0$ for $I\ge I_{i,t}$, we have

$$\begin{aligned} \frac{\partial J_t}{q_i}\bigg |_{I=I_{i,t}, \mathbf {q}=\mathbf {q}_t^*(I_{i,t})}=c_i+ L_t^{\prime }(I_{i,t}+\sum _{j\ne i}q_{j,t}^*(I_{i,t})\wedge K_j)=0. \end{aligned}$$

Hence,

$$\begin{aligned}&V_t^{\prime }(I_{i,t})=\mathbf{E}_{K_j, \forall j} L_t^{\prime }(I_{i,t}+\sum _{j=1}^{N} q_{j,t}^*(I_{i,t})\wedge K_j)\\&\quad =L_t^{\prime }(I_{i,t}+\sum _{j\ne i} q_{j,t}^*(I_{i,t})\wedge K_j)= -c_i. \end{aligned}$$

concluding the proof. $\square$

Proof of Theorem 1

To see Part (i), from $K_{j^{\prime }}\equiv +\infty$, then ${\bar{G}}_{j^{\prime }}(q_{j^{\prime }})=1$ and $q_{j^{\prime }}=q_{j^{\prime }}\wedge K_{j^{\prime }}$ for every $q_{j^{\prime }}$. Thus, for $I\le I_{j^{\prime },t}$,

$$\begin{aligned} \frac{\partial J_t}{q_{j^{\prime }}}=c_{j^{\prime }}+ \mathbf{E}_{K_i, i\ne j^{\prime }} L_t^{\prime }(I+q_{j^{\prime }}+\sum _{i\ne j^{\prime }}q_i\wedge K_i)=c_{j^{\prime }}+ \mathbf{E}_{K_i,\forall i} L_t^{\prime }(I+\sum _{i=1}^{N} q_i\wedge K_i)=0 \end{aligned}$$

Hence,

$$\begin{aligned}&\mathbf{E}_{K_i, \forall i} L_t^{\prime }(I+\sum _{i=1}^{N} q_{i,t}^*(I)\wedge K_i)=-c_{j^{\prime }};\\&V_t^{\prime }(I)=\mathbf{E}_{K_i,\forall i} L_t^{\prime }(I+\sum _{i=1}^{N} q_{i,t}^*(I)\wedge K_i)=-c_{j^{\prime }}. \end{aligned}$$

We also have if $q_i>0$, then for every $i>j^{\prime }$,

$$\begin{aligned} 0= & {} \frac{\partial J_t}{\partial q_{i}}=c_i+ \mathbf{E}_{K_j, j\ne i} L_t^{\prime }(I+q_{i,t}^*(I)+\sum _{j\ne i}q_{j,t}^*(I)\wedge K_j)\\\ge & {} c_i+ \mathbf{E}_{K_j, j\ne i} L_t^{\prime }(I+q_{i,t}^*(I)\wedge K_i+\sum _{j\ne i}q_{j,t}^*(I)\wedge K_j)\\= & {} c_i+ \mathbf{E}_{K_j,j\ne i} L_t^{\prime }(I+\sum _{j=1}^{N}q_{j,t}^*(I)\wedge K_j) =c_i - c_{j^{\prime }}>0. \end{aligned}$$

The first inequality comes from the convexity of $L_t$ and the fact that $q_{i,t}^*(I)\ge q_{i,t}^*(I)\wedge K_j$. The second inequality comes from $c_i>c_{j^{\prime }}$, $\forall i>j^{\prime }$. Therefore, $q_{i,t}^*(I)=0$ for every I and $i>j^{\prime }$.

To see Part (ii), we have shown that $V_t^{\prime }(I)= -c_{j^{\prime }}$ for all $I\le I_{j^{\prime },t}$. We also have $q_{i,t}^*(I)=q_{i,t}^*(I_{j^{\prime },t})=0$, for every $i>j^{\prime }$. Next we focus on $i\le j^{\prime }$. The FOC at $I=I_{j^{\prime },t}$ can be written as

$$\begin{aligned} \frac{\partial J_t}{\partial q_{j^{\prime }}}\bigg |_{I=I_{j^{\prime },t},\,\, \mathbf {q}_{i}=\mathbf {q}_{i,t}^*(I_{j^{\prime },t})}= & {} c_{j^{\prime }}+ \mathbf{E}_{K_j, 1\le j\le j^{\prime }-1} L_t^{\prime }(I_{j^{\prime }}+\sum _{j=1}^{j^{\prime }-1}q_j\wedge K_j)=0;\end{aligned}$$

(6)

$$\begin{aligned} \frac{\partial J_t}{\partial q_i}\bigg |_{I=I_{j^{\prime },t},\,\, \mathbf {q}_{i}=\mathbf {q}_{i,t}^*(I_{j^{\prime },t})}= & {} {\bar{G}}_i(q_i)\bigg (c_i+ \mathbf{E}_{K_j, 1\le j\le j^{\prime }-1, j\ne i} L_t^{\prime }(I_{j^{\prime }}+q_i\nonumber \\&\quad + \sum _{1\le j\le j^{\prime }-1, j\ne i}q_j\wedge K_j)\bigg ) \nonumber \\= & {} 0, \,\, \forall i<j^{\prime } \end{aligned}$$

(7)

From the result in Part (i), the objective function can be rewritten as

$$\begin{aligned} V_t(I)= & {} -c_j^{\prime } I+ \min _{x\ge I, q_i\ge 0, \forall 1\le i<j^{\prime }} f(x,q_1,\ldots ,q_{j^{\prime }-1}) \end{aligned}$$

where

$$\begin{aligned} f(x,q_1,\ldots ,q_{j^{\prime }-1})= c_j^{\prime }x + \sum _{i=1}^{j^{\prime }-1} c_i\mathbf{E}_{K_i}(q_i\wedge K_i) + \mathbf{E}_{K_i, 1\le i\le j^{\prime }-1} [L_t(x+\sum _{i=1}^{j^{\prime }-1} q_i\wedge K_i)]. \end{aligned}$$

From FOC, we have

$$\begin{aligned}&\frac{\partial f}{\partial x}=c_{j^{\prime }}+ \mathbf{E}_{K_j, 1\le j\le j^{\prime }-1} L_t^{\prime }(x+\sum _{j=1}^{j^{\prime }-1}q_j\wedge K_j)\\&\frac{\partial f}{\partial q_i}={\bar{G}}_i(q_i)\bigg (c_i+ \mathbf{E}_{K_j, 1\le j\le j^{\prime }-1, j\ne i} L_t^{\prime }(x+q_i+ \sum _{1\le j\le j^{\prime }-1, j\ne i}q_j\wedge K_j)\bigg ), \forall i<j^{\prime } \end{aligned}$$

Combining with (6) and (7), we have $I\le I_{j^{\prime }}$

$$\begin{aligned} V_t(I)=-c_j^{\prime } I+ f(I_{j^{\prime }},q_{1,t}^*(I_{j^{\prime },t}), \cdot , q_{j^{\prime }-1,t}^*(I_{j^{\prime },t})). \end{aligned}$$

That is to say, for $I\le I_{j^{\prime }}$, $q_{j^{\prime },t}^*=I_{j^{\prime },t}-I$ and $q_{i,t}^*(I)=q_{i,t}^*(I_{j^{\prime },t})$ for every $i<j^{\prime }$. Combining results with $q_{i,t}^*(I)=0$ for $i>j^{\prime }$, we conclude the proof. $\square$

Proposition 4

$I_{1,t}>I_{2,t}>\ldots >I_{N,t}$ for $c_i \le c_{i+1}$.

Proof of Proposition 4

From $c_i\le c_{i+1}$ and Theorem 7, we have $V_t^{\prime }(I_{i,t})>V_t^{\prime }(I_{i+1,t})$. Combining with the convexity of $V_t$, we have $I_{i,t}>I_{i+1,t}$ for all i. $\square$

Proof of Proposition 1

Assume there exist I and i satisfying that $q_{i,t}^*({\tilde{I}})< q_{i+1,t}^*({\tilde{I}})$. Combining with Proposition 4, there must exist ${\tilde{I}}$ and ${\tilde{q}}$ satisfying that $q_{i,t}^*({\tilde{I}})=q_{i+1,t}^*({\tilde{I}})={\tilde{q}}>0$.

From $K_i \succeq _{st} K_{i+1}$, we have $\big ({\tilde{q}} \wedge K_i\big )\succeq _{st} \big ({\tilde{q}} \wedge K_{i+1}\big )$. Combining it with the convexity of $L_t$, we have

$$\begin{aligned} \mathbf{E}_{K_i}L_t^{\prime }(x+{\tilde{q}} \wedge K_i) \ge \mathbf{E}_{K_{i+1}}L_t^{\prime }(x+{\tilde{q}} \wedge K_{i+1}), \, \, \forall x. \end{aligned}$$

(8)

From $q_{i,t}^*({\tilde{I}})=q_{i+1,t}^*({\tilde{I}})={\tilde{q}}>0$ and

$$\begin{aligned}&0=\frac{\partial J_t}{q_i}|_{I={\tilde{I}}, \mathbf {q}=\mathbf {q}_t^*(I)}={\bar{G}}_i({\tilde{q}})\big (c_i+ L_t^{\prime }({\tilde{I}}+{\tilde{q}}+ {\tilde{q}}\wedge K_{i+1}+\sum _{j\ne i,i+1}q_j\wedge K_j)\big )\end{aligned}$$

(9)

$$\begin{aligned}&0=\frac{\partial J_t}{q_{i+1}}|_{I={\tilde{I}}, \mathbf {q}=\mathbf {q}_t^*(I)}={\bar{G}}_{i+1}({\tilde{q}})\big (c_{i+1}+ L_t^{\prime }({\tilde{I}}+{\tilde{q}}+ {\tilde{q}}\wedge K_i+\sum _{j\ne i,i+1}q_j\wedge K_j)\big ).\nonumber \\ \end{aligned}$$

(10)

Combining the above with (8) and $c_i<c_{i+1}$, we have

$$\begin{aligned} 0= & {} c_{i+1}+ L_t^{\prime }({\tilde{I}}+{\tilde{q}}+ {\tilde{q}}\wedge K_i+\sum _{j\ne i,i+1}q_j\wedge K_j)\\> & {} c_i+ L_t^{\prime }({\tilde{I}}+{\tilde{q}}+ {\tilde{q}}\wedge K_{i+1}+\sum _{j\ne i,i+1}q_j\wedge K_j)=0. \end{aligned}$$

We reach a contradiction. Hence, we conclude the proof. $\square$

Proof of Theorem 2

To simplify the following discussion, we define $\mathbf {q}^s=(q,\ldots ,q)$. To see part (i)& (ii), we have that

$$\begin{aligned} \frac{d J_t(I,\mathbf {q}^s)}{dq}= & {} \sum _{i=1}^{N}{\bar{G}}_i(q)\bigg (c_i+\mathbf{E}_{K_j, j\ne i}[L_t^{\prime }(I+q+\sum _{j\ne i}q\wedge K_j)]\bigg ) \nonumber \\= & {} \sum _{i=1}^{N}{\bar{G}}_i(q) \phi _{i,t}(I,q,\ldots ,q) \end{aligned}$$

(11)

Hence, we have

$$\begin{aligned} \frac{\partial ^2 J_t(I,\mathbf {q}^s)}{\partial q \partial I} = \sum _{i=1}^{N}{\bar{G}}_i(q) \mathbf{E}_{K_j, j\ne i}[L_t^{\prime \prime }(I+q+\sum _{j\ne i}q\wedge K_j)]\ge 0. \end{aligned}$$

Thus, $J_t(I,\mathbf {q}^s)$ is supermodular in (I, q). Therefore $q_{x,t}^*(I)$ decreases in I.

To see parts (iii–v), we consider two different cases. Case (a) $q_{x,t}^*(I)=0$ and Case (b) $q_{x,t}^*(I)>0$.

Case (a), $q_{x,t}^*(I)=0$. Combining with (11) and $c_N>c_i$ for $1\le i <N$,

$$\begin{aligned} 0\le \frac{dJ(I,\mathbf {q}^s)}{dq}\big |_{q=0} =\sum _{i=1}^N\bigg ({\bar{G}}_i(q)[c_i+L_t^{\prime }(I)]\bigg ) \le \bigg (\sum _{i=1}^N {\bar{G}}_i(q)\bigg )(c_N+L_t^{\prime }(I)) \end{aligned}$$

Thus, $\phi _{N,t}(I,0,\ldots , 0)\ge 0$. Then, $q_{N,t}^*(I)=0$. Therefore $q_{N,t}^*(I)=q_{x,t}^*(I)\le q_{1,t}^*(I)$.

Case (b), $q_{x,t}^*(I)>0$. If $I\ge I_{1,t}$, then we have $c_i+L_t^{\prime }(I)>c_1+L_t^{\prime }(I)\ge 0, \,\, \forall i>1$. Hence,

$$\begin{aligned} 0=\frac{dJ_t(I,\mathbf {q}^s)}{dq}\big |_{q=0}=\sum _{i=1}^{N}\bigg ({\bar{G}}_i(q)(c_i+L_t^{\prime }(I))\bigg )>0. \end{aligned}$$

We reach a contradiction. Hence, $I<I_{1,t}$ when $q_{x,t}^*(I)>0$.

If $\min \{q_{i,t}^*(I), 1\le i\le N\}>q_{x,t}^*(I)$, then from (5), we have

$$\begin{aligned} 0=\phi _{i,t}(I,\mathbf {q}_{t}^*(I))\ge \phi _{i,t}(I,q_{x,t}^*(I),\ldots ,q_{x,t}^*(I)) \end{aligned}$$

Combining with (11), we have

$$\begin{aligned} 0=\phi _{i,t}(I,\mathbf {q}_{t}^*(I))=\phi _{i,t}(I,q_{x,t}^*(I),\ldots ,q_{x,t}^*(I))=0, \forall i. \end{aligned}$$

Therefore, $(q_{x,t}^*(I),\ldots ,q_{x,t}^*(I))$ is also the minimizer of $J_t(I,q_1, \ldots , q_N)$. It contradicts with the assumption that we always pick the smallest as the optimal solution.

If $\max \{q_{i,t}^*(I), 1\le i\le N\}<q_{x,t}^*(I)$, then from (5), we have

$$\begin{aligned} 0=\phi _{i,t}(I,\mathbf {q}_{t}^*(I))\le \phi _{i,t}(I,q_{x,t}^*(I),\ldots ,q_{x,t}^*(I)) \end{aligned}$$

Combining with (11), we have

$$\begin{aligned} 0=\phi _{i,t}(I,\mathbf {q}_{t}^*(I))=\phi _{i,t}(I,q_{x,t}^*(I),\ldots ,q_{x,t}^*(I))=0, \forall i. \end{aligned}$$

Let ${\tilde{q}}=\max \{q_{i,t}^*(I), 1\le i\le N\}$. From the convexity of $L_t$, we have

$$\begin{aligned} 0=\phi _{i,t}(I,\mathbf {q}_{t}^*(I))=\phi _{i,t}(I,{\tilde{q}},\ldots ,{\tilde{q}})=\phi _{i,t}(I,q_{x,t}^*(I),\ldots ,q_{x,t}^*(I))=0, \forall i. \end{aligned}$$

Hence,

$$\begin{aligned} \frac{d J_t(I,\mathbf {q}^s)}{dq}\bigg |_{q={\tilde{q}}}=\sum _{i=1}^{N}({\bar{G}}_i(q) \phi _{i,t}(I,{\tilde{q}},{\tilde{q}}))=0. \end{aligned}$$

Notice that ${\tilde{q}}<q_{x,t}^*(I)$ which contradicts with the fact that $q_{x,t}^*(I)$ is the smallest minimizer of $J_t(I, q, \ldots , q)$. Combining the discussions on Case a) & b), we complete the proof for Parts (iii)-(iv). $\square$

Proof of Theorem 3

To see part (i),(ii)& (iii), we will show $\frac{dq_{i,t}^*(I)}{dI}\in [-1,0]$, $i=1,2$. First, the first order condition for $q_i$ are expressed as follows. For $i=1,2$ and $j\ne i$

$$\begin{aligned}&\frac{\partial J_t}{\partial q_i}=\phi _{i,t}(I, q_1, q_2) {\bar{G}}_i(q_i) \end{aligned}$$

(12)

where $G_i(\cdot )$ is the distribution of $K_i$ with ${\bar{G}}_i(x)=1-G_j(x)$, $i=1,2$, and

$$\begin{aligned} \phi _{i,t}(I, q_1, q_2)=c_i+\mathbf{E}_{K_j}[L_t^{\prime }(I+q_i + q_j\wedge K_j)]. \end{aligned}$$

(13)

We can obtain the convexity of $L_t$ from the convexity of $V_{t+1}$ and H. Hence, $\phi _{i,t}(I, q_1, q_2)$ ($i=1,2$) increases in $q_1$ and $q_2$. From the envelop theorem, we have

$$\begin{aligned}&\frac{dq_{1,t}^*(I)}{dI}+ \frac{{\bar{G}}_2(q_2) L_t^{\prime \prime }(I+q_1+q_2)}{\mathbf{E}_{K_2} L_t^{\prime \prime }(I+q_1+q_2\wedge K_2)}\frac{dq_{2,t}^*(I)}{dI} = -1 \end{aligned}$$

(14)

$$\begin{aligned}&\frac{dq_{1,t}^*(I)}{dI}\frac{{\bar{G}}_1(q_1) L_t^{\prime \prime }(I+q_1+q_2)}{\mathbf{E}_{K_1} L_t^{\prime \prime }(I+q_1\wedge K_1+q_2)}+ \frac{dq_{2,t}^*(I)}{dI} = -1 \end{aligned}$$

(15)

To simplify the notation, denote

From the convexity of $L_t$, we have $x_i, \delta _i\ge 0$ for $i=1,2$. From (14) and (15), we have

$$\begin{aligned} \frac{dq_{1,t}^*(I)}{dI} =- \frac{ (x_1+\delta 1)(x_2+\delta _2) - x_1(x_2+\delta )}{(x_1+\delta _1)(x_2+\delta _2)-x_1x_2}= -\frac{\delta _1 x_2+\delta _1\delta _2}{\delta 1 x_2+x_1\delta _2+\delta _1\delta _2} \end{aligned}$$

It is easy to see, $\frac{dq_{1,t}^*(I)}{dI}\in [-1,0]$. By a similar argument, we have $\frac{dq_{2,t}^*(I)}{dI}\in [-1,0]$ proving part (i). Part (ii) directly follows from (i) and part (iii) directly follows from $\frac{dq_{i,t}^*(I)}{dI}\in [-1,0]$.

To see part (iv), we consider the following two cases: Case (a), $I\le I_{2,t}$ and Case (b), $I\in [I_{2,t}, I_{1,t}]$.

Case (a), we will show that $1+ \frac{dq_{1,t}^*(I)}{dI}+\frac{dq_{2,t}^*(I)}{dI}\le I$ when $I\le I_{2,t}$. From the above discussion, we have

$$\begin{aligned} -\left( \frac{dq_{1,t}^*(I)}{dI}+\frac{dq_{2,t}^*(I)}{dI}\right) =\frac{\delta _1 x_2+\delta _2 x_1+2\delta _1\delta _2}{\delta 1 x_2+x_1\delta _2+\delta _1\delta _2}\ge 1 \end{aligned}$$

That is to say, $1+ \frac{dq_{1,t}^*(I)}{dI}+\frac{dq_{2,t}^*(I)}{dI}\le I$.

Case (b) $I\in [I_{2,t}, I_{1,t}]$. We have

$$\begin{aligned} \phi _{1,t}(I,q_1,0)=c_1+\mathbf{E}_{D} L_t(I+q_1-D)=0 \end{aligned}$$

Hence, $q_{1,t}^*(I)=I_{1,t}-I$ when $I\in [I_{2,t}, I_{1,t}]$. Therefore, $I+q_{1,t}^*(I)+q_{2,t}^*(I)=I_{1,t}$. We conclude the proof. $\square$

Proof of Theorem 4

Notice that when $q_{x,t}^*(I)=0$, $f_t(I,q_{x,t}^*(I))=0<c_2-c_1$. From Proposition 4 and Theorem 2, we have $q_{2,t}^*=q_{x,t}^*(I)=0\le q_{1,t}^*(I)=0$.

Next, we focus on discussing that $q_{x,t}^*(I)>0$. The next discussions are based on the following two equations.

$$\begin{aligned}&\frac{dJ(I,q,q)}{dq}|_{q_{x,t}^*(I)}={\bar{G}}_1(I)\phi _{1,t}(I,q,q)+{\bar{G}}_2(I)\phi _{2,t}(I,q,q)=0 \end{aligned}$$

(16)

$$\begin{aligned}&\phi _{1,t}(I,q,q)-\phi _{2,t}(I,q,q)=f_t(I,q) - (c_2-c_1) \end{aligned}$$

(17)

To see part (i), if $f_t(I,q_{x,t}^*(I))= c_2-c_1$, then from (16) and (17) we have $\phi _{1,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))=\phi _{2,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))=0$. Hence, $q_{2,t}^*(I)=q_{1,t}^*(I)=q_{x,t}^*(I)$.

To see part (ii), if $f_t(I,q_{x,t}^*(I))>c_2-c_1$, then from (16) and (17) we have $\phi _{1,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))>0$ and $\phi _{2,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))<0$. Combining with Proposition 4 & Theorem 2 and $q_{x,t}^*(I)>0$, we have

$$\begin{aligned}&\phi _{1,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))>0=\phi _{1,t}(I,q_{1,t}^*(I),q_{2,t}^*(I))\\&\phi _{2,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))<0\le \phi _{2,t}(I,q_{1,t}^*(I),q_{2,t}^*(I)). \end{aligned}$$

Then

$$\begin{aligned}&\mathbf{E}_{K_2}[L_t^{\prime }(I+q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I))]>\mathbf{E}_{K_2}[L_t^{\prime }(I+q_{2,t}^*(I)\wedge K_2+q_{1,t}^*(I))] \qquad \end{aligned}$$

(18)

$$\begin{aligned}&\mathbf{E}_{K_1}[L_t^{\prime }(I+q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I))]<\mathbf{E}_{K_1}[L_t^{\prime }(I+q_{1,t}^*(I)\wedge K_1+q_{2,t}^*(I))]. \qquad \end{aligned}$$

(19)

If $q_{1,t}^*(I)=q_{2,t}^*(I)$, then we have $q_{1,t}^*(I)=q_{2,t}^*(I)=q_{x,t}^*(I)$. It contradicts with (18) and (19).

If $q_{2,t}^*(I)<q_{1,t}^*(I)$, then let $\delta _1=q_{x,t}^*(I)-q_{2,t}^*(I)$ and $\delta _2=q_{1,t}^*(I)-q_{x,t}^*(I)$. Further we have $\delta _1\ge 0$ and $\delta _2\ge 0$ from Theorem 2.

If $\delta _1\ge \delta _2$, then we have

$$\begin{aligned}&\mathbf{E}_{K_2}[L_t^{\prime }(I+q_{2,t}^*(I)\wedge K_2+q_{1,t}^*(I))] =\mathbf{E}_{K_2}[L_t^{\prime }(I+(q_{x,t}^*(I)-\delta _2)\wedge K_2+q_{x,t}^*(I)+\delta _1)]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \ge \mathbf{E}_{K_2}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I)+\delta _1-\delta _2)]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \ge \mathbf{E}_{K_2}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I))] \end{aligned}$$

The first inequality comes from $(q_{x,t}^*(I)-\delta _2)\wedge {\hat{K}}_2\ge q_{x,t}^*(I)\wedge {\hat{K}}_2-\delta _2$ for every realization of ${\hat{K}}_2$. The second inequality comes from the convexity of $L_t$ and $\delta _1\ge \delta _2$. It contradicts with (18).

If $\delta _1\le \delta _2$, then we have

$$\begin{aligned}&\mathbf{E}_{K_1}[L_t^{\prime }(I+q_{1,t}^*(I)\wedge K_1+q_{2,t}^*(I))] =\mathbf{E}_{K_1}[L_t^{\prime }(I+(q_{x,t}^*(I)+\delta _1)\wedge K_1+q_{x,t}^*(I)-\delta _2)]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \le \mathbf{E}_{K_1}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I)+\delta _1-\delta _2)]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \le \mathbf{E}_{K_1}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I))] \end{aligned}$$

The first inequality comes from $(q_{x,t}^*(I)+\delta _1)\wedge {\hat{K}}_1\le q_{x,t}^*(I)\wedge {\hat{K}}_1 + \delta _1$ for every realization of ${\hat{K}}_1$. The second inequality comes from the convexity of $L_t$ and $\delta _1\le \delta _2$. It contradicts with (19).

Combining the above discussion, we have that $q_{2,t}^*(I)>q_{1,t}^*(I)$ if $f_t(I,q_{x,t}^*(I))> c_2-c_1$.

To see part (iii), if $f_t(I,q_{x,t}^*(I))<c_2-c_1$, then from (16) and (17), we have $\phi _{1,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))<0$ and $\phi _{2,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))>0$. We assume $q_{2,t}^*(I)>q_{1,t}^*(I)$ and then reach a contradiction.

From the assumption $q_{2,t}^*(I)>q_{1,t}^*(I)$ and Proposition 4, we have $I<I_{2,t}$ and $q_{2,t}^*(I)>q_{1,t}^*(I)>0$. Hence,

$$\begin{aligned}&\phi _{1,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))<0=\phi _{1,t}(I,q_{1,t}^*(I),q_{2,t}^*(I))\\&\phi _{2,t}(I,q_{x,t}^*(I),q_{x,t}^*(I))>0=\phi _{2,t}(I,q_{1,t}^*(I),q_{2,t}^*(I)). \end{aligned}$$

Then

$$\begin{aligned}&\mathbf{E}_{K_2}[L_t^{\prime }(I+q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I))]<\mathbf{E}_{K_2}[L_t^{\prime }(I+q_{2,t}^*(I)\wedge K_2+q_{1,t}^*(I))] \qquad \end{aligned}$$

(20)

$$\begin{aligned}&\mathbf{E}_{K_1}[L_t^{\prime }(I+q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I))]>\mathbf{E}_{K_1}[L_t^{\prime }(I+q_{1,t}^*(I)\wedge K_1+q_{2,t}^*(I))]. \qquad \end{aligned}$$

(21)

let $\delta _3=q_{x,t}^*(I)-q_{1,t}^*(I)$ and $\delta _4=q_{2,t}^*(I)-q_{x,t}^*(I)$. Then we have $\delta _4\ge 0$ and $\delta _5\ge 0$.

If $\delta _4\le \delta _3$, then we have

$$\begin{aligned} \mathbf{E}_{K_2}[L_t^{\prime }(I+q_{2,t}^*(I)\wedge K_2+q_{1,t}^*(I))]= & {} \mathbf{E}_{K_2}[L_t^{\prime }(I+(q_{x,t}^*(I)+\delta _4)\wedge K_2+q_{x,t}^*(I)+\delta _3)]\\\le & {} \mathbf{E}_{K_2}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I)+\delta _4-\delta _3)]\\\le & {} \mathbf{E}_{K_2}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_2+q_{x,t}^*(I))] \end{aligned}$$

The first inequality comes from $(q_{x,t}^*(I)+\delta _4)\wedge {\hat{K}}_2\le q_{x,t}^*(I)\wedge {\hat{K}}_2 + \delta _4$ for every realization of ${\hat{K}}_2$. The second inequality comes from the convexity of $L_t$ and $\delta _4\le \delta _3$. It contradicts with (20).

If $\delta _4\ge \delta _3$, then we have

$$\begin{aligned} \mathbf{E}_{K_1}[L_t^{\prime }(I+q_{1,t}^*(I)\wedge K_1+q_{2,t}^*(I))]= & {} \mathbf{E}_{K_1}[L_t^{\prime }(I+(q_{x,t}^*(I)-\delta _3)\wedge K_1+q_{x,t}^*(I)+\delta _4)]\\\ge & {} \mathbf{E}_{K_1}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I)+\delta _4-\delta _3)]\\\ge & {} \mathbf{E}_{K_1}[L_t^{\prime }(I+ q_{x,t}^*(I)\wedge K_1+q_{x,t}^*(I))] \end{aligned}$$

The first inequality comes from $(q_{x,t}^*(I)-\delta _3)\wedge {\hat{K}}_1\ge q_{x,t}^*(I)\wedge {\hat{K}}_1-\delta _3$ for every realization of ${\hat{K}}_1$. The second inequality comes from convexity of $L_t$ and $\delta _4\ge \delta _3$. It contradicts with (21).

Combining the above discussion, we have that $q_{2,t}^*(I)\le q_{1,t}^*(I)$ if $f_t(I,q_{x,t}^*(I))<c_2-c_1$. $\square$

Proof of Theorem 5

If $\theta _1\ge \theta _2$, then $K_1\succeq _{st} K_2$. From Proposition 1, we have $q_{1,t}^*(I)\ge q_{2,t}^*(I)$ for all I. That is to say $I_{e,t}=+\infty$.

Next we will focus on discussing the scenario with $\theta _1<\theta _2$.

To see (i), when both suppliers are all-or-nothing type, the objective function can be written as

$$\begin{aligned} J_t(I,q,q)= & {} (c_1\theta _1+c_2\theta _2)q + \theta _1\theta _2 L_t(I+2q) +(\theta _1+\theta _2-2\theta _1\theta _2)L_t(I+q)\\&+\;(1-\theta _1)(1-\theta _2)(I)= (c_1\theta _1+c_2\theta _2)(x-I) + \theta _1\theta _2 L_t(2x-I)\\&+\;(\theta _1+\theta _2-2\theta _1\theta _2)L_t(x)+(1-\theta _1)(1-\theta _2)(I)\\= & {} (c_1\theta _1+c_2\theta _2)\frac{y-I}{2} + \theta _1\theta _2 L_t(y) +(\theta _1+\theta _2\\&-\;2\theta _1\theta _2)L_t\big (\frac{y+I}{2}\big )+(1-\theta _1)(1-\theta _2)(I) \end{aligned}$$

where $x=I+q$ and $y=I+2q$. From linear combinations and the convexity of $L_t$, we have J(I, q, q) is supermodular in $(I,I+q)$ and submodualar in $(I,I+2q)$. Hence, when both suppliers are all-or-nothing, $I + q_{x,t}^*(I)$ increases in I and $I + 2 q_{x,t}^*(I)$ decreases in I.

We also notice that in the all-or-nothing case

$$\begin{aligned}&f_t(I,q)=(\theta _2-\theta _1)L_t^{\prime }(I+2q)+ ((1-\theta _2)-(1-\theta _1))L_t^{\prime }(I+q)\\&\,\,\,\, =(\theta _2-\theta _1)(L_t^{\prime }(I+2q)-L_t^{\prime }(I+q)) \end{aligned}$$

From facts that $I + q_{x,t}^*(I)$ increases in I and $I + 2 q_{x,t}^*(I)$ decreases in I, we have $f_t(I,q_{x,t}^*(I))$ decreases in I. Combining with Theorem 4, we conclude the proof.

To see (ii), firstly we always pick the minimizers such that $q_{j,t}^*\le {\bar{k}}$ for $j=1,2,x$. From Theorem 2 and Theorem 3, let ${\hat{I}} =\max \{I: q_{1,t}^*(I)={\bar{k}} \hbox { or } q_{2,t}^*(I)={\bar{k}} \}$.

When $I\ge {\hat{I}}$, we still have

$$\begin{aligned} J_t(I,q,q)= & {} (c_1\theta _1+c_2\theta _2)q + \theta _1\theta _2 L_t(I+2q) +(\theta _1+\theta _2-2\theta _1\theta _2)L_t(I+q)\\&+\;(1-\theta _1)(1-\theta _2)(I) \end{aligned}$$

Following a similar argument to that in Part (i), we have $f_t(I,q_{x,t}^*(I))$ decreasing in I when $I\ge {\hat{I}}$. Combining with Theorem 4, we either have $q_{2,t}^*(I)\le q_{1,t}^*(I)$ for all $I\le {\hat{I}}$ or find an $I_{e,t} \in (I_{2,t}, {\hat{I}}_t)$ satisfying that

$$\begin{aligned} q_{1,t}^*(I)\le q_{2,t}^*(I)\,\, \hbox {for}\,\, I<I_{e,t} \,\,\,\,\hbox {and}\,\,\,\, q_{1,t}^*(I)\ge q_{2,t}^*(I)\,\,\hbox {for}\,\, I\ge I_{e,t}. \end{aligned}$$

Next we consider when $I\ge {\hat{k}}$. If it is under the first scenario, then combining the above argument with Theorem 2, we have $q_{2,t}^*(I)\le {\bar{k}}=q_{1,t}^*(I)$ for all $I\ge {\hat{I}}$. If it is under the second scenario, then combining the above argument with Theorem 2, we have $q_{2,t}^*(I)={\bar{k}}\ge q_{1,t}^*(I)$ for all $I\ge {\hat{I}}$. Taken together, we conclude the proof. $\square$

Proof of Proposition 2

To see Part (i), we assume $q_{i,t}^{*}(I)>q_{i,t}^{\delta *}(I)$ and then reach a contradiction. From $q_{i,t}^{*}(I)>q_{i,t}^{\delta *}(I)$, we have ${\bar{K}}_i>q_{i,t}^{\delta *}(I)$ and

$$\begin{aligned} \phi _i(I, q_{1,t}^*(I),q_{2,t}^*(I))\le \phi _i^{\delta }(I, q_{1,t}^{\delta *}(I),q_{2,t}^{\delta *}(I)). \end{aligned}$$

Hence,

$$\begin{aligned}&\mathbf{E}_{K_j} L_t^{\prime }(I + q_{j,t}^*(I)\wedge K_j + q_{i,t}^*(I))- \mathbf{E}_{K_j} L_t^{\delta \prime }(I + q_{j,t}^{\delta *}(I)\wedge K_j + q_{i,t}^{\delta *}(I))\nonumber \\&\quad \le c_i^{\delta }-c_i<0 \end{aligned}$$

(22)

Combining with the convexity of $L_t$, we have $q_{j,t}^{*}(I)< q_{j,t}^{\delta *}(I)$. Therefore,

$$\begin{aligned} \phi _j(I, q_{1,t}^*(I),q_{2,t}^*(I))\ge \phi _j^{\delta }(I, q_{1,t}^{\delta *}(I),q_{2,t}^{\delta *}(I)). \end{aligned}$$

Hence,

$$\begin{aligned}&\mathbf{E}_{K_i} L_t^{\prime }(I + q_{i,t}^*(I)\wedge K_i + q_{j,t}^*(I))- \mathbf{E}_{K_i} L_t^{\delta \prime }(I + q_{i,t}^{\delta *}(I)\wedge K_i + q_{j,t}^{\delta *}(I))\nonumber \\&\quad \ge c_j-c_j=0. \end{aligned}$$

(23)

From $q_{i,t}^{*}(I)>q_{i,t}^{\delta *}(I)$ and $q_{j,t}^{*}(I)<q_{j,t}^{\delta *}(I)$, we have for all the realizations of ${\hat{K}}_j$ and ${\hat{K}}_i$

$$\begin{aligned}&L_t^{\prime }(I + q_{j,t}^*(I)\wedge {\hat{K}}_j + {\hat{K}}_i)\le L_t^{\delta \prime }(I + q_{j,t}^{\delta *}(I)\wedge {\hat{K}}_j + K_i) \end{aligned}$$

(24)

$$\begin{aligned}&L_t^{\prime }(I + q_{i,t}^*(I)\wedge {\hat{K}}_i + {\hat{K}}_j)\ge L_t^{\delta \prime }(I + q_{i,t}^{\delta *}(I)\wedge {\hat{K}}_i + K_j) \end{aligned}$$

(25)

From (22) and (24), we have

$$\begin{aligned}&\mathbf{E}_{K_j,K_i} L_t^{\prime }(I + q_{j,t}^*(I)\wedge {\hat{K}}_j + q_{i,t}^*(I)\wedge {\hat{K}}_i)<\mathbf{E}_{K_j,K_i} L_t^{\delta \prime }(I + q_{j,t}^{\delta *}(I)\wedge K_j \nonumber \\&\quad +\;q_{i,t}^{\delta *}(I)\wedge {\hat{K}}_i). \end{aligned}$$

(26)

From (23) and (25), we have

$$\begin{aligned}&\mathbf{E}_{K_i,K_j} L_t^{\prime }(I + q_{i,t}^*(I)\wedge {\hat{K}}_i+q_{j,t}^*(I)\wedge {\hat{K}}_j)\ge \mathbf{E}_{K_i,K_j} L_t^{\delta \prime }(I + q_{i,t}^{\delta *}(I)\wedge {\hat{K}}_i\nonumber \\&\quad +\; q_{i,t}^{\delta *}(I)\wedge K_j). \end{aligned}$$

(27)

It contradicts with (26). Hence, we have $q_{i,t}^{*}(I)\le q_{i,t}^{\delta *}(I)$.

To see Part (ii), we assume $q_{j,t}^{*}(I)<q_{j,t}^{\delta *}(I)$ and reach a contradiction. We first have

$$\begin{aligned}&\phi _j(I, q_{1,t}^*(I),q_{2,t}^*(I))\ge \phi _j^{\delta }(I, q_{1,t}^{\delta *}(I),q_{2,t}^{\delta *}(I)). \end{aligned}$$

$$\begin{aligned}&\mathbf{E}_{K_i} L_t^{\prime }(I + q_{i,t}^*(I)\wedge K_i + q_{j,t}^*(I))- \mathbf{E}_{K_i} L_t^{\prime }(I + q_{i,t}^{\delta *}(I)\wedge K_i \nonumber \\&\quad +\; q_{j,t}^{\delta *}(I))\ge c_j-c_j=0 \end{aligned}$$

(28)

Together with $q_{j,t}^{*}(I)<q_{j,t}^{\delta *}(I)$, we have $q_{i,t}^*(I)\ge q_{i,t}^{\delta *}(I)$. Together with the result in Part (i), we have $q_{i,t}^*(I)= q_{i,t}^{\delta *}(I)$. Combining with $q_{j,t}^{*}<q_{j,t}^{\delta *}$ and (28), we have

$$\begin{aligned} L_t^{\prime }(I + {\hat{K}}_i + q_{j,t}^*(I))= & {} L_t^{\prime }(I + {\hat{K}}_i + q_{j,t}^{\delta *}(I)), \,\,\,\, \forall {\hat{K}}_i \end{aligned}$$

(29)

$$\begin{aligned} L_t^{\prime }(I + q_{i,t}^*(I) + q_{j,t}^*(I))= & {} L_t^{\prime }(I + q_{i,t}^{\delta *}(I) + q_{j,t}^{\delta *}(I))\end{aligned}$$

(30)

$$\begin{aligned} \phi _{j}^{\delta }(I,q_{1,t}^{\delta *}(I),q_{2,t}^{\delta *}(I))= & {} \phi _{j}^{\delta }(I,q_{1,t}^*(I),q_{2,t}^*(I)). \end{aligned}$$

(31)

If $q_{i,t}^*(I)= q_{i,t}^{\delta *}(I)=0$, then combining it with (29), we have

Combining with (31), we have $(q_{1,t}^*(I),q_{2,t}^*(I))$ is not only the optimal solution of $j_t$ but also the optimal solution of $J_t^{\delta }$. It contradicts with $q_{j,t}^{*}<q_{j,t}^{\delta *}$.

If $q_{i,t}^*(I)= q_{i,t}^{\delta *}(I)>0$, then $q_{i,t}^*(I) + {\hat{K}}_j=q_{i,t}^{\delta *}(I) + {\hat{K}}_j$ for every realization of ${\hat{K}}_j$. Combining with (30), we have

$$\begin{aligned} \mathbf{E}_{K_j} L_t^{\prime }(I + q_{i,t}^*(I) + q_{j,t}^*(I)\wedge K_j)= \mathbf{E}_{K_j} L_t^{\prime }(I + q_{i,t}^{\delta *}(I) + q_{j,t}^{\delta *}(I)\wedge K_j) \end{aligned}$$

Therefore

$$\begin{aligned} \phi _i^{\delta }(I, q_{1,t}^{\delta *}(I), q_{2,t}^{\delta *}(I))= & {} c_i^{\delta }\!+\!\mathbf{E}_{K_j} L_t^{\prime }(I \!+\! q_{i,t}^{\delta *}(I) \!+\! q_{j,t}^{\delta *}(I)\wedge K_j)<c_i \!+\!\mathbf{E}_{K_j} L_t^{\prime }(I + q_{i,t}^{\delta *}(I) \\&+\; q_{j,t}^{\delta *}(I)\wedge K_j)=\phi _i(I, q_{1,t}^{\delta *}(I), q_{2,t}^{\delta *}(I))=0 \end{aligned}$$

It contradicts with $q_{i,t}^{\delta *}(I)>0$. Therefore, we have $q_{j,t}^{*}\ge q_{j,t}^{\delta *}$.

To see Part (iii), we assume $q_{1,t}^*(I)+q_{2,t}^*(I)>q_{1,t}^{\delta *}(I)+q_{2,t}^{\delta *}(I)$ and reach a contradiction in the following discussion. From the result of Part (ii), we have $q_{j,t}^*(I)\ge q_{j,t}^{\delta *}(I)$. If $q_{j,t}^*(I)=q_{j,t}^{\delta *}(I)$, then we obtain that $q_{i,t}^*(I)>q_{i,t}^{\delta *}(I)$ from $q_{1,t}^*(I)+q_{2,t}^*(I)>q_{1,t}^{\delta *}(I)+q_{2,t}^{\delta *}(I)$. It contradicts with the result of Part (i) $q_{i,t}^*(I)\le q_{i,t}^{\delta *}(I)$.

Next, we discuss $q_{j,t}^*(I)>q_{j,t}^{\delta *}(I)$. Hence we have $q_{j,t}^*(I)+{\hat{K}}_i>q_{j,t}^{\delta *}(I)+{\hat{K}}_i$ for every realization ${\hat{K}}_i$. Combining with $q_{1,t}^*(I)+q_{2,t}^*(I)>q_{1,t}^{\delta *}(I)+q_{2,t}^{\delta *}(I)$, we have for every realization ${\hat{K}}_i$,

$$\begin{aligned} L_t^{\prime }(I+q_{j,t}^*(I)+q_{i,t}^*(I)\wedge {\hat{K}}_i)\ge L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)\wedge {\hat{K}}_i) \end{aligned}$$

(32)

From $q_{j,t}^*(I)>q_{j,t}^{\delta *}(I)$, we also have

Combining with the discussion of (32), we have

$$\begin{aligned} L_t^{\prime }(I+q_{j,t}^*(I)+q_{i,t}^*(I))= & {} L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)) \end{aligned}$$

(33)

$$\begin{aligned} L_t^{\prime }(I+q_{j,t}^*(I)+{\hat{K}}_i)= & {} L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ {\hat{K}}_i), \, \, \, \forall {\hat{K}}_i. \end{aligned}$$

(34)

In the following discussion, we consider two different cases.

Case (a), if $c_i+\mathbf{E}_{K_j} L_t^{\prime }(I+q_{j,t}^*(I)\wedge K_j+q_{i,t}^*(I))>0$ then there exists

$$\begin{aligned} 0<\rho _1<(q_{j,t}^*(I)+q_{i,t}^*(I))-(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)) \end{aligned}$$

satisfying that

$$\begin{aligned} \phi _i(I;q_{i,t}^*(I),q_{j,t}^*(I)-\rho )=c_i+\mathbf{E}_{K_j} L_t^{\prime }(I+(q_{j,t}^*(I)-\rho _1)\wedge K_j+q_{i,t}^*(I))\ge 0. \end{aligned}$$

Together with (33) and (34) and the convexity of $L_t$,

Further, we have

$$\begin{aligned}&\mathbf{E}_{K_i} L_t^{\prime }(I+q_{j,t}^*(I)+q_{i,t}^*(I)\wedge K_i) =\mathbf{E}_{K_i} L_t^{\prime }(I+q_{j,t}^*(I)-\rho _1+q_{i,t}^*(I)\wedge K_i)\\&\quad =\mathbf{E}_{K_i} L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)\wedge K_i)\\&\quad \phi _j(I;q_{i,t}^*(I),q_{j,t}^*(I))=\phi _j(I;q_{i,t}^*(I),q_{j,t}^*(I)-\rho _1)=\phi _j^{\delta }(I;,q_{i,t}^{\delta *}(I)q_{j,t}^{\delta *}(I)) \end{aligned}$$

Therefore, $(q_{i,t}^*(I),\, q_{j,t}^*(I)-\rho _1)$ is also the optimal solution of $J_t$. It contradicts with $(q_{i,t}^*(I),q_{j,t}^*(I))$ being the the smallest minimizer.

Case (b), if $c_i+\mathbf{E}_{K_j} L_t^{\prime }(I+q_{j,t}^*(I)\wedge K_j+q_{i,t}^*(I))=0$, then there exist

$$\begin{aligned} 0<\rho _2<(q_{j,t}^*(I)+q_{i,t}^*(I))-(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)) \end{aligned}$$

and $\rho _2>\rho _3>0$ satisfying that

$$\begin{aligned} \mathbf{E}_{K_j} L_t^{\prime }(I+(q_{j,t}^*(I)-\rho _2)\wedge K_j + q_{i,t}^*(I) +\rho _3)= \mathbf{E}_{K_j} L_t^{\prime }(I+q_{j,t}^*(I)\wedge K_j + q_{i,t}^*(I)) \end{aligned}$$

Hence, we have

$$\begin{aligned} \phi _i(I;\, q_{i,t}^*(I)+\rho _3,\, q_{j,t}^*(I)-\rho _2)=0 \end{aligned}$$

From $q_{i,t}^*(I)\le q_{i,t}^{\delta *}(I)$ and the definition of $\rho _2$ and $\rho _3$, we have

Together with (33) and (34) and the convexity of $L_t$,

$$\begin{aligned}&L_t^{\prime }(I+q_{j,t}^*(I)+q_{i,t}^*(I)) =L_t^{\prime }(I+q_{j,t}^*(I)-\rho _2+q_{i,t}^*(I)+\rho _3)\\&\quad =L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I))\\&L_t^{\prime }(I+q_{j,t}^*(I)+{\hat{K}}_i) =L_t^{\prime }(I+q_{j,t}^*(I)-\rho _2+{\hat{K}}_i)=L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ {\hat{K}}_i), \, \, \, \forall {\hat{K}}_i. \end{aligned}$$

Further, we have

$$\begin{aligned}&\mathbf{E}_{K_i} L_t^{\prime }(I+q_{j,t}^*(I)+q_{i,t}^*(I)\wedge K_i) =\mathbf{E}_{K_i} L_t^{\prime }(I+q_{j,t}^*(I)-\rho _2+(q_{i,t}^*(I)+\rho _3)\wedge K_i)\\&\quad =\mathbf{E}_{K_i} L_t^{\prime }(I + q_{j,t}^{\delta *}(I)+ q_{i,t}^{\delta *}(I)\wedge K_i)\\&\quad \phi _j(I;q_{i,t}^*(I),q_{j,t}^*(I))=\phi _j(I;q_{i,t}^*(I)+\rho _3,q_{j,t}^*(I)-\rho _2)=\phi _j^{\delta }(I;,q_{i,t}^{\delta *}(I)q_{j,t}^{\delta *}(I)) \end{aligned}$$

Therefore, $(q_{i,t}^*(I)+\rho _3,\, q_{j,t}^*(I)-\rho _2)$ is also the optimal solution of $J_t$ and $\rho _3-\rho _2<0$. It contradicts with the assumption that $(q_{i,t}^*(I),q_{j,t}^*(I))$ is the minimizer with the smallest total order quantity. $\square$

Proof of Proposition 3

We only need to show that the statement holds for a small enough $\delta$. From $p^{\delta }>p$,

$$\begin{aligned} \mathbf{E}^{\prime }_{D}[H^{\delta }(x-D)]\ge & {} E^{\prime }[H(x-D)]\nonumber \\ L_t^{\delta \prime }(x)\ge & {} L_t^{\prime }(x). \nonumber \\ \phi _{j,t}^{\delta }(I, q_1,q_2)\ge & {} \phi _{i,t}(I, q_1,q_2), \, \, \forall j=1,2. \end{aligned}$$

(35)

Case (i), if $q_{i,t}^{*}(I)=0$ for $i=1,2$, then

$$\begin{aligned} \phi _{i,t}^{\delta }(I,0,0)\ge \phi _{i,t}(I,0,0)\ge 0, \,\, \forall i=1,2. \end{aligned}$$

Hence, $q_{i,t}^{\delta *}(I)=0$ for $i=1,2$.

Case (ii), if $q_{i,t}^*(I)=0$ and $q_{j,t}^*(I)>0$, then

$$\begin{aligned} 0= & {} \phi _{j,t}(I;q_{i,t}^*(I), q_{j,t}^*(I))=c_j+ L_t(I+q_{j,t}^*(I))\nonumber \\ 0\le & {} \phi _{i,t}(I;q_{i,t}^*(I), q_{j,t}^*(I))=c_i+\mathbf{E}_{K_j} L_t(I+q_{j,t}^*(I)\wedge K_j)\nonumber \\= & {} c_i+\mathbf{E}_{K_j}[\min \{-c_j, L^{\prime }(I+K_j)\}] \end{aligned}$$

(36)

From $q_{j,t}^*(I)>0$, then from $\delta$ is a small enough number, there exists $\rho _1\in [0,q_{i,t}^*(I))$ satisfying that

$$\begin{aligned} \phi _{j,t}^{\delta }(I;q_{i,t}^*(I), q_{j,t}^*(I)-\rho _1)=\phi _{j,t}(I;q_{i,t}^*(I), q_{j,t}^*(I))=c_j+ L_t(I+q_{j,t}^*(I))=0. \end{aligned}$$

we also have that

$$\begin{aligned}&\phi _{i,t}^{\delta }(I;q_{i,t}^*(I), q_{j,t}^*(I)-\rho _1)\\&\quad = c_i+\mathbf{E}_{K_j} L_t^{\delta }(I+q_{j,t}^*(I)\wedge K_j)\\&\quad = c_i+ \mathbf{E}_{K_j} [\min (L_t^{\delta }(I+q_{j,t}^*(I)), L_t^{\delta }(I+K_j))]\\&\quad \ge c_i+ \mathbf{E}_{K_j} [\min (-c_j, L_t(I+K_j))]\ge 0 \end{aligned}$$

The first inequality comes from (35). The second inequality comes from (36). Therefore $(0,q_{j,t}^*(I)-\rho _1)$ is the optimal solution for $J_t^{\delta }$.

Case (iii), if $q_{i,t}^*(I)>0$ for $i=1,2$, then since $\delta$ is a small enough number, there exist $\rho _2\in [0,q_{1,t}^*(I))$ and $\rho _3\in [0,q_{2,t}^*(I))$ satisfying that

$$\begin{aligned}&\phi _{1,t}^{\delta }(I, q_{1,t}^*(I)-\rho _2, q_{2,t}^*(I)-\rho _3)\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =c_1 + \mathbf{E}_{K_2} [\min (L_t^{\delta }(I+q_{1,t}^*(I)-\rho _2+q_{2,t}^*(I)-\rho _3), L_t^{\delta }(I+q_{1,t}^*(I)-\rho _2+K_2))]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =c_1 + \mathbf{E}_{K_2} [\min (L_t(I+q_{1,t}^*(I)+q_{2,t}^*(I)), L_t(I+q_{1,t}^*(I)+K_2))]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =\phi _{1,t}(I, q_{1,t}^*(I), q_{2,t}^*(I))\\&\phi _{2,t}^{\delta }(I, q_{1,t}^*(I)-\rho _2, q_{2,t}^*(I)-\rho _3)\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =c_2 + \mathbf{E}_{K_1} [\min (L_t^{\delta }(I+q_{1,t}^*(I)-\rho _2+q_{2,t}^*(I)-\rho _3), L_t^{\delta }(I+q_{2,t}^*(I)-\rho _3+K_1))]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =c_2 + \mathbf{E}_{K_1} [\min (L_t(I+q_{1,t}^*(I)+q_{2,t}^*(I)), L_t(I+q_{2,t}^*(I)+K_1))]\\&\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =\phi _{2,t}(I, q_{1,t}^*(I), q_{2,t}^*(I)) \end{aligned}$$

Therefore, $(q_{1,t}^*(I)-\rho _2, q_{2,t}^*(I)-\rho _3)$ is the optimal solution for $J_t^{\delta }$. We conclude the proof. $\square$

Proof of Theorem 6

We will use mathematical induction to obtain the result.

First, we will show that for $V_{T}^{\prime }(I)\le V_{T+1}^{\prime }(I)$ almost everywhere and $q_{1,T}^*(0)>0$. Recall that $V_{T+1}(I)=c_1 I^{-} + c_e I^+$ where $c_e >h/(1-\alpha )$.

$$\begin{aligned}&\phi _{1,T}(I_{1,T},0,0)=c_1+ \mathbf{E}^{\prime }_{D}[H(I_{1,t}-D)] + \alpha \mathbf{E}^{\prime }_{D}[V_{T+1} (I_{1,t}-D)]=0\\&\phi _{1,T}(I_{1,T},0,0)=c_1+ \mathbf{E}^{\prime }_{D}[H(-D)] + \mathbf{E}^{\prime }_{D}[V_{T+1} (-D)]=c_1-p-\alpha c_1<0 \end{aligned}$$

Hence, $I_{1,t}>0$ and $q_{1,T}^*(0)>0$.

Therefore, when $I\le I_{1,t}$, combining it with Theorem 7, we have

$$\begin{aligned} V_t^{\prime }(I)\le V_t^{\prime }(I_{1,t})= -c_e \le V_{T+1}^{\prime }(I), \,\, a.s. \end{aligned}$$

The inequality comes from that $V_{T+1}(I)=c_1 I^{-} + c_e I^+$. Hence, above inequality holds almost everywhere. When $I\ge I_{1,t}$, we have $I>0$ and

$$\begin{aligned} V_t^{\prime }(I)=\mathbf{E}^{\prime }_{D}[H(I-D)] + \alpha \mathbf{E}^{\prime }_{D}[ V_{T+1} (I-D)] \le h + \alpha c_e \le c_e = V^{\prime }(I) \end{aligned}$$

The first inequality comes from the expression of H and $V_{T+1}$. The second inequality comes from $c_e> h/(1-\alpha )$. From the above discussion, we have $V_{T}^{\prime }(I)\le V_{T+1}^{\prime }(I)$ all most everywhere and $q_{1,T}^*(0)>0$.

Next we will show that if $V_{t+1}^{\prime }(I)\le V_{t+2}^{\prime }(I)$ almost everywhere and $q_{1,t+1}^*(0)>0$, then $V_{t}^{\prime }(I)\le V_{t+1}^{\prime }(I)$, $q_{1,t}^*(0)>0$ and $q_{i,t}^*(I)\ge q_{t+1}^*(I)$.

From $V_{t+1}^{\prime }(I)\le V_{t+2}^{\prime }(I)$ almost everywhere, we have

$$\begin{aligned} L_t^{\prime }(I)\le L_{t+1}^{\prime }(I), a.s. \end{aligned}$$

(37)

Comparing the first order conditions at time t and $t+1$, we have $i=1,2$ and $j\ne i$,

$$\begin{aligned} \phi _{i,t}(I,q_1, q_2)= & {} c_i + \mathbf{E}_{K_j}[L_t^{\prime }(I+q_j\wedge K_j +q_i)] \hbox { and } \phi _{i,t}(I,q_1, q_2)\nonumber \\= & {} c_i + \mathbf{E}_{K_j}[L_{t+1}^{\prime }(I+q_j\wedge K_j +q_i)] \end{aligned}$$

From (37) and following a similar argument as Proposition , we can compare the two equation systems and obtain $q_{i,t}^*(I)\ge q_{i,t+1}^*(I)$ for $i=1,2$. Thus $q_{1,t}^*(0)\ge q_{1,t+1}^*(0)>0$. Next we will show that $V_{t}^{\prime }(I)\le V_{t+1}^{\prime }(I)$. From $q_{i,t}^*(I)\ge q_{i,t+1}^*(I)$ for $i=1,2$, we have

From (37), we also have

$$\begin{aligned} \mathbf{E}_{K_1,K_2}[L_t^{\prime }(I+K_1 +K_2)]\le \mathbf{E}_{K_1,K_2}[L_{t+1}^{\prime }(I+K_1 +K_2)] \end{aligned}$$

Thus,

$$\begin{aligned}&\mathbf{E}_{K_1,K_2}[L_t^{\prime }(I+q_{1,t}^{*}(I)\wedge K_1 +q_{2,t}^{*}(I))\wedge K_2]\nonumber \\&\quad \le \mathbf{E}_{K_1,K_2}[L_{t+1}^{\prime }(I+q_{1,t+1}^{*}(I)\wedge K_1 +q_{2,t+1}^{*}(I)\wedge K_2)] \end{aligned}$$

Therefore,

$$\begin{aligned} V_t^{\prime }(I)= & {} \mathbf{E}_{K_1,K_2}[L_t^{\prime }(I+q_{1,t}^{*}(I)\wedge K_1 +q_{2,t}^{*}(I)\wedge K_2]\nonumber \\&\le \mathbf{E}_{K_1,K_2}[L_{t+1}^{\prime }(I+q_{1,t+1}^{*}(I)\wedge K_1 +q_{2,t+1}^{*}(I)\wedge K_2)]=V_{t+1}^{\prime }(I). \end{aligned}$$

We conclude the proof by the mathematical induction. $\square$