On how to allocate the fixed cost of transport systems

Abstract

In this study, we consider different cities located along a tram line. Each city may have one or several stations and information is available about the flow of passengers between any pair of stations. A fixed cost (salaries of the executive staff, repair facilities, or fixed taxes) must be divided among the cities. This cost is independent of the number of passengers and the length of the line. We propose a mathematical model to identify suitable mechanisms for sharing the fixed cost. In the proposed model, we study, and characterize axiomatically, three rules, which include the uniform split, the proportional allocation and an intermediate situation. The analyzed axioms represent the basic requirements for fairness and elemental properties of stability.

Appendix A. Logical independence of the properties

In this section we show that all of the properties used in the characterization of each solution are necessary.

Proposition 2

Symmetry, and additivity are necessary to characterize the uniform rule.

Proof

• (a) Let us consider the station-based uniform rule given by:

  $$\begin{aligned} SU_i (a) =\frac{C}{n} \cdot |S_i| . \end{aligned}$$

  It is clear that this rule does not satisfy symmetry because it depends only on the number of stations in each municipality but not on the traffic through the network. It is easy to check that this rule satisfies additivity.

• (b) The station-based proportional rule satisfies symmetry, but not additivity because it depends on the flow matrix.

$\square$

Proposition 3

Symmetry, bilateral ratio consistency, and weighted additivity are necessary to characterize the uniform rule.

Proof

• (a) Let us consider the station-based uniform rule given by:

  $$\begin{aligned} SU_i (a) =\frac{C}{n} \cdot |S_i| . \end{aligned}$$

  As stated above, it does not satisfy symmetry. It is easy to check that this rule satisfies bilateral ratio consistency and weighted additivity.

• (b) The station-based proportional rule satisfies symmetry and weighted additivity but not bilateral ratio consistency. We consider the case of a trolley line that passes across three municipalities $M=\{1,2,3\}$ with four stations $S=\{s_1,s_2,s_3,s_4\}$, which are distributed as follows:

  

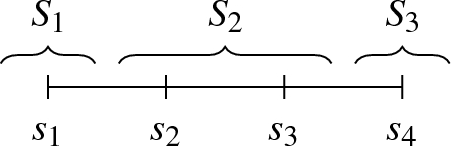
  The fixed cost is $C=4$ and the OD matrix is

  $$\begin{aligned} { OD}= \left( \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} 0 &{} 2 &{} 3 &{} 1 \\ 2 &{} 0 &{} 4 &{} 0 \\ 0 &{} 0 &{} 0 &{} 2 \\ 0 &{} 2 &{} 0 &{} 0 \\ \end{array}\right) . \end{aligned}$$

  In this case, $SP(a)= \left( 1,\frac{19}{8},\frac{5}{8} \right)$ Now, if we suppose that Municipality 3 leaves the consortium, then the new (reduced) problem is: $a_{\{1,2\}}=\left( \{1,2\},S_1 \cup S_2,{ OD}_{\{1,2\}},C\right)$, where

  $$\begin{aligned} { OD}_{\{1,2\}} = \left( \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} 0 &{} 2 &{} 3 \\ 2 &{} 0 &{} 4 \\ 0 &{} 0 &{} 0 \\ \end{array}\right) , \end{aligned}$$

  For this reduced problem, we obtain:

  $$\begin{aligned} SP(a_{\{1,2\}}) = \left( \frac{14}{11} , \frac{30}{11} \right) . \end{aligned}$$

  Now we have:

  $$\begin{aligned} \frac{1}{\frac{19}{8}} \ne \frac{\frac{14}{11}}{\frac{30}{11}}. \end{aligned}$$

  Therefore, the station-based proportional rule does not satisfy bilateral ratio consistency.

• (c) Example of a rule that satisfies symmetry, bilateral ratio consistency but does not satisfy weighted additivity. Let $a=(M,S,{ OD},C) \in {\mathbb {A}}$ be a problem. We define the following rule for each $i\in M$:

  $$\begin{aligned} R_i(a)={\left\{ \begin{array}{ll} \frac{C}{\sum _{j\in M} \Omega _{jj}(OD)} \cdot \Omega _{ii}(OD) &{} \text {if } \Omega _{kk}(OD) \ne 0 \text { for all } k\in M \\ U_i(a) &{} \text {otherwise}. \end{array}\right. } \end{aligned}$$

  By definition, this rule satisfies symmetry and bilateral ratio consistency. However, it does not satisfy weighted additivity because we can transform a problem with $\Omega _{kk}(OD) \ne 0 \text { for all } k\in M$ into problems where this condition does not hold, and we can then apply the uniform rule instead of the proportional distribution to the inner traffic in each municipality.

$\square$

Proposition 4

Null municipality, symmetry and weighted additivity are necessary in the characterization of the station-based proportional rule.

Proof

• (a) The uniform rule satisfies symmetry and weighted additivity but it does not satisfy null municipality by definition, because all municipalities are allocated with part of the fixed cost independently of the traffic in the transport system.

• (b) Let $a=(M,S,{ OD},C) \in {\mathbb {A}}$ be a problem and for each $i\in M$ we define the following rule:

  $$\begin{aligned} R_i (a) ={\left\{ \begin{array}{ll} 0 &{} \text {if } \Omega _i (\textit{OD})=0\\ \frac{C}{|K|} &{} \text {otherwise} \end{array}\right. }, \end{aligned}$$

  where $K = \{i \in M: \Omega _i (OD) \ne 0\}$.

  By definition, this rule satisfies null municipality and symmetry, but not weighted additivity. Indeed, let us consider the case of a trolley line that passes across three municipalities $M=\{1,2,3\}$ with three stations $S=\{s_1,s_2,s_3\}$, which are distributed as follows: $S_1=\{s_1\}, S_2=\{s_2\} \text { and } S_3=\{s_3\}$. The fixed cost is $C=6$ and the OD matrix is given by

  $$\begin{aligned} OD = \left( \begin{array}{c@{\quad }c@{\quad }c} 0 &{} 1 &{} 1 \\ 1 &{} 0 &{} 1 \\ 1 &{} 1 &{} 0 \\ \end{array}\right) , \end{aligned}$$

  and

  i	$\Omega ^+_i({ OD})$	$\Omega ^-_i({ OD})$	$\Omega _i({ OD})$
  1	2	2	4
  2	2	2	4
  3	2	2	4

  Therefore, all of the municipalities are symmetric so they must pay the same $\frac{C}{3}$, and thus:

  $$\begin{aligned} R(a) = \left( 2 , 2, 2 \right) . \end{aligned}$$

  Now we divide the cost C into $C_1+C_2=2+4=6$ and the OD matrix into $OD_1+OD_2$ in the following manner:

  $$\begin{aligned} { OD}= \left( \begin{array}{c@{\quad }c@{\quad }c} 0 &{} 1 &{} 1 \\ 1 &{} 0 &{} 1 \\ 1 &{} 1 &{} 0 \\ \end{array}\right) =\left( \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 1 \\ 0 &{} 1 &{} 0 \\ \end{array}\right) +\left( \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} 0 &{} 1 &{} 1 \\ 1 &{} 0 &{} 0 \\ 1 &{} 0 &{} 0 \\ \end{array}\right) . \end{aligned}$$

  For example, for Municipality 1, we obtain:

  $$\begin{aligned} \frac{6}{6} \cdot 2 \ne \frac{2}{2} \cdot 0 + \frac{4}{4} \cdot \frac{4}{3}. \end{aligned}$$

  Therefore, this rule does not satisfy weighted additivity.

• (c) Let $a=(M,S,{ OD},C) \in {\mathbb {A}}$ be a problem. For each $i\in M$, we define the following rule:

  $$\begin{aligned} R_i (a) ={\left\{ \begin{array}{ll} \frac{i}{3} \cdot C &{} \text {if } |M|=2 \text { and } |S|=2\\ SP_i(a) &{} \text {otherwise}. \end{array}\right. } \end{aligned}$$

  For $|M|=2 \text { and } |S|=2$, it is clear that this rule satisfies weighted additivity because it does not depend on the OD matrix. Furthermore, in this case, null municipality is meaningless because it implies that there is no traffic at all in the network. Finally, in this case, this rule does not satisfy symmetry because the allocation of the fixed cost depends on the names of the agents. For the remaining cases, this rule satisfies null municipality, symmetry and weighted additivity. Therefore, this rule satisfies null municipality, weighted additivity but no symmetry.

$\square$

Proposition 5

Adjacent symmetry, weak null municipality, trip decomposition, and weighted additivity are necessary to characterize the track-based proportional rule.

Proof

• (a) By the definition of the uniform rule, it is straightforward to check whether it satisfies adjacent symmetry, trip decomposition, and weighted additivity but not weak null municipality.

• (b) Before giving a rule that satisfies weak null municipality, trip decomposition, and weighted additivity but not adjacent symmetry, we introduce the following

  $$\begin{aligned} \omega _{[g,g+1]}=\sum _{\begin{array}{c} k,h \\ k \le g<g+1 \le h \end{array}} \frac{\omega _{kh} + \omega _{hk}}{|h - k|}, \end{aligned}$$

  where $\omega _{[g,g+1]}$ is the number of passengers between two consecutive stations when all passengers are distributed equally among all tracks that they use in their trips. Now, we define the following rule:

  $$\begin{aligned} R_i (a) = \frac{C}{\Omega (OD)} \sum _{s_g \in S_i} \left( \frac{g}{2g-1} \omega _{[g-1,g]} + \frac{g}{2g+1} \omega _{[g,g+1]} \right) , \text { for all } i \in M, \end{aligned}$$

  where $\omega _{[0,1]}=\omega _{[n,n+1]}=0$.

  By definition, this rule satisfies weak null municipality and trip decomposition. Analogous to the track-based proportional rule, we can prove that this rule satisfies weighted additivity. However, this rule does not satisfies adjacent symmetry because it depends on the name of the stations.

• (c) It is easy to check that the station-based proportional rule satisfies weak null municipality, adjacent symmetry, and weighted additivity. However, it does not satisfy trip decomposition as shown by the following example. Let us consider a problem with two municipalities and three stations, $S_{1}=\{s_1\}$ and $S_{2}=\{s_2,s_3\}$, where the fixed cost that needs to be distributed is 1 and the OD matrix is given by

  $$\begin{aligned} OD = \left( \begin{array}{c@{\quad }c@{\quad }c} 0 &{} 1 &{} 1 \\ 1 &{} 0 &{} 1 \\ 1 &{} 1 &{} 0 \\ \end{array}\right) . \end{aligned}$$

  The station-based proportional rule is $SP(a) =\left( \frac{1}{3},\frac{2}{3}\right)$. Now, if we distribute the passengers such that there are only trips between consecutive stations, we obtain the following $OD'$ matrix:

  $$\begin{aligned} OD' = \left( \begin{array}{c@{\quad }c@{\quad }c} 0 &{} 1\frac{1}{2} &{} 0 \\ 1\frac{1}{2} &{} 0 &{} 1\frac{1}{2} \\ 0 &{} 1\frac{1}{2} &{} 0 \\ \end{array}\right) , \end{aligned}$$

  and the station-based proportional rule is $SP(a')=\left( \frac{1}{4},\frac{3}{4}\right)$. Therefore, the station-based proportional rule does not satisfies trip decomposition.

• (d) Given an OD matrix, we define the following [OD] matrix:

  $$\begin{aligned} {[}\omega _{gh}]={\left\{ \begin{array}{ll} 0 &{} \text {if } |g - h| >1\\ \omega _{[g,h]} &{} \text {otherwise}, \end{array}\right. } \end{aligned}$$

  where $\omega _{[g,h]}$ is defined as in (b).

  Now, we define the following rule for each $i \in M$:

  $$\begin{aligned} R_i (a) ={\left\{ \begin{array}{ll} 0 &{} \text {if } \Omega _i ([OD])=0\\ \frac{C}{|K|} &{} \text {otherwise} \end{array}\right. } \end{aligned}$$

  where $K = \{i \in M: \Omega _i ([OD]) \ne 0\}$.

  By definition, we can prove that this rule satisfies weak null municipality, adjacent symmetry, and trip decomposition but not weighted additivity.

$\square$