Disclosure of quality preference-revealing information in a supply chain with competitive products

Abstract

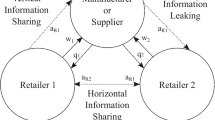
We examine price setting and the decision to disclose quality preference-revealing information in a supply chain with two competing manufacturers supplying two quality-differentiated products to a common retailer. Consumers have complete knowledge of product quality but are uncertain about how the quality will match their own preferences. We study who should provide preference-revealing information to help consumers understand their own quality preferences, and how such information disclosure affects horizontal and vertical competitions in the supply chain. We show that the manufacturer with a higher unit quality production cost has a higher incentive to provide such information, and we show how each supply chain member sets its information policy. The role of information releaser will switch from an upstream member (a manufacturer) to the downstream member (the retailer) as the market information level (the consumer’s degree of informativeness before disclosure) increases. Information disclosure softens both horizontal and vertical competitions in the supply chain. We extend our model to examine the case in which the two manufacturers make simultaneous decisions, and the case when a supply chain member incurs a cost for implementing information disclosure.

Appendix

Proof of Lemma 1

With Eq. (1), the consumer will purchase Product 1 if and only if $U_{1}^{S} \ge 0$ (which requires $\theta \ge \theta_{1}^{S} = \frac{{p_{1}^{S} }}{{q_{1} I}} - \frac{1 - I}{{2I}}$) and $U_{{1}}^{S} \ge U_{2}^{S}$ (which requires $\theta \ge \theta_{21}^{S} = \frac{{p_{1}^{S} - p_{2}^{S} }}{{(q_{1} - q_{2} )I}} - \frac{1 - I}{{2I}}$). Similarly, this consumer will purchase Product 2 if and only if $U_{2}^{S} \ge 0$ (which requires $\theta \ge \theta_{{2}}^{S} = \frac{{p_{{2}}^{S} }}{{q_{2} I}} - \frac{1 - I}{{2I}}$) and $U_{{1}}^{S} < U_{2}^{S}$ (which requires $\theta < \theta_{21}^{S} = \frac{{p_{1}^{S} - p_{2}^{S} }}{{(q_{1} - q_{2} )I}} - \frac{1 - I}{{2I}}$). With $q_{1} > q_{2}$, $p_{1}^{S} > p_{2}^{S}$ is necessary to have a positive $\theta_{{2{1}}}^{S}$.

When $\theta_{{2{1}}}^{S} > 1$ (equivalently $\frac{{p_{1}^{S} - p_{2}^{S} }}{{q_{1} - q_{2} }} > \frac{1 + I}{2}$), no consumer will buy Product 1 ($d_{{1}}^{S} = 0$) and consumers whose quality preference valuations are in the interval $[\theta_{2}^{S} ,1]$ will buy Product 2 ($d_{2}^{S} = 1 - \theta_{2}^{S}$). When $\theta_{2}^{S} > \theta_{1}^{S}$ (equivalently $\frac{{p_{2}^{S} }}{{q_{2} }} > \frac{{p_{1}^{S} }}{{q_{1} }}$), no consumer will buy Product 2 ($d_{2}^{S} = 0$) and consumers whose quality preference valuations are in the interval $[\theta_{1}^{S} ,1]$ will buy Product 1 ($d_{1}^{S} = 1 - \theta_{1}^{S}$). This implies that Product 1 can have a market share if and only if $\theta_{{2{1}}}^{S} \le 1$, while Product 2 can have a market share if and only if $\theta_{2}^{S} \le \theta_{1}^{S}$. With the above analysis, we obtain the pairwise co-existence conditions of the two products under the supply chain’s two information disclosure strategies, and we can show that two products can co-exist if and only if ${0} \le \theta_{2}^{S} \le \theta_{1}^{S} \le \theta_{21}^{S} \le 1$, for$\text{S }={\text{D}}, \text{U}$.

Manufacturer 2 maximizes its profit $\pi_{{m_{2} }}^{SB} (p_{2}^{SB} ) = (p_{2}^{SB} - c_{2} )d_{2}^{SB}$ by setting $p_{2}^{SB}$. For given $p_{1}^{SB}$, taking the derivatives of $\pi_{m2}^{SB}$ w.r.t. $p_{2}^{SB}$, we have $\frac{{\partial \pi_{{m_{2} }}^{SB} }}{{\partial p_{2}^{SB} }} = \frac{{p_{1}^{SB} - 2p_{2}^{SB} + c_{2} }}{{q_{1} - q_{2} }} + \frac{{c_{2} - 2p_{2}^{SB} }}{{q_{2} }}$. Then we can obtain $\frac{{\partial^{2} \pi^{SB}_{{m_{2} }} }}{{\partial p^{SB2}_{2} }} = - \left( {\frac{2}{{q_{1} - q_{2} }} + \frac{2}{{q_{2} }}} \right) < 0$. We conclude that there exists a unique $p_{2}^{SB}$, which is given by $p_{2}^{SB} = \frac{{q_{2} \hat{p}_{1}^{S} + q_{1} c_{2} }}{{2q_{1} }}$. The demand of Product 1 is $d_{1}^{SB} = 1 - \frac{{(2q_{1} - q_{2} )p_{1}^{SB} - q_{1} c_{2} }}{{2q_{1} (q_{1} - q_{2} )}}$. Manufacturer 1 sets $p_{1}^{SB}$ to maximize its profit $\pi_{{m_{1} }}^{SB} (p_{1}^{SB} ) = (p_{1}^{SB} - c_{1} )d_{1}^{SB}$, and we have $\frac{{\partial \pi_{{m_{1} }}^{SB} }}{{\partial p_{1}^{SB} }} = 1 + \frac{{q_{1} c_{2} + (2q_{1} - q_{2} )c_{1} - 2(2q_{1} - q_{2} )p_{1}^{SB} }}{{2q_{1} (q_{1} - q_{2} )}}$ and $\frac{{\partial^{2} \pi^{SB}_{{m_{1} }} }}{{\partial p^{SB2}_{1} }} = \frac{{ - 2q_{1} + q_{2} }}{{q_{1} (q_{1} - q_{2} )}} < 0$.

Therefore, there exists a unique solution $p_{1}^{SB*} = \frac{{(1 + I)q_{1} }}{2} - \frac{{\Delta_{1}^{S} }}{2} - \frac{{q_{1} \Delta_{2}^{S} }}{{2(2q_{1} - q_{2} )}}$. We can derive $p_{2}^{SB * } = \frac{{(1 + I)q_{2} }}{2} - \frac{{q_{2} \Delta_{1}^{S} }}{{4q_{1} }} - \frac{{(4q_{1} - q_{2} )\Delta_{2}^{S} }}{{4(2q_{1} - q_{2} )}}$ and ($d_{1}^{SB*}$, $d_{2}^{SB*}$, $\pi_{{m_{1} }}^{SB*}$, $\pi_{{m_{2} }}^{SB*}$) as in Table 1.

Proof of Lemma 2

Manufacturer 2 has an incentive to disclose information when $\Delta \pi_{{m_{2} }}^{B} = \pi_{{m_{2} }}^{DB} - \pi_{{m_{2} }}^{NB} \ge 0$. Then we can derive that when $\gamma \ge \gamma_{2} = \left( {\frac{1}{2} - E_{2} } \right)^{2} \left( {\frac{{(4q_{1} - 3q_{2} )(q_{2} - q_{1} \underline {\rho } \phi )}}{{q_{2} (q_{1} - q_{2} )}}} \right)^{2}$, then $\Delta \pi_{{m_{2} }}^{B} \ge 0$. Manufacturer 1 has an incentive to disclose information when $\Delta \pi_{{m_{1} }}^{B} = \pi_{{m_{1} }}^{DB} - \pi_{{m_{1} }}^{NB} \ge 0$. Then we can derive that when $\gamma \ge \gamma_{1} = \left( {\frac{1}{2} - E_{2} } \right)^{2} \left( {\frac{{q_{1} \overline{\rho }\phi - q_{2} }}{{q_{1} - q_{2} }}} \right)^{{2}}$, then $\Delta \pi_{{m_{1} }}^{B} \ge 0$.

Manufacturer 2 decides its information policy ($y_{2} = \{ P,N\}$), we can conclude that Manufacturer 2 will choose the provision policy ($y_{2} = P$) when $\gamma \ge \gamma_{2}$, and the no-provision policy ($y_{2} = N$), otherwise. Then we consider Manufacturer 1′s information policy ($y_{1} = \{ P,N\}$). With $\gamma_{2} \le \gamma_{1}$ if $E_{1} \le E_{2}$, and $\gamma_{1} < \gamma_{2}$ if $E_{1} > E_{2}$, Manufacturer 1 will choose the information provision policy ($y_{1} = P$) when $E_{1} > E_{2}$ and $\gamma_{1} \le \gamma < \gamma_{{2}}$, and choose the no-provision policy ($y_{1} = N$), otherwise.

Proof of Proposition 1

For given $w_{{1}}^{S}$ and $w_{{2}}^{S}$, taking the derivatives of $\pi_{r}^{S}$ w.r.t. $p_{{1}}^{S}$ and $p_{{2}}^{S}$: $\frac{{\partial \pi_{r}^{S} }}{{\partial p_{{1}}^{S} }} = \frac{1 + I}{{2I}} + \frac{{ - 2p_{{1}}^{S} + 2p_{2}^{S} + w_{{1}}^{S} - w_{2}^{S} }}{{(q_{{1}} - q_{2} )I}}$ and $\frac{{\partial \pi_{r}^{S} }}{{\partial p_{2}^{S} }} = \frac{{2p_{{1}}^{S} - 2p_{2}^{S} - w_{{1}}^{S} + w_{2}^{S} }}{{(q_{{1}} - q_{2} )I}} + \frac{{ - 2p_{2}^{S} + w_{2}^{S} }}{{q_{2} I}}$. We have $\frac{{\partial^{2} \pi_{r}^{S} }}{{\partial p_{1}^{S2} }} = - \frac{2}{{(q_{1} - q_{2} )I}} < 0$, $\frac{{\partial^{2} \pi^{S}_{r} }}{{\partial p^{S2}_{2} }} = - \frac{{2q_{1} }}{{q_{2} (q_{1} - q_{2} )I}} < 0$, and $\frac{{\partial^{2} \pi^{S}_{r} }}{{\partial p^{S2}_{1} }}\frac{{\partial^{2} \pi^{S}_{r} }}{{\partial p^{S2}_{2} }} - \frac{{\partial^{2} \pi^{S}_{r} }}{{\partial p^{S}_{1} \partial p^{S}_{2} }}\frac{{\partial^{2} \pi^{S}_{r} }}{{\partial p^{S}_{2} \partial p^{S}_{1} }} = \frac{4}{{(q_{1} - q_{2} )q_{2} I^{2} }} > 0$. This suggests that there exist unique optimal solutions to ($p_{{1}}^{S}$, $p_{{2}}^{S}$), which are given by:

$$ p_{{1}}^{S} = \frac{{(1 + I)q_{{1}} + 2w_{{1}}^{S} }}{4}\;{\text{and}}\;p_{{2}}^{S} = \frac{{(1 + I)q_{2} + 2w_{{2}}^{S} }}{4}. $$

(9)

Manufacturer 2 maximizes its profit $\pi_{{m_{2} }}^{S}$ by setting $w_{{2}}^{S}$. With $p_{{1}}^{S}$ and $p_{{2}}^{S}$ in (9), the demand of Product 2 is $d_{2}^{S} = \frac{{q_{2} w_{{1}}^{S} - q_{1} w_{{2}}^{S} }}{{{2}q_{2} (q_{{1}} - q_{2} )I}}$. Then the profit is $\pi_{{m_{2} }}^{S} = (w_{{2}}^{S} - c_{2} )(\frac{{q_{2} w_{{1}}^{S} - q_{1} w_{{2}}^{S} }}{{{2}q_{2} (q_{{1}} - q_{2} )I}})$. For a given $w_{{1}}^{S}$, then $\frac{{\partial \pi_{{m_{2} }}^{S} }}{{\partial w_{{2}}^{S} }} = \frac{{w_{{1}}^{S} - {2}w_{{2}}^{S} + c_{2} }}{{{2}(q_{{1}} - q_{2} )I}} + \frac{{ - {2}w_{{2}}^{S} + c_{2} }}{{{2}q_{2} I}}$. We thus can obtain $\frac{{\partial^{2} \pi^{S}_{{m_{2} }} }}{{\partial w^{S2}_{2} }} = - \frac{1}{{(q_{1} - q_{2} )I}} - \frac{1}{{q_{2} I}} < 0$. We conclude that there exists a unique optimal $w_{{2}}^{S}$, which is given by:

$$ w_{{2}}^{S} = \frac{{w_{{1}}^{S} q_{2} + q_{{1}} c_{2} }}{{2q_{{1}} }}. $$

(10)

The demand of Product 1 is $d_{1}^{S} = \frac{1 + I}{{4I}} + \frac{{ - 2w_{{1}}^{S} q_{{1}} + w_{{1}}^{S} q_{2} + q_{{1}} c_{2} }}{{{4}(q_{{1}} - q_{2} )q_{{1}} I}}$. Manufacturer 1′s profit is $\pi_{{m_{1} }}^{S} = (w_{{1}}^{S} - c_{1} )(\frac{1 + I}{{4I}} + \frac{{ - 2w_{{1}}^{S} q_{{1}} + w_{{1}}^{S} q_{2} + q_{{1}} c_{2} }}{{{4}(q_{{1}} - q_{2} )q_{{1}} I}})$. Then we have.

$\frac{{\partial \pi_{{m_{{1}} }}^{S} }}{{\partial w_{{1}}^{S} }} = \frac{1 + I}{{4I}} + \frac{{ - 4w_{{1}}^{S} q_{{1}} + 2w_{{1}}^{S} q_{2} + q_{{1}} c_{2} + 2c_{{1}} q_{{1}} - c_{{1}} q_{2} }}{{{4}(q_{{1}} - q_{2} )q_{1} I}}$.$\frac{{\partial^{2} \pi^{S}_{{m_{1} }} }}{{\partial w^{S2}_{1} }} = \frac{{ - 2q_{1} + q_{2} }}{{2(q_{1} - q_{2} )q_{1} I}} < 0$ implies that there exists a unique optimal solution $w_{{1}}^{S*} = \frac{{(1 + I)q_{1} }}{2} - \frac{{\Delta_{1}^{S} }}{{2}} - \frac{{q_{{1}} \Delta_{2}^{S} }}{{{2}(2q_{{1}} - q_{2} )}}$. Substituting $w_{{1}}^{S*}$ into Eq. (10), we can derive $w_{{2}}^{S*} = \frac{{(1 + I)q_{2} }}{2} - \frac{{q_{2} \Delta_{1}^{S} }}{{4q_{{1}} }} - \frac{{(4q_{{1}} - q_{2} )\Delta_{2}^{S} }}{{4(2q_{{1}} - q_{2} )}}$. Then substituting $w_{{1}}^{S*}$ and $w_{{2}}^{S*}$ into Eq. (9), we derive ($p_{{1}}^{S * }$,$p_{{2}}^{S*}$) for Proposition 1 and we can obtain $d_{1}^{S*}$, $d_{2}^{S*}$, $\pi_{{m_{1} }}^{S*}$, $\pi_{{m_{2} }}^{S*}$, and $\pi_{r}^{S*}$ as summarized in Table 2.

Proof of Lemma 3

By comparing prices and demands with the supply chain’s non-disclosure strategy to those with a disclosure strategy, we can derive:

$w_{{1}}^{{D{*}}} - w_{{1}}^{{U{*}}} = \frac{{(1 - \gamma )q_{{1}} (q_{{1}} - q_{2} )}}{{2(2q_{{1}} - q_{2} )}} > 0$, $w_{2}^{{D{*}}} - w_{2}^{{U{*}}} = \frac{{(1 - \gamma )q_{2} (q_{{1}} - q_{2} )}}{{4(2q_{{1}} - q_{2} )}} > 0$,

$p_{{1}}^{{D{*}}} - p_{{1}}^{{U{*}}} = \frac{{(1 - \gamma )q_{1} (3q_{1} - 2q_{2} )}}{{{4}(2q_{1} - q_{2} )}} > 0$, $p_{2}^{{D{*}}} - p_{2}^{{U{*}}} = \frac{{3(1 - \gamma )q_{2} (q_{1} - q_{2} )}}{{{8}(2q_{1} - q_{2} )}} > 0$,

$d_{{1}}^{D*} - d_{{1}}^{U*} = - \frac{{(1 - \gamma )\left( {(2q_{1} - q_{2} )(\frac{1}{2} - E_{1} ) - q_{2} (\frac{1}{2} - E_{2} )} \right)}}{{8(q_{1} - q_{2} )\gamma }} < 0$, and.

$d_{2}^{D*} - d_{2}^{U*} = - \frac{{(1 - \gamma )q_{1} \left( {(4q_{1} - 3q_{2} )(\frac{1}{2} - E_{2} ) - (2q_{1} - q_{2} )(\frac{1}{2} - E_{1} )} \right)}}{{{8}(q_{1} - q_{2} )(2q_{1} - q_{2} )\gamma }} < 0$.

Proof of Proposition 2

The retailer has an incentive to disclose information when $\Delta \pi_{r} = \pi_{r}^{D} - \pi_{r}^{U} \ge 0$. Then we can derive when $\gamma \ge \gamma_{r} { = }\frac{{\left( {\frac{1}{2} - E_{2} } \right)^{2} \left[ {4(q_{1} - q_{2} )\left[ {\overline{\rho }^{2} q_{1}^{2} \phi^{2} + q_{2} (4q_{1} - q_{2} )} \right] + q_{2} (q_{1} \overline{\rho }\phi - q_{2} )^{2} } \right]}}{{(4q_{1}^{2} + q_{1} q_{2} - q_{2}^{2} )(q_{1} - q_{2} )}}$, then $\Delta \pi_{r} \ge 0$.

Manufacturer $i$ has an incentive to disclose information when $\Delta \pi_{mi} = \pi_{mi}^{D} - \pi_{mi}^{U} \ge 0$, which gives that $\Delta \pi_{mi} \ge 0$ if $\gamma \ge \gamma_{i}$.

Proof of Lemma 4

By comparing the thresholds of information disclosure incentive of each member, we have when $E_{1} < E_{2}$, then $\gamma_{1} - \gamma_{r} > \frac{{4q_{2} (2q_{1} - q_{2} )^{{3}} \left( {(\frac{1}{2} - E_{1} )^{2} - (\frac{1}{2} - E_{2} )^{{2}} } \right)}}{{(4q_{1}^{2} + q_{1} q_{2} - q_{2}^{2} )(q_{1} - q_{2} )^{{2}} }} > 0$. As $\underline {\rho } < \rho^{D} < \overline{\rho }$, then $\frac{{4q_{1} - 3q_{2} }}{{2q_{1} - q_{2} }} > \frac{{1/2 - E_{1} }}{{1/2 - E_{2} }}$. Therefore, $\gamma_{r} - \gamma_{2} > \frac{{8(2q_{1} - q_{2} )^{3} (\frac{1}{2} - E_{2} )\left( {(\frac{1}{2} - E_{1} ) - (\frac{1}{2} - E_{2} )} \right)}}{{(4q_{1}^{2} + q_{1} q_{2} - q_{2}^{2} )(q_{1} - q_{2} )}} > 0$.

Thus, we can conclude that $\gamma_{2} < \gamma_{r} < \gamma_{1}$ if $E_{1} < E_{2}$. Similarly, we can show that $\gamma_{1} < \gamma_{r} < \gamma_{2}$ if $E_{1} > E_{2}$. In addition, when $E_{1} = E_{2} = E$, then $\gamma_{1} = \left( {1 - 2E} \right)^{2}$, $\gamma_{2} = \left( {1 - 2E} \right)^{2}$ and $\gamma_{r} = \left( {1 - 2E} \right)^{2}$, we have $\gamma_{{1}} = \gamma_{r} = \gamma_{{2}}$. Then we can obtain the results that are summarized in Lemma 4. With Proposition 2, we can derive results that are summarized in Table 3.

Proof of Lemma 5

Taking the derivatives of $\gamma_{{1}}$ and $\gamma_{{2}}$ w.r.t. $E_{{1}}$ and $E_{{2}}$, we have.

$\frac{{\partial \gamma_{1} }}{{\partial E_{{1}} }} = - \left( {1 - 2E_{{1}} } \right)\left( {\frac{{q_{1} \overline{\rho }}}{{q_{1} - q_{2} }}} \right)^{{2}} < 0$, $\frac{{\partial \gamma_{1} }}{{\partial E_{2} }} = \left( {1 - 2E_{2} } \right)\left( {\frac{{q_{2} }}{{q_{1} - q_{2} }}} \right)^{{2}} > 0$, $\frac{{\partial \gamma_{2} }}{{\partial E_{1} }} = \left( {1 - 2E_{{1}} } \right)\left( {\frac{{q_{1} \overline{\rho }}}{{q_{1} - q_{2} }}} \right)^{{2}} > 0$, and $\frac{{\partial \gamma_{2} }}{{\partial E_{2} }} = - \left( {1 - 2E_{2} } \right)\left( {\frac{{4q_{1} - 3q_{2} }}{{q_{1} - q_{2} }}} \right)^{{2}} < 0$.

Proof of Lemma 6

Taking the derivatives of $w_{i}^{U}$, $p_{i}^{U}$ and $d_{i}^{U}$ w.r.t. $\gamma$, we can get: $\frac{{\partial w_{{1}}^{U} }}{\partial \gamma } = \frac{{q_{1} (q_{{1}} - q_{2} )}}{{2(2q_{{1}} - q_{2} )}} > 0$, $\frac{{\partial w_{2}^{U} }}{\partial \gamma } = \frac{{q_{2} (q_{{1}} - q_{2} )}}{{4(2q_{{1}} - q_{2} )}} > 0$, $\frac{{\partial p_{1}^{U} }}{\partial \gamma } = \frac{{q_{1} (3q_{1} - 2q_{2} )}}{{{4}(2q_{1} - q_{2} )}} > 0$, $\frac{{\partial p_{2}^{U} }}{\partial \gamma } = \frac{{q_{2} (5q_{1} - 3q_{2} )}}{{8(2q_{1} - q_{2} )}} > 0$, $\frac{{\partial d_{{1}}^{U} }}{\partial \gamma } = - \frac{{(2q_{1} - q_{2} )({1} - {2}E_{1} ) - q_{2} ({1} - {2}E_{2} )}}{{{16}(q_{1} - q_{2} )\gamma^{2} }} < 0$, and $\frac{{\partial d_{{2}}^{U} }}{\partial \gamma } = - \frac{{q_{1} (4q_{1} - 3q_{2} )({1} - {2}E_{2} ) - q_{1} (2q_{1} - q_{2} )({1} - {2}E_{1} )}}{{{16}(q_{1} - q_{2} )(2q_{1} - q_{2} )\gamma^{2} }} < 0$. Then, taking the derivatives of $\pi_{{m_{1} }}^{U}$ w.r.t. $\gamma$, we have $\frac{{\partial \pi_{{m_{1} }}^{U} }}{\partial \gamma } = \frac{{q_{1} \left( {(q_{1} - q_{2} )\gamma } \right)^{2} - q_{1} \left( {(2q_{1} - q_{2} )(1 - {2}E_{1} ) - q_{2} (1 - {2}E_{2} )} \right)^{2} }}{{{32}(q_{1} - q_{2} )(2q_{1} - q_{2} )\gamma^{2} }}$. Then we can obtain $\frac{{\partial^{2} \pi_{{m_{{1}} }}^{U} }}{{\partial \gamma^{2} }} = \frac{{\left( {(2q_{1} - q_{2} )(1 - {2}E_{1} ) - q_{2} (1 - {2}E_{2} )} \right)^{2} }}{{{16}(q_{1} - q_{2} )(2q_{1} - q_{2} )\gamma^{3} }} > 0$. We conclude that there exists a unique minimum point, which is given by $\gamma = \sqrt {\gamma_{1} }$. Similarly, we can get the unique minimum point of Manufacturer 2 and the retailer, which are given by $\gamma = \sqrt {\gamma_{2} }$ and $\gamma = \sqrt {\gamma_{r} }$.

Proof of Proposition 3

When $E_{1} > E_{2}$, we have $\gamma_{1} < \gamma_{r} < \gamma_{2}$. If $y_{1} = N$ and $y_{{2}} = N$, then $y_{r} = P$ when $\gamma \ge \gamma_{r}$, and $y_{r} = N$ otherwise. Since Manufacturer 2 has an incentive to provide quality preference information when $\gamma \ge \gamma_{{2}}$, we can conclude that $y_{{2}} = N$. Since Manufacturer 1 has an incentive to provide quality preference information when $\gamma \ge \gamma_{{1}}$, then $y_{{1}} = P$ when $\gamma_{{1}} \le \gamma < \gamma_{r}$, and $y_{1} = N$ otherwise.

Similarly, when $E_{1} \le E_{2}$, we have $\gamma_{2} \le \gamma_{r} \le \gamma_{1}$. If $y_{1} = N$ and $y_{{2}} = N$, then $y_{r} = P$ when $\gamma \ge \gamma_{r}$, and $y_{r} = N$ otherwise. If $y_{1} = N$, then $y_{2} = P$ when $\gamma_{2} \le \gamma < \gamma_{r}$, and $y_{{2}} = N$ otherwise. Since Manufacturer 1 has an incentive to provide quality preference information only when $\gamma \ge \gamma_{{1}}$, thus $y_{1} = N$.

Proof of Table 4

For given $w^{\prime S}_{{1}}$ and $w^{\prime S}_{{2}}$, taking the derivatives of $\pi^{\prime S}_{r}$ w.r.t. $p^{\prime S}_{{1}}$ and $p^{\prime S}_{{2}}$:

$\frac{{\partial \pi^{\prime S}_{r} }}{{\partial p^{\prime S}_{{1}} }} = \frac{1 + I}{{2I}} + \frac{{ - 2p^{\prime S}_{{1}} + 2p^{\prime S}_{2} + w^{\prime S}_{{1}} - w^{\prime S}_{2} }}{{(q_{{1}} - q_{2} )I}}$ and $\frac{{\partial \pi^{\prime S}_{r} }}{{\partial p^{\prime S}_{2} }} = \frac{{2p^{\prime S}_{{1}} - 2p^{\prime S}_{2} - w^{\prime S}_{{1}} + w^{\prime S}_{2} }}{{(q_{{1}} - q_{2} )I}} + \frac{{ - 2p^{\prime S}_{2} + w^{\prime S}_{2} }}{{q_{2} I}}$. We have $\frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S2}_{1} }} = - \frac{2}{{(q_{1} - q_{2} )I}} < 0$, $\frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S2}_{2} }} = - \frac{{2q_{1} }}{{q_{2} (q_{1} - q_{2} )I}} < 0$, and.

$\frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S2}_{1} }}\frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S2}_{2} }} - \frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S}_{1} \partial p^{\prime S}_{2} }}\frac{{\partial^{2} \pi^{\prime S}_{r} }}{{\partial p^{\prime S}_{2} \partial p^{\prime S}_{1} }} = \frac{4}{{(q_{1} - q_{2} )q_{2} I^{2} }} > 0$. This suggests that there exist unique optimal solutions to ($p^{\prime S}_{{1}}$,$p^{\prime S}_{{2}}$), which are given by:

$$ p^{\prime S}_{{1}} = \frac{{(1 + I)q_{{1}} + 2w^{\prime S}_{{1}} }}{4}\;{\text{and}}\;p^{\prime S}_{{2}} = \frac{{(1 + I)q_{2} + 2w^{\prime S}_{{2}} }}{4} $$

(11)

Manufacturer 2 maximizes its profit $\pi^{\prime S}_{{m_{2} }}$ by setting $w^{\prime S}_{{2}}$. With $p^{\prime S}_{{1}}$ and $p^{\prime S}_{{2}}$ in (11), the demand of Product 1 and Product 2 are $d^{\prime S}_{1} = \frac{1 + I}{{4I}} - \frac{{w^{\prime S}_{1} - w^{\prime S}_{2} }}{{2(q_{1} - q_{2} )I}}$ and $d^{\prime S}_{2} = \frac{{q_{2} w^{\prime S}_{{1}} - q_{1} w^{\prime S}_{{2}} }}{{{2}q_{2} (q_{{1}} - q_{2} )I}}$, respectively. Then the profit of Manufacturer 1 and Manufacturer 2 are $\pi^{\prime S}_{{m_{1} }} = (w^{\prime S}_{1} - c_{2} )(\frac{1 + I}{{4I}} - \frac{{w^{\prime S}_{1} - w^{\prime S}_{2} }}{{2(q_{1} - q_{2} )I}})$ and $\pi^{\prime S}_{{m_{2} }} = (w^{\prime S}_{{2}} - c_{2} )(\frac{{q_{2} w^{\prime S}_{{1}} - q_{1} w^{\prime S}_{{2}} }}{{{2}q_{2} (q_{{1}} - q_{2} )I}})$, respectively. Then we have $\frac{{\partial \pi^{\prime S}_{{m_{1} }} }}{{\partial w^{\prime S}_{1} }} = \frac{1 + I}{{4I}} + \frac{{w^{\prime S}_{2} - 2w^{\prime S}_{1} + c_{2} }}{{2(q_{1} - q_{2} )I}}$ and $\frac{{\partial \pi^{\prime S}_{{m_{2} }} }}{{\partial w^{\prime S}_{{2}} }} = \frac{{w^{\prime S}_{{1}} - {2}w^{\prime S}_{{2}} + c_{2} }}{{{2}(q_{{1}} - q_{2} )I}} + \frac{{ - {2}w^{\prime S}_{{2}} + c_{2} }}{{{2}q_{2} I}}$. We thus can obtain $\frac{{\partial^{2} \pi^{\prime S}_{{m_{1} }} }}{{\partial w^{\prime S2}_{1} }} = - \frac{1}{{(q_{1} - q_{2} )I}} < 0$ and $\frac{{\partial^{2} \pi^{S}_{{m_{2} }} }}{{\partial w^{S2}_{2} }} = - \frac{1}{{(q_{1} - q_{2} )I}} - \frac{1}{{q_{2} I}} < 0$. We conclude that there exist unique optimal solutions to ($w^{\prime S}_{{1}}$,$w^{\prime S}_{{2}}$), which are given by:

$w^{\prime S*}_{1} = \frac{{(1 + I)q_{1} }}{2} - \frac{{q_{1} (2\Delta_{1}^{S} + \Delta_{2}^{S} )}}{{4q_{1} - q_{2} }}$ and $w^{\prime S*}_{2} = \frac{{(1 + I)q_{2} }}{2} - \frac{{q_{2} \Delta_{1}^{S} + 2q_{1} \Delta_{2}^{S} }}{{4q_{{1}} - q_{2} }}$.

Substituting $w^{\prime S*}_{1}$ and $w^{\prime S*}_{2}$ into (11), we can derive ($p^{\prime S*}_{{1}}$, $p^{\prime S*}_{{2}}$) and we can get ($d^{\prime S*}_{1}$, $d^{\prime S*}_{2}$, $\pi^{\prime S*}_{{m_{1} }}$, $\pi^{\prime S*}_{{m_{2} }}$, $\pi^{\prime S*}_{r}$) as in Table 4.

Manufacturer 1 has an incentive to disclose information when $\Delta \pi_{{m_{{1}} }} = \pi_{{m_{{1}} }}^{D} - \pi_{{m_{{1}} }}^{U} \ge 0$. Then we can derive when $\gamma \ge \gamma_{{1}}$, $\Delta \pi_{{m_{{1}} }} \ge 0$.

Similarly, Manufacturer 2 and the retailer have incentives to disclose information when $\Delta \pi_{{m_{{2}} }} = \pi_{{m_{{2}} }}^{D} - \pi_{{m_{{2}} }}^{U} \ge 0$ and $\Delta \pi_{r} = \pi_{r}^{D} - \pi_{r}^{U} \ge 0$, separately. Then we can derive when $\gamma \ge \gamma^{\prime}_{2} = \left( {{1} - {2}E_{2} } \right)^{2} \left( {\frac{{{2}q_{1} - q_{2} - q_{1} \phi }}{{q_{1} - q_{2} }}} \right)^{2}$ and $\gamma \ge \gamma^{\prime}_{r} { = }\left( {{1} - {2}E_{2} } \right)^{2} \frac{{q_{1} (4q_{1} - {3}q_{2} )\phi^{2} - 2q_{2}^{2} \phi + 2q_{2} (2q_{1} - q_{2} )}}{{4q_{1}^{2} + q_{1} q_{2} - 4q_{2}^{2} }}$, $\Delta \pi_{{m_{{2}} }} \ge 0$ and $\Delta \pi_{r} \ge 0$, separately.

Proof of Proposition 5

When $E_{1} > E_{2}$, we have $\gamma_{1} < \gamma_{r} < \gamma_{2}$. If $y_{1} = N$ and $y_{{2}} = N$, then $y_{r} = P$ when $\gamma \ge \gamma_{r}$ and $c_{I} \le \Delta \pi_{r}$, and $y_{r} = N$ otherwise. Since Manufacturer 2 has incentive to provide quality preference information if $\gamma \ge \gamma_{{2}}$, therefore,$y_{{2}} = N$. It is obvious that $y_{{1}} = P$ when $\gamma_{{1}} \le \gamma < \gamma_{r}$ and $c_{I} \le \Delta \pi_{{m_{1} }}$, or when $\gamma_{r} \le \gamma < \overline{\gamma }_{1}$ and $\Delta \pi_{r} < c_{I} \le \Delta \pi_{{m_{1} }}$, and $y_{1} = N$ otherwise.

Similarly, when $E_{1} \le E_{2}$, we have $\gamma_{2} \le \gamma_{r} \le \gamma_{1}$. If $y_{1} = N$ and $y_{{2}} = N$, then $y_{r} = P$ when $\gamma \ge \gamma_{r}$ and $c_{I} \le \Delta \pi_{r}$, and $y_{r} = N$ otherwise. If $y_{1} = N$, then $y_{2} = P$ when $\gamma_{2} \le \gamma < \gamma_{r}$ and $c_{I} \le \Delta \pi_{{m_{2} }}$, or when $\gamma_{r} \le \gamma < \overline{\gamma }_{2}$ and $\Delta \pi_{r} < c_{I} \le \Delta \pi_{{m_{2} }}$, and $y_{{2}} = N$ otherwise. Since Manufacturer 1 has incentive to provide quality preference information when $\gamma \ge \gamma_{{1}}$, thus $y_{1} = N$. Then we can obtain Table 5.