Retailer's optimal strategy for a perishable product with increasing demand under various payment schemes

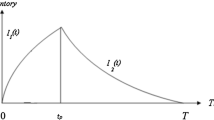
Abstract

This paper studies the optimal replenishment strategy of the retailer under partial two levels of credit. The paper also considers the following scenarios: (1) the product under consideration is a deteriorating item, (2) the demand function is an incremental function of time, and (3) the retailer pays the supplier by the payment method of Advance-Cash-Credit (ACC) and gives his/her customers a certain credit period. (4) The supplier provides the retailer with a certain price discount to facilitate sales. The goal of the paper is to decide the retailer’s order cycle, which minimizes his/her total cost per unit time. Firstly, we proved the existence and uniqueness of the optimal solution. Secondly, we validated the theoretical results and discussed the performance of upstream ACC payment and downstream credit payment by numerical analysis of key parameters, numerical results show that it is cheaper for the retailer to pay for the payment in upstream ACC and downstream credit payment than in traditional payment method (i.e., upstream cash and downstream cash payment method), which encourage the retailer to order more quantity and less frequently under the former payment method. Thirdly, we compared the retailer’s order behaviors and total cost per unit time under the following five two-level payment types: upstream cash and downstream cash payment, upstream advance, cash, credit, ACC and downstream credit payment, in which the supplier will provide a certain price discount when the retailer pays in advance. It is found that the retailer pays the supplier in upstream advance and downstream credit payment is the lowest cost to the retailer, and it will lead to order the most quantities under this payment method, while the retailer pays the supplier with upstream credit and downstream credit (upstream credit period is shorter than the downstream credit period) is the highest cost to the retailer. The research results can help the retailers make the payment selections and optimize their operational decisions.

Appendices

Appendix A: Proof of Theorem 1

From (8), let

$$ f_{1} (T) = K + c\int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} + (h + I_{c} c)\int_{0}^{T} {\int_{t}^{T} {D(x)e^{\theta (x - t)} {\text{d}}x\,{\text{d}}t} } . $$

and

$$ g_{1} (T) = T > 0 $$

Now, evaluating the first-order and second-order derivatives of $f_{1} (T)$ with respect to $T$, we obtain

$$ f^{\prime}_{1} (T) = cD(T)e^{\theta T} + (h + I_{c} c)\int_{0}^{T} {D(T)e^{\theta (T - t)} {\text{d}}t} . $$

(A1)

and

$$ f_{1}^{\prime \prime } (T) = ce^{\theta T} [D^{\prime } (T) + \theta D(T)] + (h + I_{c} c)\{ D(T) + \int_{0}^{T} {[D^{\prime } (T) + \theta D(T)]e^{\theta (T - t)} {\text{d}}t} \} > 0. $$

(A2)

Applying Theorems 3.2.9–3.2.10 from Cambini and Martein (2009), we can get the conclusion that $TC_{1} (T) = f_{1} (T)/g_{1} (T)$ is a strictly pseudo-convex function with respect to $T.$ Therefore, a unique global minimum $T_{1}^{ * }$ exists. The proof of Theorem 1 gets complete here.

Appendix B: Proof of Theorem 2

From (14), for convenience, let

$$ \begin{aligned} f_{21} (T) & = K + [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]\int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} \\ & \quad + h\int_{0}^{T} {\int_{t}^{T} {D(x)e^{\theta (x - t)} {\text{d}}x{\text{d}}t} } + (u + v)(1 - r)cI_{c} \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } \\ & \quad + w(1 - r)cI_{c} \int_{{R_{1} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } - wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {\int_{{R_{2} }}^{t} {D(x - N){\text{d}}x{\text{d}}t} } . \\ \end{aligned} $$

(B1)

and

$$ g_{21} (T) = T > 0 $$

Evaluating the first-and the second-order derivatives of $f_{21} (T)$ with respect to $T$, we obtain

$$ \begin{aligned} f_{21}^{\prime } (T) & = [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]D(T)e^{\theta T} \\ & \quad + h\int_{0}^{T} {D(T)e^{\theta (T - t)} {\text{d}}t} + (u + v)(1 - r)cI_{c} \int_{{R_{2} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} \\ & \quad + w(1 - r)cI_{c} \int_{{R_{1} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} \\ \end{aligned} $$

(B2)

and

$$ \begin{aligned} f_{21}^{\prime \prime } (T) & = [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]e^{\theta T} [D^{\prime } (T) + \theta D(T)] \\ & \quad + h\left\{ {D(T) + \int_{0}^{T} {[D^{\prime } (T) + \theta D(T)]e^{\theta (T - t)} {\text{d}}t} } \right\} \\ & \quad + (u + v)(1 - r)cI_{c} \left\{ {D(T) + \int_{{R_{2} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} } \right\} \\ & \quad + w(1 - r)cI_{c} \left\{ {D(T) + \int_{{R_{1} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} } \right\} > 0 \\ \end{aligned} $$

(B3)

Therefore, $TC_{21} (T)$ is a strictly pseudo-convex function with respect to T, thus a unique global minimum $T_{21}^{ * }$ exists. The proof of Part (a) of Theorem 2 gets complete here.

Calculating the first-order derivative of $TC_{21} (T)$ in (14) with respect to T, we yield

$$ \begin{aligned} \frac{{{\text{d}}TC_{21} (T)}}{{{\text{d}}T}} & = - \frac{1}{{T^{2} }}\{ K - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]\left[ {TD(T)e^{\theta T} - \int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} } \right] \\ & \quad - h\left[ {T\int_{0}^{T} {D(T)e^{\theta (T - t)} {\text{d}}t} - \int_{0}^{T} {\int_{t}^{T} {D(x)e^{\theta (x - t)} {\text{d}}x{\text{d}}t} } } \right] \\ & \quad - (u + v)(1 - r)cI_{c} \left[ {T\int_{{R_{2} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} - \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } } \right] \\ & \quad - w(1 - r)cI_{c} \left[ {T\int_{{R_{1} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} - \int_{{R_{1} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } } \right] \\ & \quad - wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {\int_{{R_{2} }}^{t} {D(x - R_{2} ){\text{d}}x{\text{d}}t = - \frac{1}{{T^{2} }}H_{21} (T)} } . \\ \end{aligned} $$

(B4)

Calculating the first-order derivative of $H_{21} (T)$ with respect to T, we obtain

$$ \begin{aligned} H_{21}^{\prime } (T) & = - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]Te^{\theta T} [D^{\prime } (T) + \theta D(T)] \\ & \quad - hT\left\{ {D(T) + \int_{0}^{T} {[D^{\prime } (T) + \theta D(T)]e^{\theta (T - t)} {\text{d}}t} } \right\} \\ & \quad - (u + v)(1 - r)cI_{c} T\left\{ {D(T) + \int_{{R_{2} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} } \right\} \\ & \quad - w(1 - r)cI_{c} T\left\{ {D(T) + \int_{{R_{1} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} } \right\} < 0 \\ \end{aligned} $$

(B5)

Consequently, $H_{21} (T)$ is strictly decreasing on $T \in [R_{1} - R_{2} , + \infty ).$ Obviously, $\mathop {\lim }\limits_{T \to + \infty } H_{21} (T) = - \infty .$ Hence, let

$$ \begin{aligned} \Delta_{21} & = H_{21} (R_{1} - R_{2} ) \\ & = K - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ][(R_{1} - R_{2} )D(R_{1} - R_{2} )e^{{\theta (R_{1} - R_{2} )}} \\ & \quad - \int_{0}^{{R_{1} - R_{2} }} {D(x)e^{\theta x} dx]} - h[(R_{1} - R_{2} )\int_{0}^{{R_{1} - R_{2} }} {D(R_{1} - R_{2} )e^{{\theta (R_{1} - R_{2} - t)}} dt} \\ & \quad - \int_{0}^{{R_{1} - R_{2} }} {\int_{t}^{{R_{1} - R_{2} }} {D(x)e^{\theta (x - t)} dxdt} } ] - (u + v)(1 - r)cI_{c} [(R_{1} - R_{2} )\int_{{R_{2} }}^{{R_{1} }} {D(R_{1} - R_{2} )e^{{\theta (R_{1} - t)}} dt} \\ & \quad - \int_{{R_{2} }}^{{R_{1} }} {\int_{{t - R_{2} }}^{{R_{1} - R_{2} }} {D(x)e^{{\theta (x - t + R_{2} )}} dxdt} } ] - wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {\int_{{R_{2} }}^{t} {D(x - R_{2} )dxdt} } \\ \end{aligned} $$

(B6)

If $\Delta_{21} = H_{21} (R_{1} - R_{2} ) > 0,$ applying the Mean Value Theorem, we obtain a unique $T_{21}^{ * } \in (R_{1} - R_{2} , + \infty )$ such that $H_{21} (T_{21}^{ * } ) = 0$. $H_{21} (T)$ is a positive function in $T \in [R_{1} - R_{2} ,T_{21}^{ * } ],$ and a negative function in $T \in [T_{21}^{ * } , + \infty ].$ Therefore, $TC_{21} (T)$ is decreasing in $T \in [R_{1} - R_{2} ,T_{21}^{ * } ],$ and increasing in $T \in [T_{21}^{ * } , + \infty ).$ The proof of Part (b) of Theorem 2 gets complete here.

If $\Delta_{21} = H_{21} (R_{1} - R_{2} ) < 0,$ then $H_{21} (T)$ is negative for all $T \in [R_{1} - R_{2} , + \infty ).$ Hence, $TC_{21} (T)$ is increasing in $T \in [R_{1} - R_{2} , + \infty ).$ The proof of Part (c) of Theorem 2 gets complete here.

Appendix C: Proof of Proposition 1

$$ \begin{aligned} ({\text{a}})\quad \quad \frac{{\partial TC_{21} (T)}}{\partial r}\left| {_{{T = T_{21}^{ * } }} } \right. & = \left( {\frac{{\partial TC_{21} (T)}}{\partial T} \cdot \frac{\partial T}{{\partial r}} + \frac{{\partial TC_{21} (T)}}{\partial r}} \right)\left| {_{{T = T_{21}^{ * } }} } \right. \\ & = - \frac{1}{T}\left\{ {[c + cI_{c} u(R_{2} + d) + cI_{c} vR_{2} ]\int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} } \right. \\ & \quad + (u + v)cI_{c} \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } \\ & \quad \left. { + wcI_{c} \int_{{R_{1} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } } \right\}\left| {_{{T = T_{21}^{ * } }} } \right. < 0. \\ \end{aligned} $$

(C1)

$$ \begin{aligned} ({\text{b}})\quad \quad \frac{{\partial TC_{21} (T)}}{{\partial R_{1} }}\left| {_{{T = T_{21}^{ * } }} } \right. & = \left( {\frac{{\partial TC_{21} (T)}}{\partial T} \cdot \frac{\partial T}{{\partial R_{1} }} + \frac{{\partial TC_{21} (T)}}{{\partial R_{1} }}} \right)\left| {_{{T = T_{21}^{ * } }} } \right. \\ & = - \frac{1}{T}\left\{ {w(1 - r)cI_{c} \int_{{R_{1} - R_{2} }}^{T} {D(x)e^{{\theta (x - R_{1} + R_{2} )}} {\text{d}}x} + wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {D(x - R_{2} ){\text{d}}x} } \right\}\left| {_{{T = T_{21}^{ * } }} } \right. < 0. \\ \end{aligned} $$

(C2)

$$ \begin{aligned} ({\text{c}})\quad \quad \frac{{\partial TC_{21} (T)}}{\partial d}\left| {_{{T = T_{21}^{ * } }} } \right. & = \left( {\frac{{\partial TC_{21} (T)}}{\partial T} \cdot \frac{\partial T}{{\partial d}} + \frac{{\partial TC_{21} (T)}}{\partial d}} \right)\left| {_{{T = T_{21}^{ * } }} } \right. \\ & = \frac{1}{T}\left[ {(1 - r)cI_{c} u\int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} } \right]\left| {_{{T = T_{21}^{ * } }} } \right. > 0. \\ \end{aligned} $$

(C3)

$$ \begin{aligned} ({\text{d}})\quad \quad \frac{{\partial TC_{21} (T)}}{{\partial R_{2} }}\left| {_{{T = T_{21}^{ * } }} } \right. & = \left( {\frac{{\partial TC_{21} (T)}}{\partial T} \cdot \frac{\partial T}{{\partial R_{2} }} + \frac{{\partial TC_{21} (T)}}{{\partial R_{2} }}} \right)\left| {_{{T = T_{21}^{ * } }} } \right. \\ & = \frac{1}{T}\left\{ {(u + v)(1 - r)cI_{c} \int_{{R_{2} }}^{{T + R_{2} }} {\left[ {D(t - R_{2} ) + \int_{{t - R_{2} }}^{T} {D(x)\theta } (t - R_{2} )e^{{\theta (x - t + R_{2} )}} {\text{d}}x} \right]{\text{d}}t} } \right. \\ & \quad + w(1 - r)cI_{c} \int_{{R_{1} }}^{{T + R_{2} }} {\left[ {D(t - R_{2} ) + \int_{{t - R_{2} }}^{T} {D(x)\theta } (t - R_{2} )e^{{\theta (x - t + R_{2} )}} {\text{d}}x} \right]{\text{d}}t} \\ & \quad \left. { + wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {\left[ {D(0) + \int_{{R_{2} }}^{t} {D^{\prime } (x - R_{2} ){\text{d}}x} } \right]{\text{d}}t} } \right\}\left| {_{{T = T_{21}^{ * } }} } \right. > 0. \\ \end{aligned} $$

(C4)

Appendix D: Proof of Theorem 3

From (16), we define

$$ \begin{aligned} f_{22} (T) & = K + [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]\int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} \\ & \quad + h\int_{0}^{T} {\int_{t}^{T} {D(x)e^{\theta (x - t)} {\text{d}}x{\text{d}}t} } + (u + v)(1 - r)c_{p} I_{c} \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } \\ & \quad - wpI_{e} \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{R_{2} }}^{t} {D(x - R_{2} ){\text{d}}x{\text{d}}t} } - wpI_{e} (R_{1} - T - R_{2} )\int_{0}^{T} {D(t){\text{d}}t} \\ \end{aligned} $$

(D1)

and

$$ g_{22} (T) = T > 0 $$

Now, calculating the first order and the second-order derivatives of $f_{22} (T)$ with respect to T, we have

$$ \begin{aligned} f_{22}^{\prime } (T) & = [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]D(T)e^{\theta T} \\ & \quad + h\int_{0}^{T} {D(T)e^{\theta (T - t)} dt} + (u + v)(1 - r)cI_{c} \int_{{R_{2} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} \\ & \quad - wpI_{e} \int_{{R_{2} }}^{{T + R_{2} }} {D(x - R_{2} ){\text{d}}x + } wpI_{e} \int_{0}^{T} {D(t){\text{d}}t} - wpI_{e} (R_{1} - T - R_{2} )D(T) \\ \end{aligned} $$

(D2)

and

$$ \begin{aligned} f_{22}^{\prime \prime } (T) & = \{ [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]e^{\theta T} - wpI_{e} (R_{1} - T - R_{2} )\} D^{\prime } (T) \\ & \quad + [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]D(T)\theta e^{\theta T} \\ & \quad + h\{ D(T) + \int_{0}^{T} {[D^{\prime } (T) + \theta D(T)]e^{\theta (T - t)} {\text{d}}t} \} \\ & \quad + (u + v)(1 - r)cI_{c} \{ D(T) + \int_{{R_{2} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} \} + wpI_{e} D(T) \\ \end{aligned} $$

(D3)

Without loss of generality, we may assume that $[(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]e^{\theta T} - wpI_{e} (R_{1} - T - R_{2} ) > 0$, because in the second term, $\tau$, $I_{e}$ and $(M - T - N)$ all are significantly less than 1. Therefore, $f_{22}^{\prime \prime } (T) > 0$, hence, $TC_{22} (T)$ is a strictly pseudo-convex function in T, which implies a unique global minimum $T_{22}^{ * }$ exists such that $TC_{22} (T)$ is minimized. The proof of Part (a) of Theorem 3 gets complete here.

Calculating the first-order derivative of $TC_{22} (T)$ in (21) with respect to T, we get

$$ \begin{aligned} \frac{{{\text{d}}TC_{22} (T)}}{{{\text{d}}T}} & = - \frac{1}{{T^{2} }}\left\{ {K - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]\left[ {TD(T)e^{\theta T} - \int_{0}^{T} {D(x)e^{\theta x} {\text{d}}x} } \right]} \right. \\ & \quad - (u + v)(1 - r)cI_{c} \left[ {T\int_{{R_{2} }}^{{T + R_{2} }} {D(T)e^{{\theta (T - t + R_{2} )}} {\text{d}}t} - \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{t - R_{2} }}^{T} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } } \right] \\ & \quad - h\left[ {T\int_{0}^{T} {D(T)e^{\theta (T - t)} {\text{d}}t} - \int_{0}^{T} {\int_{t}^{T} {D(x)e^{\theta (x - t)} {\text{d}}x{\text{d}}t} } } \right] \\ & \quad + wpI_{e} \left[ {T\int_{{R_{2} }}^{{T + R_{2} }} {D(x - R_{2} ){\text{d}}x - } \int_{{R_{2} }}^{{T + R_{2} }} {\int_{{R_{2} }}^{t} {D(x - R_{2} ){\text{d}}x{\text{d}}t} } } \right] \\ & \quad - \left. {wpI_{e} \left[ {T\int_{0}^{T} {D(t)dt - (R_{1} - T - R_{2} )} TD(T) + (R_{1} - T - R_{2} )\int_{0}^{T} {D(t){\text{d}}t} } \right]} \right\} = - \frac{1}{{T^{2} }}H_{22} (T) \\ \end{aligned} $$

(D4)

Furthermore,

$$ \begin{aligned} H_{22}^{\prime } (T) & = \{ - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]e^{\theta T} + wpI_{e} (R_{1} - T - R_{2} )\} TD^{\prime } (T) \\ & \quad - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]TD(T)\theta ]e^{\theta T} \\ & \quad - hT\left\{ {D(T) + \int_{0}^{T} {[D^{\prime } (T) + \theta D(T)]e^{\theta (T - t)} {\text{d}}t} } \right\} \\ & \quad - (u + v)(1 - r)cI_{c} T\left\{ {D(T) + \int_{{R_{2} }}^{{T + R_{2} }} {[D^{\prime } (T) + \theta D(T)]e^{{\theta (T - t + R_{2} )}} {\text{d}}t} } \right\} - wpI_{e} TD(T) \\ \end{aligned} $$

(D5)

Similarly, without loss of generality, we may assume that.

$$ - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]e^{\theta T} + wpI_{e} (R_{1} - T - R_{2} ) < 0. $$

Hence, $H_{22}^{\prime } (T) < 0$. Since $H_{22} (0) = K > 0$, let

$$ \begin{aligned} \Delta_{22} & = H_{22} (R_{1} - R_{2} ) \\ & = K - [(1 - r)c + (1 - r)cI_{c} u(R_{2} + d) + (1 - r)cI_{c} vR_{2} ]\left[ {(R_{1} - R_{2} )D(R_{1} - R_{2} )e^{{\theta (R_{1} - R_{2} )}} } \right. \\ & \quad - \int_{0}^{{R_{1} - R_{2} }} {D(x)e^{\theta x} {\text{d}}x]} - h[(R_{1} - R_{2} )\int_{0}^{{R_{1} - R_{2} }} {D(R_{1} - R_{2} )e^{{\theta (R_{1} - R_{2} - t)}} {\text{d}}t} \\ & \quad \left. { - \int_{0}^{{R_{1} - R_{2} }} {\int_{t}^{{R_{1} - R_{2} }} {D(x)e^{\theta (x - t)} {\text{d}}x{\text{d}}t} } } \right] - (u + v)(1 - r)cI_{c} \left[ {(R_{1} - R_{2} )\int_{{R_{2} }}^{{R_{1} }} {D(R_{1} - R_{2} )e^{{\theta (R_{1} - t)}} {\text{d}}t} } \right. \\ & \quad \left. { - \int_{{R_{2} }}^{{R_{1} }} {\int_{{t - R_{2} }}^{{R_{1} - R_{2} }} {D(x)e^{{\theta (x - t + R_{2} )}} {\text{d}}x{\text{d}}t} } } \right] - wpI_{e} \int_{{R_{2} }}^{{R_{1} }} {\int_{{R_{2} }}^{t} {D(x - R_{2} ){\text{d}}x{\text{d}}t} } \\ \end{aligned} $$

(D6)

Consequently, if $\Delta_{22} \ge 0,$ then $H_{22} (T) \ge 0$ for all $T \in [0,R_{1} - R_{2} ].$ Hence, $TC^{\prime}_{22} (T) < 0$ for all $T \in [0,R_{1} - R_{2} ],$ and $TC_{22} (T)$ is decreasing in $T \in [0,R_{1} - R_{2} ].$ This completes the proof of Part (b) of Theorem 3. On the other hand, if $\Delta_{22} < 0,$ applying the Mean Value Theorem, we know that there exists a unique $T_{22}^{ * } \in (0,R_{1} - R_{2} )$ such that $H_{22} (T) = 0$. $H_{22} (T)$ is positive in $T \in [0,T_{21}^{ * } ],$ and negative in $T \in [T_{21}^{ * } ,R_{1} - R_{2} ].$ Therefore, $TC_{21} (T)$ is decreasing in $T \in [0,T_{21}^{ * } ],$ and increasing in $T \in [T_{21}^{ * } ,R_{1} - R_{2} ].$ The proof of Part (c) of Theorem 3 gets complete here.

Appendix F: Proof of Theorem 4

From (20) and (22), we know that $\Delta_{21} = \Delta_{22}$, Moreover, $TC_{21} (R_{1} - R_{2} ) = TC_{22} (R_{1} - R_{2} ).$ Therefore, when $R_{2} \le R_{1} .$ If $\Delta_{21} = \Delta_{22} < 0,$ then (1) $TC_{22} (T)$ is decreasing on $(0,T_{22}^{ * } )$ and increasing on $(T_{22}^{ * } ,R_{1} - R_{2} )$; (2) $TC_{21} (T)$ is increasing on $(R_{1} - R_{2} , + \infty ).$ Combining (1), (2), and the fact that $TC_{22} (T_{22}^{ * } ) < TC_{21} (R_{1} - R_{2} ) = TC_{22} (R_{1} - R_{2} ).$ We can conclude that $T_{2}^{ * } = T_{22}^{ * }$. This completes the proof of Part (a) of Theorem 4.

If $\Delta_{211} = \Delta_{22} > 0,$ then (1) $TC_{21} (T)$ is decreasing on $(R_{1} - R_{2} ,T_{21}^{ * } ),$ and increasing on $(T_{21}^{ * } , + \infty )$; (2) $TC_{22} (T)$ is decreasing on $(0,R_{1} - R_{2} )$. Then from (1), (2), and the fact that $TC_{21} (T_{21}^{ * } ) < TC_{21} (R_{1} - R_{2} ) = TC_{22} (R_{1} - R_{2} ).$ We can easily get $T_{2}^{ * } = T_{21}^{ * }$. The proof of Part (b) of Theorem 4 gets complete here.