Optimal pre-order strategy with delay in payments

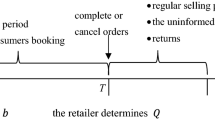
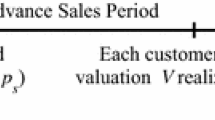Abstract

Advance selling with pre-orders has become a common practice in many industries. To encourage more consumers to purchasing in advance, retailers now try new forms of advance selling to allow consumers to complete their pre-orders after solving valuation uncertainty. Motivated by this emerging industry practice, this paper focuses on delay in payments from a retailer to consumers during the advance selling period and considers several pre-order strategies for the retailer, namely, free pre-order (FP), deposit pre-order (DP), and deposit expansion pre-order. Under the price commitment mechanism, we first derive not only the thresholds for pre-order strategies with stochastic demand and uncertain product valuation but also the retailer’s optimal price, quantity, and advance selling strategy, which are contingent on the relative size of the marginal cost and the lower bound of consumer valuation. Then, we find that the retailer should sell in advance even when the expected valuation is sufficiently low, or the marginal cost is relatively high. Besides, we reveal that the retailer is more likely to order a smaller quantity under the FP and DP strategies. Finally, we extend the model to a fixed pricing mechanism to further elucidate the retailer’s optimal decisions. By comparing these two pricing mechanisms, we find that, under certain conditions, the optimal pre-announced pricing strategy converges to the fixed pricing strategy.

Appendix

1.1 Proof of Theorem 1

Note that strategic informed consumers are willing to pre-order new products if and only if they can get more expected utilities. From the expression (11), we can obtain $X+\int _{X-em}^{p} F(v)\, dv\le p+\eta (h-p)+(e-1)m-\eta \int _{p}^{h} F(v)\, dv$. Because the left of inequality is increasing of X, we can get a maximum pre-order price $X^{*}$ if all other factors are given. Therefore, the retailer would adopt this optimal pre-order price that induces all informed consumers to purchase with pre-orders.

1.2 Proof of Proposition 1

Let $\vec {x}=(m^{*}, X^{*}, e^{*})$ be a local maximum solution for the problem of pure optimization which is expressed as follows:

$$\begin{aligned} \begin{aligned} \max&f(\vec {x}),\\ s.t.&\left\{ \begin{array}{lll} g_1(\vec {x})\le 0,\\ \cdots \\ g_m(\vec {x})\le 0, \end{array} \right. \\ \end{aligned} \end{aligned}$$

(A.1)

For this model, its constraint is $(1-\eta )\int _p^h (v-p)f(v)\, dv+m-\int _{X-em}^{h} (V-X+em)f(v)\,dv\le 0$. Then it exists a vector $\vec {r}=(r_1^{*}, r_2^{*}, r_3^{*}, r_4^{*})$, and its component $r_i^{*}\ge 0, i=1, 2, 3, 4$. It makes $\nabla f(\vec {x})-\sum _{i=1}^4 r_i^{*}\nabla g_i(\vec {x})=0$, $r_i^{*}g_i(\vec {x})=0$. So we can obtain:

$$\begin{aligned}&\Big [1-e^{*}{\bar{F}}(X^{*}-e^{*}m^{*})+e^{*}(X^{*}-c-e^{*}m^{*})f(X^{*}-e^{*}m^{*})\Big ]\mu _i\nonumber \\&\quad +r_1^{*}e^{*}{\bar{F}}(X^{*}-e^{*}m^{*})-r_2^{*}-r_3^{*}=0,\nonumber \\&\Big [{\bar{F}}(X^{*}-e^{*}m^{*})-(X^{*}-c-e^{*}m^{*})f(X^{*}-e^{*}m^{*})\Big ]\mu _i\nonumber \\&\quad -r_1^{*}{\bar{F}}(X^{*}-e^{*}m^{*})-r_3^{*}=0,\nonumber \\&\Big [-m^{*}{\bar{F}}(X^{*}-e^{*}m^{*})+m^{*}(X^{*}-c-e^{*}m^{*})f(X^{*}-e^{*}m^{*})\Big ]\mu _i\nonumber \\&\quad +r_1^{*}m^{*}{\bar{F}}(X^{*}-e^{*}m^{*})-r_4^{*}=0,\nonumber \\&r_1^{*}\Big [(1-\eta )\int _p^h (v-p)f(v)\, dv+m^{*}-\int _{X^{*}-e^{*}m^{*}}^{h} (V-X^{*}+e^{*}m^{*})f(v)\,dv\Big ]=0. \end{aligned}$$

(A.2)

By mathematical calculation, it’s easy to get the results:

$$\begin{aligned} \begin{aligned}&X^{*}=e^{*}m^{*}+c,\\&m^{*}=p-c+\eta (h-p)-\eta \int _p^h F(v)\, dv-\int _c^p F(v)\, dv; \end{aligned} \end{aligned}$$

(A.3)

or

$$\begin{aligned} \begin{aligned}&X^{*}=e^{*}m^{*}+l,\\&m^{*}=p-l+\eta (h-p)-\eta \int _p^h F(v)\, dv-\int _l^p F(v)\, dv. \end{aligned} \end{aligned}$$

(A.4)

Substituting Eqs. (A.3), (A.4) into the total profit function, we have:

$$\begin{aligned} \begin{aligned} \pi _1^{*}&=\Big [p-c+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _c^h F(v)\, dv\Big ]\mu _i\\&\quad +(p-c)\mu _u{\bar{F}}(p)-(p-s)\varphi (k){\bar{F}}(p)\sigma _u\sqrt{1-\rho ^2}; \end{aligned} \end{aligned}$$

(A.5)

or

$$\begin{aligned} \begin{aligned} \pi _1^{*}&=\Big [p-c+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv\Big ]\mu _i\\&\quad +(p-c)\mu _u{\bar{F}}(p)-(p-s)\varphi (k){\bar{F}}(p)\sigma _u\sqrt{1-\rho ^2}. \end{aligned} \end{aligned}$$

(A.6)

Intuitively, we can obtain $\pi _1^{*}=\pi _2^{*}$ if $c\le l$; otherwise, $\pi _1^{*}>\pi _2^{*}$.

1.3 Proof of Proposition 3

Similar to Proof of Proposition 1, let $\vec {x}=(m^{'*}, e^{'*})$ be a local maximum solution for the problem of pure optimization, its constraint is $(1-\eta )\int _p^h (v-p)f(v)\, dv+m^{'}-\int _{X^{*}-e^{'}m^{'}}^{h} (V-X^{*}+e^{'}m^{'})f(v)\,dv\le 0$. So we can obtain:

$$\begin{aligned} \begin{aligned}&\Big [1-e^{'*}{\overline{F}}(X^{*}-e^{'*}m^{'*})+e^{'*}(X^{*}-c-e^{'*}m^{'*})f(X^{*}-e^{'*}m^{'*})\Big ]\mu _i\\&\quad +r_1^{*}e^{'*}{\overline{F}}(X^{*}-e^{'*}m^{'*})-r_1^{*}-r_2^{*}=0,\\&\Big [-m^{'*}{\overline{F}}(X^{*}-e^{'*}m^{'*})+m^{'*}(X^{*}-c-e^{'*}m^{'*})f(X^{*}-e^{'*}m^{'*})\Big ]\mu _i\\&\quad +r_1^{*}m^{'*}{\overline{F}}(X^{*}-e^{'*}m^{'*})-r_3^{*}=0,\\&r_1^{*}\Big [p+\eta (h-p)+(e^{'*}-1)m^{'*}-\eta \int _p^h F(v)\, dv-X^{*}-\int _{X^{*}-e^{'*}m^{'*}}^p F(v)\, dv\Big ]=0. \end{aligned} \end{aligned}$$

(A.7)

By mathematical calculation, we can obtain the results:

$$\begin{aligned} e^{'*}m^{'*}=m^{*}; \end{aligned}$$

(A.8)

or

$$\begin{aligned} f(X^{*}-e^{'*}m^{'*})=0. \end{aligned}$$

(A.9)

If $e^{'*}m^{'*}=m^{*}$, and bring it to the constraint, we can obtain $m^{'*}=p-c+\eta (h-p)-\eta \int _p^h F(v)\, dv-\int _c^p F(v)\, dv=m^{*}$, leading to $e^{'*}=1$. So we neglect this case. If $f(X^{*}-e^{'*}m^{'*})=0$, $e^{'*}m^{'*}=m^{*}+c-l$ and bring it to the constraint, we can get $m^{'*}=p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv$, and $e^{'*}=\frac{p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _c^h F(v)\, dv}{p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv}>1$ if and only if $l<c$.

In summary, we can obtain optimal deposit and deposit expansion index under this setting with the condition $l<c$, i.e., $m^{'*}=p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv$, $e^{'*}=\frac{p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _c^h F(v)\, dv}{p-l+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv}$. Substituting them into the profit function, corresponding optimal total profit under this scenario

$$\begin{aligned} \begin{aligned} \pi _{DEP2}&=\Big [p-c+\eta (h-p)+(1-\eta )\int _p^h F(v)\, dv-\int _l^h F(v)\, dv\Big ]\mu _i\\&\quad +(p-c)\mu _u{\bar{F}}(p)-(p-s)\varphi (k){\bar{F}}(p)\sigma _u\sqrt{1-\rho ^2}. \end{aligned} \end{aligned}$$

(A.10)

It should be noted that optimal deposit expansion index $e^{'*}\le 1$ if retailer sets the DEP price equal to the optimal pre-order price under DP strategy, i.e., $X^{*}=m^{*}+l$. So we neglect this case.

1.4 Proof of Proposition 7

Let $\vec {x}=(m_F^{*}, X_F^{*}, e_F^{*})$ be a local maximum solution for the problem of pure optimization, its constraint is $\int _{X_F-e_Fm_F}^h F(v)\, dv-(e_F-1)m_F-\eta (h-X_F)-(1-\eta )\int _{X_F}^h F(v)\, dv\le 0$ for this model. Similarly, it exists a vector $\vec {r}=(r_1^{*}, r_2^{*}, r_3^{*}, r_4^{*})$, and its component $r_i^{*}\ge 0, i=1, 2, 3, 4$. It makes $\nabla f(\vec {x})-\sum _{i=1}^4 r_i^{*}\nabla g_i(\vec {x})=0$, $r_i^{*}g_i(\vec {x})=0$. Thus we have:

$$\begin{aligned} \begin{aligned}&\Big [1-e_F^{*}{\overline{F}}(X_F^{*}-e_F^{*}m_F^{*})+e_F^{*}(X_F^{*}-c-e_F^{*}m_F^{*})f(X_F^{*}-e_F^{*}m_F^{*})\Big ]\mu _i\\&\quad +r_1^{*}e_F^{*}{\overline{F}}(X_F^{*}-e_F^{*}m_F^{*})-r_1^{*}-r_2^{*}=0,\\&\Big [{\overline{F}}(X_F^{*}-e_F^{*}m_F^{*})-(X_F^{*}-c-e_F^{*}m_F^{*})f(X_F^{*}-e_F^{*}m_F^{*})\Big ]\mu _i+J\\&\quad +r_1^{*}F(X_F^{*}-e_F^{*}m_F^{*})-\eta r_1^{*}-(1-\eta )F(X_F^{*})r_1^{*}-r_3^{*}=0,\\&\Big [-m_F^{*}{\overline{F}}(X_F^{*}-e_F^{*}m_F^{*})+m_F^{*}(X_F^{*}-c-e_F^{*}m_F^{*})f(X_F^{*}-e_F^{*}m_F^{*})\Big ]\mu _i\\&\quad +r_1^{*}m_F^{*}{\overline{F}}(X_F^{*}-e_F^{*}m_F^{*})-r_4^{*}=0,\\&r_1^{*}\Big [\int _{X_F^{*}-e_F^{*}m_F^{*}}^{h} F(v)\, dv-(e_F^{*}-1)m_F^{*}-\eta (h-X_F^{*})-(1-\eta )\int _{X_F^{*}}^h F(v)\, dv\Big ]=0. \end{aligned} \end{aligned}$$

(A.11)

After calcution, we can obtain:

$$\begin{aligned} \begin{aligned}&r_1^{*}=\mu _i, r_2^{*}=r_3^{*}=r_4^{*}=0,\\&(1-\eta ){\bar{F}}(X_F^{*})\mu _i-(X_F^{*}-c-e_F^{*}m_F^{*})f(X_F^{*}-e_F^{*}m_F^{*}) +J=0,\\&e_F^{*}(X_F^{*}-c-e_F^{*}m_F^{*})f(X_F^{*}-e_F^{*}m_F^{*})=0, \end{aligned} \end{aligned}$$

(A.12)

where

$$\begin{aligned}&J=\Big [\mu _u-(\varphi (k)+(X_F^{*}-s)\frac{\partial {\varphi (k)}}{\partial {X_F^{*}}})\sigma _u\sqrt{1-\rho ^2}\Big ]{\bar{F}}(X_F^{*})\nonumber \\&\qquad +\Big [(X_F^{*}-s)\varphi (k)\sigma _u\sqrt{1-\rho ^2}-(X_F^{*}-c)\mu _u\Big ]f(X_F^{*}). \end{aligned}$$

(A.13)

So we can get

$$\begin{aligned} \begin{aligned}&X_{F1}^{*}=e_{F1}^{*}m_{F1}^{*}+c,\\&m_{F1}^{*}=X_{F1}^{*}-c+\eta (h-X_{F1}^{*})+(1-\eta )\int _{X_{F1}^{*}}^h F(v)\, dv-\int _c^h F(v)\, dv; \end{aligned} \end{aligned}$$

(A.14)

or

$$\begin{aligned} \begin{aligned}&X_{F2}^{*}=e_{F2}^{*}m_{F2}^{*}+l,\\&m_{F2}^{*}=X_{F2}^{*}-l+\eta (h-X_{F2}^{*})+(1-\eta )\int _{X_{F2}^{*}}^h F(v)\, dv-\int _l^h F(v)\, dv. \end{aligned} \end{aligned}$$

(A.15)

At last, corresponding optimal profits are as follows:

$$\begin{aligned} \pi _{F1}^{*}=m_{F1}^{*}\mu _i+(X_{F1}^{*}-c)\mu _u{\bar{F}}(X_{F1}^{*})-(X_{F1}^{*}-s)\varphi (k){\bar{F}}(X_{F1}^{*})\sigma _u\sqrt{1-\rho ^2}, \end{aligned}$$

(A.16)

or

$$\begin{aligned} \pi _{F2}^{*}=m_{F2}^{*}\mu _i+(X_{F2}^{*}-c)\mu _u{\bar{F}}(X_{F2}^{*})-(X_{F2}^{*}-s)\varphi (k){\bar{F}}(X_{F2}^{*})\sigma _u\sqrt{1-\rho ^2}. \end{aligned}$$

(A.17)