The influence of positive and negative salvage values on supply chain financing strategies

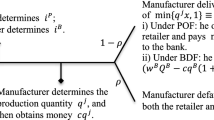
Abstract

We establish a supply chain finance scheme containing a cash-strapped supplier, a creditworthy retailer as well as a financial institution to explore whether the positive or negative salvage value has a crucial impact on the order decisions and financing strategies. Buyer-backed purchase order financing and advanced payment discount (APD) financing are considered to settle the supplier’s fund shortage problem. We found that the positive and negative salvage values affect (1) the retailer’s optimal order quantity. The buyer orders more products with positive salvage value than those with no salvage value and reduces orders for items with negative salvage value; (2) the profits in the supply chain. Ordering items with a positive salvage value can reduce the risk of loss compared to orders with no salvage value, which leads to more gains for the buyer and the whole supply chain, while orders for items with negative salvage increase the losses, resulting in lower profits; (3) the threshold of the retailer’s internal asset level under single financing. The higher salvage value brings more inventory risk to the retailer; hence the retailer should have a higher asset level to ensure that there is sufficient capital to finance the supplier via APD. Finally, we verify the results by numerical experiments and present some managerial implications for different industries.

Appendix

Proof of Theorem 1

From Eq. (4), the retailer's expected profit can be written as.

$$ \pi_{r1}^{{{\text{b}}c}} (q,w) = (p - s)\int_{0}^{q} {xf(x)dx + (p - s)\int_{q}^{\infty } {qf(x)dx - (w - s)q} } $$

(A1)

Taking the first-order and second-order partial derivative of q, we have

$$ \begin{gathered} \frac{{\partial \pi_{r1}^{bc} }}{\partial q} = (p - s)\overline{F}(q) - w + s \hfill \\ \frac{{\partial^{2} \pi_{r1}^{bc} }}{{\partial q^{2} }} = \frac{{\partial \{ (p - s)[1 - F(q)] - w + s\} }}{\partial q} = - (p - s)f(q) < 0 \hfill \\ \frac{{\partial^{2} \pi_{r1}^{bc} }}{\partial q\partial s} = 1 - \overline{F}(q) = F(q) > 0 \hfill \\ \end{gathered} $$

(A2)

Then, the first derivative with respect to s follows:

$$ \frac{dq}{{ds}} = \frac{{\partial^{2} \pi_{r1}^{bc} /\partial q\partial s}}{{ - \partial^{2} \pi_{r1}^{bc} /\partial q^{2} }} = \frac{F(q)}{{(p - s)f(q)}} > 0 $$

(A3)

Hence, $q_{1}^{bc} *$ and $\pi_{r1}^{{{\text{b}}c}}$ increase with the salvage value.

From the first-order condition: $\frac{{\partial \pi_{r}^{{{\text{b}}c}} }}{{\partial q_{1}^{bc*} }}{ = }0$, we derive $q_{1}^{{{\text{b}}c*}} = F^{ - 1} \left( {\frac{p - w}{{p - s}}} \right)$.

Proof of Theorem 2

Under BPOF, based on Eq. (6), the problem of the retailer can be expressed as:

$$ \begin{gathered} \pi_{r1}^{bpof} (q,w) = \left[\int_{0}^{q} {xf(x)dx + \int_{q}^{\infty } {qf(x)dx} } \right](p - s) \hfill \\ \, - q\Bigg[w - s + \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {(\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)\phi (A_{s} )dA_{s}\Bigg]} \hfill \\ \, - \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {(\delta \gamma c_{k} K - \delta \gamma A_{s} + \delta \gamma L_{s} )\phi (A_{s} )dA_{s} } \hfill \\ \end{gathered} $$

(A4)

Taking the first-order and second-order partial derivative of $\pi_{r1}^{bpof}$, we have

$$ \begin{gathered} \frac{{\partial \pi_{r1}^{bpof} }}{\partial q} = (p - s)\overline{F} (q) - [w - s + \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {(\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)\phi (A_{s} )dA_{s} ]} \hfill \\ \frac{{\partial^{2} \pi_{r1}^{bpof} }}{{\partial q^{2} }} = - (p - s)f(q) < 0 \hfill \\ \frac{{\partial^{2} \pi_{r1}^{bpof} }}{\partial q\partial s} = 1 - \overline{F}(q) = F(q) > 0 \hfill \\ \end{gathered} $$

(A5)

Accordingly,

$$ \frac{dq}{{ds}} = \frac{{\partial^{2} \pi_{r1}^{bpof} /\partial q\partial s}}{{ - \partial^{2} \pi_{r1}^{bpof} /\partial q^{2} }} = \frac{F(q)}{{(p - s)f(q)}} > 0 $$

(A6)

Hence,$q^{bc} *$ and $\pi_{r1}^{{{\text{bpof}}}}$ increase with the salvage value.

From the first-order condition:$\frac{{\partial \pi_{r1}^{bpof} }}{{\partial q^{bpof*} }} = 0$, we have

$$ \begin{gathered} (p - s)\bar{F}(q) = w - s + (\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)[\Phi (\tilde{A}_{S} ) - \Phi (\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{A} _{S} )] \hfill \\ \bar{F}(q) = \frac{{w - s + (\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)[\Phi (\tilde{A}_{S} ) - \Phi (\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{A} _{S} )]}}{{p - s}} \hfill \\ F(q) = 1 - \frac{{w - s + (\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)[\Phi (\tilde{A}_{S} ) - \Phi (\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{A} _{S} )]}}{{p - s}} \hfill \\ \qquad \,\,\,\,\, = \frac{{p - w - (\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)[\Phi (\tilde{A}_{S} ) - \Phi (\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{A} _{S} )]}}{{p - s}} \hfill \\ \end{gathered} $$

(A7)

Therefore, $q_{1}^{{bpof*}} = F^{{ - 1}} \left( {\frac{{p - w - (\delta \lambda w - \delta \gamma w + \delta \gamma c_{p} + \delta \gamma \lambda wr)\left[ {\Phi (\tilde{A}_{S} ) - \Phi (\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{A} _{S} )} \right]}}{{p - s}}} \right)$.

Proof of Theorem 3

Under APD, from Eq. (8),

$$ \begin{gathered} \pi_{r1}^{apd} (q,w) = (p - s)\int_{0}^{q} {xf(x)dx + (p - s)\int_{q}^{\infty } {qf(x)dx} } \hfill \\ \, - [(2 - \alpha )(1 - d)w - s]q - (1 - \alpha )[L_{r} - A_{r} ] \hfill \\ \end{gathered} $$

(A8)

We can obtain that

$$ \begin{gathered} \frac{{\partial \pi_{r1}^{apd} }}{\partial q} = (p - s)\overline{F} (q) - (2 - \alpha )(1 - d)w + s \hfill \\ \frac{{\partial^{2} \pi_{r1}^{apd} }}{{\partial q^{2} }} = - (p - s)f(q) < 0 \hfill \\ \frac{{\partial^{2} \pi_{r1}^{apd} }}{\partial q\partial s} = 1 - \overline{F}(q) = F(q) > 0 \hfill \\ \frac{dq}{{ds}} = \frac{F(q)}{{(p - s)f(q)}} > 0 \hfill \\ \end{gathered} $$

(A9)

Hence,$q^{bc} *$ and $\pi_{r1}^{{{\text{apd}}}}$ increase with the salvage value.

Proof of Proposition 1

In the case of reorganization, from Eq. (8) and the first-order condition:$\frac{{\partial \pi_{r1}^{apd} }}{{\partial q^{apd*} }} = 0$, we derive

$$ \begin{gathered} (p - s)\overline{F} (q) = (2 - \alpha )(1 - d)w - s \hfill \\ \overline{F} (q) = \frac{(2 - \alpha )(1 - d)w - s}{{p - s}} \hfill \\ F(q) = \frac{p - s - (2 - \alpha )(1 - d)w + s}{{p - s}} = \frac{p - (2 - \alpha )(1 - d)w}{{p - s}} \hfill \\ q_{1}^{apd*} = F^{ - 1} (\frac{p - (2 - \alpha )(1 - d)w}{{p - s}}) \hfill \\ \end{gathered} $$

(A10)

In the same way, we can also obtain $q_{1}^{apd*}$ in the case of continuation satisfies the first-order condition:

$$(p - s)\overline{F}\left( {q_{1}^{apd*} } \right) = w\left( {1 - d} \right) - s.$$

(A11)

Hence, $q_{1}^{apd*} = F^{ - 1} (\frac{p - (1 - d)w}{{p - s}})$.

To sum up, from the first-order condition:$\frac{{\partial \pi_{r1}^{apd} }}{{\partial q^{apd*} }} = 0$, we have

$\begin{gathered} q_{1}^{apd*} = F^{ - 1} (\frac{p - (1 - d)w}{{p - s}}){\text{ Continuation}} \hfill \\ q_{1}^{apd*} = F^{ - 1} (\frac{p - (2 - \alpha )(1 - d)w}{{p - s}}){\text{ Reorganization}} \hfill \\ \end{gathered}$.

Proof of Theorem 4

The financing strategy chosen by the retailer will bring a greater expected profit: $\pi_{r1}^{bpof*} > \pi_{r1}^{apd*}$ iff.

$$ \begin{gathered} p{\rm E}\min (D,q_{1}^{bpof*} ) - wq_{1}^{bpof*} + s(q_{1}^{bpof*} - D)^{ + } \hfill \\ \qquad - \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq_{1}^{bpof*} - \gamma \left[ {(w - c_{p} )q_{1}^{bpof*} - c_{k} K - \lambda wq_{1}^{bpof*} r + A_{s} - L_{s} } \right]} \right\}\phi (A_{s} )dA_{s} } \hfill \\ \quad > p{\rm E}\min (D,q_{1}^{apd*} ) - w(1 - d)q_{1}^{apd*} + s(q_{1}^{apd*} - D)^{ + } - (1 - \alpha )[L_{r} - A_{r} + w(1 - d)q_{1}^{apd*} ]^{ + } \hfill \\ \end{gathered} $$

(A12)

where the threshold value of the retailer's internal assets:

$$ \begin{gathered} \omega_{r1} { = }L_{r} + w\left( {1 - d} \right)q_{1}^{apd*} \hfill \\ \qquad -\,\frac{{p{\rm E}\min (D,q_{1}^{apd*} ) - w(1 - d)q_{1}^{apd*} + s(q_{1}^{apd*} - D)^{ + } - p{\rm E}\min (D,q_{1}^{bpof*} ){ + }wq_{1}^{bpof*} - s(q_{1}^{bpof*} - D)^{ + } }}{1 - \alpha } \hfill \\ \qquad +\, \frac{{\int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq_{1}^{bpof*} - \gamma \left[ {(w - c_{p} )q_{1}^{bpof*} - c_{k} K - \lambda wq_{1}^{bpof*} r + A_{s} - L_{s} } \right]} \right\}\phi (A_{s} )dA_{s} } }}{1 - \alpha } \hfill \\ \quad = L_{r} + w\left( {1 - d} \right)q_{1}^{apd*} \hfill \\ \qquad -\,\frac{{p{\rm E}\min (D,q_{1}^{apd*} ) - w(1 - d)q_{1}^{apd*} - p{\rm E}\min (D,q_{1}^{bpof*} ){ + }wq_{1}^{bpof*} + s[(q_{1}^{apd*} - D)^{ + } - (q_{1}^{bpof*} - D)^{ + } ]}}{1 - \alpha } \hfill \\ \qquad +\,\frac{{\int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq_{1}^{bpof*} - \gamma \left[ {(w - c_{p} )q_{1}^{bpof*} - c_{k} K - \lambda wq_{1}^{bpof*} r + A_{s} - L_{s} } \right]} \right\}\phi (A_{s} )dA_{s} } }}{1 - \alpha } \hfill \\ \end{gathered} $$

(A13)

Since there exist $s[(q_{1}^{apd*} - D)^{ + } - (q_{1}^{bpof*} - D)^{ + } ]$ and $q_{1}^{bpof*} > q_{1}^{apd*} > D$, the value of $\omega_{r1}$ is in direct proportion to the salvage value:

Proof of Theorem 5

From Eq. (12), in the case of reorganization, the retailer’s problem can be rewritten as.

$$ \begin{gathered} \pi_{r}^{df} \left( {q,w} \right) = (p - s)\int_{0}^{q} {xf(x)dx + (p - s)\int_{q}^{\infty } {qf(x)dx} } \hfill \\ \, - [\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]wq^{df} + sq^{df} - \left( {1 - \alpha } \right)(L_{r} - A_{r} ) \hfill \\ \end{gathered} $$

(A14)

$\omega_{r1} \left( {s > 0} \right){ > }\omega_{r0} \left( {s = 0, \, no \, salvage \, value} \right) > \omega_{r1} \left( {s < 0} \right)$.

The first and second derivatives of $\pi_{r1}^{bf}$ are

$$ \begin{gathered} \frac{{\partial \pi_{r}^{df} }}{\partial q} = (p - s)\overline{F} (q) - [\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]w + s \hfill \\ \frac{{\partial^{2} \pi_{r1}^{df} }}{{\partial q^{2} }} = - (p - s)f(q) < 0 \hfill \\ \frac{{\partial^{2} \pi_{r1}^{df} }}{\partial q\partial s} = 1 - \overline{F}(q) = F(q) > 0 \hfill \\ \end{gathered} $$

(A15)

Based on the above results, we derive the first-order derivative:

$$ \frac{dq}{{ds}} = \frac{{\partial^{2} \pi_{r1}^{df} /\partial q\partial s}}{{ - \partial^{2} \pi_{r1}^{df} /\partial q^{2} }} = \frac{F(q)}{{(p - s)f(q)}} > 0 $$

(A16)

Hence,$q^{{\text{df*}}}$ and $\pi_{r1}^{{{\text{df}}}}$ increase with the salvage value.

What’s more, in the case of continuation,

$$ \pi_{r}^{df} \left( {q,w} \right) = (p - s)\int_{0}^{q} {xf(x)dx + (p - s)\int_{q}^{\infty } {qf(x)dx} } - wq^{df} (1 - d{ + }d\beta ) + sq^{df} $$

(A17)

Then, we can obtain that

$$ \begin{gathered} \frac{{\partial \pi_{r1}^{df} }}{\partial q} = (p - s)\overline{F} (q) - w(1 - d{ + }d\beta ) + s \hfill \\ \frac{{\partial^{2} \pi_{r1}^{df} }}{{\partial q^{2} }} = - (p - s)f(q) < 0 \hfill \\ \frac{{\partial^{2} \pi_{r1}^{df} }}{\partial q\partial s} = 1 - \overline{F}(q) = F(q) > 0 \hfill \\ \frac{dq}{{ds}} = \frac{F(q)}{{(p - s)f(q)}} > 0 \hfill \\ \end{gathered} $$

(A18)

Hence, in both cases of reorganization and continuation,$q^{{\text{df*}}}$ and $\pi_{r1}^{{{\text{df}}}}$ increase with the salvage value.

Proof of Proposition 2

In the case of reorganization, from Eq. (12), when $\frac{{\partial \pi_{r}^{df} }}{\partial q} = 0$, we have

$$ \begin{gathered} {}[\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]w - s = (p - s)\overline{F} (q^{df} ) \hfill \\ \overline{F} (q^{df} ) = \frac{[\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]w - s}{{p - s}} \hfill \\ F(q^{df} ) = \frac{p - [\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]w}{{p - s}} \hfill \\ \end{gathered} $$

(A19)

Thus,$q^{{df{*}}} = F^{ - 1} (\frac{p - [\alpha - \alpha \beta - \alpha d + \alpha d\beta + \beta ]w}{{p - s}})$ in reorganization.

If the retailer does not have financial difficulties, it follows that:

$(p - s)\overline{F} (q^{df} ){ = }[1 - d + d\beta ]w - s$.

Therefore,$q^{{df{*}}} = F^{ - 1} (\frac{p - [1 - d + d\beta ]w}{{p - s}})$ in continuation.

Profits without considering salvage value In the deconcentrated benchmark without salvage value,

$$\pi_{{{\text{r0}}}}^{dsc} { = }p{\rm E}\min [D,\min (q,K)] - w\min (q,K).$$

(A20)

$$\pi_{{s{0}}}^{dsc} { = }(w - c_{p} )\min (q,K) - c_{k} K.$$

(A21)

$$\pi_{{s{\text{c0}}}}^{dsc} = p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K) - c_{k} K.$$

(A22)

In base case,

$$ \pi_{r0}^{bc} (q,w) = p{\rm E}\min [D,\min (q,K)] - w\min (q,K) $$

(A23)

$$ \begin{aligned} & \pi_{r0}^{bc} (q,w) = p{\rm E}\min [D,\min (q,K)] - w\min (q,K)\\ & \pi_{s}^{bc} (K,w) = \left\{ {\begin{array}{*{20}l} {(w - c_{p} )\min (q,K) - c_{k} K} \\ {(w - c_{p} )\min (q,K) - c_{k} K - (1 - \alpha )(L_{s} - A_{s} + c_{k} K)} \\ 0 \\ \end{array} } \right.\begin{array}{*{20}c} {{\text{Continuation}}} \\ {\text{ Reorganization}} \\ {{\text{Liquidation}}} \\ \end{array}\end{aligned} $$

(A24)

$$ \pi_{{{\text{sc0}}}}^{bc} = \left\{ \begin{gathered} p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K) - c_{k} K \hfill \\ p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K) - c_{k} K \hfill \\ - (1 - \alpha )(L_{s} - A_{s} + c_{k} K) \hfill \\ \end{gathered} \right.\begin{array}{*{20}c} {{\text{Continuation}}} \\ {\text{ Reorganization}} \\ {} \\ \end{array} $$

(A25)

In BPOF,

$$ \begin{gathered} \pi_{r0}^{bpof} (q,w) = p{\rm E}\min [D,\min (q,K)] - w\min (q,K) - \hfill \\ \, \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq - \gamma \left[ \begin{gathered} (w - c_{p} )\min (q,K) - c_{k} K - \hfill \\ - \lambda wqr + A_{s} - L_{s} \hfill \\ \end{gathered} \right]} \right\}\phi (A_{s} )dA_{s} } \hfill \\ \end{gathered} $$

(A26)

$$ \pi_{s0}^{bpof} (\lambda ,K,w) = \left\{ {\begin{array}{*{20}c} {(w - c_{p} )\min (q,K) - c_{k} K - \lambda wqr} \\ \begin{gathered} (w - c_{p} )\min (q,K) - c_{k} K - \hfill \\ (1 - \alpha )(L_{s} - A_{s} - \lambda wq + c_{k} K) - \lambda wqr \hfill \\ \end{gathered} \\ 0 \\ \end{array} } \right.\begin{array}{*{20}c} {{\text{Continuation}}} \\ {{\text{Reorganization}}} \\ {} \\ {{\text{Liquidation}}} \\ \end{array} $$

(A27)

$$ \pi_{{s{\text{c}}0}}^{bpof} = \left\{ \begin{gathered} p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K) - c_{k} K - \lambda wqr \hfill \\ - \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq - \gamma \left[ \begin{gathered} (w - c_{p} )\min (q,K) - c_{k} K \hfill \\ - \lambda wqr + A_{s} - L_{s} \hfill \\ \end{gathered} \right]} \right\}\phi (A_{s} )dA_{s} {\text{ Continuation}}} \hfill \\ p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K) - c_{k} K - \lambda wqr \hfill \\ - (1 - \alpha )(L_{s} - A_{s} - \lambda wq + c_{k} K) \hfill \\ - \int\limits_{{\underline {A}_{S} }}^{{\tilde{A}_{S} }} {\delta \left\{ {\lambda wq - \gamma \left[ \begin{gathered} (w - c_{p} )\min (q,K) - c_{k} K \hfill \\ - \lambda wqr + A_{s} - L_{s} \hfill \\ \end{gathered} \right]} \right\}\phi (A_{s} )dA_{s} } {\text{ Reorganization}} \hfill \\ \end{gathered} \right. $$

(A28)

In APD,

$$ \pi_{s0}^{apd} (\lambda ,K,w) = \left\{ {\begin{array}{*{20}c} {[w(1 - d) - c_{p} ]\min (q,K) - c_{k} K} \\ \begin{gathered} [w(1 - d) - c_{p} ]\min (q,K) - c_{k} K \hfill \\ - (1 - \alpha )(L_{s} - A_{s} + c_{k} K - w(1 - d)q) \hfill \\ \end{gathered} \\ 0 \\ \end{array} } \right.\begin{array}{*{20}c} {{\text{Continuation}}} \\ {\text{ Reorganization}} \\ {{\text{Liquidation}}} \\ \end{array} $$

(A29)

$$ \begin{gathered} \pi_{r0}^{apd} (q,w) = p{\rm E}\min [D,\min (q,K)] - w(1 - d)\min (q,K) \hfill \\ \, - (1 - \alpha )[L_{r} - A_{r} + w(1 - d)q]^{ + } \hfill \\ \end{gathered} $$

(A30)

$$ \pi_{sc0}^{apd} = \left\{ \begin{gathered} p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K){\text{ Continuation}} \hfill \\ - c_{k} K - (1 - \alpha )[L_{r} - A_{r} + w(1 - d)q]^{ + } \hfill \\ p{\rm E}\min [D,\min (q,K)] - c_{p} \min (q,K){\text{ Reorganization}} \hfill \\ - c_{k} K - (1 - \alpha )[L_{r} - A_{r} ]^{ + } - (1 - \alpha )(L_{s} - A_{s} + c_{k} K) \hfill \\ \end{gathered} \right. $$

(A31)