Abstract
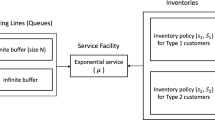
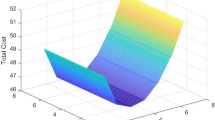
A two-commodity queueing-inventory system with phase-type service times and exponential lead times is considered. There are two types of customers; Type 1 and Type 2. Demands from each customer type occur independently according to a Poisson process with different rates whereas the service times follow a phase-type distribution. Type 1 customers have a non-preemptive priority over Type 2 customers. We assume a finite waiting space for Type 1 customers whereas there is no limit on the waiting room for Type 2 customers. Type i customers demand only commodity i, \(i=1,2\). For the ith commodity, \(S_i\) and \(s_i\) represent, respectively, the maximum inventory level and the reorder level. Whenever the inventory level of ith commodity drops to \(s_i\), an order is placed from retailer-i to make the inventory level \(S_i\). The lead times of the commodities are exponentially distributed with different parameters. When there is a Type i customer waiting in the queue, if the inventory level of ith commodity is zero (or reaches zero), a decision of immediate purchase is made so as not to lose the waiting customer. The queueing-inventory model in the steady-state is analyzed using the matrix-geometric method. The system performance is examined for different values of parameters. Besides, an optimization study is performed for some system parameters.







Similar content being viewed by others
References
Amirthakodi, M., Radhamani, V., & Sivakumar, B. (2015). A perishable inventory system with service facility and feedback customers. Annals of Operations Research, 233, 25–55. https://doi.org/10.1007/s10479-015-1821-9
Anbazhagan, N., & Arivarignan, G. (2000). Two commodity continuous review inventory system with coordinated reorder policy. Information and Management Sciences, 11(3), 19–30.
Anbazhagan, N., & Arivarignan, G. (2001). Analysis of two commodity Markovian iventory system with lead time. Korean Journal of Computational and Applied Mathematics, 8(2), 427–438. https://doi.org/10.1007/BF02941976
Anbazhagan, N., Arivarignan, G., & Irle, A. (2012). A two-commodity continuous review inventory system with substitutable items. Stochastic Analysis and Applications, 30(1), 1–19. https://doi.org/10.1080/07362994.2012.628905
Anbazhagan, N., Goh, M., & Vigneshwaran, B. (2015). Substitutable inventory systems with coordinated reorder levels. Journal of Statistics Applications and Probability, 2(3), 221–234.
Anbazhagan, N., & Jeganathan, K. (2013). Two-commodity Markovian inventory system with compliment and retrial demand. British Journal of Mathematics and Computer Science, 3(2), 115–134.
Anbazhagan, N., & Kumaresan, V. (2012). Coordinated and joint ordering policies for two commodity inventory system. Iranian Journal of Operations Research, 3(2), 94–105.
Anbazhagan, N., & Vigneshwaran, B. (2010). Two-commodity Markovian inventory system with set of reorders. International Journal of Information Systems and Supply Chain Management, 3(2), 52–67.
Balintfy, J. L. (1964). On a basic class of multi-item inventory problems. Management Science, 10(2), 287–297. https://doi.org/10.1287/mnsc.10.2.287
Benny, B., Chakravarthy, S. R., & Krishnamoorthy, A. (2018). Queueing-inventory system with two commodities. Journal of the Indian Society for Probability and Statistics, 19, 437–454. https://doi.org/10.1007/s41096-018-0052-1
Chakravarthy, S. R. (2020). Queueing-inventory models with batch demands and positive service times. Automation and Remote Control, 81(4), 713–730.
Chakravarthy, S. R., & Dudin, A. N. (2017). A queueing model for crowdsourcing. Journal of the Operational Research Society, 68, 221–236. https://doi.org/10.1057/s41274-016-0099-x
Chakravarthy, S. R., Maity, A., & Gupta, U. C. (2017). An \(^{\prime }(s, S)^{\prime }\) inventory in a queueing system with batch service facility. Annals of Operations Research, 258, 263–283. https://doi.org/10.1007/s10479-015-2041-z
Chakravarthy, S. R., & Rumyantsev, A. (2020). Analytical and simulation studies of queueing-inventory models with MAP demands in batches and positive phase type services. Simulation Modelling Practice and Theory, 103, 1–15.
Deepak, T. G., Krishnamoorthy, A., Narayanan, V. C., & Vineetha, K. (2008). Inventory with service time and transfer of customers and/inventory. Annals of Operations Research, 160, 191–213. https://doi.org/10.1007/s10479-007-0304-z
Federgruen, A., Groenevelt, H., & Tijms, H. C. (1984). Coordinated replenishments in a multi-item inventory system with compound Poisson demands. Management Science, 30(3), 344–357. https://doi.org/10.1287/mnsc.30.3.344
Gomathi, D., Jeganathan, K., & Anbazhagan, N. (2012). Two-commodity inventoory system for base-stock policy with service facility. Global Journal of Science Frontier Research Mathematics and Decision Sciencies, 12(1), 69–80.
Jeganathan, K. (2014). Two commodity perishable inventory system with negative and impatient customers, postponed demands and a finite population. International Journal of Applied Sciences and Engineering Research, 3(1), 265–280.
Jeganathan, K., Venkatesan, T., Anbazhagan, N., Padmasekaran, S., & Lakshmanan, K. (2018). Substitutable invventory model with postponed demands. International Journal of Pure and Applied Mathematics, 3(1), 265–280.
Kalpakam, S., & Arivarignan, G. (1993). A coordinated multicommodity \(({S}, s)\) inventory system. Mathematical and Computer Modelling, 18(11), 69–73. https://doi.org/10.1016/0895-7177(93)90206-E
Karthick, T., Sivakumar, B., & Arivarignan, G. (2015). An inventory system with two types of customers and retrial demands. International Journal of Systems Science: Operations and Logistics, 2(2), 90–112. https://doi.org/10.1080/23302674.2014.1001004
Karthikeyan, K., & Sudhesh, R. (2016). Recent review article on queueing inventory systems. Research Journal of Pharmacy and Technology, 9(11), 1451–1461.
Krishnamoorthy, A., Iqbal Basha, R., & Lakshmy, B. (1994). Analysis of a two commodity inventory problem. Information and Management Sciences, 5(1), 127–136.
Krishnamoorthy, A., Lakshmy, B., & Manikandan, R. (2011). A survey on inventory models with positive service time. OPSEARCH, 48, 153–169. https://doi.org/10.1007/s12597-010-0032-z
Krishnamoorthy, A., Manikandan, R., & Lakshmy, B. (2015). A revisit to queueing-inventory system with positive service time. Annals of Operations Research, 233, 221–236. https://doi.org/10.1007/s10479-013-1437-x
Krishnamoorthy, A., Shajin, D., & Lakshmy, B. (2016). On a queueing-inventory with reservation, cancellation, common life time and retrial. Annals of Operations Research, 247, 365–389. https://doi.org/10.1007/s10479-015-1849-x
Krishnamoorthy, A., Shajin, D., & Narayanan, V. C. (2020). Inventory with positive service time: a survey. In V. Anisimov & N. Limnios (Eds.), Queueing theory 2- Advanced trends (pp. 201–238). London: ISTE & J. Wiley.
Krishnamoorthy, A., & Varghese, T. V. (1994). A two commodity inventory problem. Information and Management Sciences, 5(3), 55–70.
Latouche, G., & Ramaswami, V. (1999). Introduction to matrix analytic methods in stochastic modeling. Philadelphia: SIAM.
Liu, M., Feng, M., & Wong, C. Y. (2014). Flexible service policies for a Markov inventory system with two demand classes. International Journal of Production Economics, 151, 180–185.
Manuel, P., Sivakumar, B., & Arivarignan, G. (2007). A perishable inventory system with service facilities, MAP arrivals and PH-Service times. Journal of Systems Science and Systems Engineering, 16, 62–73. https://doi.org/10.1007/s11518-006-5025-3
Melikov, A. Z., Ponomarenko, L. A., & Bagirova, S. A. (2017). Markov models of queueing-inventory systems with variable order size. Cybernetics and Systems Analysis, 53(3), 373–386.
Nair, A. N., Jacob, M. J., & Krishnamoorthy, A. (2015). The multi server M/M/(s, S) queueing inventory system. Annals of Operations Research, 233, 321–333. https://doi.org/10.1007/s10479-013-1405-5
Neuts, M. F. (1981). Matrix-geometric solutions in stochastic models: An algorithmic approach. Baltimore, MD: The Johns Hopkins University Press [1994 version is Dover Edition].
Ozkar, S., & Uzunoglu Kocer, U. (2021). Two-commodity queueing-inventory system with two classes of customers. OPSEARCH, 58, 234–256. https://doi.org/10.1007/s12597-020-00479-0
Razumchik, R., & Telek, M. (2016). Delay analysis of a queue with re-sequencing buffer and Markov environment. Queueing Systems, 82, 7–28. https://doi.org/10.1007/s11134-015-9444-z
Sapna Isotupa, K. P. (2006). An \((s,{Q})\) Markovian inventory system with lost sales and two demand classes. Mathematical and Computer Modelling, 43(7–8), 687–694. https://doi.org/10.1016/j.mcm.2005.09.027
Sapna Isotupa, K. P. (2011). An \((s,{Q})\) inventory system with two classes of customers. International Journal of Operational Research, 12(1), 1–19. https://doi.org/10.1504/IJOR.2011.041856
Sapna Isotupa, K. P. (2015). Cost analysis of an \((S-1, S)\) inventory system with two demand classes and rationing. Annals of Operations Research, 233, 411–421. https://doi.org/10.1007/s10479-013-1407-3
Sapna Isotupa, K. P., & Samanta, S. K. (2013). A continuous review \((s,{Q})\) inventory system with priority customers and arbitrarily distributed lead times. Mathematical and Computer Modelling, 57(5–6), 1259–1269. https://doi.org/10.1016/j.mcm.2012.10.029
Schwarz, M., Sauer, C., Daduna, H., Kulik, R., & Szekli, R. (2006). M/M/1 queueing systems with inventory. Queueing Systems, 54, 55–78. https://doi.org/10.1007/s11134-006-8710-5
Shajin, D., Jacob, J., & Krishnamoorthy, A. (2021). On a queueing inventory problem with necessary and optional inventories. Annals of Operations Research. https://doi.org/10.1007/s10479-021-03975-8.
Silver, E. A. (1974). A control system for coordinated inventory replenishment. International Journal of Production Research, 12(6), 647–671. https://doi.org/10.1080/00207547408919583
Sivakumar, B. (2008). Two-commodity inventory system with retrial demand. European Journal of Operational Research, 187(1), 70–83. https://doi.org/10.1016/j.ejor.2007.02.036
Sivakumar, B., Anbazhagan, N., & Arivarignan, G. (2005). A two commodity perishable inventory system. Orion, 21(2), 157–172. https://doi.org/10.5784/21-2-27
Sivakumar, B., Anbazhagan, N., & Arivarignan, G. (2006). Two commodity continuous review perishable inventory system. Information and Management Sciences, 17(3), 47–64.
Sivakumar, B., Anbazhagan, N., & Arivarignan, G. (2007). Two-commodity inventory system with individual and joint ordering policies and renewal demands. Stochastic Analysis and Applications, 25(6), 1217–1241. https://doi.org/10.1080/07362990701567314
Sivakumar, B., & Arivarignan, G. (2008). A modified lost sales inventory system with two types of customers. Quality Technology and Quantitative Management, 5(4), 339–349. https://doi.org/10.1080/16843703.2008.11673406
Sivasamy, R., & Pandiyan, P. (1998). A two commodity inventory model under \((s_k,{S}_k)\) policy. Information and Management Sciences, 9(3), 19–34.
Yadavalli, V. S. S., Adetunji, O., Sivakumar, B., & Arivarignan, G. (2010). Two-commodity perishable inventory system with bulk demand for one commodity. The South African Journal of Industrial Engineering, 21(1), 137–155. https://doi.org/10.7166/21-1-73
Yadavalli, V. S. S., Anbazhagan, N., & Arivarignan, G. (2004). A two commodity stochastic inventory system with lost sales. Stochastic Analysis and Applications, 22(2), 479–497. https://doi.org/10.1081/SAP-120028606
Yadavalli, V. S. S., Arivarignan, G., & Anbazhagan, N. (2006). Two commodity coordinated inventory system with Markovian demand. Asia-Pacific Journal of Operational Research, 23(4), 497–508. https://doi.org/10.1142/S0217595906001005
Zhao, N., & Lian, Z. (2011). A queueing-inventory system with two classes of customers. International Journal of Production Economics, 129(1), 225–231. https://doi.org/10.1016/j.ijpe.2010.10.011
Acknowledgements
No funding was received for conducting this study.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The author have no competing interests to declare that are relevant to the content of this article.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Ozkar, S. Two-commodity queueing-inventory system with phase-type distribution of service times. Ann Oper Res 331, 711–737 (2023). https://doi.org/10.1007/s10479-022-04865-3
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-022-04865-3
Keywords
- Queueing-inventory
- Two-commodity
- Two types of customers
- Lead time
- Matrix geometric method
- Phase-type distribution