Skip to main content
Springer Nature Link
Log in

An improved normal wiggly hesitant fuzzy FMEA model and its application to risk assessment of electric bus systems

  • Published:
Applied Intelligence Aims and scope Submit manuscript

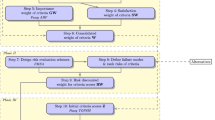
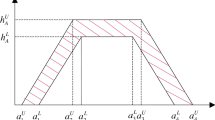
Abstract

The highly dynamic nature of the real-world environment poses significant challenges for electric bus system operations (EBSOs), which are prone to serious accidents due to their complexity and a wide variety of risk factors. The accidents are often the result of ignoring the most serious risk sources because of a lack of comprehensive risk assessments. Therefore, this paper proposes an improved failure mode and effects analysis (FMEA) multicriteria group decision-making model to ensure the reliability and safety of EBSOs. First, an expert group is invited to evaluate the risk failure modes (FMs) of the EBSOs and transform them into a normal wiggly hesitant fuzzy set (NWHFS) form. Because the risk assessment process involves a large number of team members with different backgrounds, the experts are grouped based on scoring function values using the K-medoids clustering technique. Then, the evaluation values of the expert group are integrated using the normal hesitant fuzzy weighted geometric (NWHFWG) aggregation operator to obtain the final aggregation matrix, and the weights of the three criteria of occurrence (O), severity (S) and detection (D) are determined for each FM via the CCSD method. Finally, considering the cross-correlation between factors within the system, the relationships between FMs are analyzed, and their impact and importance are quantified using the gray correlation-based DEMATEL method, followed by the final ranking of the FMs using regret theory and the PROMETHEE II methodology to achieve a rational allocation of resources. The results are analyzed with sensitivity and comparative analyses to illustrate the superiority of the model.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

The code of the current study is available from the corresponding author upon reasonable request.

References

  1. Manzolli JA, Trovão JP, Antunes CH (2022) A review of electric bus vehicles research topics -Methods and trends. Renew Sust Energ Rev 159:112211. https://doi.org/10.1016/j.rser.2022.112211

    Article  Google Scholar 

  2. Martínez-Maldonado V, Barragán-Escandón A, Serrano-Guerrero X, Zalamea-Leon EF (2023) Optimal routing for mass transit systems using multicriteria methodologies. Energy Strateg Rev 47:101077. https://doi.org/10.1016/j.esr.2023.101077

    Article  Google Scholar 

  3. Luo X, Fan W (2023) Joint design of electric bus transit service and wireless charging facilities. Transp Res E Logist Transp Rev 174:103114. https://doi.org/10.1016/j.tre.2023.103114

    Article  MathSciNet  Google Scholar 

  4. Wang G, Fang Z, Xie X et al (2021) Pricing-aware Real-time Charging Scheduling and Charging Station Expansion for Large-scale Electric Buses. Acm T Intel Syst Tec 12:1–26. https://doi.org/10.1145/3428080

    Article  Google Scholar 

  5. Thorne RJ, Hovi IB, Figenbaum E et al (2021) Facilitating adoption of electric buses through policy: Learnings from a trial in Norway. Energy Policy 155:112310. https://doi.org/10.1016/j.enpol.2021.112310

    Article  Google Scholar 

  6. Zhao L, Ke H, Li Y, Chen Y (2023) Research on personalized charging strategy of electric bus under time-varying constraints. Energy 276:127584. https://doi.org/10.1016/j.energy.2023.127584

    Article  Google Scholar 

  7. Avenali A, Catalano G, Giagnorio M, Matteucci G (2023) Assessing cost-effectiveness of alternative bus technologies: Evidence from US transit agencies. Transp Res D Transp Environ 117:103648. https://doi.org/10.1016/j.trd.2023.103648

    Article  Google Scholar 

  8. Tessler ME, Traut EJ (2022) Hurricane resiliency methods for the New York City electric bus fleet. Transp Res D Transp Environ 105:103255. https://doi.org/10.1016/j.trd.2022.103255

    Article  Google Scholar 

  9. Yazdani M, Mohammed A, Bai C, Labib A (2021) A novel hesitant-fuzzy-based group decision approach for outsourcing risk. Expert Syst Appl 184:115517. https://doi.org/10.1016/j.eswa.2021.115517

    Article  Google Scholar 

  10. Bowles JB, Peláez CE (1995) Fuzzy logic prioritization of failures in a system failure mode, effects and criticality analysis. Reliab Eng Syst Safe 50:203–213. https://doi.org/10.1016/0951-8320(95)00068-D

    Article  Google Scholar 

  11. Ren Z, Xu Z, Wang H (2018) Normal wiggly hesitant fuzzy sets and their application to environmental quality evaluation. Knowl-Based Syst 159:286–297. https://doi.org/10.1016/j.knosys.2018.06.024

    Article  Google Scholar 

  12. Wang Y-M, Luo Y (2010) Integration of correlations with standard deviations for determining attribute weights in multiple attribute decision making. Math Comput Model 51:1–12. https://doi.org/10.1016/j.mcm.2009.07.016

    Article  MathSciNet  Google Scholar 

  13. Gou X, Xu Z, Liao H, Herrera F (2021) Consensus Model Handling Minority Opinions and Noncooperative Behaviors in Large-Scale Group Decision-Making Under Double Hierarchy Linguistic Preference Relations. IEEE Trans Cybern 51:283–296. https://doi.org/10.1109/TCYB.2020.2985069

    Article  Google Scholar 

  14. Liu Z, Bi Y, Liu P (2022) An evidence theory-based large group FMEA framework incorporating bounded confidence and its application in supercritical water gasification system. Appl Soft Comput 129:109580. https://doi.org/10.1016/j.asoc.2022.109580

    Article  Google Scholar 

  15. Liang R, Xue Z, Chong H-Y (2023) Risk Evaluation of Logistics Park Projects’ Lifecycle during the COVID-19 Pandemic: Failure Mode and Effects Analysis. J Constr Eng M 149:04022153. https://doi.org/10.1061/(ASCE)CO.1943-7862.0002430

    Article  Google Scholar 

  16. Yazdi M, Nedjati A, Zarei E, Abbassi R (2020) A reliable risk analysis approach using an extension of best-worst method based on democratic-autocratic decision-making style. J Clean Prod 256:120418. https://doi.org/10.1016/j.jclepro.2020.120418

    Article  Google Scholar 

  17. Bell DE (1982) Regret in Decision Making under Uncertainty. Oper Res 30:961–981. https://doi.org/10.1287/opre.30.5.961

    Article  Google Scholar 

  18. Sun J, Liu Y, Xu J et al (2023) A probabilistic uncertain linguistic FMEA model based on the extended ORESTE and regret theory. Comput Ind Eng 180:109251. https://doi.org/10.1016/j.cie.2023.109251

    Article  Google Scholar 

  19. Perumal SSG, Lusby RM, Larsen J (2022) Electric bus planning & scheduling: A review of related problems and methodologies. Eur J Oper Res 301:395–413. https://doi.org/10.1016/j.ejor.2021.10.058

    Article  MathSciNet  Google Scholar 

  20. Deveci M, Torkayesh AE (2023) Charging Type Selection for Electric Buses Using Interval-Valued Neutrosophic Decision Support Model. IEEE Trans Eng Manag 70:4249–4262. https://doi.org/10.1109/TEM.2021.3108062

    Article  Google Scholar 

  21. Wu W, Lin Y, Liu R, Jin W (2022) The multi-depot electric vehicle scheduling problem with power grid characteristics. Transport Res B-Meth 155:322–347. https://doi.org/10.1016/j.trb.2021.11.007

    Article  Google Scholar 

  22. Zhang L, Wang S, Qu X (2021) Optimal electric bus fleet scheduling considering battery degradation and non-linear charging profile. Transport Res E Logist Transp Rev 154:102445. https://doi.org/10.1016/j.tre.2021.102445

    Article  Google Scholar 

  23. Taha HA, Yacout S, Shaban Y (2023) Online failure analysis and autonomous risk control scheme for electric buses. Eng Fail Anal 154:107629. https://doi.org/10.1016/j.engfailanal.2023.107629

    Article  Google Scholar 

  24. Akram M, Zahid S, Deveci M (2024) Enhanced CRITIC-REGIME method for decision making based on Pythagorean fuzzy rough number. Expert Syst Appl 238:122014. https://doi.org/10.1016/j.eswa.2023.122014

    Article  Google Scholar 

  25. He Y, Liu Z, Song Z (2022) Integrated charging infrastructure planning and charging scheduling for battery electric bus systems. Transp Res D Transp Environ 111:103437. https://doi.org/10.1016/j.trd.2022.103437

    Article  Google Scholar 

  26. Bahrami M, Vakilian M, Farzin H, Lehtonen M (2023) A CVaR-based stochastic framework for storm-resilient grid, including bus charging stations. Sustain Energy Grids 35:101082. https://doi.org/10.1016/j.segan.2023.101082

    Article  Google Scholar 

  27. Wu C, Wang T, Zhou D et al (2023) A distributed restoration framework for distribution systems incorporating electric buses. Appl Energy 331:120428. https://doi.org/10.1016/j.apenergy.2022.120428

    Article  Google Scholar 

  28. Li X, Li Y, Liu W, Yuan Y (2024) Optimal design of pure battery electric bus system on the grid network. Transportmetrica A 20:2152298. https://doi.org/10.1080/23249935.2022.2152298

    Article  Google Scholar 

  29. Chin K-S, Wang Y-M, Poon GKK, Yang J-B (2009) Failure mode and effects analysis by data envelopment analysis. Decis Support Syst 48:246–256. https://doi.org/10.1016/j.dss.2009.08.005

    Article  Google Scholar 

  30. Liu Z, Zhao Y, Liu P (2023) An integrated FMEA framework considering expert reliability for classification and its application in aircraft power supply system. Eng Appl Artif Intell 123:106319. https://doi.org/10.1016/j.engappai.2023.106319

    Article  Google Scholar 

  31. Kowal K, Torabi M (2021) Failure mode and reliability study for Electrical Facility of the High Temperature Engineering Test Reactor. Reliab Eng Syst Saf 210:107529. https://doi.org/10.1016/j.ress.2021.107529

    Article  Google Scholar 

  32. Wu C, Lin Y, Barnes D (2021) An integrated decision-making approach for sustainable supplier selection in the chemical industry. Expert Syst Appl 184:115553. https://doi.org/10.1016/j.eswa.2021.115553

    Article  Google Scholar 

  33. Ghadir AH, Vandchali HR, Fallah M, Tirkolaee EB (2022) Evaluating the impacts of COVID-19 outbreak on supply chain risks by modified failure mode and effects analysis: a case study in an automotive company. Ann Oper Res. https://doi.org/10.1007/s10479-022-04651-1

  34. Jiang J, Liu X, Wang W, Deveci M (2023) Assessing the impact of healthcare service risks on healthcare demand under evolving economic and social structures: An improved GLDS decision making method considering risk attitudes. Struct Change Econ Dynam 67:459–479. https://doi.org/10.1016/j.strueco.2023.09.002

    Article  Google Scholar 

  35. Shao J, Zhong S, Tian M, Liu Y (2024) Combining fuzzy MCDM with Kano model and FMEA: a novel 3-phase MCDM method for reliable assessment. Ann Oper Res. https://doi.org/10.1007/s10479-024-05878-w

  36. Zhu G-N, Ma J, Hu J (2022) A fuzzy rough number extended AHP and VIKOR for failure mode and effects analysis under uncertainty. Adv Eng Inf 51:101454. https://doi.org/10.1016/j.aei.2021.101454

    Article  Google Scholar 

  37. Zhang H, Liu S, Dong Y et al (2023) A Minimum Cost Consensus-Based Failure Mode and Effect Analysis Framework Considering Experts’ Limited Compromise and Tolerance Behaviors. IEEE Trans Cybern 53:6612–6625. https://doi.org/10.1109/TCYB.2022.3212093

    Article  Google Scholar 

  38. Liang D, Li F, Chen X (2023) Failure mode and effect analysis by exploiting text mining and multi-view group consensus for the defect detection of electric vehicles in social media data. Ann Oper Res. https://doi.org/10.1007/s10479-023-05649-z

  39. Gou X, Xu Z, Zhou W, Herrera-Viedma E (2021) The risk assessment of construction project investment based on prospect theory with linguistic preference orderings. Econ Res-Ekon Istraz 34:709–731. https://doi.org/10.1080/1331677X.2020.1868324

    Article  Google Scholar 

  40. Zhang P, Zhang Z-J, Gong D-Q (2024) An improved failure mode and effect analysis method for group decision-making in utility tunnels construction project risk evaluation. Reliab Eng Syst Saf 244:109943. https://doi.org/10.1016/j.ress.2024.109943

    Article  Google Scholar 

  41. Lin S-W, Lo H-W (2023) An FMEA model for risk assessment of university sustainability: using a combined ITARA with TOPSIS-AL approach based neutrosophic sets. Ann Oper Res.https://doi.org/10.1007/s10479-023-05250-4

  42. Garg A, Das S, Maiti J, Pal SK (2022) Granulized Z-VIKOR Model for Failure Mode and Effect Analysis. IEEE Trans Fuzzy Syst 30:297–309. https://doi.org/10.1109/TFUZZ.2020.3037933

    Article  Google Scholar 

  43. Akram M, Zahid K, Kahraman C (2024) A new ELECTRE-based decision-making framework with spherical fuzzy information for the implementation of autonomous vehicles project in Istanbul. Knowl-Based Syst 283:111207. https://doi.org/10.1016/j.knosys.2023.111207

    Article  Google Scholar 

  44. Akram M, Ilyas F, Deveci M (2024) Interval rough integrated SWARA-ELECTRE model: An application to machine tool remanufacturing. Expert Syst Appl 238:122067. https://doi.org/10.1016/j.eswa.2023.122067

    Article  Google Scholar 

  45. Hua Z, Jing X, Martínez L (2023) An ELICIT information-based ORESTE method for failure mode and effect analysis considering risk correlation with GRA-DEMATEL. Inf Fusion 93:396–411. https://doi.org/10.1016/j.inffus.2023.01.012

    Article  Google Scholar 

  46. Chen Y, Ran Y, Huang G et al (2021) A new integrated MCDM approach for improving QFD based on DEMATEL and extended MULTIMOORA under uncertainty environment. Appl Soft Comput 105:107222. https://doi.org/10.1016/j.asoc.2021.107222

    Article  Google Scholar 

  47. Luthra S, Sharma M, Kumar A et al (2022) Overcoming barriers to cross-sector collaboration in circular supply chain management: a multi-method approach. Transp Res E Logist Transp Rev 157:102582. https://doi.org/10.1016/j.tre.2021.102582

    Article  Google Scholar 

  48. Liu Z, Bi Y, Liu P (2023) A conflict elimination-based model for failure mode and effect analysis: A case application in medical waste management system. Comput Ind Eng 178:109145. https://doi.org/10.1016/j.cie.2023.109145

    Article  Google Scholar 

  49. Jin G (2023) Selection of virtual team members for smart port development projects through the application of the direct and indirect uncertain TOPSIS method. Expert Syst Appl 217:119555. https://doi.org/10.1016/j.eswa.2023.119555

    Article  Google Scholar 

  50. Liu P, Xu Y, Li Y (2023) An improved failure mode and effect analysis model for automatic transmission risk assessment considering the risk interaction. IEEE Trans Reliab 72:1107–1122. https://doi.org/10.1109/TR.2022.3215110

    Article  Google Scholar 

  51. Naz S, Akram M, Ul Hassan MM, Fatima A (2023) A Hybrid DEMATEL-TOPSIS Approach Using 2-Tuple Linguistic q-Rung Orthopair Fuzzy Information and its Application in Renewable Energy Resource Selection. Int J Inf Tech Decis 1–44. https://doi.org/10.1142/S0219622023500323

  52. Cheng X, Xu Z, Gou X (2024) A large-scale group decision-making model considering risk attitudes and dynamically changing roles. Expert Syst Appl 245:123017. https://doi.org/10.1016/j.eswa.2023.123017

    Article  Google Scholar 

  53. Liu Z, Wang X, Sun N et al (2021) FMEA Using the Normalized Projection-Based TODIM-PROMETHEE II Model for Blood Transfusion. Int J Fuzzy Syst 23:1680–1696. https://doi.org/10.1007/s40815-021-01056-3

    Article  Google Scholar 

  54. Panwar N, Kumar S (2021) Critical ranking of steam handling unit using integrated cloud model and extended PROMETHEE for maintenance purpose. Complex Intell Syst 7:367–378. https://doi.org/10.1007/s40747-020-00210-y

    Article  Google Scholar 

  55. Yu Y, Yang J, Wu S (2023) A novel FMEA approach for submarine pipeline risk analysis based on IVIFRN and ExpTODIM-PROMETHEE-II. Appl Soft Comput 136:110065. https://doi.org/10.1016/j.asoc.2023.110065

    Article  Google Scholar 

  56. Liao H, Hu Z, Zhang Z et al (2023) Outranking-based failure mode and effects analysis considering interactions between risk factors and its application to food cold chain management. Eng Appl Artif Intell 126:106831. https://doi.org/10.1016/j.engappai.2023.106831

    Article  Google Scholar 

  57. Lian X, Hou L, Zhang W et al (2023) Identifying risky components of display products for redesign considering user attention and failure causality. Soft Comput 27:2921–2942. https://doi.org/10.1007/s00500-022-07660-1

    Article  Google Scholar 

  58. Xu Z, Xia M (2011) Distance and similarity measures for hesitant fuzzy sets. Inform Sci 181:2128–2138. https://doi.org/10.1016/j.ins.2011.01.028

    Article  MathSciNet  Google Scholar 

  59. Yager RR (1988) On ordered weighted averaging aggregation operators in multicriteria decision making. IEEE Trans Syst Man Cyber 18:183–190. https://doi.org/10.1109/21.87068

    Article  Google Scholar 

  60. Liu P, Zhang P (2021) A normal wiggly hesitant fuzzy MABAC method based on CCSD and prospect theory for multiple attribute decision making. Int J Intell Syst 36:447–477. https://doi.org/10.1002/int.22306

    Article  Google Scholar 

  61. Yu D (2013) Triangular hesitant fuzzy set and its application to teaching quality evaluation. J Inf Comput Sci 10:1925–1934. https://doi.org/10.12733/jics20102025

    Article  Google Scholar 

  62. Park H-S, Jun C-H (2009) A simple and fast algorithm for K-medoids clustering. Expert Syst Appl 36:3336–3341. https://doi.org/10.1016/j.eswa.2008.01.039

    Article  Google Scholar 

  63. Yang C, Wang Q, Peng W, Zhu J (2020) a multi-criteria group decision-making approach based on improved BWM and multimoora with normal wiggly hesitant fuzzy information. Int J Comput Int Sys 13:366. https://doi.org/10.2991/ijcis.d.200325.001

    Article  Google Scholar 

  64. Zhang P, Zhang Z, Gong D, Cui X (2023) A novel normal wiggly hesitant fuzzy multi-criteria group decision making method and its application to electric vehicle charging station location. Expert Syst Appl 223:119876. https://doi.org/10.1016/j.eswa.2023.119876

    Article  Google Scholar 

  65. Liu P, Zhang P (2020) Normal wiggly hesitant fuzzy TODIM approach for multiple attribute decision making. J Intell Fuzzy Syst 39:627–644. https://doi.org/10.3233/JIFS-191569

    Article  Google Scholar 

Download references

Acknowledgments

This work was supported by Fundamental Research Funds for the Central Universities 2023YJS114, the National Natural Science Foundation of China under Grant 62276020, the Beijing Natural Science Foundation under Grant 9222025 and the MOE (Ministry of Education in China) Project of Humanities and Social Sciences under Grant 19YJC630043, China State Railway Group Co., Ltd. (B23D00030), and was partially supported by the Beijing Logistics Informatics Research Base. We very much appreciate their support.

Author information

Authors and Affiliations

  1. School of Economics and Management, Beijing Jiaotong University, Beijing, 100044, China

    Pei Zhang, Zhenji Zhang & Daqing Gong

Authors
  1. Pei Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Zhenji Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Daqing Gong
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

All authors contributed to the study’s conception and design. All the authors participated in the material preparation, data collection and analysis. Zhang Pei wrote the first draft of the paper. All the authors contributed to the revisions of the paper. All the authors approved the final manuscript.

Corresponding author

Correspondence to Daqing Gong.

Ethics declarations

Conflict of interest/Competing interests

All the authors certify that there are no conflicts of interest with any individuals or organizations involved in this work.

Ethical and informed consent for the use of the data

All the data used in this study were ethical, and informed consent was obtained from all the participants.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Information

Below is the link to the electronic supplementary material.

Supplementary file1 (DOCX 1919 KB)

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, P., Zhang, Z. & Gong, D. An improved normal wiggly hesitant fuzzy FMEA model and its application to risk assessment of electric bus systems. Appl Intell 54, 6213–6237 (2024). https://doi.org/10.1007/s10489-024-05458-2

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10489-024-05458-2

Keywords