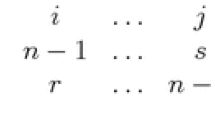Abstract
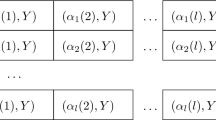
A permutation array (PA) A is a set of permutations on \(Z_n=\{0,1,\dots ,n-1\}\), for some n. A PA A has pairwise Hamming distance at least d, if for every pair of permutations \(\sigma \) and \(\tau \) in A, there are at least d integers i in \(Z_n\) such that \(\sigma (i)\ne \tau (i)\). Let M(n, d) denote the maximum number of permutations in any PA with pairwise Hamming distance at least d. Recently considerable effort has been devoted to improving known lower bounds for M(n, d) for all \(n>d>3\). We give a partition and extension operation that enables the production of a new PA \(A'\) for \(M(n+1,d)\) from an existing PA A for \(M(n,d-1)\). In particular, this operation allows for improvements for PA’s for \(M(q+1,q)\) for powers of prime numbers q, as well as for many other choices of n and d, where n is not a power of a prime. Finally, for prime numbers p, the partition and extension technique provides an asymptotically better lower bound for \(M(p+1,p)\) than that given by current knowledge about mutually orthogonal Latin squares. We prove a new asymptotic lower bound for the set of primes p, namely, \(M(p+1,p)\ge p^{1.5}/2-O(p)\).
Similar content being viewed by others
References
Bereg S., Morales L., Sudborough I.: Table of lower bounds for \(M(n,n-1)\). http://www.utdallas.edu/~sxb027100/soft/permutation-arrays/n-1/ (2016).
Bereg S., Morales L., Sudborough I.: PAs exhibiting results from partition and extension. http://www.utdallas.edu/~sxb027100/soft/permutation-arrays/n-1/data.zip (2016).
Beth T.: Eine Bemerkung zur Abschätzung der Anzahl orthogonaler lateinischer Quadrate mittels Siebverfahren. Abh. Math. Sem. Hamburg. 53, 284–288 (1983).
Chu W., Colbourn C.J., Dukes P.: Constructions for permutation codes in powerline communications. Des. Codes Cryptogr. 32, 51–64 (2004).
Colbourn C.J., Dinitz J.H.: Handbook of Combinatorial Designs, 2nd edn. Chapman and Hall/CRC, New York (2006).
Colbourn C.J., Kløve T., Ling A.C.H.: Permutation arrays for powerline communication and mutually orthogonal latin squares. IEEE Trans. Inf. Theory 50, 1289–1291 (2004).
Deza M., Vanstone S.A.: Bounds for permutation arrays. J. Stat. Plan. Inference 2, 197–209 (1978).
Frankl P., Deza M.: On the maximum number of permutations with given maximal or minimal distance. J. Comb. Theory A 22, 352–360 (1977).
Gao F., Yang Y., Ge G.: An improvement on the Gilbert-Varshamov bound for permutation codes. IEEE Trans. Inf. Theory 59, 3059–3063 (2013).
Huang Y.-Y., Tsai S.-C., Wu H.-L.: On the construction of permutation arrays via mappings from binary vectors to permutations. Des. Codes Cryptogr. 40, 139–155 (2006).
Huczynska S.: Powerline communication and the 36 officers problem. Philos. Trans. R. Soc. Lond. A 364, 3199–3214 (2006).
Janiszczak I., Staszewski R.: An Improved Bound for Permutation Arrays of Length 10. Technical Report 4. Institute for Experimental Mathematics, University Duisburg-Essen, Essen (2008).
Janiszczak I., Lempken W., Östergård P.R.J., Staszewski R.: Permutation codes invariant under isometries. Des. Codes Cryptogr. 75, 497–507 (2015).
Lin T.-T., Tsai S.-C., Tzeng W.-G.: Efficient encoding and decoding with permutation arrays. In: IEEE International Symposium on Information Theory (ISIT’08), pp. 211–214 (2008).
Nguyen Q.T.: Transitivity and hamming distance of permutation arrays. PhD thesis, University of Texas at Dallas Richardson, TX (2013).
Passman D.: Permutation Groups. Benjamin Inc, New York (1968).
Pavlidou N., Vinck A.H., Yazdani J., Honary B.: Power line communications: state of the art and future trends. IEEE Commun. Mag. 41, 34–40 (2003).
Quistorff J.: A new nonexistence result for sharply multiply transitive permutation sets. Discret. Math. 288, 185–186 (2004).
Rotman J.J.: An Introduction to the Theory of Groups, 4th edn. Springer, New York (1995).
Smith D.H., Montemanni R.: A new table of permutation codes. Des. Codes Cryptogr. 63, 241–253 (2012).
The Sage Developers: Mutually Orthogonal Latin Squares (MOLS). http://doc.sagemath.org/html/en/reference/combinat/sage/combinat/designs/latin_squares.html.
Acknowledgments
We would like to thank the referees for their many helpful suggestions and valuable comments.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by D. Jungnickel.
Rights and permissions
About this article
Cite this article
Bereg, S., Morales, L. & Sudborough, I.H. Extending permutation arrays: improving MOLS bounds. Des. Codes Cryptogr. 83, 661–683 (2017). https://doi.org/10.1007/s10623-016-0263-y
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10623-016-0263-y