A product strategy for daily deal campaigns utilizing demand expansion and consumer leakage

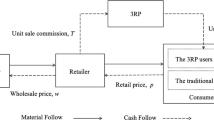
Abstract

Daily deal campaigns help local service merchants expand their market demand, which also result in consumer leakage concerns. This paper investigates how to exercise product strategies, i.e., price and quality decisions, for daily deal campaigns through a systematic exploration of demand expansion and consumer leakage. We consider a local service merchant who currently sells a product in his or her local market and who might contract with a platform to introduce daily deal campaigns. We document how the demand expansion and consumer leakage effects jointly affect a merchant’s product strategy. Particularly, we find it not always profitable to introduce daily deal campaigns amid relatively low levels of the demand expansion effect. Moreover, a merchant should adopt the product strategy of overlooking (inhibiting) consumer leakage amid relatively low (high) levels of the consumer leakage effect. In addition, we discuss the strategic role of a platform’s agency fees on leveraging a merchant’s product strategy. Based on this, we take a platform’s perspective to examine how to establish optimal agency fees amid various market conditions. Our results provide useful insights for firms in the daily deal business on how to carry out more intelligent marketing strategies. Our conclusions also cater to other businesses, wherein offline merchants could adopt online platforms to expand their demand while addressing the issue of consumer leakage.

Appendix: proofs of propositions

Proposition 1

By using the product strategy of overlooking consumer leakage, the merchant designs price ${p}_{d}$ and quality ${q}_{d}$ in daily deal campaigns to optimize the following problem:

$$\mathop {\max }\limits_{{p_d},{q_d}} \;\;\;\Delta \pi = \left( {{p_d} - \frac{1}{2}q_d^2} \right)n - \left( {\left( {p_l^* - \frac{1}{2}{{\left( {q_l^*} \right)}^2}} \right) - \left( {{p_d} - \frac{1}{2}q_d^2} \right)} \right)\beta$$

$$s.t.\;\;\;\left\{ {\begin{array}{*{20}{c}} {{p_d} < p_l^* = 1\;} \\ {{q_d} < q_l^* = 1\;} \\ {\theta {q_d} - {p_d} \geq 0} \end{array}} \right.$$

It shows that $\Delta \pi$ is increasing in ${p}_{d}$, and thus, given any feasible quality ${q}_{d}<{q}_{l}^{*}=1$, the optimal price should be set as ${p}_{d}({q}_{d})=\theta {q}_{d}<{p}_{l}^{*}=1$. We enter this optimal mapping into the function $\Delta \pi$ and obtain the optimal quality as ${q}_{d}^{*}=\theta <{q}_{l}^{*}=1$, the optimal price as ${p}_{d}^{*}={\theta }^{2}<{p}_{l}^{*}=1$, and the optimal profit change as ${\Delta \pi }^{*}=\frac{1}{2}({\theta }^{2}n-(1-{\theta }^{2})\beta )$. Thus, it is profitable for the merchant to introduce daily deal campaigns by using the product strategy of overlooking consumer leakage given ${\Delta \pi }^{*}\ge 0$, i.e., $\beta \le \frac{{\theta }^{2}}{1-{\theta }^{2}}n$.

These complete the proof of proposition 1.

Proposition 2

By using the product strategy of inhibiting consumer leakage, the merchant designs price ${p}_{d}$ and quality ${q}_{d}$ in daily deal campaigns and redesigns retail price ${p}_{l}$ to optimize the following problem:

$$\mathop {\max }\limits_{{p_d},{q_d},{p_l}} \;\;\;\Delta \pi = \left( {{p_d} - \frac{1}{2}q_d^2} \right)n - \left( {1 - {p_l}} \right)$$

$$s.t.\;\;\;\left\{ {\begin{array}{*{20}{c}} {{p_d} < {p_l}} \\ {{q_d} < q_l^* = 1} \\ {q_l^* - {p_l} \geq 0} \\ {\theta {q_d} - {p_d} \geq 0} \\ {q_l^* - {p_l} \geq {q_d} - {p_d}} \\ {\theta {q_d} - {p_d} \geq \theta q_l^* - {p_l}} \end{array}} \right.$$

The final two inequalities indicate that ${p}_{d}+\theta ({q}_{l}^{*}-{q}_{d})\le {p}_{l}\le {p}_{d}+{q}_{l}^{*}-{q}_{d}$. Moreover, it shows that $\Delta \pi$ increases in ${p}_{l}$ given any feasible bundle of ${p}_{d}$ and ${q}_{d}$. Thus, the optimal redesigned price in the local market should be set as ${p}_{l}={p}_{d}+{q}_{l}^{*}-{q}_{d}$. Based on this optimal mapping, we readily obtain ${p}_{d}<{p}_{l}$ due to ${q}_{d}<{q}_{l}^{*}$, which allows us to remove the first inequality from the problem. Moreover, simple exploration shows that we have ${q}_{l}^{*}-{p}_{l}\ge 0$ given $\theta {q}_{d}-{p}_{d}\ge 0$ and ${q}_{l}^{*}-{p}_{l}\ge {q}_{d}-{p}_{d}$. Thus, we can remove the third inequality from the problem. By using these properties we rewrite the original problem as follows:

$$\mathop {\max }\limits_{{p_d},{q_d}} \;\;\Delta \pi = \left( {{p_d} - \frac{1}{2}q_d^2} \right)n - \left( {1 - \left( {{p_d} + q_l^* - {q_d}} \right)} \right)$$

$$s.t.\;\;\left\{ {\begin{array}{*{20}{c}} {{q_d} < q_l^* = 1\;} \\ {\theta {q_d} - {p_d} \geq 0} \end{array}} \right.$$

This shows that $\Delta \pi$ is increasing in ${p}_{d}$ under any feasible ${q}_{d}$, and thus the optimal price should be set as ${p}_{d}({q}_{d})=\theta {q}_{d}$. Entering ${p}_{d}=\theta {q}_{d}$ and ${q}_{l}^{*}=1$ into function $\Delta \pi$, we obtain that $\Delta \pi =-\frac{n}{2}{q}_{d}^{2}+(n\theta -(1-\theta )){q}_{d}$. Therefore, the optimal quality in daily deal campaigns is ${q}_{d}^{*}=\theta -\frac{1-\theta }{n}$ and the optimal price is ${p}_{d}^{*}=\theta {q}_{d}^{*}={\theta }^{2}-\frac{\theta \left(1-\theta \right)}{n}$. The optimal redesigned price in the local market is ${p}_{l}^{*}={p}_{d}^{*}+{q}_{l}^{*}-{q}_{d}^{*}=\frac{({\theta }^{2}+1)(n+1)}{n}-\frac{\theta (n+2)}{n}$. The optimal profit change is ${\Delta \pi }^{*}=(\theta {q}_{d}^{*}-\frac{1}{2}{\left({q}_{d}^{*}\right)}^{2})n-({q}_{d}^{*}-\theta {q}_{d}^{*})=\frac{1}{2n}{(\theta n-(1-\theta ))}^{2}$. Thus, the merchant introduces daily deal campaigns under a condition $n\ge \frac{1-\theta }{\theta }$, which also ensures ${q}_{d}^{*}\ge 0$ and ${p}_{d}^{*}\ge 0$. Otherwise, if $n\le \frac{1-\theta }{\theta }$, then it is unprofitable to introduce daily deal campaigns for the merchant by using the product strategy of inhibiting consumer leakage.

These complete the proof of proposition 2.

Proposition 3

We first discuss the optimal product strategy in daily deal markets with $n\le \frac{1-\theta }{\theta }$. Proposition 1 indicates that the merchant introduces daily deal campaigns under $n\le \frac{1-\theta }{\theta }$ if $\beta \le {\beta }_{1}=\frac{{\theta }^{2}}{1-{\theta }^{2}}n$ by using the product strategy of overlooking consumer leakage. Proposition 2 shows that it is not applicable to introduce daily deal campaigns under $n\le \frac{1-\theta }{\theta }$ by using the product strategy of inhibiting consumer leakage. Therefore, it is optimal for the merchant to introduce daily deal campaigns by the product strategy of overlooking consumer leakage under $n\le \frac{1-\theta }{\theta }$ with $\beta \le {\beta }_{1}$. Otherwise, the merchant should not introduce daily deal campaigns under $n\le \frac{1-\theta }{\theta }$ with $\beta \ge {\beta }_{1}$.

We then examine the optimal product strategy in daily deal markets with $n\ge \frac{1-\theta }{\theta }$. Proposition 1 indicates that the merchant should introduce daily deal campaigns under $n\ge \frac{1-\theta }{\theta }$ if $\beta \le {\beta }_{1}$ by using the product strategy of overlooking consumer leakage. Proposition 2 shows that it is profitable to introduce daily deal campaigns under $\frac{\theta }{1-\theta }n\ge 1$ by using the product strategy of inhibiting consumer leakage. Thus, we need to contrast these two strategies to draw the final conclusions. We know that the optimal profit change is $\frac{1}{2}({\theta }^{2}n-(1-{\theta }^{2})\beta )$ in proposition 1 and $\frac{1}{2n}{(n\theta -(1-\theta ))}^{2}$ in proposition 2. Thus, we let $\frac{1}{2}({\theta }^{2}n-(1-{\theta }^{2})\beta )-\frac{1}{2n}{(n\theta -(1-\theta ))}^{2}\ge 0$ and obtain $\beta \le {\beta }_{2}=\frac{2n\theta +\theta -1}{n(1+\theta )}$. Moreover, simple exploration shows that ${\beta }_{2}\le {\beta }_{1}$. Therefore, it is optimal for the merchant to introduce daily deal campaigns by using the product strategy of inhibiting consumer leakage under $n\ge \frac{1-\theta }{\theta }$ with $\beta \ge {\beta }_{2}$. Otherwise, it is optimal for the merchant to introduce daily deal campaigns by using the product strategy of overlooking consumer leakage under $n\ge \frac{1-\theta }{\theta }$ with $\beta \le {\beta }_{2}$.

These complete the proof of proposition 3.

Proposition 4

Similar to the proof of proposition 1, the merchant designs the price ${p}_{d}$ and quality ${q}_{d}$ in daily deal campaigns to optimize the following problem:

$$\mathop {\max }\limits_{{p_d},{q_d}} \;\;\Delta \pi = \left( {\left( {1 - \gamma } \right){p_d} - \frac{1}{2}q_d^2} \right)n - \left( {\left( {p_l^* - \frac{1}{2}{{\left( {q_l^*} \right)}^2}} \right) - \left( {\left( {1 - \gamma } \right){p_d} - \frac{1}{2}q_d^2} \right)} \right)\beta$$

$$s.t.\;\;\;\left\{ {\begin{array}{*{20}{c}} {{p_d} < p_l^* = 1\;} \\ {{q_d} < q_l^* = 1\;} \\ {\theta {q_d} - {p_d} \geq 0} \end{array}} \right.$$

It shows that $\Delta \pi$ is increasing in ${p}_{d}$, and thus, given any feasible quality ${q}_{d}<{q}_{l}^{*}=1$ and agency fee $\gamma$, the optimal price should be set as ${p}_{d}({q}_{d})=\theta {q}_{d}<{p}_{l}^{*}=1$. We enter this optimal mapping into function $\Delta \pi$ and obtain the optimal quality as ${q}_{d}^{*}=(1-\gamma )\theta <{q}_{l}^{*}=1$, the optimal price as ${p}_{d}^{*}=\left(1-\gamma \right){\theta }^{2}<{p}_{l}^{*}=1$, and the optimal profit change as ${\Delta \pi }^{*}=\frac{1}{2}({(1-\gamma )}^{2}{\theta }^{2}n-(1-{(1-\gamma )}^{2}{\theta }^{2})\beta )$. Thus, after considering agency fees, it is profitable for the merchant to introduce daily deal campaigns by using the product strategy of overlooking consumer leakage with ${\Delta \pi }^{*}\ge 0$, which gives $\beta \le \frac{{\left(1-\gamma \right)}^{2}{\theta }^{2}}{1-{\left(1-\gamma \right)}^{2}{\theta }^{2}}n$.

These complete the proof of proposition 4.

Proposition 5

Similar to the proof of proposition 2, the merchant designs the price ${p}_{d}$ and quality ${q}_{d}$ in daily deal campaigns and redesigns retail price ${p}_{l}$ in the local market to optimize the following problem:

$$\mathop {\max }\limits_{{p_d},{q_d},{p_l}} \;\;\;\Delta \pi = \left( {\left( {1 - \gamma } \right){p_d} - \frac{1}{2}q_d^2} \right)n - \left( {1 - {p_l}} \right)$$

$$s.t.\;\;\;\left\{ {\begin{array}{*{20}{c}} {{p_d} < {p_l}} \\ {{q_d} < q_l^* = 1} \\ {q_l^* - {p_l} \geq 0} \\ {\theta {q_d} - {p_d} \geq 0} \\ {q_l^* - {p_l} \geq {q_d} - {p_d}} \\ {\theta {q_d} - {p_d} \geq \theta q_l^* - {p_l}} \end{array}} \right.$$

By a similar token used in the proof of proposition 2, we can reduce the above problem as follows:

$$\mathop {\max }\limits_{{p_d},{q_d}} \;\;\;\Delta \pi = \left( {\left( {1 - \gamma } \right){p_d} - \frac{1}{2}q_d^2} \right)n - \left( {1 - \left( {{p_d} + q_l^* - {q_d}} \right)} \right)$$

$$s.t.\;\;\;\left\{ {\begin{array}{*{20}{c}} {{q_d} < q_l^* = 1\;} \\ {\theta {q_d} - {p_d} \geq 0} \end{array}} \right.$$

It shows that $\Delta \pi$ is increasing in ${p}_{d}$ given any feasible ${q}_{d}$ and $\gamma$, and thus the optimal price should be set as ${p}_{d}({q}_{d})=\theta {q}_{d}$. Entering ${p}_{d}=\theta {q}_{d}$ and ${q}_{l}^{*}=1$ into function $\Delta \pi$, we obtain that $\Delta \pi =-\frac{n}{2}{q}_{d}^{2}+((1-\gamma )n\theta -(1-\theta )){q}_{d}$. Therefore, the optimal quality in daily deal campaigns is ${q}_{d}^{*}=(1-\gamma )\theta -\frac{1-\theta }{n}$, and the optimal price is ${p}_{d}^{*}=\theta {q}_{d}^{*}=(1-\gamma ){\theta }^{2}-\frac{\theta \left(1-\theta \right)}{n}$. The optimal redesigned price in the local market is ${p}_{l}^{*}={p}_{d}^{*}+{q}_{l}^{*}-{q}_{d}^{*}=\frac{({\theta }^{2}+1)(n+1)}{n}-\frac{\theta \left(n+2\right)}{n}+\gamma \theta (1-\theta )$. The optimal profit change is ${\Delta \pi }^{*}=\frac{1}{2n}{(\left(1-\gamma \right)\theta n-(1-\theta ))}^{2}$. Thus, after considering a platform’s agency fees, it is profitable for the merchant to introduce daily deal campaigns by using the product strategy of inhibiting consumer leakage with ${\Delta \pi }^{*}\ge 0$, which further gives $\gamma \le 1-\frac{1-\theta }{\theta n}$.

These complete the proof of proposition 5.

Proposition 6

We first discuss the optimal product strategy in daily deal markets with $\gamma \ge 1-\frac{1-\theta }{n\theta }$. Proposition 4 indicates that the merchant should introduce daily deal campaign by using the product strategy of overlooking consumer leakage under $\gamma \ge 1-\frac{1-\theta }{n\theta }$ if $\beta \le {\stackrel{\sim }{\beta }}_{2}=\frac{{\left(1-\gamma \right)}^{2}{\theta }^{2}}{1-{\left(1-\gamma \right)}^{2}{\theta }^{2}}n$. Proposition 5 shows that it is not applicable to introduce daily deal campaigns by using the product strategy of inhibiting consumer leakage under $\gamma \ge 1-\frac{1-\theta }{n\theta }$. Thus, it is optimal to introduce daily deal campaigns by the product strategy of overlooking consumer leakage under $\gamma \ge 1-\frac{1-\theta }{n\theta }$ with $\beta \le {\stackrel{\sim }{\beta }}_{2}$. Otherwise, the merchant should not introduce daily-deal campaigns under $\gamma \ge 1-\frac{1-\theta }{n\theta }$ with $\beta \ge {\stackrel{\sim }{\beta }}_{2}$.

We then examine the optimal product strategy in daily deal markets with $\gamma \le 1-\frac{1-\theta }{n\theta }$. We need to contrast two product strategies to obtain the final conclusions. We know that the optimal profit change is $\frac{1}{2}({(1-\gamma )}^{2}{\theta }^{2}n-(1-{(1-\gamma )}^{2}{\theta }^{2})\beta )$ in proposition 4 and $\frac{1}{2n}{(\left(1-\gamma \right)\theta n-(1-\theta ))}^{2}$ in proposition 5. Thus, we let $\frac{1}{2}({(1-\gamma )}^{2}{\theta }^{2}n-(1-{(1-\gamma )}^{2}{\theta }^{2})\beta )-\frac{1}{2n}{(\left(1-\gamma \right)\theta n-(1-\theta ))}^{2}\ge 0$ and obtain $\beta \le \frac{2\left(1-\gamma \right)\theta \left(1-\theta \right)-\frac{{(1-\theta )}^{2}}{n}}{1-{(1-\gamma )}^{2}{\theta }^{2}}={\stackrel{\sim }{\beta }}_{1}$. Moreover, simple exploration shows ${\stackrel{\sim }{\beta }}_{1}\le {\stackrel{\sim }{\beta }}_{2}$. Therefore, it is optimal for the merchant to introduce daily deal campaigns by using the product strategy of inhibiting consumer leakage under $\gamma \le 1-\frac{1-\theta }{n\theta }$ with $\beta \ge {\stackrel{\sim }{\beta }}_{1}$. Otherwise, it is optimal for the merchant to introduce daily deal campaigns by using the product strategy of overlooking consumer leakage under $\gamma \le 1-\frac{1-\theta }{n\theta }$ with $\beta \le {\stackrel{\sim }{\beta }}_{1}$.

These complete the proof of proposition 6.