Abstract
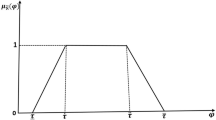
In this study we first present the preference structures in decision making as a generalized non-linear function. Then, we incorporate it into a fuzzy linear inequality of which the progressive and conservative manners are described by the concept of target hyperplanes. Thus, one’s preference domain is said to be bounded by such soft constraint. When facing different situations, a membership function is defined as an evaluation function for incorporating one’s optimistic or pessimistic attitude into this soft constraint. Once a goal is pursued, the problem is transformed into a symmetric fuzzy linear program. Based on max–min principle, an auxiliary crisp model in the form of a generalized fractional program is derived. Then, Dinkelbach-type-2 and the bisection algorithms are adopted for solution. Finally their simulation results of an uncertain production scheduling problem are reported.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
R. Bellman L. A. Zadeh (1970) ArticleTitleDecision-making in a Fuzzy Environment Management Science 17 B141–B164 Occurrence Handle10.1287/mnsc.17.4.B141 Occurrence Handle301613
C. Carlsson P. Korhonen (1986) ArticleTitleA Parametric Approach to Fuzzy Linear Programming Fuzzy Sets and Systems 20 17–30 Occurrence Handle0603.90093 Occurrence Handle852865
J. P. Crouzeix J. A. Ferland S. Schaible (1986) ArticleTitleA Note on an Algorithms for Generalized Fractional Programs Journal of Optimization Theory and Applications 50 183–187 Occurrence Handle0573.90090 Occurrence Handle851133
J. Dombi (1990) ArticleTitleMembership Function as an Evaluation Fuzzy Sets and System 35 1–21 Occurrence Handle0701.04006 Occurrence Handle1048208
S. Fang S. -C. S. Puthenpura (1993) Linear Optimization and Extensions: Theory and Algorithms Prentice-Hall New Jersey Occurrence Handle0799.90080
J. A. Ferland J. -Y. Potvin (1985) ArticleTitleGeneralized Fractional Programming: Algorithms and Numerical Experimentation European Journal of Operational Research 20 92–101 Occurrence Handle0564.90081 Occurrence Handle785837
H. Frenk S. Schaible (2001) Fractional Programming C.A. Floudas P.M. Pardalos (Eds) Encyclopedia of Optimization Kluwer Academic Publishers Dordrecht 162–172
C. -F. Hu S. -C. Fang (1999) ArticleTitleSolving Fuzzy Inequalities with Piecewise Linear Membership Functions IEEE Transactions on Fuzzy Systems 7 230–235
ILOG. (1997). Using the CPLEX Callable Library. Mountain View: ILOG CPLEX Division.
M. Inuiguchi H. Ichihashi Y. Kume (1990) ArticleTitleA Solution Algorithm for Fuzzy Linear Programming with Piecewise Linear Membership Functions Fuzzy Sets and Systems 34 15–31 Occurrence Handle0693.90064 Occurrence Handle1039708
J. Kacprzyk (1998) ”Fuzzy Optimization” E. Ruspini P. Bonissone W. Pedrycz (Eds) Handbook of Fuzzy Computation Institute of Physics Press Philadelphia
U. Kaymak J. M. Sousa (2003) ArticleTitleWeighted Constraint Aggregation in Fuzzy Optimization Constraints 8 61–78 Occurrence Handle1040.68112 Occurrence Handle1966217
X Lui (2001) ArticleTitleMeasuring the Satisfaction of Constraints in Fuzzy Linear Programming Fuzzy Sets and Systems 122 263–275 Occurrence Handle1854818
J. Ramík H. Rommelfanger (1996) ArticleTitleFuzzy Mathematical Programming based on Some New Inequality Relations Fuzzy Sets and Systems 81 77–87 Occurrence Handle0877.90085 Occurrence Handle1402277
H. Rommelfanger (1996) ArticleTitleFuzzy Mathematical Programming and Application European Journal of Operational Research 92 512–527 Occurrence Handle0914.90265
H. Tanaka T. Okuda K. Asai (1974) ArticleTitleOn Fuzzy Mathematical Programming Journal of Cybernetics 3 37–46 Occurrence Handle449722
S. Tong (1994) ArticleTitleInterval Number and Fuzzy Number Linear Programmings Fuzzy Sets and Systems 66 301–306 Occurrence Handle1300286
P. M. Vasant (2003) ArticleTitleApplication of Fuzzy Linear Programming in Production Planning Fuzzy Optimization and Decision Making 3 229–241 Occurrence Handle2002431
H. F. Wang K. Y. Wu (2003) ArticleTitleModeling and Analysis for Multi-period, Multi-product and Multi-resource Production Scheduling Journal of Intelligent Manufacturing 14 297–309
L. A. Zadeh (1965) ArticleTitleFuzzy Sets Information and Control 8 338–353 Occurrence Handle0139.24606 Occurrence Handle219427
H.-J. Zimmermann (1976) ArticleTitleDescription and Optimization of Fuzzy Systems International Journal of General Systems 2 209–215
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Wang, HF., Wu, KY. Preference Approach to Fuzzy Linear Inequalities and Optimizations. Fuzzy Optim Decis Making 4, 7–23 (2005). https://doi.org/10.1007/s10700-004-5567-0
Issue Date:
DOI: https://doi.org/10.1007/s10700-004-5567-0