Skip to main content

Advertisement

Springer Nature Link
Log in

Reversed hazard function of uncertain lifetime

  • Published:
Fuzzy Optimization and Decision Making Aims and scope Submit manuscript

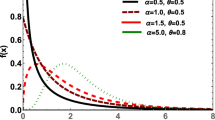
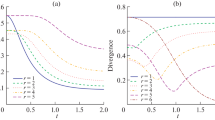
Abstract

Reversed hazard function is widely applied in reliability analysis. This paper considers the human uncertainty in a system, and employs uncertain variable to model the lifetime of a component. Concepts of mean residual life and residual entropy are proposed to describe a failed system, and their relationships with the reversed hazard function are discussed. In addition, this paper provides some applications of reversed hazard function to the mean past lifetime and past entropy.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  • Brito, G., Zequeira, I. R., & Valdés, E. J. (2011). On the hazard rate and reversed hazard rate orderings in two-component series systems with active redundancies. Statistics and Probability Letters, 81, 201–206.

    Article  MathSciNet  Google Scholar 

  • Dai, W., & Chen, X. W. (2012). Entropy of function of uncertain variables. Mathematical and Computer Modelling, 55(3-4), 754–760.

    Article  MathSciNet  Google Scholar 

  • Finkelstein, M. (2002). On the reversed hazard rate. Reliability Engineering and System Safety, 78, 71–75.

    Article  Google Scholar 

  • Fussell, J. B. (1975). How to hand-calculate system reliability and safety characteristics. IEEE Transactions on Reliability, 24(3), 169–174.

    Article  Google Scholar 

  • Gao, R., & Yao, K. (2016). Importance index of components in uncertain random systems. Knowledge-Based Systems, 109, 208–217.

    Article  Google Scholar 

  • Gao, Y. (2011). Shortest path problem with uncertain arc lengths. Computers and Mathematics with Applications, 62(6), 2591–2600.

    Article  MathSciNet  Google Scholar 

  • Gupta, C. R., & Gupta, D. R. (2007). Proportional reversed hazard rate model and its applications. Journal of Statistical Planning and Inference, 137, 525–3536.

    MathSciNet  MATH  Google Scholar 

  • Kahneman, D., & Tversky, A. (1979). Prospect theory: An analysis of decision under risk. Econometrica, 47(2), 263–292.

    Article  Google Scholar 

  • Kang, R., Zhang, Q. Y., Zeng, Z. G., Zio, E., & Li, X. Y. (2016). Measuring reliability under epistemic uncertainty: Review on non-probabilistic reliability metrics. Chinese Journal of Aeronautics, 29(3), 571–579.

    Article  Google Scholar 

  • Ke, H., & Yao, K. (2016). Block replacement policy in uncertain environment. Reliability Engineering and System Safety, 148, 119–124.

    Article  Google Scholar 

  • Kuo, W., Zhang, W., & Zuo, M. J. (1990). A consecutive k-out-of-n: G system: the mirror image of a consecutive k-out-of-n: F system. IEEE Transactions on Reliability, 39(2), 244–253.

    Article  Google Scholar 

  • Li, X. H., Da, G. F., & Zhao, P. (2010). On reversed hazard rate in general mixture models. Statistics and Probability Letters, 80, 654–661.

    Article  MathSciNet  Google Scholar 

  • Li, X., & Liu, B. (2009). Hybrid logic and uncertain logic. Journal of Uncertain Systems, 3(2), 83–94.

    Google Scholar 

  • Liu, B. (2007). Uncertainty theory (2nd ed.). Berlin: Springer.

    MATH  Google Scholar 

  • Liu, B. (2009). Some research problems in uncertainty theory. Journal of Uncertain Systems, 3(1), 3–10.

    Google Scholar 

  • Liu, B. (2010a). Uncertain risk analysis and uncertain reliability analysis. Journal of Uncertain Systems, 4(3), 163–170.

    Google Scholar 

  • Liu, B. (2010b). Uncertainty theory: A branch of mathematics for modeling human uncertainty. Berlin: Springer.

    Book  Google Scholar 

  • Liu, Y. H., & Ha, M. H. (2010). Expected value of function of uncertain variables. Journal of Uncertain Systems, 4(3), 181–186.

    Google Scholar 

  • Peng, Z. X., & Iwamura, K. (2010). A sufficient and necessary condition of uncertainty distribution. Journal of Interdisciplinary Mathematics, 13(3), 277–285.

    Article  MathSciNet  Google Scholar 

  • Qin, Z. F., & Kar, S. (2013). Single-period inventory problem under uncertain environment. Applied Mathematics and Computation, 219(18), 9630–9638.

    Article  MathSciNet  Google Scholar 

  • Rosyida, I., Peng, J., Chen, L., Widodo, W., Indrati, R., & Sugeng, K. A. (2016). An uncertain chromatic number of an uncertain graph based on alpha-cut coloring. Fuzzy Optimization and Decision Making,. https://doi.org/10.1007/s10700-016-9260-x.

    Article  MATH  Google Scholar 

  • Yao, K. (2015). A formula to calculate the variance of uncertain variable. Soft Computing, 19(10), 2947–2953.

    Article  Google Scholar 

  • Yao, K., & Ralescu, D. A. (2014). Age replacement policy in uncertain environment. Iranian Journal of Fuzzy Systems, 10(4), 1991–1997.

    MathSciNet  Google Scholar 

  • Zeng, Z. G., Wen, M. L., & Kang, R. (2013). Belief reliability: A new metrics for products’ reliability. Fuzzy Optimization and Decision Making, 12(1), 15–27.

    Article  MathSciNet  Google Scholar 

  • Zhang, Z. Q., Ralescu, A. D., & Liu, W. Q. (2016). Valuation of interest rate ceiling and floor in uncertain financial market. Fuzzy Optimization and Decision Making, 15(2), 139–154.

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Statistics, University of Sistan and Baluchestan, Zahedan, Iran

    Hamed Ahmadzade

  2. School of Economics and Management, Hebei University of Technology, Tianjin, 300401, China

    Rong Gao

Authors
  1. Hamed Ahmadzade
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Rong Gao
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Rong Gao.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ahmadzade, H., Gao, R. Reversed hazard function of uncertain lifetime. Fuzzy Optim Decis Making 17, 387–400 (2018). https://doi.org/10.1007/s10700-017-9281-0

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10700-017-9281-0

Keywords