Multicast Network Security with Asymmetric Cooperative Relaying

Abstract

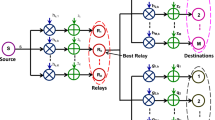
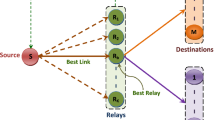
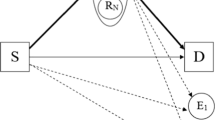
This paper exhibits the confidentiality performance study of a cooperative multicast network consisting of ${\mathcal {K}}$ asymmetric relays over Nakagami-m fading channels, where the communication links face uneven signal-to-noise ratios (SNRs). Although, conventional symmetric deployment of relays are convenient for analysis purposes but this assumption is not always effective in real cooperative network applications. So in this analysis, a cooperative multicast network is considered where a group of asymmetric relays cooperate in communication between a base station and multiple destinations under the nose of multiple eavesdroppers. Based on the given probability density function (PDF) of SNR for a point-to-point communication system, the analytical expressions of the PDF of SNRs are developed for multicast and eavesdroppers channels. Then, using these PDFs, the analytical representations for the probability of non-zero secrecy multicast capacity and the secure outage probability are derived for analyzing the performance of the proposed model. Finally, analytical results are verified with Monte-Carlo simulations.

Appendices

Appendix I

The evaluation of the integral, ${\mathcal {I}}_{1}$ is given as follows:

$$\begin{aligned} {\mathcal {I}}_{1}&=\int _{0}^{\alpha _{l}}\sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1} {\mathcal {B}}\beta _{n}^{a_{2}}e^{-b_{2}\beta _{n}} \Biggl [\sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}}\Biggl \{\frac{a_{2}!}{b_{2}^{a_{2}+1}}\nonumber \\&\quad -\sum _{\kappa _{1}=0}^{a_{2}} \frac{a_{2}!\beta _{n}^{\kappa _{1}}e^{-b_{2}\beta _{n}}}{\kappa _{1}!b_{2}^{a_{2} -\kappa _{1}+1}}\Biggl \}\Biggl ]d\beta _{n}\nonumber \\&=\int _{0}^{\alpha _{l}} \sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}}\sum ^{{\mathcal {K}}+1}_{\jmath } \sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}} \Biggl [\Biggl \{\frac{a_{2}!\beta _{n}^{a_{2}}e^{-b_{2}\beta _{n}}}{b_{2}^{a_{2}+1}}\nonumber \\&\quad -\sum _{\kappa _{1}=0}^{a_{2}} \frac{a_{2}!\beta _{n}^{a_{2}+\kappa _{1}}e^{-2b_{2}\beta _{n}}}{\kappa _{1}!b_{2}^{a_{2} -\kappa _{1}+1}}\Biggl \}\Biggl ]d\beta _{n}\nonumber \\&=\sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}}\sum ^{{\mathcal {K}}+1}_{\jmath } \sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}}\Biggl [{\mathcal {D}}_{1} \int _{0}^{\alpha _{l}}\beta _{n}^{a_{2}}e^{-b_{2}\beta _{n}}d\beta _{n}\nonumber \\&\quad -{\mathcal {F}}_{1}\int _{0}^{\alpha _{l}} \beta _{n}^{\eta _{1}}e^{-\theta _{1}\beta _{n}}d\beta _{n}\Biggl ], \end{aligned}$$

(25)

where ${\mathcal {D}}_{1}=a_{2}!b_{2}^{-(a_{2}+1)}$, ${\mathcal {F}}_{1}=\sum _{\kappa _{1}=0}^{a_{2}}\frac{a_{2}!}{\kappa _{1}!b_{2}^{\eta _{2}}}$, $\eta _{1}=a_{2}+\kappa _{1}$, $\eta _{2}=a_{2}-\kappa _{1}+1$ and $\theta _{1}=2b_{2}$. Performing integration using eqn. (8), we obtain

$$\begin{aligned} {\mathcal {I}}_{1}&=\sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}} \sum ^{{\mathcal {K}}+1}_{\jmath }\sum ^{m_{\jmath }}_{\kappa =1}{\mathcal {B}}\Biggl [{\mathcal {D}}_{1} \Biggl ({\mathcal {D}}_{1}-{\mathcal {F}}_{2}\frac{\gamma _{l}^{\kappa _{2}}}{e^{b_{2}\gamma _{l}}}\Biggl ) -{\mathcal {F}}_{1}\Biggl ({\mathcal {D}}_{2}-{\mathcal {F}}_{3}\frac{\alpha _{l}^{\kappa _{3}}}{e^{\theta _{1}\alpha _{l}}}\Biggl )\Biggl ]. \end{aligned}$$

(26)

where ${\mathcal {D}}_{2}=\eta _{1}!\theta _{1}^{-(\eta _{1}+1)}$, ${\mathcal {F}}_{2}=\sum _{\kappa _{2}=0}^{a_{2}}\frac{a_{2}!}{\kappa _{2}!b_{2}^{\eta _{3}}}$, ${\mathcal {F}}_{3}=\sum _{\kappa _{3}=0}^{\eta _{1}}\frac{\eta _{1}!}{\kappa _{3}!\theta _{1}^{\eta _{4}}}$, $\eta _{3}=a_{2}-\kappa _{2}+1$ and $\eta _{4}=\eta _{1}-\kappa _{3}+1$.

Appendix II

The evaluation of the integral, ${\mathcal {I}}_{2}$ is given as follows:

$$\begin{aligned} {\mathcal {I}}_{2}&=\int _{L}^{\infty }\sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1} {\mathcal {A}}\frac{\alpha _{l}^{a_{1}}}{e^{b_{1}\alpha _{l}}}\Biggl [1- \sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1}{\mathcal {A}}\Biggl ({\mathcal {E}}_{1} -\sum _{k_{1}=0}^{a_{1}} {\mathcal {G}}_{1}\frac{\alpha _{l}^{k_{1}}}{e^{b_{1}\alpha _{l}}}\Biggl )\Biggl ]d\alpha _{l}. \end{aligned}$$

(27)

Performing integration using the following identity of [27, eq. (3.351.2)],

$$\begin{aligned} \int ^{\infty }_{u}x^{n}e^{-\mu x}dx&=e^{-\mu u}\sum ^{n}_{\phi =0}\frac{n!}{\phi !}\times \frac{u^{\phi }}{\mu ^{n-\phi +1}}, \end{aligned}$$

we obtain

$$\begin{aligned} {\mathcal {I}}_{2}&=\sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1}{\mathcal {A}} \Biggl [\sum _{\phi _{1}=0}^{a_{1}}\frac{a_{1}!\sigma ^{\phi _{1}}e^{-b_{1}\sigma }}{b_{1}^{a_{1}-\phi _{1}+1}}- \sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1}{\mathcal {A}} \Biggl ({\mathcal {E}}_{1}\sum _{\phi _{1}=0}^{a_{1}} \frac{a!\sigma ^{\phi _{1}}e^{-b_{1}\sigma }}{b_{1}^{a_{1}-\phi _{1}+1}}\\&-\sum _{k_{1}=0}^{a_{1}}{\mathcal {G}}_{1}\sum _{\phi _{2}=0}^{k_{1}}\frac{k_{1}!\sigma ^{\phi _{2}}e^{-2b_{1}\sigma }}{b_{1}^{k_{1}-\phi _{2}+1}}\Biggl )\Biggl ]. \end{aligned}$$

Substituting the value of $\sigma$ and after some mathematical manipulation, we have

$$\begin{aligned} {\mathcal {I}}_{2}&=\sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1}{\mathcal {A}} \biggl [\varPi _{1}\frac{\beta _{n}^{\phi _{4}}}{e^{\theta _{3}\beta _{n}}} -\sum ^{{\mathcal {K}}+1}_{i}\sum ^{m_{i}}_{k=1}{\mathcal {A}} \left( \varPi _{2}\frac{\beta _{n}^{\phi _{6}}}{e^{-\theta _{3}\beta _{n}}} -\varPi _{3}\frac{\beta _{n}^{\phi _{8}}}{e^{-\theta _{4}\beta _{n}}}\right) \biggl ], \end{aligned}$$

(28)

where

$$\begin{aligned} \varPi _{1}\,=\,&\sum _{\phi _{1}=0}^{a_{1}}\sum _{\phi _{3}=0}^{\phi _{1}}\left( {\begin{array}{c}\phi _{1}\\ \phi _{3}\end{array}}\right) \sum _{\phi _{4}=0}^{\phi _{3}}\left( {\begin{array}{c}\phi _{3}\\ \phi _{4}\end{array}}\right) \frac{a_{1}!(-1)^{\phi _{1}-\phi _{3}} e^{2{\mathcal {R}}_{s}\phi _{3}}}{b_{1}^{a_{1}-\phi _{1}+1}e^{b_{1}(e^{2R_{s}}-1)}},\\ \varPi _{2}\,=\,&{\mathcal {E}}_{1}\sum _{\phi _{1}=0}^{a_{1}} \sum _{\phi _{5}=0}^{\phi _{1}}\left( {\begin{array}{c}\phi _{1}\\ \phi _{5}\end{array}}\right) \sum _{\phi _{6}=0}^{\phi _{5}}\left( {\begin{array}{c}\phi _{5}\\ \phi _{6}\end{array}}\right) \frac{a_{1}!(-1)^{\phi _{1}-\phi _{5}} }{b_{1}^{a_{1}-\phi _{1}+1}} \frac{e^{2{\mathcal {R}}_{s}\phi _{5}}}{e^{b_{1}(e^{2R_{s}}-1)}},\\ \varPi _{3}\,=\,&\sum _{k_{1}=0}^{a_{1}}{\mathcal {G}}_{1}\sum _{\phi _{2}=0}^{k_{1}} \sum _{\phi _{7}=0}^{\phi _{2}}\left( {\begin{array}{c}\phi _{2}\\ \phi _{7}\end{array}}\right) \sum _{\phi _{8}=0}^{\phi _{7}}\left( {\begin{array}{c}\phi _{7}\\ \phi _{8}\end{array}}\right) \frac{k_{1}!(-1)^{\phi _{2}-\phi _{7}} }{b_{1}^{k_{1}-\phi _{2}+1}} \frac{e^{2{\mathcal {R}}_{s}\phi _{7}}}{e^{2b_{1}(e^{2{\mathcal {R}}_{s}}-1)}},\\ \theta _{3}=&b_{1}e^{2{\mathcal {R}}_{s}} \,\, \text {and} \,\, \theta _{4}=2b_{1}e^{2R_{s}}. \end{aligned}$$