Abstract
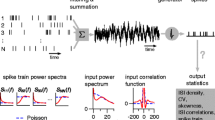
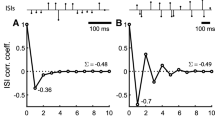
Neural spike trains are commonly characterized as a Poisson point process. However, the Poisson assumption is a poor model for spiking in auditory nerve fibres because it is known that interspike intervals display positive correlation over long time scales and negative correlation over shorter time scales. We have therefore developed a biophysical model based on the well-known Meddis model of the peripheral auditory system, to produce simulated auditory nerve fibre spiking statistics that more closely match the firing correlations observed in empirical data. We achieve this by introducing biophysically realistic ion channel noise to an inner hair cell membrane potential model that includes fractal fast potassium channels and deterministic slow potassium channels. We succeed in producing simulated spike train statistics that match empirically observed firing correlations. Our model thus replicates macro-scale stochastic spiking statistics in the auditory nerve fibres due to modeling stochasticity at the micro-scale of potassium channels.








Similar content being viewed by others
References
Berkefeld, H., Fakler, B., & Schulte, U. (2010). Ca2+-activated K+ channels: from protein complexes to function. Physiological Reviews, 90(4), 1437–1459.
Briaire, J. J., & Frijns, J. H. (2006). The consequences of neural degeneration regarding optimal cochlear implant position in scala tympani: a model approach. Hearing Research, 214(1), 17–27.
Burkitt, A. N., & Clark, G. M. (2001). Synchronization of the neural response to noisy periodic synaptic input. Neural Computation, 13(12), 2639–2672.
Connor, M., Vaughan, C. W., Chieng, B., & Christie, M. J. (1996). Nociceptin receptor coupling to a potassium conductance in rat locus coeruleus neurones in vitro. British Journal of Pharmacology, 119, 1614–1618.
FitzHugh, R. (1961). Impulses and physiological states in theoretical models of nerve membran. The Biophysical Journal, 1(6), 445–466.
Fuchs, P. A., & Evans, M. G. (1990). Potassium currents in hair cells isolated from the cochlea of the chick. The Journal of Physiology, 429(1), 529–551.
Gao, X., Grayden, D. B., & McDonnell, M. D. (2014). Stochastic information transfer from cochlear implant electrodes to auditory nerve fibres. Physical Review E, 90(2), 022722.
Gaumond, R. P., Kim, D. O., & Molnar, C. E. (1983). Responses of cochlear nerve fibres to brief acoustic stimuli: role of discharge history effects. The Journal of the Acoustical Society of America, 74, 1392–1398.
Gaumond, R. P. (1993). Ratio of variance to mean of action potential counts for an auditory nerve fibre model with second order refractory behavior. The Journal of the Acoustical Society of America, 93, 2035–2037.
Geisler, C. D., Rhode, W. S., & Kennedy, D. T. (1974). Responses to tonal stimuli of single auditory nerve fibres and their relationship to basilar membrane motion in the squirrel monkey. The Journal of Neurophysiology, 37 (6), 1156–1172.
Glowatzki, E., & Fuchs, P. A. (2002). Transmitter release at the hair cell ribbon synapse. Nature Neuroscience, 5(2), 147–154.
Goldwyn, J. H., & Shea-Brown, E. (2011). The what and where of adding channel noise to the Hodgkin-Huxley equations. PLoS Computational Biology, 7(11), 1–9.
Hodgkin, A. L., & Huxley, A. F. (1952). A quantitative description of membrane current and its application to conduction and excitation in nerve. The Journal of Physiology, 117, 500–544.
Jackson, M.B. (1985). Stochastic behavior of a many-channel membrane system. Biophysical Journal, 47, 129.
Jackson, B. S., & Carney, L. H. (2005). The spontaneous-rate histogram of the auditory nerve can be explained by only two or three spontaneous rates and long-range dependence. The Journal of the Association for Research in Otolaryngology, 6(2), 148–159.
Javel, E., & Viemeister, N. F. (2000). Stochastic properties of cat auditory nerve responses to electric and acoustic stimuli and application to intensity discrimination. The Journal of the Acoustical Society of America, 107 (2), 908–921.
Kelly, O. E. (1994). Analysis of long-range dependence in auditory-nerve fibre recordings. Masters Thesis, Rice University, Houston.
Kelly, O. E., Johnson, D. H., Delgutte, B., & Cariani, P. (1996). Fractal noise strength in auditory-nerve fibre recordings. The Journal of the Acoustical Society of America, 99(4), 2210–2220.
Kiang, N. Y., Rho, J. M., Northrop, C. C., Liberman, M. C., & Ryugo, D. K. (1982). Hair-cell innervation by spiral ganglion cells in adult cats. Science, 217(4555), 175–177.
Köppl, C. (1997). Phase locking to high frequencies in the auditory nerve and cochlear nucleus magnocellularis of the barn owl, Tyto alba. The Journal of Neuroscience, 17(9), 3312–3321.
Kros, C. J., & Crawford, A. C. (1990). Potassium currents in inner hair cells isolated from the guinea-pig cochlea. The Journal of Physiology, 421(1), 263–291.
Liberman, M. C. (1978). Auditory-nerve response from cats raised in a low-noise chamber. The Journal of the Acoustical Society of America, 63(2), 442–455.
Liberman, M. C. (1980). Morphological differences among radial afferent fibres in the cat cochlea: an electron-microscopic study of serial sections. Hearing Research, 3(1), 45–63.
Liebovitch, L. S., & Sullivan, J. M. (1987). Fractal analysis of a voltage-dependent potassium channel from cultured mouse hippocampal neurons. The Biophysical Journal, 52(6), 979–988.
Liebovitch, L. S., Fischbarg, J., & Koniarek, J. P. (1987). Ion channel kinetics: A model based on fractal scaling rather than multistate Markov processes. Mathematical Biosciences, 84(1), 37– 68.
Liebovitch, L. S. (1989). Testing fractal and markov models of ion channel kinetics. The Biophysical Journal, 55(2), 373– 1989.
Liebovitch, L. S., & Toth, T. I. (1990). Using fractals to understand the opening and closing of ion channels. Annals of Biomedical Engineering, 18(2), 177–194.
Liebovitch, L. S., Scheurle, D., Rusek, M., & Zochowski, M. (2001). Fractal methods to analyze ion channel kinetics. Methods, 24(4), 359–375.
Lopez-Poveda, E. A., & Eustaquio-Martin, A. (2006). A biophysical model of the inner hair cell: The contribution of potassium currents to peripheral auditory compression. The Journal of the Association for Research in Otolaryngology, 7(3), 218–235.
Lowen, S. B., & Teich, M. C. (1992). Auditory nerve action potentials form a nonrenewal point process over short as well as long time scales. The Journal of the Acoustical Society of America, 92, 803–806.
Lowen, S. B., Cash, S. S., Poo, M. M., & Teich, M. C. (1997). Quantal neurotransmitter secretion rate exhibits fractal behavior. The Journal of Neuroscience, 17(15), 5666–5677.
Lowen, S. B., Liebovitch, L. S., & White, J. A. (1999). Fractal ion-channel behavior generates fractal firing patterns in neuronal models. Physical Review E, 59(5), 5970–5980.
Lowen, S. B., & Teich, M. C. (2005). Fractal-based point processes (p. 366). Wiley.
Martinez-Dunst, C., Michaels, R. L., & Fuchs, P. A. (1997). Release sites and calcium channels in hair cells of the chicks cochlea. The Journal of Neuroscience, 17(23), 9133–9144.
Matthews, G., & Fuchs, P. (2010). The diverse roles of ribbon synapses in sensory neurotransmission. Nature Review Neuroscience, 11(12), 812–822.
McDonnell, M. D., Stocks, N. G., Pearce, C. E. M., & Abbott, D. (2008). Stochastic resonance: From suprathreshold stochastic resonance to stochastic signal quantization. Cambridge University Press.
Meddis, R. (2006). Auditory-nerve first-spike latency and auditory absolute threshold: A computer model. The Journal of Acoustical Society of America, 119(1), 406–417.
Middleton, J. W., Chacron, M. J., Lindner, B., & Longtin, A. (2003). Firing statistics of a neuron model driven by long-range correlated noise. Physical Review E, 68(2), 021920.
Millhauser, G. L., Salpeter, E. E., & Oswald, R. E. (1988). Diffusion models of ion-channel gating and the origin of power-law distributions from single-channel recording. Proceedings of the National Academy of Sciences, 85 (5), 1503–1507.
Moezzi, B., Iannella, N., & McDonnell, M. D. (2014). Modelling the influence of short term depression in vesicle release and stochastic calcium channel gating on auditory nerve spontaneous firing statistics. Frontiers in Computational Neuroscience, 8, 163.
Morse, R. P., & Evans, E. F. (1996). Enhancement of vowel coding for cochlear implants by addition of noise. Nature Medicine, 2, 928–93228.
Moser, T., Brandt, A., & Lysakowski, A. (2006). Hair cell ribbon synapses. Cell and Tissue Research, 326 (2), 347–359.
Nagumo, J., Arimoto, S., & Yoshizawa, S. (1962). An active pulse transmission line simulating nerve axon. Proceedings of the IRE, 50(10), 2061–2070.
Nawrot, M. P., Boucsein, C., Molina, V. R., Riehle, A., Aertsen, A., & Rotter, S. (2008). Measurement of variability dynamics in cortical spike trains. Journal of Neuroscience Methods, 169(2), 374– 390.
Peterson, A. J., Irvine, D. R., & Heil, P. (2014). A model of synaptic vesicle-pool depletion and replenishment can account for the interspike interval distributions and nonrenewal properties of spontaneous spike trains of auditory-nerve fibres. The Journal of Neuroscience, 34(45), 15097–15109.
Rudnicki, M., Schoppe, O., Isik, M., Volk, F., & Hemmert, W. (2015). Modeling auditory coding: From sound to spikes. Cell and Tissue Research, 361(1), 159–175.
Sansom, M. S., Ball, F. G., Kerry, C. J., McGee, R., Ramsey, R. L., & Usherwood, P. N. (1989). Markov, fractal, diffusion, and related models of ion channel gating. A comparison with experimental data from two ion channels. The Biophysical Journal, 56(6), 1229–1243.
Scott, P. C., Cowan, A. I., & Stricker, C. (2012). Quantifying impacts of short-term plasticity on neuronal information transfer. Physical Review E, 85(041921).
Taberner, A. M., & Liberman, M. C. (2005). Response properties of single auditory nerve fibres in the mouse. The Journal of Neurophysiology, 93(1), 557–569.
Teich, M. C. (1989). Fractal character of the auditory neural spike train. IEEE Transactions on Biomedical Engineering, 36(1), 150–160.
Teich, M. C., Turcott, R. G., & Lowen, S. B. (1990). The fractal doubly stochastic Poisson point process as a model for the cochlear neural spike train. The Mechanics and Biophysics of Hearing, 87, 354–361.
Teich, M. C., & Lowen, S. B. (1994). Fractal patterns in auditory nerve-spike trains. IEEE Engineering in Medicine and Biology Magazine, 13, 197–202.
Thurm, H., Fakler, B., & Oliver, D (2005). Ca2+independent activation of BKCa channels at negative potentials in mammalian inner hair cells. The Journal of Physiology, 569(1), 137–151.
Zampini, V., Johnson, S. L., Franz, C., Knipper, M., Holley, M. C., Magistretti, J., Masetto, S., & Marcott, W. (2013). Burst activity and ultrafast activation kinetics of CaV1.3 Ca2+ channels support presynaptic activity in adult gerbil hair cell ribbon synapse. The Journal of Physiology, 591(16), 3811–3820.
Zhang, X., Heinz, M. G., Bruce, I. C., & Carney, L. H. (2001). A phenomenological model for the responses of auditory-nerve fibres: I. Nonlinear tuning with compression and suppression. The Journal of the Acoustical Society of America, 109, 648–670.
Zilany, M. S., Bruce, I. C., Nelson, P. C., & Carney, L. H. (2009). A phenomenological model of the synapse between the inner hair cell and auditory nerve: Long-term adaptation with power-law dynamics. The Journal of the Acoustical Society of America, 126, 2390–2412.
Zilany, M. S., & Carney, L. H. (2010). Power-law dynamics in an auditory-nerve model can account for neural adaptation to sound-level statistics. The Journal of Neuroscience, 30(31), 10380–10390.
Zilany, M. S., Bruce, I. C., & Carney, L. H. (2014). Updated parameters and expanded simulation options for a model of the auditory periphery. The Acoustical Society of America, 135, 283–286.
Acknowledgments
Mark D. McDonnell’s contribution was supported by an Australian Research Fellowship from the Australian Research Council (project number DP1093425) and the National Health and Medical Research Council (NHMRC) of Australia (project grant, APP1050832). N. Iannella’s contribution was supported by the People Programme (Marie Curie Actions) of the European Unions Seventh Framework Programme (FP7/2007-2013) under REA grant agreement No PCOFUND-GA-2012-600181. We would like to thank Peter Heil from Leibniz Institute for Neurobiology, Idan Segev of Hebrew University and Luke Hallam of Charles Sturt University for helpful discussions.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interests
Bahar Moezzi, Nicolangelo Iannella and Mark D. McDonnell declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Additional information
Action Editor: S.A. Shamma
Rights and permissions
About this article
Cite this article
Moezzi, B., Iannella, N. & McDonnell, M.D. Ion channel noise can explain firing correlation in auditory nerves. J Comput Neurosci 41, 193–206 (2016). https://doi.org/10.1007/s10827-016-0613-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10827-016-0613-9