Skip to main content
Springer Nature Link
Log in

Nogood-FC for solving partitionable constraint satisfaction problems

  • Published:
Journal of Intelligent Manufacturing Aims and scope Submit manuscript

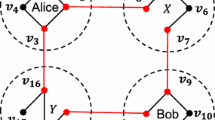
Abstract

Many real problems can be naturally modelled as constraint satisfaction problems (CSPs). However, some of these problems are of a distributed nature, which requires problems of this kind to be modelled as distributed constraint satisfaction problems (DCSPs). In this work, we present a distributed model for solving CSPs. Our technique carries out a partition over the constraint network using a graph partitioning software; after partitioning, each sub-CSP is arranged into a DFS-tree CSP structure that is used as a hierarchy of communication by our distributed algorithm. We show that our distributed algorithm outperforms well-known centralized algorithms solving partitionable CSPs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Abou-Rjeili, A., & Karypis, G. (2006). Multilevel algorithms for partitioning power-law graphs. In Parallel and Distributed Processing Symposium. 10 pp.

  • Dechter R. (1992) Constraint networks (survey). In: Shapiro S.C. (eds) Encyclopedia Artificial Intelligence.. Wiley, New York, pp 276–285

    Google Scholar 

  • Dechter, R. (2003). Constraint processing. Morgan Kaufman.

  • Ezzahir, R., Bessiere, C., Belaissaoui, M., & Bouyakhf, E.-H. (2007). DisChoco: A platform for distributed constraint programming. In Proceedings of IJCAI-2007 Eighth International Workshop on Distributed Constraint Reasoning (DCR’07), pp. 16–27.

  • Faltings, B. (2006). Distributed constraint programming. In F. Rossi, P. van Beek, & T. Walsh (Eds.), Handbook of constraint programming, foundations of artificial intelligence (pp. 699–729). Elsevier.

  • Karypis, G., & Kumar, V. (1995). Using METIS and parMETIS. http://www.cs.umn.edu/~meti..

  • Meisels, A., Kaplansky, E., Razgon, I., & Zivan, R. (2002). Comparing performance of distributed constraint processing algorithms. In Proceedings of the 4th Workshop on Distributed Constraint Reasoning.

  • Pellegrini, F. (2007). A parallelisable multi-level banded diffusion scheme for computing balanced partitions with smooth boundaries. In Lecture Notes in Computer Science, Vol. 4641 (pp. 191–200). Springer-Verlag.

  • Salido M.A., Barber F. (2006) Distributed CSPs by graph partitioning. Applied Mathematics and Computation 183: 491–498

    Article  Google Scholar 

  • Yokoo, M., & Hirayama, K. (1998). Distributed constraint satisfaction algorithm for complex local problems. In Proceedings of the 3rd International Conference on Multi Agent Systems.

  • Yokoo M., Hirayama K. (2000) Algorithms for distributed constraint satisfaction: A review. Autonomous Agents and Multi-Agent Systems 3: 185–207

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Information Systems and Computation, Technical University of Valencia, Camino de Vera s/n, 46022, Valencia, Spain

    Montserrat Abril, Miguel A. Salido & Federico Barber

Authors
  1. Montserrat Abril
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Miguel A. Salido
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Federico Barber
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Federico Barber.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Abril, M., Salido, M.A. & Barber, F. Nogood-FC for solving partitionable constraint satisfaction problems. J Intell Manuf 21, 101–110 (2010). https://doi.org/10.1007/s10845-008-0168-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10845-008-0168-3

Keywords