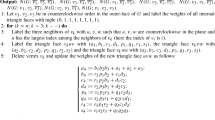Abstract
For a given graph and an integer t, the Min–Max 2-Clustering problem asks if there exists a modification of a given graph into two maximal disjoint cliques by inserting or deleting edges such that the number of the editing edges incident to each vertex is at most t. It has been shown that the problem can be solved in polynomial time for \(t<n/4\), where n is the number of vertices. In this paper, we design parameterized algorithms for different ranges of t. Let \(k=t-n/4\). We show that the problem is polynomial-time solvable when roughly \(k<\sqrt{n/32}\). When \(k\in o(n)\), we design a randomized and a deterministic algorithm with sub-exponential time parameterized complexity, i.e., the problem is in SUBEPT. We also show that the problem can be solved in \(O({2}^{n/r}\cdot n^2)\) time for \(k<n/12\) and in \(O(n^2\cdot 2^{3n/4+k})\) time for \(n/12\le k< n/4\), where \(r=2+\lfloor (n/4-3k-2)/(2k+1) \rfloor \ge 2\).

Similar content being viewed by others
References
Ailon N, Charikar M, Newman A (2008) Aggregating inconsistent information: Ranking and clustering. J ACM 55(5):231:23–27
Bansal N, Blum A, Chawla S (2004) Correlation clustering. Mach Learn 56:89–113
Böcker S, Briesemeister S, Bui Q, Truss A (2009) Going weighted: parameterized algorithms for cluster editing. Theoret Comput Sci 410(52):5467–5480
Böcker S (2012) A golden ratio parameterized algorithm for Cluster Editing. J Discrete Algorithms 16:79–89
Bonizzoni P, Vedova GD, Dondi R (2009) A PTAS for the minimum consensus clustering problem with a fixed number of clusters. In: Eleventh Italian Conference on Theoretical Computer Science
Bonizzoni P, Vedova GD, Dondi R, Jiang T (2008) On the approximation of correlation clustering and consensus clustering. J Comput Syst Sci 74(5):671–696
Chen J, Meng J (2012) A \(2k\) kernel for the cluster editing problem. J Comput Syst Sci 78(1):211–220
Chen LH, Chang MS, Wang CC, Wu BY (2013) On the min–max 2-cluster editing problem. J Inf Sci Eng 29:1109–1120
Damaschke P (2009) Bounded-degree techniques accelerate some parameterized graph algorithms. In: Chen J, Fomin F (eds) Parameterized and exact computation, vol 5917., Lecture Notes in Computer ScienceSpringer, Berlin, pp 98–109
Damaschke P (2010) Fixed-parameter enumerability of cluster editing and related problems. Theory Comput Syst 46:261–283
Damaschke P (2016) Sufficient conditions for edit-optimal clusters. Inf Process Lett 116(4):267–272
Damaschke P, Mogren O (2014) Editing simple graphs. J Graph Algorithms Appl 18:557–576
Fellows MR, Guo J, Komusiewicz C, Niedermeier R, Uhlmann J (2011) Graph-based data clustering with overlaps. Discrete Optim 8(1): 2–17 (Parameterized Complexity of Discrete Optimization)
Filkov V, Skiena S (2004) Integrating microarray data by consensus clustering. Int J Artif Intell Tools 13(04):863–880
Finney RL, Weir WD, Giordano FR (2001) Thomas’ calculus, vol 10. Addison-Wesley, Reading
Fomin FV, Kratsch S, Pilipczuk M, Pilipczuk M, Villanger Y (2014) Tight bounds for parameterized complexity of cluster editing with a small number of clusters. J Comput Syst Sci 80(7):1430–1447
Giotis I, Guruswami V (2006) Correlation clustering with a fixed number of clusters. Theory Comput 2(13):249–266
Gramm J, Guo J, Hüffner F, Niedermeier R (2005) Graph-modeled data clustering: exact algorithms for clique generation. Theory Comput Syst 38(4):373–392
Gramm J, Guo J, Hüffner F, Niedermeier R (2004) Automated generation of search tree algorithms for hard graph modification problems. Algorithmica 39:321–347
Guo J (2009) A more effective linear kernelization for cluster editing. Theoret Comput Sci 410(8–10):718–726
Harary F (1953) On the notion of balance of a signed graph. Mich Math J 2(2):143–146
Hüffner F, Komusiewicz C, Moser H, Niedermeier R (2010) Fixed-parameter algorithms for cluster vertex deletion. Theory Comput Syst 47:196–217
Komusiewicz C, Uhlmann J (2012) Cluster editing with locally bounded modifications. Discrete Appl Math 160(15):2259–2270
Kováč I, Selečéniová I, Steinová M (2014) On the clique editing problem. In: Csuhaj-Varjú E, Dietzfelbinger M, Ésik Z (eds) Mathematical foundations of computer science 2014, vol 8635., Lecture notes in computer scienceSpringer, Berlin, pp 469–480
Schaeffer SE (2007) Graph clustering. Comput Sci Rev 1(1):27–64
Shamir R, Sharan R, Tsur D (2004) Cluster graph modification problems. Discrete Appl Math 144(1–2):173–182
Wasserman S, Faust K (1994) Social network analysis: methods and applications. Cambridge University Press, Cambridge
Wu BY, Chen LH (2015) Parameterized algorithms for the 2-clustering problem with minimum sum and minimum sum of squares objective functions. Algorithmica 72:818–835
Acknowledgments
This work was supported in part by NSC 101-2221-E-194-025-MY3 and MOST 103-2221-E-194-025-MY3 from National Science Council/Ministry of Science and Technology, Taiwan, R.O.C.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
We give a proof of that \( (1-x)^{(1/x)}< 1/e \) for all \(1>x>0\).
Let \(j=(1-x)^{(1/x)}\), i.e., \(j^x=1-x\). Taking derivatives on both sides, we have that \(\frac{dj^x}{dx}=\frac{d(1-x)}{dx}=-1\). Since
and \(j>0\), we have that
for all \(0<x<1\). The proof is completed by the following well-known identity Finney et al. (2001)
Rights and permissions
About this article
Cite this article
Chen, LH., Wu, B.Y. Parameterized algorithms for min–max 2-cluster editing. J Comb Optim 34, 47–63 (2017). https://doi.org/10.1007/s10878-016-0059-z
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10878-016-0059-z