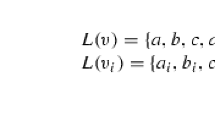Abstract
This paper considers the channel assignment problem in mobile communications systems. Suppose there are many base stations in an area, each of which demands a number of channels to transmit signals. The channels assigned to the same base station must be separated in some extension, and two channels assigned to two different stations that are within a distance must be separated in some other extension according to the distance between the two stations. The aim is to assign channels to stations so that the interference is controlled within an acceptable level and the spectrum of channels used is minimized. This channel assignment problem can be modeled as the multiple t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labeling of the interference graph. In this paper, we consider the case when all base stations demand the same number of channels. This case is referred as n-fold t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labeling of a graph. This paper first investigates the basic properties of n-fold t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labelings of graphs. And then it focuses on the special case when \(m=1\). The optimal n-fold t-separated L(j)-labelings of all complete graphs and almost all cycles are constructed. As a consequence, the optimal n-fold t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labelings of the triangular lattice and the square lattice are obtained for the case \(j_1=j_2=\cdots =j_m\). This provides an optimal solution to the corresponding channel assignment problems with interference graphs being the triangular lattice and the square lattice, in which each base station demands a set of n channels that are t-separated and channels from two different stations at distance at most m must be \(j_1\)-separated. We also study a variation of n-fold t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labeling, namely, n-fold t-separated consecutive \(L(j_1,j_2,\ldots ,j_m)\)-labeling. And present the optimal n-fold t-separated consecutive L(j)-labelings of all complete graphs and cycles.

Similar content being viewed by others
References
Audhya GK, Sinha K, Ghosh SC, Sinha BP (2011) A survey on the channel assignment problem in wireless networks. Wirel Commun Mob Comput 11:583–609
Calamoneri T (2011) The L(h, k)-labelling problem: an updated survey and annotated bibliography. Comput J 54(8):1344–1371
Campêloa M, Corrêa RC, Mourac PFS, Santos MC (2013) On optimal \(k\)-fold colorings of webs and antiwebs. Discrete Appl Math 161:60–70
Chvátal V, Garey MR, Johnson DS (1978) Two results concerning multicoloring. Ann Discrete Math 2:151–154
Díaz IM, Zabala P (1999) A generalization of the graph coloring problem. Investig Oper 8(1–3):167–184
Ghosh SC, Sinha BP, Das N (2003) Channel assignment using genetic algorithm based on geometric. IEEE Trans Veh Technol 52(4):860–875
Griggs JR, Jin XT (2007) Recent progress in mathematics and engineering on optimal graph labellings with distance conditions. J Comb Optim 14(2–3):249–257
Griggs JR, Yeh RK (1992) Labeling graphs with a condition at distance 2. SIAM J Discrete Math 5:586–595
Hale WK (1980) Frequency assignment: theory and applications. Proc IEEE 68:1497–1514
Janssen J (2002) Channel assignment and graph labeling. In: Handbook of wireless networks and mobile computing. John Wiley & Sons, Inc., pp 95–117
Johnson A, Holroyd FC, Stahl S (1997) Multichromatic numbers, star chromatic numbers and Kneser graphs. J Graph Theory 26:137–145
Khanna S, Kumaran K (1998) On wireless spectrum estimation and generalized graph coloring. In: Proceedings of IEEE INFOCOM98,
Klostermeyer W, Zhang CQ (2002) \(n\)-tuple coloring of planar graphs with large odd girth. Graphs and Comb 18:119–132
Lin W (2008) Multicolouring and Mycielski construction. Discrete Math 308:3565–3573
Lin W, Zhang P (2012) On \(n\)-fold \(L(j, k)\)- and circular \(L(j, k)\)-labeling of graphs. Discrete Appl Math 160(16–17):2452–2461
Lin W, Liu DDF, Zhu X (2010) Multi-colouring the Mycielskian of graphs. J Graph Theory 63(4):311–323
Pan Z, Zhu X (2010) Mutiple coloring of cone graphs. SIAM J Discrete Math 24(4):1515–1526
Ren G, Bu Y (2010) \(k\)-fold coloring of planar graphs. Sci China Math 53(10):2791–2800
Stahl S (1976) \(n\)-tuple colorings and associated graphs. J Comb Theory Ser B 20:185–203
Thevenin S, Zufferey N, Potvin J-Y (2016) Graph multi-coloring for a job scheduling application. Discrete Appl Math. https://doi.org/10.1016/j.dam.2016.05.023
van den Heuvel J, Leese RA, Shepherd MA (1998) Graph labeling and radio channel assignment. J Graph Theory 29:263–283
Yeh RK (2006) A survey on labeling graphs with a condition at distance two. Discrete Math 306:1217–1231
Zhang P, Lin W (2014) Multiple \(L(j,1)\)-labeling of the triangular lattice. J Comb Optim 27(4):695–710
Acknowledgements
The authors wish to thank the reviewers for many valuable suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Supported by Natural Science Foundation of Jiangsu Province of China (No. BK20151399) and NSFC 11771080.
Rights and permissions
About this article
Cite this article
Lin, W., Shen, C. Channel assignment problem and n-fold t-separated \(L(j_1,j_2,\ldots ,j_m)\)-labeling of graphs. J Comb Optim 35, 1147–1167 (2018). https://doi.org/10.1007/s10878-018-0255-0
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10878-018-0255-0