Abstract
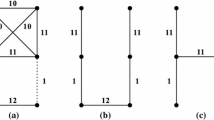
Given an edge weighted graph, and an acyclic edge set, the target of the partial inverse maximum spanning tree problem (PIMST) is to get a new weight function such that the given set is included in some maximum spanning tree associated with the new function, and the difference between the two functions is minimum. In this paper, we research PIMST under the Chebyshev norm. Firstly, the definition of extreme optimal solution is introduced, and its some properties are gained. Based on these properties, a polynomial scale optimal value candidate set is obtained. Finally, strongly polynomial-time algorithms for solving this problem are proposed. Thus, the computational complexity of PIMST is completely solved.


Similar content being viewed by others
Data availibility
Enquiries about data availability should be directed to the authors.
References
Ben-Ayed O, Blair CE (1990) Computational difficulties of bilevel linear programming. Oper Res 38(3):556–560
Bondy JA, Murty USR (2008) Graph theory. Springer, New York
Cai M-C, Duin CW, Yang X, Zhang J (2008) The partial inverse minimum spanning tree problem when weight increasing is forbidden. Eur J Oper Res 188:348–353
Gassner E (2010) The partial inverse minimum cut problem with \(L_1\)-norm is strongly NP-hard. RAIRO Oper Res 44:241–249
Guan X, He X, Pardalos PM, Zhang B (2017) Inverse max + sum spanning tree problem under Hamming distance by modifying the sum-cost vector. J Glob Optim 69(4):911–925
Guan X, Pardalos PM, Zhang B (2018) Inverse max + sum spanning tree problem under weighted \(l_1\) norm by modifying the sum-cost vector. Optim Lett 12(5):1065–1077
Guan X, Pardalos PM, Zuo X (2015) Inverse Max + Sum spanning tree problem by modifying the sum-cost vector under weighted \(l_\infty \) norm. J Glob Optim 61(1):165–182
Hansen P, Jaumard B, Savard G (1992) New branch-and-bound rules for linear bilevel programming. SIAM J Sci Stat Comput 13:1194–1217
He Y, Zhang B, Yao E (2005) Weighted inverse minimum spanning tree problems under hamming distance. J Comb Optim 9:91–100
Hochbaum DS (2003) Efficient algorithms for the inverse spanning-tree problem. Oper Res 51(5):785–797
Hochbaum DS, Queyranne M (2003) Minimizing a convex cost closure set. SIAM J Discrete Math 16(2):192–207
Lai T, Orlin J (2003) The complexity of preprocessing. Research Report of Sloan School of Management. MIT
Li S, Zhang Z, Lai H-J (2016) Algorithms for constraint partial inverse matroid problem with weight increase forbidden. Theor Comput Sci 640:119–124
Li X, Shu X, Huang H, Bai J (2019) Capacitated partial inverse maximum spanning tree under the weighted Hamming distance. J Comb Optim 38:1005–1018
Li X, Zhang Z, Du D-Z (2018) Partial inverse maximum spanning tree in which weight can only be decreased under \(l_p\)-norm. J Glob Optim 30:677–685
Li X, Zhang Z, Yang R, Zhang H, Du D-Z (2020) Approximation algorithms for capacitated partial inverse maximum spanning tree problem. J Glob Optim 77(2):319–340
Liu L, Wang Q (2009) Constrained inverse min–max spanning tree problems under the weighted Hamming distance. J Glob Optim 43:83–95
Liu L, Yao E (2008) Inverse min–max spanning tree problem under the Weighted sum-type Hamming distance. Theor Comput Sci 196:28–34
Sokkalingam PT, Ahuja RK, Orlin JB (1999) Solving inverse spanning tree problems through network flow techniques. Oper Res 47:291–298
Tayyebi J, Sepasian AR (2020) Partial inverse min–max spanning tree problem. J Comb Optim 40:1075–1091
Wang H, Guan X, Zhang Q, Zhang B (2021) Capacitated inverse optimal value problem on minimum spanning tree under bottleneck Hamming distance. J Comb Optim 41:861–887
Yang X (2001) Complexity of partial inverse assignment problem and partial inverse cut problem. RAIRO Oper Res 35:117–126
Yang X, Zhang J (2007) Partial inverse assignment problem under \(l_1\) norm. Oper Res Lett 35:23–28
Yang X, Zhang J (2007) Inverse sorting problem by minimizing the total weighted number of changers and partial inverse sorting problem. Comput Optim Appl 36(1):55–66
Zhang B, Guan X, Zhang Q (2020) Inverse optimal value problem on minimum spanning tree under unit \(l_{\infty }\)-norm. Optim Lett 14(8):2301–2322
Zhang B, Zhang J, He Y (2006) Constrained inverse minimum spanning tree problems under the bottleneck-type Hamming distance. J Glob Optim 34:467–474
Zhang J, Xu S, Ma Z (1997) An algorithm for inverse minimum spanning tree problem. Optim Methods Softw 8(1):69–84
Zhang Z, Li S, Lai H-J, Du D-Z (2016) Algorithms for the partial inverse matroid problem in which weights can only be increased. J Glob Optim 65(4):801–811
Acknowledgements
This research work is supported in part by National Natural Science Foundation of China (Nos. U20A2068, 12071194, 11871256 and 11771013), Basic Research Project of Qinghai (No. 2021-ZJ-703), and Zhejiang Provincial Natural Science Foundation of China (No. LD19A010001).
Funding
Funding was provided by National Natural Science Foundation of China (Grant Numbers U20A2068, 12071194, 11871256 and 11771013), Basic Research Project of Qinghai (Grant Number 2021-ZJ-703), and Zhejiang Provincial Natural Science Foundation of China (Grant Number LD19A010001).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors have not disclosed any competing interests.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Li, X., Yang, R., Zhang, H. et al. Partial inverse maximum spanning tree problem under the Chebyshev norm. J Comb Optim 44, 3331–3350 (2022). https://doi.org/10.1007/s10878-022-00903-9
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10878-022-00903-9