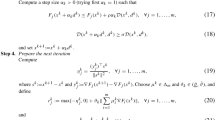Abstract
According to the characterization of eigenvalues of a real symmetric matrix A, the largest eigenvalue is given by the maximum of the quadratic form 〈xA, x〉 over the unit sphere; the second largest eigenvalue of A is given by the maximum of this same quadratic form over the subset of the unit sphere consisting of vectors orthogonal to an eigenvector associated with the largest eigenvalue, etc. In this study, we weaken the conditions of orthogonality by permitting the vectors to have a common inner product r where 0 ≤ r < 1. This leads to the formulation of what appears—from the mathematical programming standpoint—to be a challenging problem: the maximization of a convex objective function subject to nonlinear equality constraints. A key feature of this paper is that we obtain a closed-form solution of the problem, which may prove useful in testing global optimization software. Computational experiments were carried out with a number of solvers.
Similar content being viewed by others
References
Aitken A.C.: Determinants and Matrices. Oliver and Boyd, Edinburgh (1964)
Bellman R.: Introduction to Matrix Analysis. McGraw-Hill, New York (1960)
Floudas, C.A., Adjiman, C.S., Esposito, W.R., Gümüs, Z.H., Harding, S.T., Klepeis, J.L., Meyer, C.A., Schweiger,C.A. (eds.):Handbook of Test Problems in Local andGlobalOptimization. Kluwer Academic Publishers, Boston (1999)
Hardy G.H., Littlewood J.E., Pólya G.: Inequalities, 2nd edn. Cambridge University Press, Cambridge (1959)
Marshall A.W., Olkin I.: Theory of Majorization and its Applications. Academic Press, London (1979)
Parker W.V.: The characteristic roots of matrices. Duke Math. J. 12, 519–526 (1945)
von Neumann J.: Some matrix inequalities and metrization of matrix-space. Tomsk Univ. Rev. 1, 286–300 (1937)
Author information
Authors and Affiliations
Corresponding author
Additional information
We dedicate this paper to the memory of our great friend and colleague, Gene H. Golub.
Rights and permissions
About this article
Cite this article
Cottle, R.W., Olkin, I. Closed-form solution of a maximization problem. J Glob Optim 42, 609–617 (2008). https://doi.org/10.1007/s10898-008-9338-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10898-008-9338-2
Keywords
- Constrained optimization
- Test problem
- Quadratic forms
- Intraclass correlation matrices
- Eigenvalues
- Eigenvectors