Skip to main content
Springer Nature Link
Log in

Equal Risk Bounding is better than Risk Parity for portfolio selection

  • Published:
Journal of Global Optimization Aims and scope Submit manuscript

Abstract

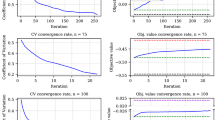
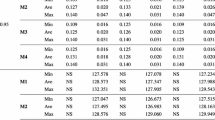
Risk Parity (RP), also called equally weighted risk contribution, is a recent approach to risk diversification for portfolio selection. RP is based on the principle that the fractions of the capital invested in each asset should be chosen so as to make the total risk contributions of all assets equal among them. We show here that the Risk Parity approach is theoretically dominated by an alternative similar approach that does not actually require equally weighted risk contribution of all assets but only an equal upper bound on all such risks. This alternative approach, called Equal Risk Bounding (ERB), requires the solution of a nonconvex quadratically constrained optimization problem. The ERB approach, while starting from different requirements, turns out to be strictly linked to the RP approach. Indeed, when short selling is allowed, we prove that an ERB portfolio is actually an RP portfolio with minimum variance. When short selling is not allowed, there is a unique RP portfolio and it contains all assets in the market. In this case, the ERB approach might lead to the RP portfolio or it might lead to portfolios with smaller variance that do not contain all assets, and where the risk contributions of each asset included in the portfolio is strictly smaller than in the RP portfolio. We define a new riskiness index for assets that allows to identify those assets that are more likely to be excluded from the ERB portfolio. With these tools we then provide an exact method for small size nonconvex ERB models and a very efficient and accurate heuristic for larger problems of this type. In the case of a common constant pairwise correlation among all assets, a closed form solution to the ERB model is obtained and used to perform a parametric analysis when varying the level of correlation. The practical advantages of the ERB approach over the RP strategy are illustrated with some numerical examples. Computational experience on real-world and on simulated data confirms accuracy and efficiency of our heuristic approach to the ERB model also in comparison with some state-of-the-art local and global optimization codes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Notes

  1. See also http://www.riskparity.com/, http://www.thierry-roncalli.com/riskparity.html, and references therein.

References

  1. Anderson, R.M., Bianchi, S.W., Goldberg, L.R.: Will my risk parity strategy outperform? Financ. Anal. J. 68(6), 75–93 (2012)

    Article  Google Scholar 

  2. Aneja, Y.P., Chandra, R., Gunay, E.: A portfolio approach to estimating the average correlation coefficient for the constant correlation model. J. Financ. 44, 1435–1438 (1989)

    Article  Google Scholar 

  3. Asness, C.S., Frazzini, A., Pedersen, L.H.: Leverage aversion and risk parity. Financ. Anal. J. 68(1), 47–59 (2012)

    Article  Google Scholar 

  4. Bai, X., Scheinberg, K., Tutuncu, R.: Least-squares approach to risk parity in portfolio selection. Quant. Financ. 16, 357–376 (2016)

    Article  MathSciNet  Google Scholar 

  5. Bertrand, P., Lapointe, V.: How performance of risk-based strategies is modified by socially responsible investment universe? Int. Rev. Financ. Anal. 38, 175–190 (2015)

    Article  Google Scholar 

  6. Boros, E., Hammer, P.L.: Pseudo-boolean optimization. Discrete. Appl. Math. 123, 155–225 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  7. Boudt, K., Carl, P., Peterson, B.: Asset allocation with conditional value-at-risk budgets. J. Risk 15, 39–68 (2013)

    Article  Google Scholar 

  8. Cesarone, F., Colucci, S.: Minimum Risk vs. Capital and Risk Diversification strategies for portfolio construction. J. Oper. Res. Soc. SSRN: http://ssrn.com/abstract=2552455 (2015) (submitted)

  9. Cesarone, F., Scozzari, A., Tardella, F.: A new method for mean-variance portfolio optimization with cardinality constraints. Ann. Oper. Res. 205, 213–234 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  10. Cesarone, F., Scozzari, A., Tardella, F.: Linear vs. quadratic portfolio selection models with hard real-world constraints. Comput. Manage. Sci. 12(3), 345–370 (2015)

    MATH  Google Scholar 

  11. Chan-Lau, J.A.: Frontier equity markets: risk parity lessons for asset allocation. J. Altern. Invest 16(4), 28–36 (2014)

    Article  Google Scholar 

  12. Chaves, D., Hsu, J., Li, F., Shakernia, O.: Risk parity portfolio vs. other asset allocation heuristic portfolios. J. Invest. 20(1), 108–118 (2011)

    Article  Google Scholar 

  13. Clarke, R., De Silva, H., Thorley, S.: Risk parity, maximum diversification, and minimum variance: An analytic perspective. J. Portf. Manag. 39(3), 39–53 (2013)

    Article  Google Scholar 

  14. Corkery, M., Cui, C., Grind, K.: Fashionable “risk parity” funds hit hard. Wall Street J. (2013). http://www.wsj.com/articles/SB10001424127887323689204578572050047323638

  15. Dagher, V.: New allocation funds redefine idea of ’balance’. Wall Street J. (2012). http://www.wsj.com/articles/SB10001424052970204542404577158970902886322

  16. DeMiguel, V., Garlappi, L., Uppal, R.: Optimal versus naive diversification: How inefficient is the 1/N portfolio strategy? Rev. Financ. Stud. 22, 1915–1953 (2009)

    Article  Google Scholar 

  17. Elton, E.J., Gruber, M.J.: Estimating the dependence structure of share prices—implications for portfolio selection. J. Financ. 28, 1203–1232 (1973)

    Google Scholar 

  18. Elton, E.J., Gruber, M.J., Padberg, M.W.: Simple criteria for optimal portfolio selection. J. Financ. 31, 1341–1357 (1976)

    Article  Google Scholar 

  19. Elton, E.J., Gruber, M.J., Spitzer, J.: Improved estimates of correlation coefficients and their impact on optimum portfolios. Eur. Financ. Manag. 12, 303–318 (2006)

    Article  Google Scholar 

  20. Lee, W.: Risk-based asset allocation: A new answer to an old question? J. Portf. Manag. 37, 11–12 (2011)

    Article  Google Scholar 

  21. Lohre, H., Opfer, H., Orszag, G.: Diversifying risk parity. J. Risk 16(5), 53–79 (2014)

    Article  Google Scholar 

  22. Maillard, S., Roncalli, T., Teiletche, J.: The properties of equally weighted risk contribution portfolios. J. Portf. Manag. 36, 60–70 (2010)

    Article  Google Scholar 

  23. Pflug, G.C., Pichler, A., Wozabal, D.: The 1/N investment strategy is optimal under high model ambiguity. J. Bank Financ. 36, 410–417 (2012)

    Article  Google Scholar 

  24. Qian, E.: Risk parity and diversification. J. Invest. 20(1), 119–127 (2011)

  25. Qian, E.: Risk parity portfolios: Efficient portfolios through true diversification. Panagora Asset Management, September (2005)

  26. Roncalli, T.: Introduction to Risk Parity and Budgeting. Chapman & Hall/CRC Financial Mathematics Series. CRC Press, Boca Raton, FL (2014)

    MATH  Google Scholar 

  27. Scherer, B.: Portfolio Construction and Risk Budgeting, 3rd edn. Risk Books, London (2007)

    MATH  Google Scholar 

  28. Sorensen, E.H., Alonso, N.F.: The resale value of risk-parity equity portfolios. J. Portf. Manag. 41, 23–32 (2015)

    Article  Google Scholar 

  29. Spinu, F.: An algorithm for computing risk parity weights. SSRN: http://ssrn.com/abstract=2297383 (July 30, 2013)

  30. Tawarmalani, M., Sahinidis, N.V.: A polyhedral branch-and-cut approach to global optimization. Math. Prog. 103(2), 225–249 (2005)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

We are grateful to the anonymous reviewers for carefully reading our work and for suggesting several improvements. In particular, one of the reviewers prompted us to extend our original approach for the long-only case to the case where short sellings are allowed.

Author information

Authors and Affiliations

  1. Dip. di Studi Aziendali, Università degli Studi Roma Tre, Rome, Italy

    Francesco Cesarone

  2. Dip. di Metodi e Modelli per l’Economia, il Territorio e la Finanza, Sapienza Università di Roma, Rome, Italy

    Fabio Tardella

Authors
  1. Francesco Cesarone
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Fabio Tardella
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Francesco Cesarone.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Supplementary material 1 (xlsx 19 KB)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cesarone, F., Tardella, F. Equal Risk Bounding is better than Risk Parity for portfolio selection. J Glob Optim 68, 439–461 (2017). https://doi.org/10.1007/s10898-016-0477-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10898-016-0477-6

Keywords