Abstract
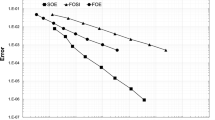
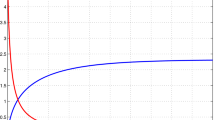
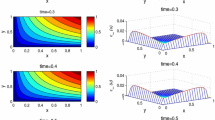
In this paper we develop a high order explicit finite difference weighted essentially non-oscillatory (WENO) scheme for solving a hierarchical size-structured population model with nonlinear growth, mortality and reproduction rates. The main technical complication is the existence of global terms in the coefficient and boundary condition for this model. We carefully design approximations to these global terms and boundary conditions to ensure high order accuracy. Comparing with the first order monotone and second order total variation bounded schemes for the same model, the high order WENO scheme is more efficient and can produce accurate results with far fewer grid points. Numerical examples including one in computational biology for the evolution of the population of Gambussia affinis, are presented to illustrate the good performance of the high order WENO scheme.
Similar content being viewed by others
References
Ackleh, A.S., Deng, K., Hu, S.: A quasilinear hierarchical size structured model: well-posedness and approximation. App. Math. Optim. 51, 35–59 (2005)
Angulo, O., Durán, A., López-Marcos, J.C.: Numerical study of size-structured population models: a case of Gambussia affinis. C. R. Biol. 328, 387–402 (2005)
Balsara, D.S., Shu, C.-W.: Monotonicity preserving weighted essentially non-oscillatory schemes with increasingly high order of accuracy. J. Comput. Phys. 160, 405–452 (2000)
Blayneh, K.W.: Hierarchical size-structured population model. Dyn. Syst. Appl. 9, 527–540 (2002)
Calsina, A., Saldana, J.: Asymptotic behavior of a model of hierarchically structured population dynamics. J. Math. Biol. 35, 967–987 (1997)
Cushing, J.M.: The dynamics of hierarchical age-structured population. J. Math. Biol. 32, 705–729 (1994)
Cushing, J.M., Li, J.: Juvenile versus adult competition. J. Math. Biol. 29, 457–473 (1991)
Gottlieb, S., Shu, C.-W.: Total variation diminishing Runge–Kutta schemes. Math. Comput. 67, 73–85 (1998)
Gottlieb, S., Shu, C.-W., Tadmor, E.: Strong stability preserving high order time discretization methods. SIAM Rev. 43, 89–112 (2001)
Henson, S.M., Cushing, J.M.: Hierarchical models of intra-specific competition: scramble versus contest. J. Math. Biol. 34, 755–772 (1996)
Jiang, G., Shu, C.-W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126, 202–228 (1996)
Kraev, E.A.: Existence and uniqueness for height structured hierarchical population models. Nat. Res. Model. 14, 45–70 (2001)
Krumholtz, L.A.: Reproduction in the western mosquitofish, Gambusia affinis (Baird and Girard), and its use in mosquito control. Ecol. Monogr. 18, 1–43 (1948)
Shen, J., Shu, C.-W., Zhang, M.: High resolution schemes for a hierarchical size-structured model. SIAM J. Numer. Anal. 45, 352–370 (2007)
Shi, J., Hu, C., Shu, C.-W.: A technique of treating negative weights in WENO schemes. J. Comput. Phys. 175, 108–127 (2002)
Shu, C.-W.: Essentially non-oscillatory and weighted essentially non-oscillatory schemes for hyperbolic conservation laws. In: Quarteroni, A. (ed.) Advanced Numerical Approximation of Nonlinear Hyperbolic Equations, Lecture Notes in Mathematics, vol. 1697, pp. 325–432. Springer, Berlin (1998)
Shu, C.-W.: High-order finite difference and finite volume WENO schemes and discontinuous Galerkin methods for CFD. Int. J. Comput. Fluid Dyn. 17, 107–118 (2003)
Shu, C.-W., Osher, S.: Efficient implementation of essentially non-oscillatory shock-capturing schemes. J. Comput. Phys. 77, 439–471 (1988)
Weiner, J., Thomas, S.X.: Size variability and competition in plant monocultures. Oikos 47, 211–222 (1986)
Author information
Authors and Affiliations
Corresponding author
Additional information
Chi-Wang Shu was supported in part by NSFC grant 10671190 while he was visiting the Department of Mathematics, University of Science and Technology of China, Hefei, Anhui 230026, China. Additional support was provided by ARO grant W911NF-04-1-0291 and NSF grant DMS-0510345.
Mengping Zhang was supported in part by NSFC grant 10671190.
Rights and permissions
About this article
Cite this article
Shen, J., Shu, CW. & Zhang, M. A High Order WENO Scheme for a Hierarchical Size-Structured Population Model. J Sci Comput 33, 279–291 (2007). https://doi.org/10.1007/s10915-007-9152-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-007-9152-x