Skip to main content
Springer Nature Link
Log in

A Fast Explicit Operator Splitting Method for Passive Scalar Advection

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

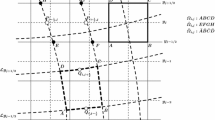
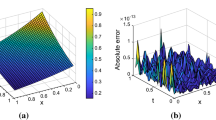
Abstract

The dispersal and mixing of scalar quantities such as concentrations or thermal energy are often modeled by advection-diffusion equations. Such problems arise in a wide variety of engineering, ecological and geophysical applications. In these situations a quantity such as chemical or pollutant concentration or temperature variation diffuses while being transported by the governing flow. In the passive scalar case, this flow prescribed and unaffected by the scalar. Both steady laminar and complex (chaotic, turbulent or random) time-dependent flows are of interest and such systems naturally lead to questions about the effectiveness of the stirring to disperse and mix the scalar. The development of reliable numerical methods for advection-diffusion equations is crucial for understanding their properties, both physical and mathematical. In this paper, we extend a fast explicit operator splitting method, recently proposed in (A. Chertock, A. Kurganov, G. Petrova, Int. J. Numer. Methods Fluids 59:309–332, 2009), for solving deterministic convection-diffusion equations, to the problems with random velocity fields and singular source terms. A superb performance of the method is demonstrated on several two-dimensional examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Chertock, A., Kashdan, E., Kurganov, A.: In: Benzoni-Gavage, S., Serre, D. (eds.) Propagation of Diffusing Pollutant by a Hybrid Eulerian-Lagrangian Method. Hyperbolic Problems: Theory, Numerics, Applications, Lyon, 2006, pp. 371–380. Springer, Berlin (2008)

    Google Scholar 

  2. Chertock, A., Kurganov, A.: On splitting-based numerical methods for convection-diffusion equations. In: Puppo, G., Russo, G. (eds.) Numerical Methods for Balance Laws, Aracne editrice S.r.l., Rome (2010)

    Google Scholar 

  3. Chertock, A., Kurganov, A., Petrova, G.: Fast explicit operator splitting method. Application to the polymer system. In: Benkhaldoun, F., Ouazar, D., Raghay, S. (eds.) Finite Volumes for Complex Applications, vol. IV, pp. 63–72. Hermes Science Publishing, London (2005)

    Google Scholar 

  4. Chertock, A., Kurganov, A., Petrova, G.: Fast explicit operator splitting method for convection-diffusion equations. Int. J. Numer. Meth. Fluids 59, 309–332 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  5. Cockburn, B., Johnson, C., Shu, C.-W., Tadmor, E.: Advanced numerical approximation of nonlinear hyperbolic equations. In: Quarteroni, A. (ed.) CIME Lecture Notes. Lecture Notes in Mathematics, vol. 1697. Springer, Berlin (1997)

    Google Scholar 

  6. Doering, C.R., Thiffeault, J.-L.: Multiscale mixing efficiencies for steady sources. Phys. Rev. E 74, 025301(R) (2006)

    Article  Google Scholar 

  7. Drummond, I.T.: Path-integral methods for turbulent diffusion. J. Fluid Mech. 123, 59–68 (1982)

    Article  MATH  MathSciNet  Google Scholar 

  8. Godlewski, E., Raviart, P.-A.: Numerical Approximation of Hyperbolic Systems of Conservation Laws. Applied Mathematical Sciences, vol. 118. Springer, New York (1996)

    MATH  Google Scholar 

  9. Gottlieb, S., Shu, C.-W., Tadmor, E.: Strong stability-preserving high-order time discretization methods. SIAM Rev. 43, 89–112 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  10. Hundsdorfer, W., Verwer, J.: Numerical Solution of Time-dependent Advection-Diffusion-Reaction Equations. Springer Series in Computational Mathematics, vol. 33. Springer, Berlin (2003)

    MATH  Google Scholar 

  11. Kröner, D.: Numerical Schemes for Conservation Laws. Wiley-Teubner Series Advances in Numerical Mathematics. John Wiley & Sons Ltd., Chichester (1997)

    MATH  Google Scholar 

  12. Kurganov, A., Lin, C.-T.: On the reduction of numerical dissipation in central-upwind schemes. Commun. Comput. Phys. 2, 141–163 (2007)

    MATH  MathSciNet  Google Scholar 

  13. Kurganov, A., Noelle, S., Petrova, G.: Semi-discrete central-upwind scheme for hyperbolic conservation laws and Hamilton-Jacobi equations. SIAM J. Sci. Comput. 23, 707–740 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  14. Kurganov, A., Tadmor, E.: New high resolution central schemes for nonlinear conservation laws and convection-diffusion equations. J. Comput. Phys. 160, 241–282 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  15. Kurganov, A., Tadmor, E.: Solution of two-dimensional Riemann problems for gas dynamics without Riemann problem solvers. Numer. Methods Partial Differ. Equ. 18, 584–608 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  16. Lee, J., Fornberg, B.: A split step approach for the 3-D Maxwell’s equations. J. Comput. Appl. Math. 158(2), 485–505 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  17. LeVeque, R.J.: Finite Volume Methods for Hyperbolic Problems. Cambridge Texts in Applied Mathematics. Cambridge University Press, Cambridge (2002)

    Book  MATH  Google Scholar 

  18. Lie, K.-A., Noelle, S.: On the artificial compression method for second-order nonoscillatory central difference schemes for systems of conservation laws. SIAM J. Sci. Comput. 24(4), 1157–1174 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  19. Majda, A.J., Kramer, P.R.: Simplified models for turbulent diffusion: theory, numerical modelling, and physical phenomena. Phys. Rep. 314(4–5), 237–574 (1999)

    Article  MathSciNet  Google Scholar 

  20. Marchuk, G.I.: Metody Rasshchepleniya (Russian) [Splitting Methods] Nauka, Moscow (1988)

    MATH  Google Scholar 

  21. Marchuk, G.I.: Splitting and alternating direction methods. In: Handbook of Numerical Analysis, vol. I, pp. 197–462. North-Holland, Amsterdam (1990)

    Google Scholar 

  22. Medovikov, A.A.: DUMKA 3 code, available at http://dumkaland.org/

  23. Medovikov, A.A.: High order explicit methods for parabolic equations. BIT 38(2), 372–390 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  24. Nessyahu, H., Tadmor, E.: Nonoscillatory central differencing for hyperbolic conservation laws. J. Comput. Phys. 87(2), 408–463 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  25. Okabe, T., Eckhardt, B., Thiffeault, J.-L., Doering, C.R.: Mixing effectiveness depends on the source-sink structure: simulation results. J. Stat. Mech. Theory Exp. P07018, 1–13 (2008)

    Google Scholar 

  26. Plasting, S.C., Young, W.R.: A bound on scalar variance for the advection-diffusion equation. J. Fluid Mech. 552, 289–298 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  27. Russo, G.: Central schemes and systems of balance laws. In: Hyperbolic Partial Differential Equations, Hamburg, 2001, pp. 59–114. Vieweg, Braunschweig (2002)

    Google Scholar 

  28. Shaw, T.A., Thiffeault, J.-L., Doering, C.R.: Stirring up trouble: multi-scale mixing measures for steady scalar sources. Phys. D 231(2), 143–164 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  29. Strang, G.: On the construction and comparison of difference schemes. SIAM J. Numer. Anal. 5, 506–517 (1968)

    Article  MATH  MathSciNet  Google Scholar 

  30. Sweby, P.K.: High resolution schemes using flux limiters for hyperbolic conservation laws. SIAM J. Numer. Anal. 21(5), 995–1011 (1984)

    Article  MATH  MathSciNet  Google Scholar 

  31. Yoshida, H.: Construction of higher order symplectic integrators. Phys. Lett. A 150(5–7), 262–268 (1990)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, North Carolina State University, Raleigh, NC, 27695, USA

    Alina Chertock

  2. Departments of Mathematics & Physics, University of Michigan, Ann Arbor, MI, 48109-1043, USA

    Charles R. Doering

  3. Department of Applied Mathematics, Tel Aviv University, Tel Aviv, Ramat Aviv, 69978, Israel

    Eugene Kashdan

  4. Department of Mathematics, Tulane University, New Orleans, LA, 70118, USA

    Alexander Kurganov

Authors
  1. Alina Chertock
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Charles R. Doering
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Eugene Kashdan
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Alexander Kurganov
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Alina Chertock.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Chertock, A., Doering, C.R., Kashdan, E. et al. A Fast Explicit Operator Splitting Method for Passive Scalar Advection. J Sci Comput 45, 200–214 (2010). https://doi.org/10.1007/s10915-010-9381-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-010-9381-2

Keywords