Abstract
This work is devoted to the study of the numerical treatment of linear elliptic problems in adjoined domains nonlinearly coupled at the interface. The problem arises in semi-discretization of mass diffusion problems typically when an osmotic effect is taken into account. Convergence of both the Conjugate Gradient and the Fixed Point method are considered and compared.


Similar content being viewed by others
Notes
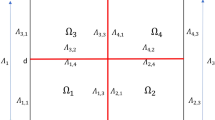
The case of non conformal mesh for the other type of domains was not reported because the zones of overlap of the triangles are difficult to treat and the two algorithms converge to different solutions; in this case we would need a finite element method that could exactly describe the geometry as is in the Isogeometric modelling. For an application of Isogeometric Analysis to a similar problem see [22].
References
Bresch, D., Koko, J.: An optimization-based domani decomposition method for nonlinear wall laws in coupled systems. M3AS 14(7), 1085–1101 (2004)
Calabrò, F., Zunino, P.: Analysis of parabolic problems on partitioned domains with nonlinear conditions at the interface, applications to mass transfer through semi-permeable, membranes. M3AS 16(4), 1–23 (2006)
Canuto, C., Urban, K.: Adaptive optimization of convex functionals in Banach spaces. SIAM J. Numer. Anal. 42(5), 2043–2075 (2005)
Du, Q.: Optimization based nonoverlapping domain decomposition algorithms and their convergence. SIAM J. Numer. Anal. 39(3), 1056–1077 (2001) (electronic). MR MR1860457 (2003b:65117)
Du, Q., Gunzburger, M.D.: A gradient method approach to optimization-based multidisciplinary simulations and nonoverlapping domain decomposition algorithms. SIAM J. Numer. Anal. 37(5), 1513–1541 (2000) (electronic). MR MR1759905 (2001d:65162)
Gervasio, P., Lions, J.-L., Quarteroni, A.: Heterogeneous coupling by virtual control methods. Numer. Math. 90(2), 241–264 (2001)
Gunzburger, M.D., Peterson, J.S., Kwon, H.: An optimization based domain decomposition method for partial differential equations. Comput. Math. Appl. 37(10), 77–93 (1999)
Gunzburger, M.D., Lee, H.K.: An optimization-based domain decomposition method for the Navier-Stokes equations. SIAM J. Numer. Anal. 37(5), 1455–1480 (2000) (electronic)
Hecht, F., Bernardi, D., Ohtsuka, K., Pironneau, O., Morice, J., Le Hyaric, A.: http://www.freefem.org/ff++/
Hetzer, G., Meir, A.J.: On an interface problem with a nonlinear jump condition, numerical approximation of solutions. Int. J. Numer. Anal. Model. 4(3–4), 519–530 (2007)
Hron, J., Neuss-Radu, M., Pustějovská, P.: Mathematical modeling and simulation of flow in domains separated by leaky semipermeable membrane including osmotic effect. Appl. Math. 56(1), 51–68 (2011)
Kedem, O., Katchalsky, A.: Thermodynamic analysis of the permeability of biological membranes to non-electrolytes. Biochimica et Biophysica Acta. 37, 229–246 (1958)
Koko, J.: Uzawa conjugate gradient domain decomposition methods for coupled stokes flows. J. Sci. Comput. 26(2), 195–216 (2006)
Koko, Jonas: Convergence analysis of optimization-based domain decomposition methods for a bonded structure. Appl. Numer. Math. 58(1), 69–87 (2008)
Lee, H.K.: An optimization-based domain decomposition method for a nonlinear problem. Appl. Math. Comput. 113(1), 23–42 (2000)
Lui, S.H.: On linear monotone iteration and Schwarz methods for nonlinear elliptic PDEs. Numer. Math. 93(1), 109–129 (2002)
Neuss-Radu, M., Jäger, W.: Effective transmission conditions for reaction-diffusion processes in domains separated by an interface. SIAM J. Math. Anal. 39(3), 687–720 (2007) (electronic)
Pao, C.V.: Nonlinear Parabolic and Elliptic Equations. Plenum Press, New York (1992)
Quarteroni, A., Sacco, R., Saleri, F.: Numerical Mathematics, 2nd edn, Texts in Applied Mathematics, vol. 37. Springer, Berlin (2007)
Quarteroni, A., Valli, A.: Numerical Approximation of Partial Differential Equations, Springer Series in Computational Mathematics, vol. 23. Springer, Berlin (1994)
Quarteroni, A., Veneziani, A., Zunino, P.: Mathematical and numerical modeling of solute dynamics in blood flow and arterial walls. SIAM J. Numer. Anal. 39(5), 1488–1511 (2002)
van der Zee, K.G., Verhoosel, C.V.: Isogeometric analysis-based goal-oriented error estimation for free-boundary problems. Finite Elem. Anal. Des. 47(6), 600–609 (2011)
Vurro, M., Castellano, L.: Numerical treatments of the interface discontinuity in solid-water mass transfer problems. Comput. Math. Appl. 45(4–5), 785–788 (2003)
Acknowledgments
The author is grateful to Paolo Zunino (Politecnico di Milano) for introducing this topic and sharing techniques suitable to deal with it. He also wish to thank the referees for carefully reading the original manuscript and suggesting numerous improvements.
Author information
Authors and Affiliations
Corresponding author
Appendix: Fixed Point Iteration
Appendix: Fixed Point Iteration
In this section we consider a fixed point procedure for problem (1.1).
Recall definition (2.1) and consider:
For our analysis we need to assume that \(g(\zeta ,\xi )\) is locally lipschitz:
In this hypothesis we can prove that the fixed point iterations are convergent. Our proof relies on a classic trace estimate, see ad example [20]:
Theorem 4.1
(Trace inequality) Let \(\Omega \) be regular and take \(v \in H^1(\Omega )\). Then \(\forall \varepsilon >0\) there is \(K_\varepsilon \) such that:
In order to prove convergence, take \(e_i^n=u_i^{(n)}-u_i\). By Eq. (4.1), function \(e_i^n\) solves the following problem:
Now, taking in the previous equation as test function \(v_i\) the same function \(e_i^n\) and summing the two one obtains:
Now we notice that \(a_i(e^n_i, e^n_i) \equiv \Vert \nabla e^n_i \Vert ^2_{L^2 (\Omega _i)}\) and \( \alpha (e^n_i, e^n_i)_{\Omega _i} \equiv \alpha \Vert e^n_i \Vert ^2_{L^2 (\Omega _i)}\) thus the previous can be written as:
In order to estimate the right hand side, we use the lipschitz condition, the triangular, Jensen and Joung inequalities to obtain:
Summarizing we get:
Now we can use Theorem 3.1 at \(\Vert e_i^n \Vert _{L^2(\Gamma )} \) with \(\alpha = \dfrac{K_\varepsilon }{\varepsilon } \) and \(k_1= \dfrac{1}{\varepsilon }= \displaystyle \left\{ \dfrac{\Vert l_K \Vert _{L^1(\Gamma )}}{2}\right. \)+ \(\left. \gamma \Vert l_K \Vert _{L^1(\Gamma )} \right\} \), where \(\gamma >1\) is a parameter.
With all these positions we have:
Substituting this we have:
Now we can conclude on convergence because the parameter \(\gamma \) has been chosen \(\gamma >1\).
Rights and permissions
About this article
Cite this article
Calabrò, F. Numerical Treatment of Elliptic Problems Nonlinearly Coupled Through the Interface. J Sci Comput 57, 300–312 (2013). https://doi.org/10.1007/s10915-013-9706-z
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-013-9706-z