Abstract
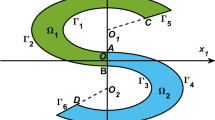
In this paper, we propose a domain decomposition spectral method for exterior problems with arbitrary polygonal obstacles. Some results on the composite Legendre–Laguerre quasi-orthogonal approximation are established, which play important roles in the spectral method for exterior problems. As examples of applications, the composite spectral schemes are provided for two model problems, with the convergence analysis. Numerical results demonstrate the spectral accuracy of this new approach. The approximation results and techniques developed in this paper are also applicable to other problems defined on unbounded domains with complex geometry.



Similar content being viewed by others
References
Bernardi, C., Maday, Y.: Spectral methods. In: Ciarlet, P.G., Lions, J.L. (eds.) Handbook of Numerical Analysis, pp. 209–486. Elsevier, Amsterdam (1997)
Coulaud, O., Funaro, D., Kavian, O.: Laguerre spectral approximation of elliptic problems in exterior domains. Comput. Mech. Appl. Mech. Eng. 80, 451–458 (1990)
Canuto, C., Hussaini, M.Y., Quarteroni, A., Zang, T.A.: Spectral Methods: Fundamentals in Single Domains. Springer, Berlin (2006)
Canuto, C., Hussaini, M.Y., Quarteroni, A., Zang, T.A.: Spectral Methods: Evolution to complex Geometries and Applications to Fluid Dynamics. Springer, Berlin (2007)
Funaro, D.: Polynomial Approxiamtions of Differential Equations. Springer, Berlin (1992)
Funaro, D., Kavian, O.: Approximation of some diffusion evolution equation in unbounded domains by Hermite function. Math. Comput. 57, 597–619 (1999)
Gottlieb, D., Orszag, S.A.: Numerical Analysis of Spectral Methods: Theory and Applications. SIAM-CBMS, Philadelphia (1977)
Guo, B.-Y.: Spectral Methods and Their Applications. World Scientific, Singapore (1998)
Guo, B.-Y.: Error estimation of Hermite spectral method for nonlinear partial differential equations. Math. Comput. 68, 1067–1078 (1999)
Guo, B.-Y.: Gegenbauer approximation in certain Hilbert spaces and its applications to singular differential equations. SIAM J. Numer. Anal. 37, 621–645 (2000)
Guo, B.-Y.: Jacobi approximations in certain Hilbert spaces and their applications to singular differential equations. J. Math. Anal. Appl. 243, 373–408 (2000)
Guo, B.-Y.: Some progress in spectral methods. Sci. China Math. DOI:10.1007/s11425-013-4660-7
Guo, B.-Y., Jia, H.-L.: Spectral method on quadrilaterals. Math. Comput. 79, 2237–2264 (2010)
Guo, B.-Y., Ma, H.-P.: Composite Legendre-Laguerre approximation in unbounded domains. J. Comput. Math. 19, 101–112 (2001)
Guo, B.-Y., Shen, J.: Laguerre–Galerkin method for nonlinear partial differential equations on a semi-infinite interval. Numer. Math. 86, 635–654 (2000)
Guo, B.-Y., Shen, J., Wang, L.-L.: Optimal spectral-Galerkin methods using generalized Jacobi polynomials. J. Sci. Comput. 27, 305–322 (2006)
Guo, B.-Y., Shen, J., Xu, C.-L.: Generalized Laguerre approximation and its applications to exterior problems. J. Comput. Math. 23, 113–130 (2005)
Guo, B.-Y., Sun, T., Zhang, C.: Jacobi and Laguerre quasi-orthogonal approximations and related interpolations. Math. Comput. 82, 413–441 (2013)
Guo, B.-Y., Wang, L.-L.: Jacobi approximations in non-uniformly Jacobi-weighted Sobolev spaces. J. Approx. Theory 128, 1–41 (2004)
Guo, B.-Y., Wang, L.-L., Wang, Z.-Q.: Generalized Laguerre interpolation and pseudospectral method for unbounded domains. SIAM J. Numer. Anal. 43, 2567–2589 (2006)
Guo, B.-Y., Wang, T.-J.: Composite generalized Laguerre–Legendre spectral method with its application to Fokker–Planck equation in an finite channel. Math. Comput. 78, 129–151 (2009)
Guo, B.-Y., Wang, T.-J.: Composite Laguerre–Legendre spectral method for exterior problems. Adv. Comput. Math. 32, 393–429 (2010)
Guo, B.-Y., Xu, C.-L.: Hermite pseudospectral method for nonlinear partial differential equations. RAIRO Math. Model Numer. Anal. 34, 859–872 (2000)
Guo, B.-Y., Zhang, K.-J: On non-isotropic Jacobi pseudospectral method. J. Comput. Math. 26, 511–535 (2008)
Guo, B.-Y., Zhang, X.-Y.: A new generalized Laguerre approximation and its applications. J. Comput. Appl. Math. 181, 342–363 (2007)
Guo, B.-Y., Zhang, X.-Y.: Spectral method for differential equations of degenerate type by using generalized Laguerre functions. Appl. Numer. Math. 57, 455–471 (2007)
Jia, H.-L., Guo, B.-Y.: Petrov-Galerkin spectral element method for mixed inhomogeneous boundary value problems on polygons. Chin. Ann. Math. 31B, 855–878 (2010)
Karniadakis, G.E., Sherwin, S.J.: Spectral/hp Element Methods for CFD, 2nd edn. Oxford Univ. Press, Oxford (2005)
Maday, Y., Pernaud-Thomas, B., Vandeven, H.: Oneréhabilitation des méthods spèctrales de type Laguerre. Rech. Aréospat. 6, 353–379 (1985)
Shen, J.: Stable and efficient spectral methods in unbounded domains using Laguerre functions. SIAM J. Numer. Anal. 38, 1113–1133 (2000)
Shen, J., Tang, T., Wang, L.-L.: Spectral Methods: Algorithms. Analysis and Applications. Springer, Berlin (2011)
Author information
Authors and Affiliations
Corresponding author
Additional information
The work of this author is supported in part by NSF of China N.11171227, Fund for Doctoral Authority of China N.20123127110001, Fund for E-institute of Shanghai Universities N.E03004, and Leading Academic Discipline Project of Shanghai Municipal Education Commission N.J50101.
Rights and permissions
About this article
Cite this article
Guo, BY., Yu, XH. Composite Spectral Method for Exterior Problems with Polygonal Obstacles. J Sci Comput 59, 439–472 (2014). https://doi.org/10.1007/s10915-013-9769-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-013-9769-x