Skip to main content
Springer Nature Link
Log in

Unconditionally Optimal Error Estimates of a Linearized Galerkin Method for Nonlinear Time Fractional Reaction–Subdiffusion Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

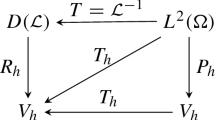
Abstract

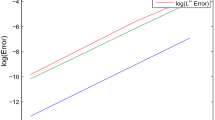
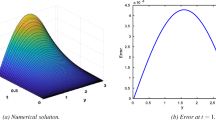
This paper is concerned with unconditionally optimal error estimates of linearized Galerkin finite element methods to numerically solve some multi-dimensional fractional reaction–subdiffusion equations, while the classical analysis for numerical approximation of multi-dimensional nonlinear parabolic problems usually require a restriction on the time-step, which is dependent on the spatial grid size. To obtain the unconditionally optimal error estimates, the key point is to obtain the boundedness of numerical solutions in the \(L^\infty \)-norm. For this, we introduce a time-discrete elliptic equation, construct an energy function for the nonlocal problem, and handle the error summation properly. Compared with integer-order nonlinear problems, the nonlocal convolution in the time fractional derivative causes much difficulties in developing and analyzing numerical schemes. Numerical examples are given to validate our theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Akrivis, G., Crouzeix, M., Makridakis, C.: Implicit–explicit multistep methods for quasilinear parabolic equations. Numer. Math. 82, 521–541 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  2. Amblard, F., Maggs, A.C., Yurke, B., Pargellis, A.N., Leibler, S.: Subdiffusion and anomalous local viscoelasticity in actin networks. Phys. Rev. Lett. 77, 4470 (1996)

    Article  Google Scholar 

  3. Bhrawy, A.H., Zaky, M.A.: Numerical simulation for two-dimensional variable-order fractional nonlinear cable equation. Nonlinear Dyn. 80, 101–116 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bouchaud, J., Georges, A.: Anomalous diffusion in disordered media: statistical mechanisms: models and physical applications. Phys. Rep. 195, 127–293 (1990)

    Article  MathSciNet  Google Scholar 

  5. Cannon, J.R., Lin, Y.: Nonclassical H1 projection and Galerkin methods for nonlinear parabolic integro-differential equations. SIAM. J. Numer. Anal. 25, 187–201 (1988)

    MATH  Google Scholar 

  6. Chen, C.M., Liu, F., Turner, I., Anh, V.: A Fourier method for the fractional diffusion equation describing sub-diffusion. J. Comput. Phys. 227, 886–897 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  7. Ford, N.J., Rodrigues, M.M., Vieira, N.: A numerical method for the fractional Schrödinger type equation of spatial dimension two. Frac. Cacl. Appl. Anal. 16, 1–15 (2013)

    Article  MATH  Google Scholar 

  8. Gao, H.: Optimal error analysis of Galerkin FEMs for nonlinear joule heating equations. J. Sci. Comput 58, 627–647 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  9. Gao, G.H., Sun, Z.Z.: A compact difference scheme for the fractional sub-diffusion equations. J. Comput. Phys. 230, 586–595 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Gu, Y., Zhuang, P., Liu, F.: An advanced implicit meshless approach for the non-linear anomalous subdiffusion equation. Comput. Model. Eng. Sci. 56(3), 303–334 (2010)

    MathSciNet  MATH  Google Scholar 

  11. Henry, B.I., Langlands, T.A.M., Wearne, S.L.: Fractional cable models for spiny neuronal dendrites. Phys. Rev. Lett. 100, 128103 (2008)

    Article  Google Scholar 

  12. Heywood, J.G., Rannacher, R.: Finite element approximation of the nonstationary Navier–Stokes problem IV: error analysis for second-order time discretization. SIAM. J. Numer. Anal. 27, 353–384 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  13. Jiang, S., Zhang, J., Zhang, Q., Zhang, Z.: Fast evaluation of the Caputo fractional derivative and its applications to fractional diffusion equations. Commun. Comput. Phys. 21, 650–678 (2017)

    Article  MathSciNet  Google Scholar 

  14. Jin, B., Lazarov, R., Zhou, Z.: Error estimates for a semidiscrete finite element method for fractional order parabolic equations. SIAM. J. Numer. Anal. 51, 445–466 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  15. Jin, B., Li, B., Zhou, Z.: Numerical analysis of nonlinear subdiffusion equations. arXiv:1705.07398

  16. Jin, B., Li, B., Zhou, Z.: An analysis of the Crank–Nicolson method for subdiffusion. IMA J. Numer. Anal. (2017). https://doi.org/10.1093/imanum/drx019

  17. Ke, R., Ng, M., Sun, H.: A fast direct method for block triangular Toeplitz-like with tridiagonal block systems from time-fractional partial differential equations. J. Comput. Phys. 303, 203–211 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  18. Langlands, T.A.M., Henry, B.I.: The accuracy and stability of an implicit solution method for the fractional diffusion equation. J. Comput. Phys. 205, 719–736 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  19. Li, B.: Mathematical modeling, analysis and computation for some complex and nonlinear flow problems. Ph.D. Thesis, City University of Hong Kong, Hong Kong, July, 2012

  20. Li, B., Sun, W.: Unconditional convergence and optimal error estimates of a Galerkin-mixed FEM for incompressible miscible flow in porous media. SIAM J. Numer. Anal. 51, 1959–1977 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  21. Li, B., Gao, H., Sun, W.: Uncondtional optimal error estimates of a Crank–Nicolson Galerkin method the nonlinear thermistor equations. SIAM J. Numer. Anal. 52, 933–954 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  22. Li, D., Wang, J.: Unconditionally optimal error analysis of Crank–Nicolson Galerkin FEMs for a strongly nonlinear parabolic system. J. Comput. Sci 72, 892–915 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  23. Li, D., Wang, J., Zhang, J.: Unconditionally convergent \(L1\)-Galerkin FEMs for nonlinear time-fractional Schrödinger equations. SIAM J. Sci. Comput. 39, A3067–A3088 (2017)

    Article  MATH  Google Scholar 

  24. Li, D., Zhang, J.: Efficient implementation to numerically solve the nonlinear time fractional parabolic problems on unbounded spatial domain. J. Comput. Phys. 322, 415–428 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  25. Li, D., Zhang, C., Ran, M.: A linear finite difference scheme for generalized time fractional Burgers equation. Appl. Math. Model. 40, 6069–6081 (2016)

    Article  MathSciNet  Google Scholar 

  26. Lin, Y., Li, X., Xu, C.: Finite difference/spectral approximations for the fractional cable equation. Math. Comput. 80, 1369–1396 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  27. Liu, Y., Du, Y., Li, H., He, S., Gao, W.: Finite difference/finite element method for a nonlinear time-fractional fourth-order reaction–diffusion problem. Comput. Math. Appl. 70, 573–591 (2015)

    Article  MathSciNet  Google Scholar 

  28. López-Marcos, J.C., Sanz-serna, J.M.: A definition of stability for nonlinear problems. In: Strehmel, K. (ed.) Numerical Treatment of Differential Equations, Teubner-Texte zur Mathematik, Band 104, Leipzig, pp. 216–226 (1988)

  29. Luskin, M.: A Galerkin method for nonlinear parabolic equations with nonlinear boundary conditions. SIAM J. Numer. Anal. 16, 284–299 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  30. Mustapha, K.: A second-order accurate numerical method for a semilinear integro-differential equation with a weakly singular kernel. IMA J. Numer. Anal. 30, 555–578 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  31. Mustapha, K.: Uniform convergence for a discontinuous Galerkin, time-stepping method applied to a fractional diffusion equation. IMA J. Numer. Anal. 32, 906–925 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  32. Podlubny, I.: Fractional Differential Equations. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  33. Samko, S.G., Kilbas, A.A., Marichev, O.I.: Fractional Integrals and Derivatives—Theory and Applications. Gordon and Breach Science Publishers, New York (1993)

    MATH  Google Scholar 

  34. Seki, K., Wojcik, M., Tachiya, M.: Fractional reaction–diffusion equation. J. Chem. Phys. 119, 2165–2174 (2003)

    Article  Google Scholar 

  35. Thomée, V.: Galerkin Finite Element Methods for Parabolic Problems. Springer, Berlin (1997)

    Book  MATH  Google Scholar 

  36. Wang, J., Si, Z., Sun, W.: A new error analysis of characteristics-mixed FEMs for miscible displacement in porous media. SIAM J. Numer. Anal. 52, 3000–3020 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  37. Wang, J.: A new error analysis of Crank–Nicolson Galerkin FEMs for a generalized nonlinear Schrödinger equation. J. Sci. Comput. 52, 390–407 (2014)

    Article  MATH  Google Scholar 

  38. Yan, Y., Sun, Z., Zhang, J.: Fast evaluation of the caputo fractional derivative and its applications to fractional diffusion equations: a second-order scheme. Commun. Comput. Phys. 22(4), 1028–1048 (2017)

    Article  MathSciNet  Google Scholar 

  39. Yu, B., Jiang, X.: Numerical identification of the fractional derivatives in the two-dimensional fractional cable equation. J. Sci. Comput. 68, 252–272 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  40. Yuste, S.B., Lindenberg, K.: Reaction front in an \(A+B\rightarrow C\) reaction–subdiffusion process. Phys. Rev. E. 69, 036126 (2004)

    Article  Google Scholar 

  41. Yuste, S.B., Lindenberg, K.: Subdiffusion-limited reactions. Chem. Phys. 284, 169–180 (2002)

    Article  Google Scholar 

  42. Zhao, X., Sun, Z., Hao, Z.: A fourth-order compact ADI scheme for two-dimensional nonlinear space fractional Schödinger equation. SIAM. J. Sci. Comput. 36(6), 2865–2886 (2014)

    Article  MathSciNet  Google Scholar 

  43. Zhang, X., He, Y., Wei, L., Tang, B., Wang, S.: A fully discrete local discontinuous Galerkin method for one-dimensional time-fractional Fisher’s equation. Int. J. Comput. Math. 91, 2021–2038 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  44. Zhuang, P., Liu, F., Anh, V., Turner, I.: New solution and analytical techniques of the implicit numerical method for the anomalous subdiffusion equation. SIAM J. Numer. Anal. 46, 1079–1095 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  45. Zhuang, P., Liu, F., Anh, V., Turner, I.: Stability and convergence of an implicit numerical methods for nonlinear fractional reaction–subdiffusion process. IMA J. Appl. Math. 6, 1–23 (2009)

    MATH  Google Scholar 

  46. Zhuang, P., Liu, F., Turner, I., Anh, V.: Galerkin finite element method and error analysis for the fractional cable equaiton. Numer. Algorithm 72, 447–466 (2016)

    Article  MATH  Google Scholar 

  47. Zlámal, M.: Curved elements in the finite element method. I. SIAM J. Numer. Anal. 10, 229–240 (1973)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Huazhong University of Science and Technology, Wuhan, 430074, China

    Dongfang Li

  2. Hubei Key Laboratory of Engineering Modeling and Scientific Computing, Huazhong University of Science and Technology, Wuhan, 430074, China

    Dongfang Li

  3. Beijing Computational Science Research Center, Beijing, 100193, China

    Jiwei Zhang & Zhimin Zhang

  4. Department of Mathematics, Wayne State University, Detroit, MI, 48202, USA

    Zhimin Zhang

Authors
  1. Dongfang Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Jiwei Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Zhimin Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jiwei Zhang.

Additional information

This work is partially supported by NSFC under Grant Nos. 11771035, 11771162, 11571128, 91430216 and U1530401.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, D., Zhang, J. & Zhang, Z. Unconditionally Optimal Error Estimates of a Linearized Galerkin Method for Nonlinear Time Fractional Reaction–Subdiffusion Equations. J Sci Comput 76, 848–866 (2018). https://doi.org/10.1007/s10915-018-0642-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-018-0642-9

Keywords