Skip to main content
Springer Nature Link
Log in

A Posteriori Error Estimates of Virtual Element Method for a Simplified Friction Problem

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

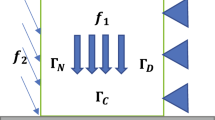
Abstract

We study a posteriori error analysis of the virtual element method (VEM) for a simplified friction problem, which is a representative elliptic variational inequality of the second kind. By treating hanging nodes as vertices of polygonal elements, the virtual element method does not require any local mesh post-processing after the adaptive mesh refinement. In this work, residual type error estimators are derived for designing adaptive VEM to solve the simplified friction problem. Furthermore, the reliability and efficiency of the error estimators are proved. Finally, a numerical example is given to verify the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Duvaut, G., Lions, J.L.: Inequalities in Mechanics and Physics. Springer, Berlin (1976)

    Book  MATH  Google Scholar 

  2. Kikuchi, N., Oden, J.T.: Contact Problems in Elasticity: A Study of Variational Inequalities and Finite Element Methods. SIAM, Philadelphia (1988)

    Book  MATH  Google Scholar 

  3. Han, W., Sofonea, M.: Quasistatic Contact Problems in Viscoelasticity and Viscoplasticity. American Mathematical Society and International Press, New York (2002)

    Book  MATH  Google Scholar 

  4. Brezzi, F., Hager, W.W., Raviart, P.A.: Error estimates for the finite element solution of variational inequalities. Numer. Math. 28, 431–443 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  5. Glowinski, R.: Numerical Methods for Nonlinear Variational Problems. Springer, New York (1984)

    Book  MATH  Google Scholar 

  6. Djoko, J.K., Ebobisse, F., McBride, A.T., Reddy, B.D.: A discontinuous Galerkin formulation for classical and gradient plasticity–part 1: formulation and analysis. Comput. Methods Appl. Mech. Eng. 196, 3881–3897 (2007)

    Article  MATH  Google Scholar 

  7. Wang, F., Han, W., Cheng, X.: Discontinuous Galerkin methods for solving elliptic variational inequalities. SIAM J. Numer. Anal. 48, 708–733 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  8. Wang, F., Han, W., Cheng, X.: Discontinuous Galerkin methods for solving Signorini problem. IMA J. Numer. Anal. 31, 1754–1772 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  9. Wang, F., Han, W., Cheng, X.: Discontinuous Galerkin methods for solving the quasistatic contact problem. Numer. Math. 126, 771–800 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  10. Da Beirão, V.L., Brezzi, F., Marini, L.D.: Virtual elements for linear elasticity problems. SIAM J. Numer. Anal. 51, 794–812 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  11. Antonietti, P.F., Da Beirão, V.L., Mora, D., Verani, M.: A stream function formulation of the Stokes problem for the virtual element method. SIAM J. Numer. Anal. 52, 386–404 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  12. Perugia, I., Pietra, P., Russo, A.: A plane wave virtual element method for the Helmholtz problem. ESAIM M2AN 50, 783–808 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  13. Zhao, J., Chen, S., Zhang, B.: The nonconforming virtual element method for plate bending problems. Math. Models Methods Appl. Sci. 26, 1671–1687 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  14. Chen, L., Wei, H., Wen, M.: An interface-fitted mesh generator and virtual element methods for elliptic interface problems. J. Comput. Phys. 334, 327–348 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  15. Da Beirão, V.L., Lovadina, C., Vacca, G.: Virtual elements for the Navier-Stokes problem on polygonal meshes. SIAM J. Numer. Anal. 56, 1210–1242 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  16. Wriggers, P., Rust, W.T., Reddy, B.D.: A virtual element method for contact. Comput. Mech. 58, 1039–1050 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  17. Wang, F., Wei, H.: Virtual element method for simplified friction problem. Appl. Math. Lett. 85, 125–131 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  18. Wang, F., Wei, H.: Virtual element methods for obstacle problem. IMA J. Numer. Anal. 40, 708–728 (2020)

    Article  MathSciNet  Google Scholar 

  19. Feng, F., Han, W., Huang, J.: Virtual element methods for elliptic variational inequalities of the second kind. J. Sci. Comput. 80, 60–80 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  20. Feng, F., Han, W., Huang, J.: Virtual element method for an elliptic hemivariational inequality with applications to contact mechanics. J. Sci. Comput. 81, 2388–2412 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  21. Wang, F., Zhao, J.: Conforming and nonconforming virtual element methods for a Kirchhoff plate contact problem. IMA J. Numer. Anal. (2019). https://doi.org/10.1093/imanum/draa005

    Article  Google Scholar 

  22. Ling, M., Wang, F., Han, W., Wang, C.: Virtual element method for an elliptic hemivariational inequality, submitted

  23. Verfürth, R.: A Review of a Posteriori Error Estimation and Adaptive Mesh-refinement Techniques. Wiley, London (1996)

    MATH  Google Scholar 

  24. Ainsworth, M., Oden, T.J.: A Posteriori Error Estimation in Finite Element Analysis. Wiley, Chichester (2000)

    Book  MATH  Google Scholar 

  25. Babuška, I., Strouboulis, T.: The Finite Element Method and its Reliability. Oxford University Press, Oxford (2001)

    MATH  Google Scholar 

  26. Cangiani, A., Georgoulis, E.H., Pryer, T., Sutton, O.J.: A posteriori error estimates for the virtual element method. Numer. Math. 137, 857–893 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  27. Mora, D., Rivera, G., Rodrguez, R.: A posteriori error estimates for a virtual element method for the Steklov eigenvalue problem. Comput. Math. Appl. 74, 2172–2190 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  28. Da Beirão, L., Da Veiga, G.M., Mascotto, L.: A posteriori error estimation and adaptivity in hp virtual elements. Numer. Math. 143, 139–175 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  29. Chi, H., Da Beirão, L.V., Paulino, G.H.: A simple and effective gradient recovery scheme and a posteriori error estimator for the virtual element method. Comput. Methods Appl. Mech. Eng. 347, 21–58 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  30. Chen, Z., Nochetto, R.H.: Residual type a posteriori error estimates for elliptic obstacle problems. Numer. Math. 84, 527–548 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  31. Veeser, A.: Efficient and reliable a posteriori error estimators for elliptic obstacle problems. SIAM J. Numer. Anal. 39, 146–167 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  32. Yan, N.: A posteriori error estimators of gradient recovery type for elliptic obstacle problems. Adv. Comput. Math. 15, 333–362 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  33. Nochetto, R.H., Siebert, K.G., Veeser, A.: Pointwise a posteriori error control for elliptic obstacle problem. Numer. Math. 95, 163–195 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  34. Bartels, S., Carstensen, C.: Averaging techniques yield reliable a posteriori finite element error control for obstacle problems. Numer. Math. 99, 225–249 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  35. Braess, D.: A posteriori error estimators for obstacle problems-another look. Numer. Math. 101, 415–421 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  36. Gudi, T., Porwal, K.: A posteriori error control of discontinuous Galerkin methods for elliptic obstacle problems. Math. Comput. 83, 579–602 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  37. Wang, F., Han, W., Eichholz, J., Cheng, X.: A posteriori error estimates for discontinuous Galerkin methods of obstacle problems. Nonlinear Anal. Real World Appl. 22, 664–679 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  38. Bostan, V., Han, W.: Recovery-based error estimation and adaptive solution of elliptic variational inequalities of the second kind. Commun. Math. Sci. 2, 1–18 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  39. Bostan, V., Han, W., Reddy, B.D.: A posteriori error estimation and adaptive solution of elliptic variational inequalities of the second kind. Appl. Numer. Math. 52, 13–38 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  40. Bostan, V., Han, W.: A posteriori error analysis for a contact problem with friction. Comput. Methods Appl. Mech. Eng. 195, 1252–1274 (2006)

    Article  MATH  Google Scholar 

  41. Wang, F., Han, W.: Another view for a posteriori error estimates for variational inequalities of the second kind. Appl. Numer. Math. 72, 225–233 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  42. Wang, F., Han, W.: Reliable and efficient a posteriori error estimates of DG methods for a simplified frictional contact problem. Int. J. Numer. Anal. Model. 16, 49–62 (2019)

    MathSciNet  MATH  Google Scholar 

  43. Atkinson, K., Han, W.: Theoretical Numerical Analysis: A Functional Analysis Framework, 3rd edn. Springer, New York (2009)

    MATH  Google Scholar 

  44. Da Beirão, V.L., Brezzi, F., Cangiani, A., Manzini, G., Marini, L.D., Russo, A.: Basic principles of virtual elements methods. Math. Models Methods Appl. Sci. 23, 199–214 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  45. Da Beirão, V.L., Lovadina, C., Russo, A.: Stability analysis for the virtual element method. Math. Models Methods Appl. Sci. 27, 2557–2594 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  46. Chen, L., Huang, J.: Some error analysis on virtual element methods. Calcolo 55, 5 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  47. Ahmad, B., Alsaedi, A., Brezzi, F., Marini, L.D., Russo, A.: Equivalent projectors for virtual element methods. Comput. Math. Appl. 66, 376–391 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  48. Da Beirão, V.L., Brezzi, F., Marini, L.D., Russo, A.: Virtual element methods for general second order elliptic problems on polygonal meshes. Math. Models Methods Appl. Sci. 26, 729–750 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  49. Cangiani, A., Manzini, G., Sutton, O.J.: Conforming and nonconforming virtual element methods for elliptic problems. IMA J. Numer. Anal. 37, 1317–1354 (2017)

    MathSciNet  MATH  Google Scholar 

  50. WeiBer, S.: Residual based error estimate and quasi-interpolation on polygonal meshes for high order BEM-based FEM. Comput. Math. Appl. 73, 187–202 (2017)

    Article  MathSciNet  Google Scholar 

  51. Han, W.: Finite element analysis of a holonomic elastic-plastic problem. Numer. Math. 60, 493–508 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  52. Wei, H., FEALPy: Finite Element Analysis Library in Python, https://github.com/weihuayi/fealpy, Xiangtan University, 2017–2020

Download references

Acknowledgements

We thank the two anonymous referees for their valuable comments and suggestions, which significantly improve this work.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Xi’an Jiaotong University, Xi’an, 710049, Shaanxi, People’s Republic of China

    Yanling Deng & Fei Wang

  2. Hunan Key Laboratory for Computation and Simulation in Science and Engineering, School of Mathematics and Computational Science, Xiangtan University, Xiangtan, 411105, Hunan, People’s Republic of China

    Huayi Wei

Authors
  1. Yanling Deng
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Fei Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Huayi Wei
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Fei Wang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The work of this author (Fei Wang) was partially supported by the National Natural Science Foundation of China (Grant No. 11771350). The work of this author (Huayi Wei) was partially supported by the National Natural Science Foundation of China (Grant No. 11871413).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Deng, Y., Wang, F. & Wei, H. A Posteriori Error Estimates of Virtual Element Method for a Simplified Friction Problem. J Sci Comput 83, 52 (2020). https://doi.org/10.1007/s10915-020-01242-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-020-01242-9

Keywords