Skip to main content
Springer Nature Link
Log in

Optimal Energy Conserving Local Discontinuous Galerkin Methods for Elastodynamics: Semi and Fully Discrete Error Analysis

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

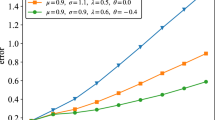
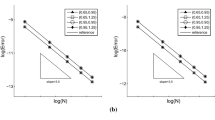
We present an arbitrary high-order local discontinuous Galerkin (LDG) method with alternating fluxes for solving linear elastodynamics problems in isotropic media. Both the semi-discrete analysis and fully discrete analysis for a leap-frog LDG method are given to show that the proposed method simultaneously enjoys the energy conserving property and optimal convergence rates in both the displacement and stress, when the tensor product polynomials of the degree k are used on Cartesian meshes. Numerical experiments demonstrate that the proposed method has several advantages including the exact energy conservation, slow-growing errors in long time simulation, and subtle dependence on the first Lamé parameter \(\lambda \).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Data Availability Statement

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Antonietti, P.F., de Dios, B.A., Mazzieri, I., Quarteroni, A.: Stability analysis of discontinuous galerkin approximations to the elastodynamics problem. J. Sci. Comput. 68(1), 143–170 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  2. Antonietti, P.F., Mazzieri, I.: High-order discontinuous Galerkin methods for the elastodynamics equation on polygonal and polyhedral meshes. Comput. Methods Appl. Mech. Eng. 342, 414–437 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  3. Appelö, D., Hagstrom, T.: An energy-based discontinuous Galerkin discretization of the elastic wave equation in second order form. Comput. Methods Appl. Mech. Eng. 338, 362–391 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  4. Arnold, D.N., Lee, J.J.: Mixed methods for elastodynamics with weak symmetry. SIAM J. Numer. Anal. 52(6), 2743–2769 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bassi, F., Rebay, S.: A high-order accurate discontinuous finite element method for the numerical solution of the compressible Navier-Stokes equations. J. Comput. Phys. 131(2), 267–279 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  6. Bécache, E., Joly, P., Tsogka, C.: A new family of mixed finite elements for the linear elastodynamic problem. SIAM J. Numer. Anal. 39(6), 2109–2132 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bona, J.L., Chen, H., Karakashian, O.A., Xing, Y.: Conservative discontinuous Galerkin methods for the generalized Korteweg-de Vries equation. Math. Comput. 82, 1401–1432 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  8. Castillo, P., Cockburn, B., Perugia, I., Schötzau, D.: An a priori error analysis of the local discontinuous Galerkin method for elliptic problems. SIAM J. Numer. Anal. 38(5), 1676–1706 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  9. Cheng, Y., Shu, C.-W.: Superconvergence of discontinuous Galerkin and local discontinuous Galerkin schemes for linear hyperbolic and convection-diffusion equations in one space dimension. SIAM J. Numer. Anal. 47(6), 4044–4072 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  10. Chou, C.-S., Shu, C.-W., Xing, Y.: Optimal energy conserving local discontinuous Galerkin methods for second-order wave equation in heterogeneous media. J. Comput. Phys. 272, 88–107 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  11. Chung, E.T., Du, J., Lam, C.Y.: Discontinuous Galerkin methods with staggered hybridization for linear elastodynamics. Comput. Math. Appl. 74(6), 1198–1214 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  12. Chung, E.T., Engquist, B.: Optimal discontinuous Galerkin methods for wave propagation. SIAM J. Numer. Anal. 44(5), 2131–2158 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  13. Cockburn, B., Zhixing, F., Hungria, A., Ji, L., Sanchez, M.A., Sayas, F.-J.: Stormer-numerov HDG methods for acoustic waves. J. Sci. Comput. 75, 597–624 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  14. Cockburn, B., Kanschat, G., Perugia, I., Schötzau, D.: Superconvergence of the local discontinuous Galerkin method for elliptic problems on cartesian grids. SIAM J. Numer. Anal. 39(1), 264–285 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  15. Cockburn, B., Shu, C.-W.: TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws ii: General framework. Math. Comput. 52(186), 411–435 (1989)

    MathSciNet  MATH  Google Scholar 

  16. Cockburn, B., Shu, C.-W.: The local discontinuous Galerkin method for time-dependent convection-diffusion systems. SIAM J. Numer. Anal. 35(6), 2440–2463 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  17. De Basabe, J.D., Sen, M.K., Wheeler, M.F.: The interior penalty discontinuous Galerkin method for elastic wave propagation: grid dispersion. Geophys. J. Int. 175(1), 83–93 (2008)

    Article  Google Scholar 

  18. Demkowicz, L., Oden, J.T.: Application of hp-adaptive BE/FE methods to elastic scattering. Comput. Methods Appl. Mech. Eng. 133(3), 287–317 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  19. Di Pietro, D.A., Nicaise, S.: A locking-free discontinuous Galerkin method for linear elasticity in locally nearly incompressible heterogeneous media. Appl. Numer. Math. 63, 105–116 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  20. Dong, B., Shu, C.-W.: Analysis of a local discontinuous Galerkin method for linear time-dependent fourth-order problems. SIAM J. Numer. Anal. 47(5), 3240–3268 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  21. Douglas Jr., J., Gupta, C.P.: Superconvergence for a mixed finite element method for elastic wave propagation in a plane domain. Numer. Math. 49, 189–202 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  22. Du, S., Sayas, F.-J.: New analytical tools for HDG in elasticity, with applications to elastodynamics. Math. Comput. 89, 1745–1782 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  23. Etienne, V., Chaljub, E., Virieux, J., Glinsky, N.: An hp-adaptive discontinuous Galerkin finite-element method for 3-D elastic wave modelling. Geophys. J. Int. 183(2), 941–962 (2010)

    Article  Google Scholar 

  24. Falk, R.S., Richter, G.R.: Explicit finite element methods for symmetric hyperbolic equations. SIAM J. Numer. Anal. 36(3), 935–952 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  25. Fernandez, P., Christophe, A., Terrana, S., Nguyen, N.C., Peraire, J.: Hybridized discontinuous Galerkin methods for wave propagation. J. Sci. Comput. 77(3), 1566–1604 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  26. García, C., Gatica, G.N., Meddahi, S.: A new mixed finite element method for elastodynamics with weak symmetry. J. Sci. Comput. 72(3), 1049–1079 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  27. Grote, M.J., Schötzau, D.: Optimal error estimates for the fully discrete interior penalty dg method for the wave equation. J. Sci. Comput. 40(1), 257–272 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  28. Guo, K., Acosta, S., Chan, J.: A weight-adjusted discontinuous Galerkin method for wave propagation in coupled elastic–acoustic media. J. Comput. Phys. 418, 109632 (2020)

    Article  MathSciNet  Google Scholar 

  29. Guo, R., Lin, T., Lin, Y.: Recovering elastic inclusions by shape optimization methods with immersed finite elements. J. Comput. Phys. 404, 109123 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  30. Han, W., He, L., Wang, F.: Optimal order error estimates for discontinuous Galerkin methods for the wave equation. J. Sci. Comput. 78(1), 121–144 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  31. Hesthaven, J.S., Warburton, T.: Nodal discontinuous Galerkin methods, volume 54 of Texts in Applied Mathematics. Springer, New York. Algorithms, analysis, and applications (2008)

  32. Huang, Y., Liu, H., Yi, N.: A conservative discontinuous Galerkin method for the Degasperis-Procesi equation. Methods Appl. Anal. 21, 67–90 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  33. Hufford, C., Xing, Y.: Superconvergence of the local discontinuous Galerkin method for the linearized korteweg-de vries equation. J. Comput. Appl. Math. 255, 441–455 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  34. Hughes, T.J.R., Hulbert, G.M.: Space-time finite element methods for elastodynamics: formulations and error estimates. Comput. Methods Appl. Mech. Eng. 66(3), 339–363 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  35. Joly, P.: Variational methods for time-dependent wave propagation problems. Springer, Berlin, Heidelberg (2003)

    Book  MATH  Google Scholar 

  36. Komatitsch, D., Vilotte, J.-P., Vai, R., Castillo-Covarrubias, J.M., Sánchez-Sesma, F.J.: The spectral element method for elastic wave equations–application to 2-d and 3-d seismic problem. Int. J. Numer. Methods Eng. 45(9), 1139–1164 (1999)

    Article  MATH  Google Scholar 

  37. Li, X., Sun, W., Xing, Y., Chou, C.-S.: Energy conserving local discontinuous Galerkin methods for the improved Boussinesq equation. J. Comput. Phys. 401, 109002 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  38. Liang, X., Khaliq, A.Q.M., Xing, Y.: Fourth order exponential time differencing method with local discontinuous Galerkin approximation for coupled nonlinear Schrodinger equations. Commun. Comput. Phys. 17, 510–541 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  39. Liu, H., Xing, Y.: An invariant preserving discontinuous Galerkin method for the Camassa–Holm equation. SIAM J. Sci. Comput. 38, A1919–A1934 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  40. Matuszyk, P.J., Demkowicz, L.F., Torres-Verdin, C.: Solution of coupled acoustic-elastic wave propagation problems with anelastic attenuation using automatic hp-adaptivity. Comput. Methods Appl. Mech. Eng. 213–216, 299–313 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  41. Meng, X., Shu, C.-W., Boying, W.: Optimal error estimates for discontinuous Galerkin methods based on upwind-biased fluxes for linear hyperbolic equations. Math. Comput. 85(299), 1225–1261 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  42. Nguyen, N.C., Peraire, J., Cockburn, B.: High-order implicit hybridizable discontinuous Galerkin methods for acoustics and elastodynamics. J. Comput. Phys. 230(10), 3695–3718 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  43. Rivière, B., Shaw, S., Wheeler, M.F., Whiteman, J.R.: Discontinuous Galerkin finite element methods for linear elasticity and quasistatic linear viscoelasticity. Numer. Math. 95(2), 347–376 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  44. Schuster, G.T.: Seismic inversion. Society of Exploration Geophysicists, Houston (2017)

    Book  Google Scholar 

  45. Shubin, G.R., Bell, J.B.: A modified equation approach to constructing fourth order methods for acoustic wave propagation. SIAM J. Sci. Stat. Comput. 8(2), 135–151 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  46. Sjögreen, B., Petersson, N.A.: A fourth order accurate finite difference scheme for the elastic wave equation in second order formulation. J. Sci. Comput. 52(1), 17–48 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  47. Sticko, S., Kreiss, G.: Higher order cut finite elements for the wave equation. J. Sci. Comput. 80(3), 1867–1887 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  48. Sun, Z., Xing, Y.: Optimal error estimates of discontinuous Galerkin methods with generalized fluxes for wave equations on unstructured meshes. Math. Comput. (in press) https://doi.org/10.1090/mcom/3605

  49. Terrana, S., Vilotte, J.P., Guillot, L.: A spectral hybridizable discontinuous Galerkin method for elastic-acoustic wave propagation. Geophys. J. Int. 213(1), 574–602 (2017)

    Article  Google Scholar 

  50. Virieux, J.: P-sv wave propagation in heterogeneous media: velocity-stress finite-difference method. Geophysics 51(4), 889–901 (1986)

    Article  Google Scholar 

  51. Wang, H., Wang, S., Zhang, Q., Shu, C.-W.: Local discontinuous Galerkin methods with implicit–explicit time-marching for multi-dimensional convection-diffusion problems. ESAIM Math. Model. Numer. Anal. 50(4), 1083–1105 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  52. Wang, H., Zhang, Q.: Error estimate on a fully discrete local discontinuous Galerkin method for linear convection–diffusion problem. J. Comput. Math. 31(3), 283–307 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  53. Warburton, T., Hesthaven, J.S.: On the constants in \(hp\)-finite element trace inverse inequalities. Comput. Methods Appl. Mech. Eng. 192(25), 2765–2773 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  54. Wihler, T.P.: Locking-free adaptive discontinuous Galerkin FEM for linear elasticity problems. Math. Comput. 75(255), 1087–1102 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  55. Wilcox, L.C., Stadler, G., Burstedde, C., Ghattas, O.: A high-order discontinuous Galerkin method for wave propagation through coupled elastic–acoustic media. J. Comput. Phys. 229(24), 9373–9396 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  56. Xing, Y., Chou, C.-S., Shu, C.-W.: Energy conserving local discontinuous Galerkin methods for wave propagation problems. Inverse Problems Imag. 7, 967 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  57. Yan, X., Shu, C.-W.: Local discontinuous Galerkin methods for high-order time-dependent partial differential equations. Commun. Comput. Phys. 7, 1–46 (2010)

    MathSciNet  MATH  Google Scholar 

  58. Zhang, Q., Shu, C.-W.: Stability analysis and a priori error estimates of the third order explicit Runge–Kutta discontinuous Galerkin method for scalar conservation laws. SIAM J. Numer. Anal. 48(3), 1038–1063 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of California Irvine, Irvine, CA, 92697, USA

    Ruchi Guo

  2. Department of Mathematics, The Ohio State University, Columbus, OH, 43210, USA

    Yulong Xing

Authors
  1. Ruchi Guo
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yulong Xing
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yulong Xing.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Y. Xing: The work of this author is partially supported by the NSF grant DMS-1753581.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Guo, R., Xing, Y. Optimal Energy Conserving Local Discontinuous Galerkin Methods for Elastodynamics: Semi and Fully Discrete Error Analysis. J Sci Comput 87, 13 (2021). https://doi.org/10.1007/s10915-021-01418-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01418-x

Keywords

Mathematics Subject Classification