Skip to main content
Springer Nature Link
Log in

Efficient Spectral Methods for PDEs with Spectral Fractional Laplacian

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

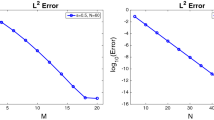
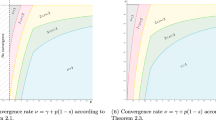
We develop efficient spectral methods for the spectral fractional Laplacian equation and parabolic PDEs with spectral fractional Laplacian on rectangular domains. The key idea is to construct eigenfunctions of discrete Laplacian (also referred to Fourier-like basis) by using the Fourierization method. Under this basis, the non-local fractional Laplacian operator can be trivially evaluated, leading to very efficient algorithms for PDEs involving spectral fractional Laplacian. We provide a rigorous error analysis of the proposed methods for the case with homogeneous boundary conditions, as well as ample numerical results to show their effectiveness.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Ainsworth, M., Glusa, C.: Hybrid finite element-spectral method for the fractional Laplacian: approximation theory and efficient solver. SIAM J. Sci. Comput. 40, A2383–A2405 (2018)

    Article  MathSciNet  Google Scholar 

  2. Ainsworth, M., Mao, Z.: Analysis and approximation of a fractional Cahn-Hilliard equation. SIAM J. Numer. Anal. 55, 1689–1718 (2017)

    Article  MathSciNet  Google Scholar 

  3. Banjai, L., Melenk, J.M., Nochetto, R.H., Otarola, E., Salgado, A.J., Schwab, C.: Tensor FEM for spectral fractional diffusion. Found. Comput. Math. 19, 901–962 (2019)

    Article  MathSciNet  Google Scholar 

  4. Bonito, A., Borthagaray, J.P., Nochetto, R.H., Otárola, E., Salgado, A.J.: Numerical methods for fractional diffusion. Comput. Vis. Sci. 19, 1–28 (2018)

    Article  MathSciNet  Google Scholar 

  5. Bonito, A., Pasciak, J.: Numerical approximation of fractional powers of elliptic operators. Math. Comput. 84, 2083–2110 (2015)

    Article  MathSciNet  Google Scholar 

  6. Brezis, H.: Analyse Fonctionnelle. Théorie et Applications. (French)[Functional Analysis. Theory and Applications], Collection Mathématiques Appliquées pour la Maıtrise., Masson, Paris, (1983)

  7. Bueno-Orovio, A., Kay, D., Burrage, K.: Fourier spectral methods for fractional-in-space reaction-diffusion equations. BIT 54, 937–954 (2014)

    Article  MathSciNet  Google Scholar 

  8. Burrage, K., Hale, N., Kay, D.: An efficient implicit FEM scheme for fractional-in-space reaction-diffusion equations. SIAM J. Sci. Comput. 34, A2145–A2172 (2012)

    Article  MathSciNet  Google Scholar 

  9. Caffarelli, L., Silvestre, L.: An extension problem related to the fractional Laplacian. Commun. Partial Differ. Equ. 32, 1245–1260 (2007)

    Article  MathSciNet  Google Scholar 

  10. Chen, L.Q., Shen, J.: Applications of semi-implicit Fourier-spectral method to phase field equations. Comput. Phys. Commun. 108, 147–158 (1998)

    Article  Google Scholar 

  11. Chen, S., Shen, J.: An efficient and accurate numerical method for the spectral fractional Laplacian equation. J. Sci. Comput. 82, 1–25 (2020)

    Article  MathSciNet  Google Scholar 

  12. Fujita, H., Suzuki, T.: Evolution problems. In: Handbook of Numerical Analysis, vol. 2, pp. 789–928. II, North-Holland, Amsterdam (1991)

  13. Gu, Y.: Spectral methods for boundary value problems in complex domains, Ph.d thesis, Purdue University (2019)

  14. Guo, B.: Spectral Methods and Their Applications. World Scientific, Singapore (1998)

    Book  Google Scholar 

  15. Guo, B., Shen, J., Wang, L.: Optimal spectral-Galerkin methods using generalized Jacobi polynomials. J. Sci. Comput. 27, 305–322 (2006)

    Article  MathSciNet  Google Scholar 

  16. Harizanov, S., Lazarov, R., Margenov, S., Marinov, P., Pasciak, J.: Analysis of numerical methods for spectral fractional elliptic equations based on the best uniform rational approximation. J. Comput. Phys. 408, 10928521 (2020)

    Article  MathSciNet  Google Scholar 

  17. Ilic, M., Liu, F., Turner, I., Anh, V.: Numerical approximation of a fractional-in-space diffusion equation. I. Fract. Calc. Appl. Anal. 8, 323–341 (2005)

    MathSciNet  MATH  Google Scholar 

  18. Kato, T.: Fractional powers of dissipative operators. J. Math. Soc. Jpn 13, 246–274 (1961)

    Article  MathSciNet  Google Scholar 

  19. Knoll, D.A., Keyes, D.E.: Jacobian-free Newton-Krylov methods: a survey of approaches and applications. J. Comput. Phys. 193, 357–397 (2004)

    Article  MathSciNet  Google Scholar 

  20. Lions, J.L., Magenes, E.: Non-homogeneous boundary value problems and applications, vol. 1. Springer, Berlin (2012)

    MATH  Google Scholar 

  21. Lischke, A., Pang, G., Gulian, M., Song, F., Glusa, C., Zheng, X., Mao, Z., Cai, W., Meerschaert, M.M., Ainsworth, M., et al.: What is the fractional Laplacian? A comparative review with new results. J. Comput. Phys. 404, (2020)

    Article  MathSciNet  Google Scholar 

  22. Nochetto, R.H., Otárola, E., Salgado, A.J.: A PDE approach to fractional diffusion in general domains: a priori error analysis. Found. Comput. Math. 15, 733–791 (2015)

    Article  MathSciNet  Google Scholar 

  23. Nochetto, R.H., Otarola, E., Salgado, A.J.: A PDE approach to space-time fractional parabolic problems. SIAM J. Numer. Anal. 54, 848–873 (2016)

    Article  MathSciNet  Google Scholar 

  24. Shen, J.: Efficient spectral-Galerkin method I. Direct solvers of second-and fourth-order equations using legendre polynomials. SIAM J. Sci. Comput. 15, 1489–1505 (1994)

  25. Shen, J.: Efficient Chebyshev-Legendre Galerkin methods for elliptic problems. Proceedings of ICOSAHOM 95, 233–240 (1996)

    Google Scholar 

  26. Shen, J.: A new dual-Petrov-Galerkin method for third and higher odd-order differential equations: application to the KDV equation. SIAM J. Numer. Anal. 41, 1595–1619 (2003)

    Article  MathSciNet  Google Scholar 

  27. Shen, J., Tang, T., Wang, L.: Spectral methods: algorithms, analysis and applications, vol. 41. Springer, Berlin (2011)

    Book  Google Scholar 

  28. Shen, J., Wang, L.: Fourierization of the Legendre-Galerkin method and a new space-time spectral method. Appl. Numer. Math. 57, 710–720 (2007)

    Article  MathSciNet  Google Scholar 

  29. Song, F., Xu, C., Karniadakis, G.E.: A fractional phase-field model for two-phase flows with tunable sharpness: algorithms and simulations. Comput. Methods Appl. Mech. Eng. 305, 376–404 (2016)

    Article  MathSciNet  Google Scholar 

  30. Song, F., Xu, C., Karniadakis, G.E.: Computing fractional Laplacians on complex-geometry domains: algorithms and simulations. SIAM J. Sci. Comput. 39, A1320–A1344 (2017)

    Article  MathSciNet  Google Scholar 

  31. Stinga, P., Torrea, J.: Extension problem and Harnack’s inequality for some fractional operators. Commun. Partial Differ. Equ. 35, 2092–2122 (2010)

    Article  MathSciNet  Google Scholar 

  32. Strang, G.: Variational crimes in the finite element method. The mathematical foundations of the finite element method with applications to partial differential equations, pp. 689–710 (1972)

  33. Weinan, E.: Convergence of spectral methods for Burgers equation. SIAM J. Numer. Anal. 29, 1520–1541 (1992)

    Article  MathSciNet  Google Scholar 

  34. Zhai, S., Gui, D., Zhao, J., Feng, X.: High accuracy spectral method for the space-fractional diffusion equations. J. Math. Study 47, 274–286 (2014)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics, Shanghai University of Finance and Economics, Shanghai, 200433, China

    Changtao Sheng

  2. Department of Mathematics, Purdue University, West Lafayette, IN, 47907-1957, USA

    Duo Cao & Jie Shen

Authors
  1. Changtao Sheng
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Duo Cao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Jie Shen
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jie Shen.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The work of J. Shen is partially supported by NSF DMS-2012585 and AFOSR FA9550-20-1-0309.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sheng, C., Cao, D. & Shen, J. Efficient Spectral Methods for PDEs with Spectral Fractional Laplacian. J Sci Comput 88, 4 (2021). https://doi.org/10.1007/s10915-021-01491-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01491-2

Keywords

Mathematics Subject Classification