Skip to main content
Springer Nature Link
Log in

An Optimal Finite Difference Scheme with Minimized Dispersion and Adaptive Dissipation Considering the Spectral Properties of the Fully Discrete Scheme

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

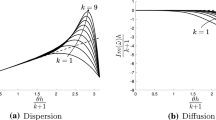
Abstract

For the simulation of flow fields with a broad range of length scales, designing numerical schemes with good spectral properties is one of the most important issues. To improve the spectral properties of the semi-discrete finite different schemes, the authors have previously proposed the idea of optimizing the dispersion and dissipation properties separately and a class of finite difference scheme with minimized dispersion and controllable dissipation properties is thus developed (Sun et al. J Comput Phys 230:4616–4635, 2011, 270:238–254, 2014). In the present paper, we further investigated this idea and extend it to the fully discrete scheme. In other words, the dispersion and dissipation errors induced by the temporal discretization are taken into consideration in the present paper. Moreover, a scale sensor is designed in the control of dissipation error to ensure the numerical scheme can automatically adjust its dissipation according to the local characteristic of the flow field. To achieve the shock-capturing capability, this optimized scheme is blended with the WENO-Z scheme to form a hybrid scheme. A number of benchmark test cases including the transportation of a linear wave, the propagation of a sound wave packet, the Shu–Osher problem, the double Mach reflection problem and the Rayleigh–Taylor instability problem are employed to verify the good spectral properties and robust shock-capturing capability of the proposed scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

References

  1. Lele, S.: Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42 (1992)

    Article  MathSciNet  Google Scholar 

  2. Tam, C., Webb, J.: Dispersion-relation-preserving finite difference schemes for computational acoustics. J. Comput. Phys. 107, 262–281 (1993)

    Article  MathSciNet  Google Scholar 

  3. Harten, A., Engquist, B., Osher, S., et al.: Uniformly high order essentially non-oscillatory schemes III. J. Comput. Phys. 71, 231–303 (1987)

    Article  MathSciNet  Google Scholar 

  4. Jiang, G.S., Shu, C.W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126, 202–228 (1996)

    Article  MathSciNet  Google Scholar 

  5. Martín, M., Taylor, E., Wu, M., et al.: A bandwidth-optimized WENO scheme for effective direct numerical simulation of compressible turbulence. J. Comput. Phys. 220, 270–289 (2006)

    Article  Google Scholar 

  6. Adams, N., Shariff, K.: A high-resolution hybrid compact-eno scheme for shock/turbulence interaction problems. J. Comput. Phys. 127, 27–51 (1996)

    Article  MathSciNet  Google Scholar 

  7. Pirozzoli, S.: Conservative hybrid compact-weno schemes for shock-turbulence interaction. J. Comput. Phys. 178, 81–117 (2002)

    Article  MathSciNet  Google Scholar 

  8. Ren, Y.X., Liu, M., Zhang, H.X.: A characteristic-wise compact weno scheme for solving hyperbolic conservation laws. J. Comput. Phys. 192, 365–386 (2003)

    Article  MathSciNet  Google Scholar 

  9. Shi, Y.F., Gao, Y.: A fifth order alternative compact-weno finite difference scheme for compressible Euler equations. J. Comput. Phys. 397, 108873 (2019)

    Article  MathSciNet  Google Scholar 

  10. Kim, D., Kwon, J.: A high-order accurate hybrid scheme using a central flux scheme and a weno scheme for compressible flow field analysis. J. Comput. Phys. 210, 554–583 (2005)

    Article  MathSciNet  Google Scholar 

  11. Costa, B., Don, W.: Multi-domain hybrid spectral-weno methods for hyperbolic conservation laws. J. Comput. Phys. 224, 970–991 (2007)

    Article  MathSciNet  Google Scholar 

  12. Hu, X.Y., Wang, Q., Adams, N.: An adaptive central-upwind weighted essentially non-oscillatory scheme. J. Comput. Phys. 229, 8952–8965 (2010)

    Article  MathSciNet  Google Scholar 

  13. Zhao, G.Y., Sun, M.B., Mei, Y., et al.: An efficient adaptive central-upwind weno-cu6 numerical scheme with a new sensor. J. Sci. Comput. 81, 649–670 (2019)

    Article  MathSciNet  Google Scholar 

  14. Vevek, U., Zhang, B., New, T.: An efficient hybrid method for solving Euler equations. J. Sci. Comput. 81, 732–762 (2019)

    Article  MathSciNet  Google Scholar 

  15. Yu, C.H., Wang, D., He, Z., et al.: An optimized dispersion-relation-preserving combined compact difference scheme to solve advection equations. J. Comput. Phys. 300, 92–115 (2015)

    Article  MathSciNet  Google Scholar 

  16. Henrick, A., Aslam, T., Powers, J.: Mapped weighted essentially non-oscillatory schemes: achieving optimal order near critical points. J. Comput. Phys. 207, 542–567 (2005)

    Article  Google Scholar 

  17. Taylor, E., Wu, M., Martín, M.: Optimization of nonlinear error for weighted essentially non-oscillatory methods in direct numerical simulations of compressible turbulence. J. Comput. Phys. 223, 384–397 (2007)

    Article  Google Scholar 

  18. Borges, R., Carmona, M., Costa, B., et al.: An improved weighted essentially non-oscillatory scheme for hyperbolic conservation laws. J. Comput. Phys. 227, 3191–3211 (2008)

    Article  MathSciNet  Google Scholar 

  19. Castro, M., Costa, B., Don, W.: High order weighted essentially non-oscillatory weno-z schemes for hyperbolic conservation laws. J. Comput. Phys. 230, 1766–1792 (2011)

    Article  MathSciNet  Google Scholar 

  20. Wang, B.S., Li, P., Gao, Z., et al.: An improved fifth order alternative weno-z finite difference scheme for hyperbolic conservation laws. J. Comput. Phys. 374, 469–477 (2018)

    Article  MathSciNet  Google Scholar 

  21. Xu, W.Z., Wu, W.G.: An improved third-order weno-z scheme. J. Sci. Comput. 75, 1808–1841 (2018)

    Article  MathSciNet  Google Scholar 

  22. Wang, Y.H., Wang, B.S., Don, W.: Generalized sensitivity parameter free fifth order weno finite difference scheme with z-type weights. J. Sci. Comput. 81, 1329–1358 (2019)

    Article  MathSciNet  Google Scholar 

  23. Arshed, G., Hoffmann, K.: Minimizing errors from linear and nonlinear weights of weno schemes for broadband applications with shock waves. J. Sci. Comput. 246, 58–77 (2013)

    MathSciNet  MATH  Google Scholar 

  24. Weirs, V., Candler, G.: Optimization of weighted eno schemes for dns of compressible turbulence. AIAA Paper 97–1940 (1997)

  25. Sun, Z.S., Ren, Y.X., Larricq, C., et al.: A class of finite difference scheme with low dispersion and controllable dissipation for dns of compressible turbulence. J. Comput. Phys. 230, 4616–4635 (2011)

    Article  MathSciNet  Google Scholar 

  26. Sun, Z.S., Luo, L., Ren, Y.X., et al.: A sixth order hybrid finite difference scheme based on the minimized dispersion and controllable dissipation technique. J. Comput. Phys. 270, 238–254 (2014)

    Article  MathSciNet  Google Scholar 

  27. Hu, X.G., Zhang, H.X.: Developing high-order weighted compact nonlinear schemes. J. Comput. Phys. 165, 22–44 (2000)

    Article  MathSciNet  Google Scholar 

  28. Hu, F.Q., Hussaini, M., Manthey, J.: Low-dissipation and low-dispersion Runge–Kutta schemes for computational acoustics. J. Comput. Phys. 124, 177–191 (1996)

    Article  MathSciNet  Google Scholar 

  29. Bogey, C., Bailly, C.: A family of low dispersive and low dissipative explicit schemes for flow and noise computations. J. Comput. Phys. 194, 194–214 (2004)

    Article  Google Scholar 

  30. Bernardini, M., Pirozzoli, S.: A general strategy for the optimization of Runge–Kutta schemes for wave propagation phenomena. J. Comput. Phys. 228, 4182–4199 (2009)

    Article  Google Scholar 

  31. Ramboer, J., Broeckhoven, T., Smirnov, S., et al.: Optimization of time integration schemes coupled to spatial discretization for use in CAA applications. J. Comput. Phys. 213, 777–802 (2006)

    Article  Google Scholar 

  32. Rajpoot, M., Sengupta, T., Dutt, P.: Optimal time advancing dispersion relation preserving schemes. J. Comput. Phys. 229, 3625–3651 (2010)

    Article  MathSciNet  Google Scholar 

  33. Sengupta, T., Rajpoot, M., Bhumkar, Y.: Space-time discretizing optimal drp schemes for flow and wave propagation problems. Computers & fluids 47, 144–154 (2011)

    Article  MathSciNet  Google Scholar 

  34. Hu, X.Y., Adams, N.: Dispersion-dissipation condition for finite difference schemes arXiv:1204.5088v1

  35. Li, Y.H., Chen, C.W., Ren, Y.X.: A class of high-order finite difference schemes with minimized dispersion and adaptive dissipation for solving compressible flow. J. Comput. Phys. (under review)

Download references

Acknowledgements

This work is supported by the project 91952110 of NSFC, the project 2019-JCJQ-JJ-103 and the project 201801U8001.

Author information

Authors and Affiliations

  1. Rocket Force University of Engineering, Xi’an, 710025, Shaanxi, China

    Zhensheng Sun, Yu Hu & Kai Mao

  2. Department of Engineering Mechanics, Tsinghua University, Beijing, 100084, China

    Yuxin Ren

Authors
  1. Zhensheng Sun
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yu Hu
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Yuxin Ren
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Kai Mao
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Zhensheng Sun.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sun, Z., Hu, Y., Ren, Y. et al. An Optimal Finite Difference Scheme with Minimized Dispersion and Adaptive Dissipation Considering the Spectral Properties of the Fully Discrete Scheme. J Sci Comput 89, 32 (2021). https://doi.org/10.1007/s10915-021-01637-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01637-2

Keywords