Skip to main content
Springer Nature Link
Log in

A Parallel Robin–Robin Domain Decomposition Method based on Modified Characteristic FEMs for the Time-Dependent Dual-porosity-Navier–Stokes Model with the Beavers–Joseph Interface Condition

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

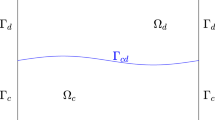
In this paper, we propose and analyze the parallel Robin–Robin domain decomposition method based on the modified characteristic finite element method for the time-dependent dual-porosity-Navier–Stokes model with the Beavers–Joseph interface condition. For the coupling terms, we treat them in an explicit manner which takes advantage of information obtained in previous time steps to construct a non-iteration domain decomposition method. By this means, two single dual-porosity equations and a single Navier–Stokes equation are needed to solve at each time. In particular, we solve the Navier–Stokes equation by the modified characteristic finite element method, which avoids the computational inefficiency caused by the nonlinear convection term. Furthermore, we prove the error convergence of solutions by mathematical induction, whose proof implies the uniform \(L^{\infty }\)-boundedness of the fully discrete velocity solution in conduit flow. Finally, some numerical examples are presented to show the effectiveness and efficiency of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Discacciati, M.: Domain decomposition methods for the coupling of surface and groundwater flows. Ph.D. Thesis, Ecole Polytechnique Federale de Sausanne, Sausanne, Switzerland (2004)

  2. Cesmelioǧlu, A., Riviere, B.: Primal discontinuous Galerkin methods for time-dependent coupled surface and subsurface flow. J. Sci. Comput. 40(1), 115–140 (2009)

    MathSciNet  MATH  Google Scholar 

  3. Layton, W., Tran, H., Trenchea, C.: Analysis of long time stability and errors of two partitioned methods for uncoupling evolutionary groundwater-surface water flows. SIAM J. Numer. Anal. 51(1), 248–272 (2013)

    MathSciNet  MATH  Google Scholar 

  4. Nassehi, V.: Modelling of combined Navier–Stokes and Darcy flows in crossflow membrane filtration. Chem. Eng. Sci. 53(6), 1253–1265 (1998)

    Google Scholar 

  5. Hanspal, N., Waghode, A., Nassehi, V., Wakeman, R.: Numerical analysis of coupled Stokes/Darcy flows in industrial filtrations. Transp. Porous Media 64(1), 73–101 (2006)

    MATH  Google Scholar 

  6. Arbogast, T., Brunson, D.: A computational method for approximating a Darcy–Stokes system governing a vuggy porous medium. Comput. Geosci. 11(3), 207–218 (2007)

    MathSciNet  MATH  Google Scholar 

  7. Chen, J., Sun, S., Wang, X.: A numerical method for a model of two-phase flow in a coupled free flow and porous media system. J. Comput. Phys. 268, 1–16 (2014)

    MathSciNet  MATH  Google Scholar 

  8. D’Angelo, C., Zunino, P.: Robust numerical approximation of coupled Stokes’ and Darcy’s flows applied to vascular hemodynamics and biochemical transport. ESAIM Math. Modell. Numer. Anal. 45(3), 447–476 (2011)

  9. Chen, N., Gunzburger, M., Wang, X.: Asymptotic analysis of the differences between the Stokes–Darcy system with different interface conditions and the Stokes–Brinkman system. J. Math. Anal. Appl. 368(2), 658–676 (2010)

    MathSciNet  MATH  Google Scholar 

  10. Mu, M., Xu, J.: A two-grid method of a mixed Stokes–Darcy model for coupling fluid flow with porous media flow. SIAM J. Numer. Anal. 45(5), 1801–1813 (2007)

    MathSciNet  MATH  Google Scholar 

  11. Gatica, N., Meddahi, S., Oyarzúa, R.: A conforming mixed finite-element method for the coupling of fluid flow with porous media flow. IMA J. Numer. Anal. 29(1), 86–108 (2008)

    MathSciNet  MATH  Google Scholar 

  12. Badea, L., Discacciati, M., Quarteroni, A.: Numerical analysis of the Navier–Stokes/Darcy coupling. Numer. Math. 115(2), 195–227 (2010)

    MathSciNet  MATH  Google Scholar 

  13. Rui, H., Zhang, R.: A unified stabilized mixed finite element method for coupling Stokes and Darcy flows. Comput. Methods Appl. Mech. Eng. 198(33–36), 2692–2699 (2009)

    MathSciNet  MATH  Google Scholar 

  14. Shan, L., Zheng, H.: Partitioned time stepping method for fully evolutionary Stokes–Darcy flow with Beavers–Joseph interface conditions. SIAM J. Numer. Anal. 51(2), 813–839 (2013)

    MathSciNet  MATH  Google Scholar 

  15. Cao, Y., Chu, Y., He, X., Wei, M.: Decoupling the stationary Navier–Stokes–Darcy system with the Beavers–Joseph–Saffman interface condition. Abstract Appl. Anal. 2013, 1–10 (2013)

    MathSciNet  MATH  Google Scholar 

  16. Mu, M., Zhu, X.: Decoupled schemes for a non-stationary mixed Stokes–Darcy model. Math. Comput. 79(270), 707–731 (2010)

    MathSciNet  MATH  Google Scholar 

  17. Chen, W., Gunzburger, M., Dong, S., Wang, X.: Efficient and long-time accurate second-order methods for Stokes–Darcy system. SIAM J. Numer. Anal. 51(5), 2563–2584 (2013)

    MathSciNet  MATH  Google Scholar 

  18. Girault, V., Rivière, B.: DG approximation of coupled Navier–Stokes and Darcy equations by Beaver–Joseph–Saffman interface condition. SIAM J. Numer. Anal. 47(3), 2052–2089 (2009)

    MathSciNet  MATH  Google Scholar 

  19. Cao, Y., Gunzburger, M., He, X., Wang, X.: Robin–Robin domain decomposition methods for the steady-state Stokes–Darcy system with the Beavers–Joseph interface condition. Numer. Math. 117(4), 601–629 (2011)

    MathSciNet  MATH  Google Scholar 

  20. Cai, M., Mu, M., Xu, J.: Numerical solution to a mixed Navier–Stokes/Darcy model by the two-grid approach. SIAM J. Numer. Anal. 47(5), 3325–3338 (2009)

    MathSciNet  MATH  Google Scholar 

  21. He, X., Jiang, N., Qiu, C.: An artificial compressibility ensemble algorithm for a stochastic Stokes–Darcy model with random hydraulic conductivity and interface conditions. Int. J. Numer. Methods Eng. 121(4), 712–739 (2020)

    MathSciNet  Google Scholar 

  22. Cao, L., He, Y., Li, J., Yang, D.: Decoupled modified characteristic FEMs for fully evolutionary Navier–Stokes–Darcy model with the Beavers–Joseph interface condition. J. Comput. Appl. Math. (2021). https://doi.org/10.1016/j.cam.2020.113128

    Article  MathSciNet  MATH  Google Scholar 

  23. Sun, Y., Sun, W., Zheng, H.: Domain decomposition method for the fully-mixed Stokes–Darcy coupled problem. Comput. Methods Appl. Mech. Eng. (2021). https://doi.org/10.1016/j.cam.2020.113578

    Article  MathSciNet  MATH  Google Scholar 

  24. Discacciati, M., Quarteroni, A., Valli, A.: Robin–Robin domain decomposition methods for the Stokes–Darcy coupling. SIAM J. Numer. Anal. 45(3), 1246–1268 (2007)

    MathSciNet  MATH  Google Scholar 

  25. Chen, W., Gunzburger, M., Hua, F., Wang, X.: A parallel Robin–Robin domain decomposition method for the Stokes–Darcy system. SIAM J. Numer. Anal. 49(3), 1064–1084 (2011)

    MathSciNet  MATH  Google Scholar 

  26. Cao, Y., Gunzburger, M., He, X., Wang, X.: Parallel, non-iterative, multi-physics domain decomposition methods for time-dependent Stokes–Darcy systems. Math. Comput. 83(288), 1617–1644 (2014)

    MathSciNet  MATH  Google Scholar 

  27. Qiu, C., He, X., Li, J., Lin, Y.: A domain decomposition method for the time-dependent Navier–Stokes–Darcy model with Beavers–Joseph interface condition and defective boundary condition. J. Comput. Phys. (2020). https://doi.org/10.1016/j.jcp.2020.109400

    Article  MathSciNet  MATH  Google Scholar 

  28. Warren, J., Root, P.: The behavior of naturally fractured reservoirs. Soc. Pet. Eng. J. 3(03), 245–255 (1963)

    Google Scholar 

  29. Aguilera, R.: Naturally Fractured Reservoirs, 1st edn. Pennwell Corp (1980)

  30. Serra, K., Reynolds, A., Raghavan, R.: New pressure transient analysis methods for naturally fractured reservoirs. J. Pet. Technol. 35(12), 2271–2283 (1983)

    Google Scholar 

  31. Hou, J., Qiu, M., He, X., Guo, C., Wei, M., Bai, B.: A dual-porosity-Stokes model and finite element method for coupling dual-porosity flow and free flow. SIAM J. Sci. Comput. 38(5), B710–B739 (2016)

    MathSciNet  MATH  Google Scholar 

  32. Shan, L., Hou, J., Yan, W., Chen, J.: Partitioned time stepping method for a dual-porosity-Stokes model. J. Sci. Comput. 79(1), 389–413 (2019)

    MathSciNet  MATH  Google Scholar 

  33. Mahbub, M., He, X., Nasu, N., Qiu, C., Zheng, H.: Coupled and decoupled stabilized mixed finite element methods for nonstationary dual-porosity-Stokes fluid flow model. Int. J. Numer. Methods Eng. 120(6), 803–833 (2019)

    MathSciNet  Google Scholar 

  34. Mahbub, M., Shi, F., Nasu, N., Wang, Y., Zheng, H.: Mixed stabilized finite element method for the stationary Stokes-dual-permeability fluid flow model. Comput. Methods Appl. Mech. Eng. (2020). https://doi.org/10.1016/j.cma.2019.112616

    Article  MathSciNet  MATH  Google Scholar 

  35. Hou, J., Yan, W., Hu, D., He, Z.: Robin–Robin domain decomposition methods for the dual-porosity-conduit system. Adv. Comput. Math. 47(1), 1–33 (2021)

    MathSciNet  MATH  Google Scholar 

  36. Cao, L., He, Y., Li, J., Mahbub, M.: Decoupled modified characteristic finite element method with different subdomain time steps for nonstationary dual-porosity-Navier–Stokes model. Appl. Numer. Math. 166, 238–271 (2021)

    MathSciNet  MATH  Google Scholar 

  37. Benque, J., Labadie, G., Ronat, J.: A new finite element method for Navier–Stokes equations coupled with a temperature equation. In: Fourth International Symposium on Finite Elements in Flow Problems, NorthHolland, Amsterdam, pp. 295–302 (1982)

  38. Douglas, J., Russell, T.: Numerical methods for convection-dominated diffusion problems based on combining the method of characteristics with finite element or finite difference procedures. SIAM J. Numer. Anal. 19(5), 871–885 (1982)

    MathSciNet  MATH  Google Scholar 

  39. Pironneau, O.: On the transport-diffusion algorithm and its applications to the Navier–Stokes equations. Numer. Math. 38(3), 309–332 (1982)

    MathSciNet  MATH  Google Scholar 

  40. Allievi, A., Bermejo, R.: Finite element modified method of characteristics for the Navier–Stokes equations. Int. J. Numer. Methods Fluids 32(4), 439–463 (2000)

    MATH  Google Scholar 

  41. Chen, Z., Ewing, R., Jiang, Q., Spagnuolo, A.: Error analysis for characteristics-based methods for degenerate parabolic problems. SIAM J. Numer. Anal. 40(4), 1491–1515 (2002)

    MathSciNet  MATH  Google Scholar 

  42. Si, Z., Wang, J., Sun, W.: Unconditional stability and error estimates of modified characteristics FEMs for the Navier–Stokes equations. Numer. Math. 134(1), 139–161 (2016)

    MathSciNet  MATH  Google Scholar 

  43. Wang, J., Si, Z., Sun, W.: A new error analysis of characteristics-mixed FEMs for miscible displacement in porous media. SIAM J. Numer. Anal. 52(6), 3000–3020 (2014)

    MathSciNet  MATH  Google Scholar 

  44. Evans, L.: Partial Differential Equations, 2nd edn. American Mathematical Society (2010)

  45. Cao, Y., Gunzburger, M., Hua, F., Wang, X.: Coupled Stokes–Darcy model with Beavers–Joseph interface boundary condition. Commun. Math. Sci. 8(1), 1–25 (2010)

    MathSciNet  MATH  Google Scholar 

  46. Brenner, S., Scott, R.: The Mathematical Theory of Finite Element Methods, 3rd edn. Springer (2008)

  47. Achdou, Y., Guermond, J.: Convergence analysis of a finite element projection/Lagrange–Galerkin method for the incompressible Navier–Stokes equations. SIAM J. Numer. Anal. 37(3), 799–826 (2000)

    MathSciNet  MATH  Google Scholar 

  48. Heywood, J., Rannacher, R.: Finite-element approximation of the nonstationary Navier–Stokes problem. Part iv: error analysis for second-order time discretization. SIAM J. Numer. Anal. 27(2), 353–384 (1990)

    MathSciNet  MATH  Google Scholar 

  49. Süli, E.: Convergence and nonlinear stability of the Lagrange–Galerkin method for the Navier–Stokes equations. Numer. Math. 53(4), 459–483 (1988)

    MathSciNet  MATH  Google Scholar 

  50. Russell, T.: Time stepping along characteristics with incomplete iteration for a Galerkin approximation of miscible displacement in porous media. SIAM J. Numer. Anal. 22(5), 970–1013 (1985)

    MathSciNet  MATH  Google Scholar 

  51. Hecht, F., Pironneau, O., Ohtsuka, K.: Freefem++. http://www.freefem.org/

  52. Taber, J., Seright, R.: Horizontal injection and production wells for EOR or waterflooding. In: Permian Basin Oil and Gas Recovery Conference, Midland, Texas, March (1992). https://doi.org/10.2118/23952-MS

  53. Li, J., Yao, M., Mahbub, M., Zheng, H.: The efficient rotational pressure-correction schemes for the coupling Stokes/Darcy problem. Comput. Math. Appl. 79(2), 337–353 (2020)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work is partially supported by the Natural Science Foundation of China under Grant No. 11771348 and No. 11771259 and the Major Research and Development Program of China under Grant No. 2016YFB0200901. It is also supported by the China Scholarship Council Grant 202006280440 and the special support program to develop innovative talents in the region of Shaanxi province, innovation team on computationally efficient numerical methods based on new energy problems in Shaanxi province, and innovation team project of Shaanxi Provincial Department of Education (No. 21JP013).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Xi’an Jiaotong University, Xi’an, 710049, China

    Luling Cao & Yinnian He

  2. Department of Mathematics, Shaanxi University of Science and Technology, Xi’an, 710021, China

    Jian Li

Authors
  1. Luling Cao
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yinnian He
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Jian Li
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yinnian He.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cao, L., He, Y. & Li, J. A Parallel Robin–Robin Domain Decomposition Method based on Modified Characteristic FEMs for the Time-Dependent Dual-porosity-Navier–Stokes Model with the Beavers–Joseph Interface Condition. J Sci Comput 90, 16 (2022). https://doi.org/10.1007/s10915-021-01674-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01674-x

Keywords